
Concept explainers
(a)
Particle’s velocity at time
(a)
Answer to Problem 59E
The velocity at
Explanation of Solution
Given information:
Position at time t (sec) of a particle moving along a coordinate axis:
Where,
f is the differentiable function
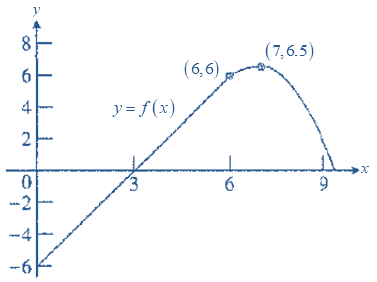
The velocity v of s is s’ .
Such that
Since
The velocity at
From the graph,
Thus,
The velocity at
(b)
Whether the acceleration of particle at time
(b)
Answer to Problem 59E
The acceleration of particle is positive at
Explanation of Solution
Given information:
Position at time t (sec) of a particle moving along a coordinate axis:
Where,
f is the differentiable function
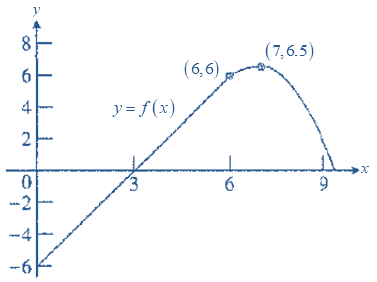
The acceleration of s is s”.
Since
Then
According to FTC (Fundamental Theorem of Calculus),
Second derivative:
That means
From the graph,
The slope of f at 3 is positive.
Thus,
Therefore,
The acceleration of particle is positive at
(c)
Particle’s position at time
(c)
Answer to Problem 59E
Particle’s position at 3 seconds is −9 units.
Explanation of Solution
Given information:
Position at time t (sec) of a particle moving along a coordinate axis:
Where,
f is the differentiable function
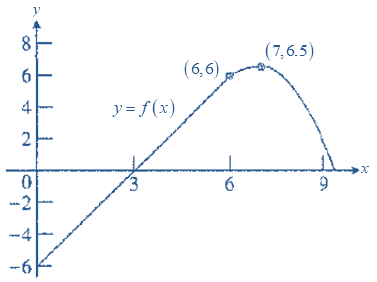
The region between
Then
The position at 3 seconds:
Therefore,
Particle’s position at 3 seconds is −9 units.
(d)
Time during which the particle passes through the origin.
(d)
Answer to Problem 59E
Particle is at origin when
Explanation of Solution
Given information:
Position at time t (sec) of a particle moving along a coordinate axis:
Where,
f is the differentiable function
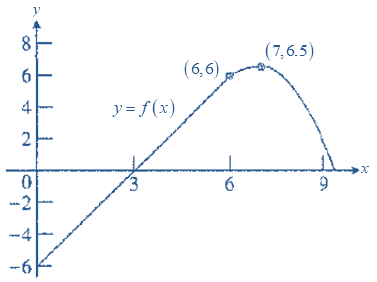
If the particle is at origin,
Then
Since
That means
We need to find some time t where the area between the f and x − axis is 0.
Note that
In the graph, the triangle between 0 and 3 has same area as the triangle between 3 and 6.
For both the triangle,
Base length is 5
And
Height is 6.
Since one triangle is above the x − axis (positive area) and one triangle is below the x − axis (negative axis), the net area is 0 when we add both the triangles.
Thus,
Therefore,
The particle is at origin when
(e)
Approximate the zero value of acceleration.
(e)
Answer to Problem 59E
The acceleration is zero when
Explanation of Solution
Given information:
Position at time t (sec) of a particle moving along a coordinate axis:
Where,
f is the differentiable function
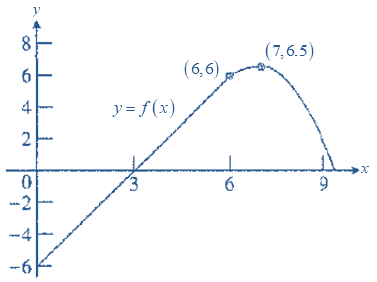
If
Then
The acceleration will be zero.
Thus,
We are required to find the second derivative of s .
Since
Then
According to FTC (Fundamental Theorem of Calculus),
We have
Then
Second derivative:
This implies
If
Then
From the graph,
At
The slope of f is 0.
Thus,
We have
Therefore,
Acceleration is zero at 7 seconds.
(f)
Movement of the particle towards and away from the origin.
(f)
Answer to Problem 59E
The particle moves away from the origin in the positive direction on the interval [0, 3] and t > 6.
The particle moves towards the origin in the negative direction on the interval [3, 6].
Explanation of Solution
Given information:
Position at time t (sec) of a particle moving along a coordinate axis:
Where,
f is the differentiable function
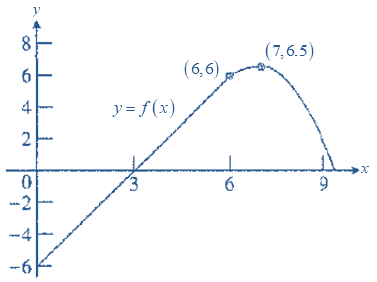
Note that
Such that
Thus,
The particle is at origin at
And
If
The particle is moving towards the right.
If
The particle is moving towards the left.
On the interval [0, 3]:
We have
Thus,
The particle moves away from the origin in the negative direction.
Since it starts at 0 at
On the interval [3, 6]:
We have
And
Thus,
The particle moves towards the origin since it was on the negative coordinate axis and moved to the right.
For
Since
And
Thus,
The particle moves away from the origin in the positive direction since it was on the origin and then moved to the right.
(g)
Side of the origin for the particle at time
(g)
Answer to Problem 59E
The particle lies on positive side of the origin at time
Explanation of Solution
Given information:
Position at time t (sec) of a particle moving along a coordinate axis:
Where,
f is the differentiable function
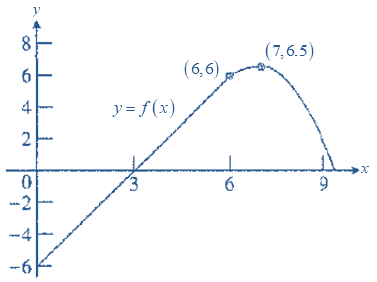
Since the particle starts at the origin,
Then
Also,
The area below the x -axis between
Thus,
Therefore,
The particle lies on the positive side of the origin at 9 seconds.
Chapter 6 Solutions
Calculus: Graphical, Numerical, Algebraic
Additional Math Textbook Solutions
Elementary Statistics
A First Course in Probability (10th Edition)
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Algebra and Trigonometry (6th Edition)
Elementary Statistics (13th Edition)
Elementary Statistics: Picturing the World (7th Edition)
- For each of the following series, determine whether the absolute convergence series test determines absolute convergence or fails. For the ¿th series, if the test is inconclusive then let Mi = 4, while if the test determines absolute convergence let Mi 1 : 2: ∞ Σ(−1)"+¹ sin(2n); n=1 Σ n=1 Σ ((−1)”. COS n² 3+2n4 3: (+ 4: 5 : n=1 ∞ n 2+5n3 ПП n² 2 5+2n3 пп n² Σ(+)+ n=1 ∞ n=1 COS 4 2 3+8n3 П ηπ n- (−1)+1 sin (+727) 5 + 2m³ 4 = 8. Then the value of cos(M₁) + cos(2M2) + cos(3M3) + sin(2M) + sin(M5) is -0.027 -0.621 -1.794 -1.132 -1.498 -4.355 -2.000 2.716arrow_forwardi need help with this question i tried by myself and so i am uploadding the question to be quided with step by step solution and please do not use chat gpt i am trying to learn thank you.arrow_forwardi need help with this question i tried by myself and so i am uploadding the question to be quided with step by step solution and please do not use chat gpt i am trying to learn thank you.arrow_forward
- 1. 3 2 fx=14x²-15x²-9x- 2arrow_forwardNo it is not a graded assignment, its a review question but i only have the final answer not the working or explanationarrow_forwardClass, the class silues, and the class notes, whether the series does alternate and the absolute values of the terms decrease), and if the test does apply, determine whether the series converges or diverges. For the ith series, if the test does not apply the let Mi = 2, while if the test determines divergence then M¿ = 4, and if it determines convergence then M¿ = 8. 1: 2: 3 : 4: 5 : ∞ n=1 ∞ (−1)n+1. Σ(-1) +1 n=1 ∞ п 3m² +2 Σ(-1)+1 sin(2n). n=1 ∞ 2n² + 2n +3 4n2 +6 1 e-n + n² 3n23n+1 9n² +3 In(n + 1) 2n+1 Σ(-1) +1 n=1 ∞ Σ(-1)". n=1 Then the value of cos(M₁) + cos(2M2) + cos(3M3) + sin(2M4) + sin(M5) is 1.715 0.902 0.930 -1.647 -0.057 ● 2.013 1.141 4.274arrow_forward
- 3. FCX14) = x²+3xx-y3 +.arrow_forwardA cylindrical chemical storage tank with a capacity of 950m3 is going to be constructed in a warehouse that is 11m by 14m with a height of 10m. The specifications call for the case to be made of sheet metal that costs $90/m2, the top to be made from sheet metal that costs $45/m2 and the wall to be made of sheet metal that costs $80/m2. If you want to minimize the cost to make the storage house, how much would you end up spending to build the tank?arrow_forwardCalculate the max value of the directional derivatearrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





