
Concept explainers
(a)
The upper estimate for the speed when t=5 using LRAM5
(a)
Answer to Problem 26E
The upper estimate for the speed when t=5 using LRAM5 is
Explanation of Solution
Given information:
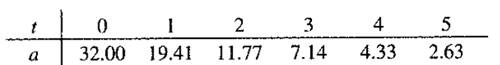
Formula used:
The left-hand point rectangle approximation method (LRAM) is used.
Calculation:
For left-hand point rectangle approximation method (LRAM), area is divided into rectangles. Total time is divided into equal intervals and at left-hand point of interval calculates the velocity which is equal to height of rectangle then calculates the area or small rectangles then adds all the area. Total area gives the Speed. There is no need to measure height because we have recorded data.
Use LRAM with 5 equal subintervals. Take the value at left-end point of interval and add all the values then multiply with the length of subinterval which is unity.
Conclusion:
The upper estimate for the speed when t=5 using LRAM5 is
(a)
The lower estimate for the speed when t=5 using RRAM5
(a)
Answer to Problem 26E
The lower estimate for the speed when t=5 using RRAM5 is
Explanation of Solution
Given information:
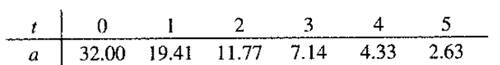
Formula used:
The right-hand point rectangle approximation method (RRAM) is used.
< p>Calculation:For right-hand point rectangle approximation method (RRAM), area is divided into rectangles. Total time is divided into equal intervals and at right-hand point of interval calculates the velocity which is equal to height of rectangle then calculates the area or small rectangles then adds all the area. Total area gives the Speed. There is no need to measure height because we have recorded data.
Use RRAM with 5 equal subintervals. Take the value at right-end point of interval and add all the values then multiply with the length of subinterval which is unity.
Conclusion:
The lower estimate for the speed when t=5 using RRAM5 is
(c)
The upper estimate for the distance fallen when t=3.
(c)
Answer to Problem 26E
The distance fallen up to 3 second is 146.59 feet
Explanation of Solution
Given information:
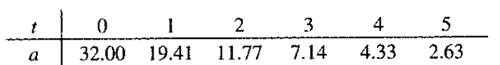
Formula used:
The upper estimate formula is used.
Calculation:
Upper estimate for first second
Upper estimate for next second
Upper estimate for third second
So,
Upper estimate for the distance fallen up to 3 second
Conclusion:
The distance fallen up to 3 second is 146.59 feet
Chapter 6 Solutions
Calculus: Graphical, Numerical, Algebraic
Additional Math Textbook Solutions
A First Course in Probability (10th Edition)
Thinking Mathematically (6th Edition)
Elementary Statistics: Picturing the World (7th Edition)
Elementary Statistics
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Algebra and Trigonometry (6th Edition)
- The spread of an infectious disease is often modeled using the following autonomous differential equation: dI - - BI(N − I) − MI, dt where I is the number of infected people, N is the total size of the population being modeled, ẞ is a constant determining the rate of transmission, and μ is the rate at which people recover from infection. Close a) (5 points) Suppose ẞ = 0.01, N = 1000, and µ = 2. Find all equilibria. b) (5 points) For the equilbria in part a), determine whether each is stable or unstable. c) (3 points) Suppose ƒ(I) = d. Draw a phase plot of f against I. (You can use Wolfram Alpha or Desmos to plot the function, or draw the dt function by hand.) Identify the equilibria as stable or unstable in the graph. d) (2 points) Explain the biological meaning of these equilibria being stable or unstable.arrow_forwardFind the indefinite integral. Check Answer: 7x 4 + 1x dxarrow_forwardshow sketcharrow_forward
- Find the indefinite integral. Check Answer: 7x 4 + 1x dxarrow_forwardQuestion 1: Evaluate the following indefinite integrals. a) (5 points) sin(2x) 1 + cos² (x) dx b) (5 points) t(2t+5)³ dt c) (5 points) √ (In(v²)+1) 4 -dv ขarrow_forwardFind the indefinite integral. Check Answer: In(5x) dx xarrow_forward
- Find the indefinite integral. Check Answer: 7x 4 + 1x dxarrow_forwardHere is a region R in Quadrant I. y 2.0 T 1.5 1.0 0.5 0.0 + 55 0.0 0.5 1.0 1.5 2.0 X It is bounded by y = x¹/3, y = 1, and x = 0. We want to evaluate this double integral. ONLY ONE order of integration will work. Good luck! The dA =???arrow_forward43–46. Directions of change Consider the following functions f and points P. Sketch the xy-plane showing P and the level curve through P. Indicate (as in Figure 15.52) the directions of maximum increase, maximum decrease, and no change for f. ■ 45. f(x, y) = x² + xy + y² + 7; P(−3, 3)arrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





