HEART OF MATHEMATICS
4th Edition
ISBN: 9781119760061
Author: Burger
Publisher: WILEY
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 6.4, Problem 4MS
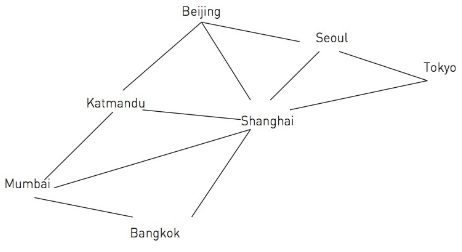
Hamiltonian holiday (S). You are interning for a nonprofit organization that works with similar agencies in Asia. Your boss is planning to visit the cities in the graph below. He knows you’ve studied graph theory and so asks you to find a Hamiltonian circuit that starts in Beijing, travels to each of the other cities only once, and returns to Beijing. Is there more than one such route?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Q3
3 Points
1 0 2
4
0 6
01 5
-
-1
0
10
00 0
0
1 1
The reduced row echelon form of the augmented matrix of a linear system is given above.
Describe the solution set of this linear system in parametric vector form.
Please select file(s) Select file(s)
Save Answer
3. Suppose you have 12 professors in a department and you have to chose members of departmental
committees.
(a) How many ways can you pick members for one 4 people committee?
(b) How many ways can you pick members for two different 4 people committees if no-one can serve
on both committees?
(c) How many ways can you pick members for two different 4 people committees if people can serve
on both committees?
(d) How about if you need members for a 4 person, a 5 person, and a 3 person committee, people
cannot serve on more than one committee?
(e) How about if you need members for a 4 person, a 5 person, and a 3 person committee, people can
serve on more than one committee?
(f) How about if you need members for a 4 person, a 5 person, and a 3 person committee, people
cannot serve on more than one committee, and the first person chosen for each committee serves
as chair?
Peggy conducted a study to identify the randomness of rainy days in fall. For 15 days, she recorded whether it rained that day or not. They denoted a rainy day with the letter R, a day without rain with the letter N.
R N N R R N N R R N N R R R R
Test the sequence for randomness. Use .
Chapter 6 Solutions
HEART OF MATHEMATICS
Ch. 6.1 - Map maker, map maker make me a graph. Represent...Ch. 6.1 - Unabridged list. Represent cach landmass from...Ch. 6.1 - Will the walk work? Does your graph from...Ch. 6.1 - Walk around the house. Is it possibel to traverse...Ch. 6.1 - Walk the line. Does this graph above have an Euler...Ch. 6.1 - Walkabout. Does this graph have an Euler circuit?...Ch. 6.1 - Linking the loops. In this map, the following...Ch. 6.1 - Scenic drive. (S) Here is a map of Rockystone...Ch. 6.1 - Under-edged. (H) Does this graph have an Euler...Ch. 6.1 - No man is an island. The country of Pelago...
Ch. 6.1 - Path-o-rama. For each graph below, determine if...Ch. 6.1 - Walk around the block. Create a graph of the...Ch. 6.1 - Walking the dogs. Your dogs, Abbey and Bear, love...Ch. 6.1 - Delivery query. The next time you see a postal...Ch. 6.1 - Snow job. (ExH) Shown here is a map of the tiny...Ch. 6.1 - Special delivery. (ExH) Julia is the letter...Ch. 6.1 - Draw this old house. Suppose you wanted to trace...Ch. 6.1 - Path of no return. Consider this map showing a...Ch. 6.1 - Without a trace. Is it possibel to trace out...Ch. 6.1 - New Euler. In the three previous Mindscapes, you...Ch. 6.1 - New edge—new circuit. Look at the graph for...Ch. 6.1 - New edge—new path. Review your work for...Ch. 6.1 - Path to proof. Suppose you have a connected graph...Ch. 6.1 - No Euler no how. Look at graph (a) for Mindscape...Ch. 6.1 - Degree day. (S) For cach graph below, determine...Ch. 6.1 - degrees of proof. Review your work for Mindscape...Ch. 6.1 - Degrees in sequence. Can you draw a graph that has...Ch. 6.1 - Even Steven. Review your work in Mindscape 28 to...Ch. 6.1 - Little League lesson. (H) You are in charge of...Ch. 6.1 - With a group of folks. In a small group, discuss...Ch. 6.1 - Power beyond the mathematics. Provide several...Ch. 6.1 - Here we celebrate the power of algebra as a...Ch. 6.1 - Here we celebrate the power of algebra as a...Ch. 6.1 - Here we celebrate the power of algebra as a...Ch. 6.1 - Here we celebrate the power of algebra as a...Ch. 6.1 - Here we celebrate the power of algebra as a...Ch. 6.2 - What a character! What expression gives the Euler...Ch. 6.2 - Count, then verify. What are the values of V, E,...Ch. 6.2 - Sneeze, then verify. Look at an unopened tissue...Ch. 6.2 - Blow, then verify. Inflate a ballon and use a...Ch. 6.2 - Add one. Find the values V, E, and F for the graph...Ch. 6.2 - Bowling. What is the Euler Characteristic of the...Ch. 6.2 - Making change. We begin with the graph pictured at...Ch. 6.2 - Making a point. Take a connected graph and add a...Ch. 6.2 - On the edge (H). Is it possible to add an edge to...Ch. 6.2 - Soap films. Consider the following sequence of...Ch. 6.2 - Dualing. What is the relationship between the...Ch. 6.2 - Prob. 12MSCh. 6.2 - Lots of separation. Suppose we are told that a...Ch. 6.2 - Prob. 14MSCh. 6.2 - Psychic readings. Someone is thinking of a...Ch. 6.2 - Prob. 16MSCh. 6.2 - Prob. 17MSCh. 6.2 - Circular reasoning. Create a connected graph as...Ch. 6.2 - Prob. 19MSCh. 6.2 - More circles. Consider the sphere described in...Ch. 6.2 - In the rough (S). Count the number of facets,...Ch. 6.2 - Cutting corners (H). The following collection of...Ch. 6.2 - Stellar. The following collection of pictures...Ch. 6.2 - A torus graph (ExH). The Euler Characteristic...Ch. 6.2 - Regular unfolding. Each graph below represents...Ch. 6.2 - A tale of two graphs. Suppose we draw a graph that...Ch. 6.2 - Two graph conjectures (S). Can you conjecture a...Ch. 6.2 - Lots of graphs conjecture. Can you conjecture a...Ch. 6.2 - Torus count. Three hollowed, triangular prisms...Ch. 6.2 - Torus two count (H). Carefully count the number of...Ch. 6.2 - Torus many count. Using the preceding calculations...Ch. 6.2 - Prob. 32MSCh. 6.2 - Tell the truth. Someone said that she made a...Ch. 6.2 - No sphere. Suppose we have a sphere built out of...Ch. 6.2 - Soccer ball. A soccer ball is made of pentagons...Ch. 6.2 - Klein bottle. Using the diagram here for building...Ch. 6.2 - Not many neighbors. Show that every map has at...Ch. 6.2 - Infinite edges. Suppose we consider a conn ected...Ch. 6.2 - Here we celebrate the power of algebra as a...Ch. 6.2 - Prob. 44MSCh. 6.2 - Prob. 45MSCh. 6.2 - Here we celebrate the power of algebra as a...Ch. 6.2 - Here we celebrate the power of algebra as a...Ch. 6.3 - Dont be cross. Here is a drawing of a graph with...Ch. 6.3 - De Plane! De Plane! (S) Is the graph given in...Ch. 6.3 - Countdown (H). For the graph drawing shown, count...Ch. 6.3 - Prob. 4MSCh. 6.3 - Criss-Cross. Is it possible to redraw the graph...Ch. 6.3 - Dont cross in the edge. Each of the graphs drawn...Ch. 6.3 - Hot crossed buns. Each of the graphs drawn below...Ch. 6.3 - Prob. 8MSCh. 6.3 - Spider on a mirror. Is it possible to redraw the...Ch. 6.3 - One more vertex. The graph here is drawn to show...Ch. 6.3 - Yet one more vertex (H). The graph shown is drawn...Ch. 6.3 - Familiar freckles. Is it possible to redraw the...Ch. 6.3 - Remind you of anyone you know? Is it possible to...Ch. 6.3 - Final countdown. For this graph drawing, count the...Ch. 6.3 - Euler check-up. Use your answer to the previous...Ch. 6.3 - Euler second opinion. For the graph drawing shown...Ch. 6.3 - Prob. 17MSCh. 6.3 - Prob. 18MSCh. 6.3 - A colorful museum. This figure shows the floor...Ch. 6.3 - Limit of 5. Start drawing a planar graph. Keep...Ch. 6.3 - Starring the hexagon. Is it possible to redraw...Ch. 6.3 - Prob. 22MSCh. 6.3 - Prob. 23MSCh. 6.3 - Getting greedy. (H) Suppose you are asked to color...Ch. 6.3 - Stingy rather than greedy. By coloring the...Ch. 6.3 - Getting more colorful. Graphs dont have to be...Ch. 6.3 - Prob. 27MSCh. 6.3 - Prob. 28MSCh. 6.3 - Chromatically applied. There are eight radio...Ch. 6.3 - Prob. 30MSCh. 6.3 - Personal perspectives. Write a short essay...Ch. 6.3 - Here we celebrate the power of algebra as a...Ch. 6.3 - Here we celebrate the power of algebra as a...Ch. 6.3 - Prob. 37MSCh. 6.3 - Here we celebrate the power of algebra as a...Ch. 6.3 - Here we celebrate the power of algebra as a...Ch. 6.4 - Up close and personal. Create a graph to model...Ch. 6.4 - Network lookout. Find an examle of a network...Ch. 6.4 - Prob. 3MSCh. 6.4 - Hamiltonian holiday (S). You are interning for a...Ch. 6.4 - Home style. Create a graph to model the rooms in...Ch. 6.4 - Six degrees or less. Suppose this graph is a model...Ch. 6.4 - Degrees of you. Find ten willing friends or...Ch. 6.4 - Campus shortcut. Find a map of your campus and...Ch. 6.4 - Arborist lesson. Which of the graphs below are...Ch. 6.4 - Prob. 10MSCh. 6.4 - Prob. 11MSCh. 6.4 - Prob. 12MSCh. 6.4 - Prob. 13MSCh. 6.4 - Prob. 14MSCh. 6.4 - Prob. 15MSCh. 6.4 - Hamilton Study. Look at the graph you drew to...Ch. 6.4 - Business trip redux. Look back in the section and...Ch. 6.4 - Handling Hamiltons. For each graph below, find a...Ch. 6.4 - Road trip. You are checking out gradua te programs...Ch. 6.4 - Back to Hatties trip. Look back in this section...Ch. 6.4 - Solve the Icosian Game. Find a Hamiltonian circuit...Ch. 6.4 - Hunt for Hamilton (S). A large island country has...Ch. 6.4 - Has no Hamilton. Give some characteristics that...Ch. 6.4 - Cubing Hamilton (ExH). Can you find a Hamihonian...Ch. 6.4 - Hamiltonian path. A Hamiltonian path is a path in...Ch. 6.4 - Sorry, no path. Give some characteristics that...Ch. 6.4 - Prob. 27MSCh. 6.4 - Prob. 28MSCh. 6.4 - Prob. 29MSCh. 6.4 - Prob. 30MSCh. 6.4 - Edge count. Look at all the trees you drew in the...Ch. 6.4 - Personal perspecthes. Write a short essay...Ch. 6.4 - Prob. 33MSCh. 6.4 - Prob. 34MSCh. 6.4 - Dollars and cents. Your spanning tree has three...Ch. 6.4 - Adding up. Your spanning tree has four edges with...Ch. 6.4 - Prob. 38MSCh. 6.4 - Vertex search (H). Your graph has a Hamiltonian...Ch. 6.4 - Binary gossip tree. You told a secret to two of...
Additional Math Textbook Solutions
Find more solutions based on key concepts
In Exercises 11-20, express each decimal as a percent.
11. 0.59
Thinking Mathematically (6th Edition)
Fill in each blank so that the resulting statement is true. An equation that expresses a relationship between t...
Algebra and Trigonometry (6th Edition)
Two cards are randomly selected from an ordinary playing deck. What is the probability that they loin, a blackj...
A First Course in Probability (10th Edition)
Drug for Nausea Ondansetron (Zofran) is a drug used by some pregnant women for nausea. There was some concern t...
Introductory Statistics
Testing Hypotheses. In Exercises 13-24, assume that a simple random sample has been selected and test the given...
Elementary Statistics Using The Ti-83/84 Plus Calculator, Books A La Carte Edition (5th Edition)
Finding the Margin of Error In Exercises 33 and 34, use the confidence interval to find the estimated margin of...
Elementary Statistics: Picturing the World (7th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- Consider the grades for the math and history exams for 10 students on a scale from 0 to 12 in the following table. Student Math History 1 4 8 2 5 9 3 7 9 4 12 10 5 10 8 6 8 5 7 9 6 8 9 6 9 11 9 10 7 10 Compute the Spearman correlation coefficient. Round your answer to three decimal places.arrow_forwardQ4 3 Points 1 Let A = 2 3 7 5 11 Give one nontrivial solution X of the homogeneous system Ax = 0. (Your vector x should have explicit numbers as its entries, as opposed to variables/parameters). Show your work for how you found it. Please select file(s) Select file(s) Save Answerarrow_forwardTo compare two elementary schools regarding teaching of reading skills, 12 sets of identical twins were used. In each case, one child was selected at random and sent to school A, and his or her twin was sent to school B. Near the end of fifth grade, an achievement test was given to each child. The results follow: Twin Pair 1 2 3 4 5 6 School A 169 157 115 99 119 113 School B 123 157 112 99 121 122 Twin Pair 7 8 9 10 11 12 School A 120 121 124 145 138 117 School B 153 90 124 140 142 102 Suppose a sign test for matched pairs with a 1% level of significance is used to test the hypothesis that the schools have the same effectiveness in teaching reading skills against the alternate hypothesis that the schools have different levels of effectiveness in teaching reading skills. Let p denote portion of positive signs when the scores of school B are subtracted from the corresponding scores of school…arrow_forward
- A horse trainer teaches horses to jump by using two methods of instruction. Horses being taught by method A have a lead horse that accompanies each jump. Horses being taught by method B have no lead horse. The table shows the number of training sessions required before each horse performed the jumps properly. Method A 25 23 39 29 37 20 Method B 41 21 46 42 24 44 Method A 45 35 27 31 34 49 Method B 26 43 47 32 40 Use a rank-sum test with a5% level of significance to test the claim that there is no difference between the training sessions distributions. If the value of the sample test statistic R, the rank-sum, is 150, calculate the P-value. Round your answer to four decimal places.arrow_forwardA data processing company has a training program for new salespeople. After completing the training program, each trainee is ranked by his or her instructor. After a year of sales, the same class of trainees is again ranked by a company supervisor according to net value of the contracts they have acquired for the company. The results for a random sample of 11 salespeople trained in the last year follow, where x is rank in training class and y is rank in sales after 1 year. Lower ranks mean higher standing in class and higher net sales. Person 1 2 3 4 5 6 x rank 8 11 2 4 5 3 y rank 7 10 1 3 2 4 Person 7 8 9 10 11 x rank 7 9 10 1 6 y rank 8 11 9 6 5 Using a 1% level of significance, test the claim that the relation between x and y is monotone (either increasing or decreasing). Verify that the Spearman rank correlation coefficient . This implies that the P-value lies between 0.002 and 0.01. State…arrow_forwardSand and clay studies were conducted at a site in California. Twelve consecutive depths, each about 15 cm deep, were studied and the following percentages of sand in the soil were recorded. 34.4 27.1 30.8 28.0 32.2 27.6 32.8 25.2 31.4 33.5 24.7 28.4 Converting this sequence of numbers to a sequence of symbols A and B, where A indicates a value above the median and B denotes a value below the median gives ABABABABAABB. Test the sequence for randomness about the median with a 5% level of significance. Verify that the number of runs is 10. What is the upper critical value c2? arrow_forward
- Sand and clay studies were conducted at a site in California. Twelve consecutive depths, each about 15 cm deep, were studied and the following percentages of sand in the soil were recorded. 34.4 27.1 30.8 28.0 32.2 27.6 32.8 25.2 31.4 33.5 24.7 28.4 Converting this sequence of numbers to a sequence of symbols A and B, where A indicates a value above the median and B denotes a value below the median gives ABABABABAABB. Test the sequence for randomness about the median with a 5% level of significance. Verify that the number of runs is 10. What is the upper critical value c2?arrow_forward29% of all college students major in STEM (Science, Technology, Engineering, and Math). If 46 college students are randomly selected, find the probability thata. Exactly 11 of them major in STEM. b. At most 12 of them major in STEM. c. At least 11 of them major in STEM. d. Between 11 and 15 (including 11 and 15) of them major in STEM.arrow_forwardSand and clay studies were conducted at a site in California. Twelve consecutive depths, each about 15 cm deep, were studied and the following percentages of sand in the soil were recorded. 27.3 34.6 30.6 27.8 33.4 31.5 27.3 31.2 32.0 24.7 24.4 28.2 Test this sequence for randomness about the median. Converting this sequence of numbers to a sequence of symbols A and B, where A indicates a value above the median and B denotes a value below the median gives BAABAABAABBB. Verify that the number of runs is 7, the lower critical number is 3, and the upper critical number is 11. Use a 5% level of significance. State the conclusion of the test and interpret your results.arrow_forward
- 29% of all college students major in STEM (Science, Technology, Engineering, and Math). If 46 college students are randomly selected, find the probability thata. Exactly 11 of them major in STEM. b. At most 12 of them major in STEM. c. At least 11 of them major in STEM. d. Between 11 and 15 (including 11 and 15) of them major in STEM.arrow_forward4. Assume that a risk-free money market account is added to the market described in Q3. The continuously compounded rate of return on the money market account is log (1.1). (i) For each given μ, use Lagrange multipliers to determine the proportions (as a function of μ) of wealth invested in the three assets available for the minimum variance portfolio with expected return μ. (ii) Determine the market portfolio in this market and calculate its Sharp ratio.arrow_forward3. A market consists of two risky assets with rates of return R₁ and R2 and no risk-free asset. From market data the following have been estimated: ER₁ = 0.25, ER2 = 0.05, Var R₁ = 0.01, Var R2 = 0.04 and the correlation between R1 and R2 is p = -0.75. (i) Given that an investor is targeting a total expected return of μ = 0.2. What portfolio weights should they choose to meet this goal with minimum portfolio variance? Correct all your calculations up to 4 decimal points. (ii) Determine the global minimum-variance portfolio and the expected return and variance of return of this portfolio (4 d.p.). (iii) Sketch the minimum-variance frontier in the μ-σ² plane and indicate the efficient frontier. (iv) Without further calculation, explain how the minimum variance of the investor's portfolio return will change if the two risky assets were independent.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Elementary AlgebraAlgebraISBN:9780998625713Author:Lynn Marecek, MaryAnne Anthony-SmithPublisher:OpenStax - Rice University
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Elementary Algebra
Algebra
ISBN:9780998625713
Author:Lynn Marecek, MaryAnne Anthony-Smith
Publisher:OpenStax - Rice University
Graph Theory: Euler Paths and Euler Circuits; Author: Mathispower4u;https://www.youtube.com/watch?v=5M-m62qTR-s;License: Standard YouTube License, CC-BY
WALK,TRIAL,CIRCUIT,PATH,CYCLE IN GRAPH THEORY; Author: DIVVELA SRINIVASA RAO;https://www.youtube.com/watch?v=iYVltZtnAik;License: Standard YouTube License, CC-BY