Bundle: Physics For Scientists And Engineers With Modern Physics, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Multi-term
10th Edition
ISBN: 9781337888516
Author: Raymond A. Serway, John W. Jewett
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 6, Problem 31AP
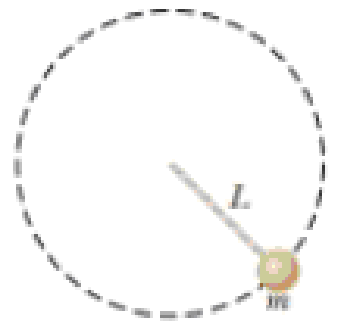
A ball of mass m = 0.275 kg swings in a vertical circular path on a string L = 0.850 in long as in Figure P6.31. (a) What are the forces acting on the ball at any point on the path? (b) Draw force diagrams for the ball when it is at the bottom of the circle and when it is at the top. (c) If its speed is 5.20 m/s at the top of the circle, what is the tension in the string there? (d) If the string breaks when its tension exceeds 22.5 N, what is the maximum speed the ball can have at the bottom before that happens?
Figure P6.31
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
A 0.250 kg ball swings in a vertical circle on the end of a string that is 1.20 m long. The tension in the string is 15.0 N when it is falling toward the lowest point on the circle and the angle between the string and the vertical is 40.0°.
What is the speed of the ball at this moment?
A loop-the-loop is a track that lies in a vertical circle. A 272 kg roller
coaster car is moving at 29 m/s when it enters the bottom of a loop-
the-loop with a radius of 9.1 m. What is the magnitude of the normal
force acting on the car when it is at the top of the loop-the-loop? Air
resistance and friction are negligible
Enter a positive value in units of N with no decimal.
Your cousin Throckmorton skateboards from rest down a curved, frictionless ramp. If we treat Throcky and his skateboard as a particle, he moves through a quarter-circle with radius R = 3.00 m. Throcky and his skateboard have a total mass of 25.0 kg. (a) Find his speed at the bottom of the ramp. (b) Find the normal force that acts on him at the bottom of the curve.
Chapter 6 Solutions
Bundle: Physics For Scientists And Engineers With Modern Physics, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Multi-term
Ch. 6.1 - You are riding on a Ferris wheel that is rotating...Ch. 6.2 - A bead slides at constant speed along a curved...Ch. 6.3 - Consider the passenger in the car making a left...Ch. 6.4 - A basketball and a 2-inch-diameter steel ball,...Ch. 6 - In the Bohr model of the hydrogen atom, an...Ch. 6 - Whenever two Apollo astronauts were on the surface...Ch. 6 - A car initially traveling eastward turns north by...Ch. 6 - A curve in a road forms part of a horizontal...Ch. 6 - In a cyclotron (one type of particle accelerator),...Ch. 6 - Why is the following situation impossible? The...
Ch. 6 - You are working during your summer break as an...Ch. 6 - Prob. 8PCh. 6 - Prob. 9PCh. 6 - A 40.0-kg child swings in a swing supported by two...Ch. 6 - Prob. 11PCh. 6 - One end of a cord is fixed and a small 0.500-kg...Ch. 6 - A roller coaster at the Six Flags Great America...Ch. 6 - An object of mass m = 5.00 kg, attached to a...Ch. 6 - A person stands on a scale in an elevator. As the...Ch. 6 - Review. A student, along with her backpack on the...Ch. 6 - A small container of water is placed on a...Ch. 6 - The mass of a sports car is 1 200 kg. The shape of...Ch. 6 - Review. A window washer pulls a rubber squeegee...Ch. 6 - A small piece of Styrofoam packing material is...Ch. 6 - Prob. 21PCh. 6 - Assume the resistive force acting on a speed...Ch. 6 - You can feel a force of air drag on your hand if...Ch. 6 - A car travels clockwise at constant speed around a...Ch. 6 - A string under a tension of 50.0 N is used to...Ch. 6 - Disturbed by speeding cars outside his workplace,...Ch. 6 - A car of mass m passes over a hump in a road that...Ch. 6 - A childs toy consists of a small wedge that has an...Ch. 6 - A seaplane of total mass m lands on a lake with...Ch. 6 - An object of mass m1 = 4.00 kg is tied to an...Ch. 6 - A ball of mass m = 0.275 kg swings in a vertical...Ch. 6 - Why is the following situation impossible? A...Ch. 6 - The pilot of an airplane executes a loop-the-loop...Ch. 6 - A basin surrounding a drain has the shape of a...Ch. 6 - Review. While learning to drive, you arc in a 1...Ch. 6 - A truck is moving with constant acceleration a up...Ch. 6 - Prob. 37APCh. 6 - A puck of mass m1 is tied to a string and allowed...Ch. 6 - Prob. 39APCh. 6 - Members of a skydiving club were given the...Ch. 6 - A car rounds a banked curve as discussed in...Ch. 6 - Prob. 42APCh. 6 - Review. A piece of putty is initially located at...Ch. 6 - A model airplane of mass 0.750 kg flies with a...Ch. 6 - A 9.00-kg object starting from rest falls through...Ch. 6 - For t 0, an object of mass m experiences no force...Ch. 6 - A golfer tees off from a location precisely at i =...Ch. 6 - A single bead can slide with negligible friction...Ch. 6 - Prob. 49CPCh. 6 - You have a great job working at a major league...
Additional Science Textbook Solutions
Find more solutions based on key concepts
An aluminum calorimeter with a mass of 100 g contains 250 g of water. The calorimeter and water are in thermal ...
Physics for Scientists and Engineers, Technology Update (No access codes included)
1. Rub your hands together vigorously. What happens? Discuss the energy transfers and transformations that take...
College Physics: A Strategic Approach (4th Edition)
Scientific Method.
Glencoe Physics: Principles and Problems, Student Edition
8. The formula ΔU = nCvΔT for the change in the internal energy of a fixed amount of an ideal gas is valid
only...
College Physics (10th Edition)
an exact quantity that people agree to use to compare measurements.
Glencoe Physical Science 2012 Student Edition (Glencoe Science) (McGraw-Hill Education)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- Instead of moving back and forth, a conical pendulum moves in a circle at constant speed as its string traces out a cone (Fig. P6.68). One such pendulum is constructed with a string of length L = 12.0cm and bob of mass 0.210 kg. The string makes an angle = 7.00 with the vertical, a. What is the radial acceleration of the bob? b. What are the horizontal and vertical components of the tension force exerted by the string on the bob?arrow_forwardA car of mass m passes over a hump in a road that follows the arc of a circle of radius R as shown. (a) If the car travels at a speed υ, what force does the road exert on the car as the car passes the highest point of the hump? (b) What If? What is the maximum speed the car can have without losing contact with the road as it passes this highest point?arrow_forwardA 0.5 kg object moves in a horizontal circular track with a radius of 2.5 m. An external force of 3.0 N, acting always tangent to the track, causes the object to speed up as it goes around. If it starts from rest, its speed at the end of one revolution is:arrow_forward
- small block with mass 0.0500 kg slides in a vertical circle of radius R = 0.800 m on the inside of a circular track. There is no friction between the track and the block. At the bottom of the block’s path, the normal force the track exerts on the block has magnitude 3.40 N. What is the magnitude of the normal force that the track exerts on the block when it is at the top of its path?arrow_forwardA roller coaster at an amusement park has a dip that bottoms out in a vertical circle of radius r. A passenger feels the seat of the car pushing upward on her with a force equal to four times her weight as she goes through the dip. If r = 16.5 m, how fast is the roller coaster traveling at the bottom of the dip?arrow_forwardPlease Asaparrow_forward
- A 3.00-kg block is placed at the top of a track consisting of two frictionless quarter circles of radius ?=3.00 m connected by a 5.00-m-long, straight, horizontal surface as shown in the figure. The coefficient of kinetic friction between the block and the horizontal surface is ?k=0.100. The block is released from rest. What maximum vertical height ? does the block reach on the right‑hand section of the track? y = ? (in m)arrow_forwardA roller coaster car passes the top of an 8 m radius vertical loop. At this point the normal force exerted on the passengers by the seat is ¼ their weight. Find the speed of the roller coaster car at this point. Group of answer choices 7 m/s 15 m/s 13 m/s 10 m/sarrow_forwardSuppose that a 1800 kg car passes over a bump in a roadway that follows the arc of a circle of radius 21.4 m as in the figure below. (a) What force does the road exert on the car as the car passes the highest point of the bump if the car travels at 31.0 km/h? (Neglect friction.) (b) What is the maximum speed the car can have without losing contact with the road as it passes this highest point? km/harrow_forward
- Disturbed by speeding cars outside his workplace, Nobel laureate Arthur Holly Compton designed a speed bump (called the "Holly hump") and had it installed. Suppose a 1 800-kg car passes over a hump in a roadway that follows the arc of a circle of radius 19.6 m (a) If the car travels at 25.0 km/h what force does the road exert on the car as the car passes the highest point of the hump? magnitude N direction (b) What is the maximum speed the car can have without losing contact with the road as it passes this highest point?arrow_forwardAnd don't worry about whether the passengers would actually survive the trip. They are lightweight crash test dummies. NTC A cart of mass m= 347 kg is going around a circular loop-the-loop. There is no motor. The cart moves only under the influence of gravity. Ignore friction and let g = 9.81 meters per second squared. The loop has a radius of r meters. When the cart is at the top of the loop, the normal force on the cart (exerted by the track) pushes down on the cart with a force of N = 1,330 newtons. When it is at the bottom of the loop, the cart has a speed of 30.7 meters per second. What is the radius of the loop (in units of meters)?arrow_forwardA father places his 20 kg child on a 5.0 kg cart, to which a 2.0 m rope is attached. He then holds the rope and spins the cart in a circle around him, keeping the rope parallel to the ground. If the Tension in the rope is 100 N, what is the cart’s speed v? Neglect friction.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Newton's First Law of Motion: Mass and Inertia; Author: Professor Dave explains;https://www.youtube.com/watch?v=1XSyyjcEHo0;License: Standard YouTube License, CC-BY