Bundle: Physics For Scientists And Engineers With Modern Physics, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Multi-term
10th Edition
ISBN: 9781337888516
Author: Raymond A. Serway, John W. Jewett
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 6, Problem 7P
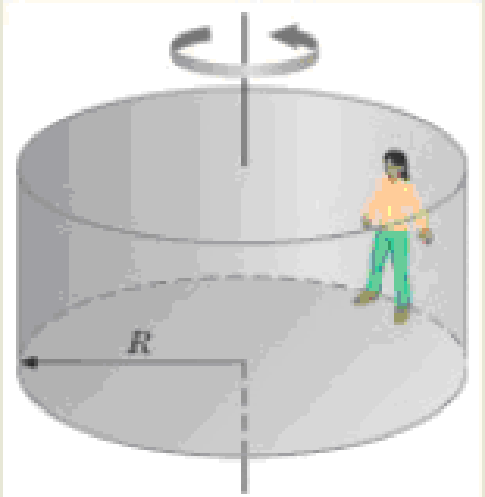
You are working during your summer break as an amusement park ride operator. The ride you are controlling consists of a large vertical cylinder that spins about its axis fast enough that any person inside is held up against the wall when the floor drops away (Fig. P6.7). The coefficient of static friction between a person of mass m and the wall is μs, and the radius of the cylinder is R. You are rotating the ride with an angular speed ω suggested by your supervisor. (a) Suppose a very heavy person enters the ride. Do you need to increase the angular speed so that this person will not slide down the wall? (b) Suppose someone enters the ride wearing a very slippery satin workout outfit. In this case, do you need to increase the angular speed so that this person will not slide down the wall?
Figure P6.7
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
In a scene from The Avengers (the first one) Black Widow is boosted directly upwards by Captain America, where she then grabs on to a Chitauri speeder that is 15.0 feet above her and hangs on. She is in the air for 1.04 s. A) With what initial velocity was Black Widow launched? 1 m = 3.28 ft B) What was Black Widow’s velocity just before she grabbed the speeder? Assume upwards is the positive direction.
In Dark Souls 3 you can kill the Ancient Wyvern by dropping on its head from above it. Let’s say you jump off the ledge with an initial velocity of 3.86 mph and spend 1.72 s in the air before hitting the wyvern’s head. Assume the gravity is the same as that of Earth and upwards is the positive direction. Also, 1 mile = 1609 m. A) How high up is the the ledge you jumped from as measured from the wyvern’s head? B) What is your velocity when you hit the wyvern?
A) If Yoshi flings himself downwards at 9.76 miles per hour to hit an enemy 10.5 m below him, how fast is Yoshi traveling when he hits the enemy? 1 mile = 1609 m
Chapter 6 Solutions
Bundle: Physics For Scientists And Engineers With Modern Physics, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Multi-term
Ch. 6.1 - You are riding on a Ferris wheel that is rotating...Ch. 6.2 - A bead slides at constant speed along a curved...Ch. 6.3 - Consider the passenger in the car making a left...Ch. 6.4 - A basketball and a 2-inch-diameter steel ball,...Ch. 6 - In the Bohr model of the hydrogen atom, an...Ch. 6 - Whenever two Apollo astronauts were on the surface...Ch. 6 - A car initially traveling eastward turns north by...Ch. 6 - A curve in a road forms part of a horizontal...Ch. 6 - In a cyclotron (one type of particle accelerator),...Ch. 6 - Why is the following situation impossible? The...
Ch. 6 - You are working during your summer break as an...Ch. 6 - Prob. 8PCh. 6 - Prob. 9PCh. 6 - A 40.0-kg child swings in a swing supported by two...Ch. 6 - Prob. 11PCh. 6 - One end of a cord is fixed and a small 0.500-kg...Ch. 6 - A roller coaster at the Six Flags Great America...Ch. 6 - An object of mass m = 5.00 kg, attached to a...Ch. 6 - A person stands on a scale in an elevator. As the...Ch. 6 - Review. A student, along with her backpack on the...Ch. 6 - A small container of water is placed on a...Ch. 6 - The mass of a sports car is 1 200 kg. The shape of...Ch. 6 - Review. A window washer pulls a rubber squeegee...Ch. 6 - A small piece of Styrofoam packing material is...Ch. 6 - Prob. 21PCh. 6 - Assume the resistive force acting on a speed...Ch. 6 - You can feel a force of air drag on your hand if...Ch. 6 - A car travels clockwise at constant speed around a...Ch. 6 - A string under a tension of 50.0 N is used to...Ch. 6 - Disturbed by speeding cars outside his workplace,...Ch. 6 - A car of mass m passes over a hump in a road that...Ch. 6 - A childs toy consists of a small wedge that has an...Ch. 6 - A seaplane of total mass m lands on a lake with...Ch. 6 - An object of mass m1 = 4.00 kg is tied to an...Ch. 6 - A ball of mass m = 0.275 kg swings in a vertical...Ch. 6 - Why is the following situation impossible? A...Ch. 6 - The pilot of an airplane executes a loop-the-loop...Ch. 6 - A basin surrounding a drain has the shape of a...Ch. 6 - Review. While learning to drive, you arc in a 1...Ch. 6 - A truck is moving with constant acceleration a up...Ch. 6 - Prob. 37APCh. 6 - A puck of mass m1 is tied to a string and allowed...Ch. 6 - Prob. 39APCh. 6 - Members of a skydiving club were given the...Ch. 6 - A car rounds a banked curve as discussed in...Ch. 6 - Prob. 42APCh. 6 - Review. A piece of putty is initially located at...Ch. 6 - A model airplane of mass 0.750 kg flies with a...Ch. 6 - A 9.00-kg object starting from rest falls through...Ch. 6 - For t 0, an object of mass m experiences no force...Ch. 6 - A golfer tees off from a location precisely at i =...Ch. 6 - A single bead can slide with negligible friction...Ch. 6 - Prob. 49CPCh. 6 - You have a great job working at a major league...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- Two objects get pushed by the same magnitude of force. One object is 10x more massive. How does the rate of change of momentum for the more massive object compare with the less massive one? Please be able to explain why in terms of a quantitative statement found in the chapter.arrow_forwardA box is dropped on a level conveyor belt that is moving at 4.5 m/s in the +x direction in a shipping facility. The box/belt friction coefficient is 0.15. For what duration will the box slide on the belt? In which direction does the friction force act on the box? How far will the box have moved horizontally by the time it stops sliding along the belt?arrow_forwardNo chatgpt pls will upvotearrow_forward
- No chatgpt pls will upvotearrow_forwardA toy car speeds up at 1.0 m/s2 while rolling down a ramp, and slows down at a rate of 2.0 m/s2 while rolling up the same ramp. What is the slope of the ramp in degrees? Grade in %? The friction coefficient?arrow_forwardPlz solution should be complete No chatgpt pls will upvote .arrow_forward
- A box with friction coefficient of 0.2 rests on a 12 foot long plank of wood. How high (in feet) must one side of the plank be lifted in order for the box to begin to slide?arrow_forwardWhat is a good general rule to follow in order to find the best choice of coordinate system to solve a dynamics problem?arrow_forwardWhat is the meaning of a first order approximation?arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
University Physics Volume 1
Physics
ISBN:9781938168277
Author:William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:OpenStax - Rice University
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
College Physics
Physics
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
What Is Circular Motion? | Physics in Motion; Author: GPB Education;https://www.youtube.com/watch?v=1cL6pHmbQ2c;License: Standard YouTube License, CC-BY