Calculus Early Transcendentals, Binder Ready Version
11th Edition
ISBN: 9781118883822
Author: Howard Anton, Irl C. Bivens, Stephen Davis
Publisher: WILEY
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 5, Problem 66RE
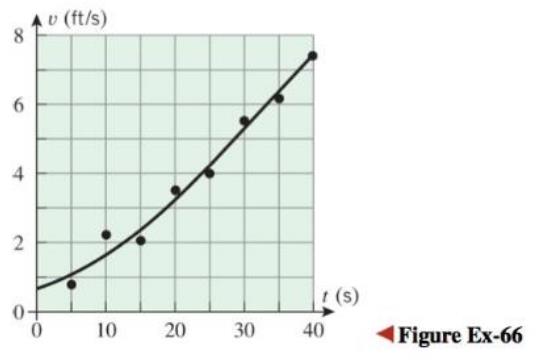
The velocity of a particle moving along an s-axis is measured at
, and the velocity function is modeled by a smooth curve. (The curve and the data points are shown in the accompanying figure.) Use this model in each part.
(a) Does the particle have constant acceleration? Explain your reasoning.
(b) Is there any
(c) Estimate the distance traveled by the particle from time
(d) Estimate the average velocity of the particle over the
time period.
(e) Is the particle ever slowing down during the
(f) Is there sufficient information for you to determine the s-coordinate of the particle at time
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
1. The parametric equations
x = u, y = u cos v, z = usin v, with Ou≤ 2,
0 ≤ v ≤ 2π
represent the cone that is obtained by revolving (about x-axis) the line y = x (for 0 ≤ x ≤2) in
the xy-plane. Answer the following questions.
(A) [50%] Sketch the cone and compute its surface area, which is given by
dS =
[ |
Ər Or
ди მა
×
du dv
with S being the cone surface and D being the projection of S on the uv-plane.
(B) [50%] Suppose that the density of the thin cone is σ(x, y, z) = 0.25x gr/cm². Compute the
total mass of the cone.
The value of sin (2V · F) at x = 3, y = 3, z = −4, where F
-0.592
-0.724
0.661
-0.113
-0.822
-0.313
0.171
0.427
=
(-2x² + -4,2yz − x − 3, −5xz - 2yz), is
The correct answer is C
Could you show me why
Chapter 5 Solutions
Calculus Early Transcendentals, Binder Ready Version
Ch. 5.1 - Let R denote the region below the graph of fx=1x2...Ch. 5.1 - Suppose that when the area A between the graph of...Ch. 5.1 - The area under the graph of y=x2 over the interval...Ch. 5.1 - Find a formula for the area Ax between the graph...Ch. 5.1 - The area under the graph y=fx over the interval...Ch. 5.1 - Prob. 1ESCh. 5.1 - Prob. 2ESCh. 5.1 - Prob. 3ESCh. 5.1 - Prob. 4ESCh. 5.1 - Prob. 5ES
Ch. 5.1 - Estimate the area between the graph of the...Ch. 5.1 - Prob. 7ESCh. 5.1 - Prob. 8ESCh. 5.1 - Prob. 9ESCh. 5.1 - Prob. 10ESCh. 5.1 - Estimate the area between the graph of the...Ch. 5.1 - Prob. 12ESCh. 5.1 - Prob. 13ESCh. 5.1 - Prob. 14ESCh. 5.1 - Prob. 15ESCh. 5.1 - Prob. 16ESCh. 5.1 - Prob. 17ESCh. 5.1 - Prob. 18ESCh. 5.1 - Determine whether the statement is true or false....Ch. 5.1 - Determine whether the statement is true or false....Ch. 5.1 - Determine whether the statement is true or false....Ch. 5.1 - Determine whether the statement is true or false....Ch. 5.1 - Explain how to use the formula for found in the...Ch. 5.1 - Repeat Exercise for the interval .
Ch. 5.1 - Let A denote the area between the graph of fx=x...Ch. 5.1 - Let A denote the area between the graph of fx=1/x...Ch. 5.1 - The area Ax under the graph of f and over the...Ch. 5.1 - The area Ax under the graph of f and over the...Ch. 5.2 - A function is an antiderivative of a function on...Ch. 5.2 - Write an equivalent integration formula for each...Ch. 5.2 - Evaluate the integrals.
(a)
(b)
Ch. 5.2 - The graph of y=x2+x is an integral curve for the...Ch. 5.2 - A slope field for the differential equation...Ch. 5.2 - In each part, confirm that the formula is correct,...Ch. 5.2 - In each part, confirm that the stated formula is...Ch. 5.2 - What is a constant of integration? Why does an...Ch. 5.2 - What is an integral curve of a function f ? How...Ch. 5.2 - Find the derivative and state a corresponding...Ch. 5.2 - Find the derivative and state a corresponding...Ch. 5.2 - Find the derivative and state a corresponding...Ch. 5.2 - Find the derivative and state a corresponding...Ch. 5.2 - Evaluate the integral by rewriting the integrand...Ch. 5.2 - Evaluate the integral by rewriting the integrand...Ch. 5.2 - Evaluate each integral by applying Theorem 5.2.3...Ch. 5.2 - Evaluate each integral by applying Theorem 5.2.3...Ch. 5.2 - Evaluate each integral by applying Theorem 5.2.3...Ch. 5.2 - Evaluate each integral by applying Theorem 5.2.3...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral and check your answer by...Ch. 5.2 - Evaluate the integral 11+sinxdx by multiplying the...Ch. 5.2 - Use the double-angle formula cos2x=2cos2x1 to...Ch. 5.2 - True-False Determine whether the statement is true...Ch. 5.2 - True-False Determine whether the statement is true...Ch. 5.2 - True-False Determine whether the statement is true...Ch. 5.2 - True-False Determine whether the statement is true...Ch. 5.2 - Use a graphing utility to generate some...Ch. 5.2 - Use a graphing utility to generate some...Ch. 5.2 - Solve the initial-value problems. (a) dydx=x3,y1=2...Ch. 5.2 - Solve the initial-value problems. (a)...Ch. 5.2 - Solve the initial-value problems. (a)...Ch. 5.2 - Solve the initial-value problems. (a)...Ch. 5.2 - A particle moves along an s-axis with position...Ch. 5.2 - A particle moves along an s-axis with position...Ch. 5.2 - A particle moves along an s-axis with position...Ch. 5.2 - A particle moves along an s-axis with position...Ch. 5.2 - Find the general form of a function whose second...Ch. 5.2 - Find a function f such that fx=x+cosx and such...Ch. 5.2 - Find an equation of the curve that satisfies the...Ch. 5.2 - Find an equation of the curve that satisfies the...Ch. 5.2 - Find an equation of the curve that satisfies the...Ch. 5.2 - Find an equation of the curve that satisfies the...Ch. 5.2 - Find an equation of the curve that satisfies the...Ch. 5.2 - In each part, use a CAS to solve the initial-value...Ch. 5.2 - (a) Use a graphing utility to generate a slope...Ch. 5.2 - (a) Use a graphing utility to generate a slope...Ch. 5.2 - The given slope field figure corresponds to one of...Ch. 5.2 - The given slope field figure corresponds to one of...Ch. 5.2 - The given slope field figure corresponds to one of...Ch. 5.2 - The given slope field figure corresponds to one of...Ch. 5.2 - (a) Show that Fx=tan1x and Gx=tan11/x differ by a...Ch. 5.2 - Let F and G be the functions defined by...Ch. 5.2 - Use a trigonometric identity to evaluate the...Ch. 5.2 - Use a trigonometric identity to evaluate the...Ch. 5.2 - Use the identities cos2=12sin2=2cos21 to help...Ch. 5.2 - Recall that ddxsec1x=1xx21 Use this to verify...Ch. 5.2 - The speed of sound in air at 0C (or 273K on the...Ch. 5.2 - The time t between tosses of a juggling ball is a...Ch. 5.2 - Suppose that a uniform metal rod 50cm long is...Ch. 5.2 - Writing What is an initial-value problem? Describe...Ch. 5.2 - Writing What is a slope field? How are slope...Ch. 5.3 - Indicate the u- substitution. (a) 3x21+x325x=u25du...Ch. 5.3 - Supply the missing integrand corresponding to the...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Evaluate the integrals using the indicated...Ch. 5.3 - Explain the connection between the chain rule for...Ch. 5.3 - Explain how the substitution u=ax+b helps to...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate the integrals using appropriate...Ch. 5.3 - Evaluate each integral by first modifying the form...Ch. 5.3 - Evaluate each integral by first modifying the form...Ch. 5.3 - Evaluate each integral by first modifying the form...Ch. 5.3 - Evaluate each integral by first modifying the form...Ch. 5.3 - Evaluate the integrals with the aid of Formulas...Ch. 5.3 - Evaluate the integrals with the aid of Formulas...Ch. 5.3 - Evaluate the integrals assuming that n is a...Ch. 5.3 - Evaluate the integrals assuming that n is a...Ch. 5.3 - Evaluate the integrals assuming that n is a...Ch. 5.3 - (a) Evaluate the integral sinxcosxdx by two...Ch. 5.3 - (a) Evaluate the integral 5x12dx by two methods:...Ch. 5.3 - Solve the initial-value problems. dydx=5x+1,y3=2Ch. 5.3 - Solve the initial-value problems....Ch. 5.3 - Solve the initial-value problems. dydt=e2t,y0=6Ch. 5.3 - Solve the initial-value problems....Ch. 5.3 - (a) Evaluate x/x2+1dx . (b) Use a graphing utility...Ch. 5.3 - (a) Evaluate x/x2+1dx . (b) Use a graphing utility...Ch. 5.3 - Find a function f such that the slope of the...Ch. 5.3 - A population of minnows in a lake is estimated to...Ch. 5.3 - Let yt denote the number of E . coli cells in a...Ch. 5.3 - Derive integration Formula 6 .Ch. 5.3 - Derive integration Formula 7 .Ch. 5.4 - (a) Write the sum in two ways:...Ch. 5.4 - Prob. 2QCECh. 5.4 - Divide the interval 1,3 into n=4 subintervals of...Ch. 5.4 - Prob. 4QCECh. 5.4 - The right endpoint approximation for the net...Ch. 5.4 - Evaluate. (a) k=13k3 (b) j=263j1 (c) i=41i2i (d)...Ch. 5.4 - Evaluate. (a) k=14ksink2 (b) j=051j (c) i=7202 (d)...Ch. 5.4 - Write each expression in sigma notation but do not...Ch. 5.4 - Write each expression in sigma notation but do not...Ch. 5.4 - Write each expression in sigma notation but do not...Ch. 5.4 - Write each expression in sigma notation but do not...Ch. 5.4 - Write each expression in sigma notation but do not...Ch. 5.4 - Write each expression in sigma notation but do not...Ch. 5.4 - (a) Express the sum of the even integers from 2 to...Ch. 5.4 - Express in sigma notation. (a) a1a2+a3a4+a5 (b)...Ch. 5.4 - Use Theorem 5.4.2 to evaluate the sums. Check your...Ch. 5.4 - Use Theorem 5.4.2 to evaluate the sums. Check your...Ch. 5.4 - Use Theorem 5.4.2 to evaluate the sums. Check your...Ch. 5.4 - Use Theorem 5.4.2 to evaluate the sums. Check your...Ch. 5.4 - Prob. 15ESCh. 5.4 - Prob. 16ESCh. 5.4 - Express the sums in closed form. k=1n3knCh. 5.4 - Express the sums in closed form.
Ch. 5.4 - Express the sums in closed form.
Ch. 5.4 - Express the sums in closed form. k=1n5n2knCh. 5.4 - True-False Determine whether the statement is true...Ch. 5.4 - True-False Determine whether the statement is true...Ch. 5.4 - True-False Determine whether the statement is true...Ch. 5.4 - True-False Determine whether the statement is true...Ch. 5.4 - Prob. 25ESCh. 5.4 - For a function f that is continuous on a,b ,...Ch. 5.4 - Prob. 27ESCh. 5.4 - Prob. 28ESCh. 5.4 - Prob. 29ESCh. 5.4 - Prob. 30ESCh. 5.4 - Use a calculating utility with summation...Ch. 5.4 - Use a calculating utility with summation...Ch. 5.4 - Prob. 33ESCh. 5.4 - Prob. 34ESCh. 5.4 - Use Definition 5.4.3 with xk* as the right...Ch. 5.4 - Use Definition with as the right endpoint of...Ch. 5.4 - Use Definition 5.4.3 with xk* as the right...Ch. 5.4 - Use Definition 5.4.3 with xk* as the right...Ch. 5.4 - Use Definition 5.4.3 with xk* as the right...Ch. 5.4 - Use Definition with as the right endpoint of...Ch. 5.4 - Use Definition 5.4.3 with xk* as the left endpoint...Ch. 5.4 - Use Definition with as the left endpoint of each...Ch. 5.4 - Use Definition with as the left endpoint of each...Ch. 5.4 - Use Definition with as the left endpoint of each...Ch. 5.4 - Use Definition with as the midpoint of each...Ch. 5.4 - Use Definition with as the midpoint of each...Ch. 5.4 - Use Definition with as the midpoint of each...Ch. 5.4 - Use Definition 5.4.3 with xk* as the midpoint of...Ch. 5.4 - Use Definition 5.4.5 with xk* as the right...Ch. 5.4 - Use Definition 5.4.5 with xk* as the right...Ch. 5.4 - Use Definition 5.4.5 with xk* as the right...Ch. 5.4 - Use Definition with as the right endpoint of...Ch. 5.4 - (a) Show that the area under the graph of y=x3 and...Ch. 5.4 - Find the area between the graph of y=x and the...Ch. 5.4 - An artist wants to create a rough triangular...Ch. 5.4 - An artist wants to create a sculpture by gluing...Ch. 5.4 - Prob. 57ESCh. 5.4 - Prob. 58ESCh. 5.4 - Prob. 59ESCh. 5.4 - Prob. 60ESCh. 5.4 - (a) Show that
(b) Use the result in part (a) to...Ch. 5.4 - (a) Show that
(b) Use the result in part (a) to...Ch. 5.4 - Let denote the arithmetic average of the numbers...Ch. 5.4 - Let
Show that and hence that
(A sum to this...Ch. 5.4 - By writing out the sums, determine whether the...Ch. 5.4 - Which of the following are valid identities? (a)...Ch. 5.4 - Prove parts (a) and (c) of Theorem 5.4.1.Ch. 5.4 - Prove Theorem 5.4.4.Ch. 5.4 - Writing What is net signed areal How does this...Ch. 5.5 - In each part, use the partition of 2,7 in the...Ch. 5.5 - Let fx=2x8 . Use the partition of 2,7 in Quick...Ch. 5.5 - Use the accompanying figure to evaluate 272x8dxCh. 5.5 - Suppose that gx is a function for which...Ch. 5.5 - Find the value of
(a)
(b) .
Ch. 5.5 - Find the value of (a) k=1nfxkx (b) maxxk ....Ch. 5.5 - Find the value of (a) k=1nfxkx (b) maxxk ....Ch. 5.5 - Find the value of (a) k=1nfxkx (b) maxxk ....Ch. 5.5 - Use the given values of and to express the...Ch. 5.5 - Use the given values of and to express the...Ch. 5.5 - Use the given values of and to express the...Ch. 5.5 - Use the given values of a and b to express the...Ch. 5.5 - Use Definition 5.5.1 to express the integrals as...Ch. 5.5 - Use Definition to express the integrals as limits...Ch. 5.5 - Sketch the region whose signed area is represented...Ch. 5.5 - Sketch the region whose signed area is represented...Ch. 5.5 - Sketch the region whose signed area is represented...Ch. 5.5 - Sketch the region whose signed area is represented...Ch. 5.5 - In each part, evaluate the integral, given that...Ch. 5.5 - In each part, evaluate the integral, given that...Ch. 5.5 - Use the areas shown in the figure to find (a)...Ch. 5.5 - Use the areas shown in the figure to find (a)...Ch. 5.5 - Find 12fx+2gxdx if 12fxdx=5 and 12gxdx=3Ch. 5.5 - Find 143fxgxdx if 14fxdx=2 and 14gxdx=10Ch. 5.5 - Find 15fxdx if 01fxdx=2 and 05fxdx=1Ch. 5.5 - Find 32fxdx if 21fxdx=2 and 13fxdx=6Ch. 5.5 - Use Theorem 5.5.4 and appropriate formulas from...Ch. 5.5 - Use Theorem 5.5.4 and appropriate formulas from...Ch. 5.5 - Use Theorem 5.5.4 and appropriate formulas from...Ch. 5.5 - Use Theorem 5.5.4 and appropriate formulas from...Ch. 5.5 - True-False Determine whether the statement is true...Ch. 5.5 - True-False Determine whether the statement is true...Ch. 5.5 - True-False Determine whether the statement is true...Ch. 5.5 - True-False Determine whether the statement is true...Ch. 5.5 - Use Theorem 5.5.6 to determine whether the value...Ch. 5.5 - Use Theorem 5.5.6 to determine whether the value...Ch. 5.5 - Prove that if f is continuous and if mfxM on a,b ,...Ch. 5.5 - Find the maximum and minimum values of x3+2 for...Ch. 5.5 - Evaluate the integrals by completing the square...Ch. 5.5 - Evaluate the integrals by completing the square...Ch. 5.5 - Evaluate the limit by expressing it as a definite...Ch. 5.5 - Evaluate the limit by expressing it as a definite...Ch. 5.5 - Let fx=C be a constant function. (a) Use a formula...Ch. 5.5 - Define a function f on 0,1 by fx=1,0x10,x=0 Use...Ch. 5.5 - It can be shown that every interval contains both...Ch. 5.5 - Define the function f by fx=1x,x00,x=0 It follows...Ch. 5.5 - In each part, use Theorems 5.5.2 and 5.5.8 to...Ch. 5.6 - (a) If Fx is an antiderivative for fx , then...Ch. 5.6 - (a) 023x22xdx= (b) cosxdx= (c) 012ln5exdx= (d)...Ch. 5.6 - For the function fx=3x22x and an interval a,b ,...Ch. 5.6 - The area of an oil spill is increasing at a rate...Ch. 5.6 - In each part, use a definite integral to find the...Ch. 5.6 - In each part, use a definite integral to find the...Ch. 5.6 - In each part, sketch the analogue of Figure 5.6.10...Ch. 5.6 - In each part, sketch the analogue of Figure 5.6.10...Ch. 5.6 - Find the area under the curve y=fx over the stated...Ch. 5.6 - Find the area under the curve y=fx over the stated...Ch. 5.6 - Find the area under the curve y=fx over the stated...Ch. 5.6 - Find the area under the curve y=fx over the stated...Ch. 5.6 - Find the area under the curve y=fx over the stated...Ch. 5.6 - Find the area under the curve y=fx over the stated...Ch. 5.6 - Find all values of x in the stated interval that...Ch. 5.6 - Find all values of x in the stated interval that...Ch. 5.6 - Evaluate the integrals using Part 1 of the...Ch. 5.6 - Evaluate the integrals using Part 1 of the...Ch. 5.6 - Evaluate the integrals using Part of the...Ch. 5.6 - Evaluate the integrals using Part of the...Ch. 5.6 - Evaluate the integrals using Part of the...Ch. 5.6 - Evaluate the integrals using Part 1 of the...Ch. 5.6 - Evaluate the integrals using Part of the...Ch. 5.6 - Evaluate the integrals using Part 1 of the...Ch. 5.6 - Evaluate the integrals using Part of the...Ch. 5.6 - Evaluate the integrals using Part 1 of the...Ch. 5.6 - Evaluate the integrals using Part of the...Ch. 5.6 - Evaluate the integrals using Part 1 of the...Ch. 5.6 - Evaluate the integrals using Part 1 of the...Ch. 5.6 - Evaluate the integrals using Part of the...Ch. 5.6 - Evaluate the integrals using Part 1 of the...Ch. 5.6 - Evaluate the integrals using Part of the...Ch. 5.6 - Evaluate the integrals using Part 1 of the...Ch. 5.6 - Evaluate the integrals using Part 1 of the...Ch. 5.6 - Use Theorem to evaluate the given integrals.
(a)...Ch. 5.6 - Use Theorem 5.5.5 to evaluate the given integrals....Ch. 5.6 - Use Theorem 5.5.5 to evaluate the given integrals....Ch. 5.6 - Use Theorem 5.5.5 to evaluate the given integrals....Ch. 5.6 - A function fx is defined piecewise on an interval....Ch. 5.6 - A function fx is defined piecewise on an interval....Ch. 5.6 - Determine whether the statement is true or false....Ch. 5.6 - Determine whether the statement is true or false....Ch. 5.6 - Determine whether the statement is true or false....Ch. 5.6 - Determine whether the statement is true or false....Ch. 5.6 - Use a calculating utility to find the midpoint...Ch. 5.6 - Prob. 42ESCh. 5.6 - Use a calculating utility to find the midpoint...Ch. 5.6 - Use a calculating utility to find the midpoint...Ch. 5.6 - Sketch the region described and find its area. The...Ch. 5.6 - Sketch the region described and find its...Ch. 5.6 - Sketch the region described and find its...Ch. 5.6 - Sketch the region described and find its...Ch. 5.6 - Sketch the curve and find the total area between...Ch. 5.6 - Sketch the curve and find the total area between...Ch. 5.6 - Sketch the curve and find the total area between...Ch. 5.6 - Sketch the curve and find the total area between...Ch. 5.6 - A student wants to find the area enclosed by the...Ch. 5.6 - Explain why the Fundamental Theorem of Calculus...Ch. 5.6 - (a) If ht is the rate of change of a child’s...Ch. 5.6 - (a) Use a graphing utility to generate the graph...Ch. 5.6 - Define Fx by Fx=1x3t23dt (a) Use Part 2 of the...Ch. 5.6 - Define Fx by Fx=/4xcos2tdt (a) Use Part 2 of the...Ch. 5.6 - Use Part 2 of the Fundamental Theorem of Calculus...Ch. 5.6 - Use Part 2 of the Fundamental Theorem of Calculus...Ch. 5.6 - Use Part 2 of the Fundamental Theorem of Calculus...Ch. 5.6 - Use Part 2 of the Fundamental Theorem of Calculus...Ch. 5.6 - Let Fx=4xt2+9dt . Find (a) F4 (b) F4 (c) F4 .Ch. 5.6 - Let Fx=3xtan1tdt . Find (a) F3 (b) F3 (c) F3 .Ch. 5.6 - Let Fx=0xt3t2+7dt for x+ . (a) Find the value of x...Ch. 5.6 - Use the plotting and numerical integration...Ch. 5.6 - (a) Over what open interval does the formula...Ch. 5.6 - (a) Over what open interval does the formula...Ch. 5.6 - (a) Suppose that a reservoir supplies water to an...Ch. 5.6 - A traffic engineer monitors the rate at which cars...Ch. 5.6 - Suppose that t days after a diagnosed outbreak of...Ch. 5.6 - Suppose that t hours after a pitcher of iced tea...Ch. 5.6 - Evaluate each limit by interpreting it as a...Ch. 5.6 - Evaluate each limit by interpreting it as a...Ch. 5.6 - Prove the Mean-Value Theorem for Integrals...Ch. 5.7 - Suppose that a particle is moving along an s-axis...Ch. 5.7 - Let t denote the velocity function of a particle...Ch. 5.7 - Let t denote the velocity function of a particle...Ch. 5.7 - Based on the free-fall model, from what height...Ch. 5.7 - In each part, the velocity versus time curve is...Ch. 5.7 - Prob. 2ESCh. 5.7 - The accompanying figure shows the acceleration...Ch. 5.7 - The accompanying figure shows the velocity versus...Ch. 5.7 - A particle moves along an s-axis. Use the given...Ch. 5.7 - A particle moves along an s-axis. Use the given...Ch. 5.7 - A particle moves along an s-axis. Use the given...Ch. 5.7 - A particle moves along an s-axis. Use the given...Ch. 5.7 - A particle moves with a velocity of tm/s along an...Ch. 5.7 - A particle moves with a velocity of tm/s along an...Ch. 5.7 - A particle moves with a velocity of tm/s along an...Ch. 5.7 - A particle moves with a velocity of tm/s along an...Ch. 5.7 - A particle moves with acceleration atm/s2 along an...Ch. 5.7 - A particle moves with acceleration atm/s2 along an...Ch. 5.7 - A particle moves with acceleration atm/s2 along an...Ch. 5.7 - A particle moves with acceleration atm/s2 along an...Ch. 5.7 - In each part, use the given information to find...Ch. 5.7 - In each part, use the given information to find...Ch. 5.7 - The velocity of an ant running along the edge of a...Ch. 5.7 - The velocity of a mouse running alongside the...Ch. 5.7 - Suppose that the velocity function of a particle...Ch. 5.7 - Suppose that the acceleration function of a...Ch. 5.7 - True-False Determine whether the statement is true...Ch. 5.7 - True-False Determine whether the statement is true...Ch. 5.7 - True-False Determine whether the statement is true...Ch. 5.7 - True-False Determine whether the statement is true...Ch. 5.7 - For the given velocity function t : (a) Generate...Ch. 5.7 - For the given velocity function t : (a) Generate...Ch. 5.7 - For the given velocity function t : (a) Generate...Ch. 5.7 - For the given velocity function t : (a) Generate...Ch. 5.7 - Suppose that at time t=0 a particle is at the...Ch. 5.7 - In these exercises assume that the object is...Ch. 5.7 - In these exercises assume that the object is...Ch. 5.7 - In these exercises assume that the object is...Ch. 5.7 - In these exercises assume that the object is...Ch. 5.7 - In these exercises assume that the object is...Ch. 5.7 - In these exercises assume that the object is...Ch. 5.7 - In these exercises assume that the object is...Ch. 5.7 - Assume that a free-fall model applies. Solve these...Ch. 5.7 - Assume that a free-fall model applies. Solve these...Ch. 5.7 - Assume that a free-fall model applies. Solve these...Ch. 5.7 - Assume that a free-fall model applies. Solve these...Ch. 5.7 - Assume that a free-fall model applies. Solve these...Ch. 5.7 - Assume that a free-fall model applies. Solve these...Ch. 5.7 - Assume that a free-fall model applies. Solve these...Ch. 5.8 - The arithmetic average of n numbers, a1,a2,,an is ...Ch. 5.8 - If f is continuous on a,b , then the average value...Ch. 5.8 - If f is continuous on a,b , then the Mean-Value...Ch. 5.8 - The average value of fx=4x3 on 1,3 is .Ch. 5.8 - (a) Find fave of fx=2x over 0,4 . (b) Find a point...Ch. 5.8 - (a) Find fave of fx=x2 over 0,2 . (b) Find a point...Ch. 5.8 - Find the average value of the function over the...Ch. 5.8 - Find the average value of the function over the...Ch. 5.8 - Find the average value of the function over the...Ch. 5.8 - Find the average value of the function over the...Ch. 5.8 - Find the average value of the function over the...Ch. 5.8 - Find the average value of the function over the...Ch. 5.8 - Find the average value of the function over the...Ch. 5.8 - Find the average value of the function over the...Ch. 5.8 - Find the average value of the function over the...Ch. 5.8 - Find the average value of the function over the...Ch. 5.8 - Let fx=3x2 (a) Find the arithmetic average of the...Ch. 5.8 - In parts (a)-(d), let fx=1+1/x . (a) Find the...Ch. 5.8 - In each part, the velocity versus time curve is...Ch. 5.8 - Suppose that a particle moving along a line starts...Ch. 5.8 - Suppose that f is a linear function. Using the...Ch. 5.8 - Suppose that a particle moves along a coordinate...Ch. 5.8 - True-False Determine whether the statement is true...Ch. 5.8 - True-False Determine whether the statement is true...Ch. 5.8 - True-False Determine whether the statement is true...Ch. 5.8 - True-False Determine whether the statement is true...Ch. 5.8 - (a) Suppose that the velocity function of a...Ch. 5.8 - (a) Suppose that the acceleration function of a...Ch. 5.8 - Water is run at a constant rate of 1ft3/min to...Ch. 5.8 - (a) The temperature of a 10m long metal bar is 15C...Ch. 5.8 - A traffic engineer monitors the rate at which cars...Ch. 5.8 - Suppose that the value of a yacht in dollars after...Ch. 5.8 - Prob. 29ESCh. 5.8 - For the years 19901999 , the number P of gray...Ch. 5.8 - For the years 20002011 , the number P of gray...Ch. 5.8 - Suppose that a tumor grows at the rate of rt=kt...Ch. 5.9 - Assume that g is continuous on a,b and that f is...Ch. 5.9 - In each part, use the substitution to replace the...Ch. 5.9 - Evaluate the integral by making an appropriate...Ch. 5.9 - Express the integral in terms of the variable u ,...Ch. 5.9 - Express the integral in terms of the variable u ,...Ch. 5.9 - Express the integral in terms of the variable u ,...Ch. 5.9 - Express the integral in terms of the variable u ,...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral two ways: first by...Ch. 5.9 - Evaluate the definite integral by expressing it in...Ch. 5.9 - Evaluate the definite integral by expressing it in...Ch. 5.9 - Evaluate the definite integral by expressing it in...Ch. 5.9 - Evaluate the definite integral by expressing it in...Ch. 5.9 - A particle moves with a velocity of t=sintm/s...Ch. 5.9 - A particle moves with a velocity of t=3cos2tm/s...Ch. 5.9 - Find the area under the curve y=9/x+22 over the...Ch. 5.9 - Prob. 26ESCh. 5.9 - Find the area of the region enclosed by the graphs...Ch. 5.9 - Find the area of the region enclosed by the graphs...Ch. 5.9 - Find the average value of fx=x/5x2+12 over the...Ch. 5.9 - Find the average value of fx=e3x/1+e6x over the...Ch. 5.9 - Evaluate the integrals by any method. 15dx2x1Ch. 5.9 - Evaluate the integrals by any method. 125x1dxCh. 5.9 - Evaluate the integrals by any method. 11x2dxx3+9Ch. 5.9 - Evaluate the integrals by any method....Ch. 5.9 - Evaluate the integrals by any method....Ch. 5.9 - Evaluate the integrals by any method. 12dxx26x+9Ch. 5.9 - Evaluate the integrals by any method....Ch. 5.9 - Evaluate the integrals by any method....Ch. 5.9 - Evaluate the integrals by any method. 05xcosx2dxCh. 5.9 - Evaluate the integrals by any method. 2421xsinxdxCh. 5.9 - Evaluate the integrals by any method. /12/9sec23dCh. 5.9 - Evaluate the integrals by any method. 0/6tan2dCh. 5.9 - Evaluate the integrals by any method. 01y2dy43yCh. 5.9 - Evaluate the integrals by any method. 14xdx5+xCh. 5.9 - Evaluate the integrals by any method. 0edx2x+eCh. 5.9 - Evaluate the integrals by any method. 12xex2dxCh. 5.9 - Evaluate the integrals by any method. 01x43x4dxCh. 5.9 - Evaluate the integrals by any method. 121x4xdxCh. 5.9 - Evaluate the integrals by any method. 01/311+9x2dxCh. 5.9 - Evaluate the integrals by any method. 12x3+x4dxCh. 5.9 - (a) Use a CAS to find the exact value of the...Ch. 5.9 - (a) Use a CAS to find the exact value of the...Ch. 5.9 - (a) Find 01f3x+1dx if 14fxdx=5 . (b) Find 03f3xdx...Ch. 5.9 - Given that m and n are positive integers, show...Ch. 5.9 - Given that n is a positive integers, show that...Ch. 5.9 - Given that n is a positive integer, evaluate the...Ch. 5.9 - Medication can be administered to a patient in...Ch. 5.9 - Medication can be administered to a patient in...Ch. 5.9 - Medication can be administered to a patient in...Ch. 5.9 - Medication can be administered to a patient in...Ch. 5.9 - Suppose that at time t=0 there are 750 bacteria in...Ch. 5.9 - Suppose that a particle moving along a coordinate...Ch. 5.9 - (a) The accompanying table shows the fraction of...Ch. 5.9 - Electricity is supplied to homes in the form of...Ch. 5.9 - Find a positive value of k such that the area...Ch. 5.9 - Use a graphing utility to estimate the value of...Ch. 5.9 - (a) Find the limit limn+k=1nsink/nn by evaluating...Ch. 5.9 - Let I=1111+x2dx (a) Explain why I0 . (b) Show that...Ch. 5.9 - (a) Prove that if f is an odd function, then...Ch. 5.9 - Show that if f and g are continuous functions,...Ch. 5.9 - (a) Let I=0afxfx+faxdx Show that I=a/2 . (b) Use...Ch. 5.9 - Evaluate (a) 11xcosx2dx (b) 0sin8xcos5xdxCh. 5.10 - 11/e1tdt=Ch. 5.10 - Estimate In 2 using Definition 5.10.1 and (a) a...Ch. 5.10 - 1/ln=Ch. 5.10 - A solution to the initial-value problem...Ch. 5.10 - ddx0ex11+t4dt=Ch. 5.10 - Sketch the curve y=1/t , and shade a region under...Ch. 5.10 - Sketch the curve y=1/t , and shade two different...Ch. 5.10 - Given that lna=2 and lnc=5 , find (a) 1ac1tdt (b)...Ch. 5.10 - Given that lna=9 , find (a) 1a1tdt (b) 12a1tdt (c)...Ch. 5.10 - Approximate ln5 using the midpoint approximation...Ch. 5.10 - Approximate ln3 using the midpoint approximation...Ch. 5.10 - Simplify the expression and state the values of x...Ch. 5.10 - (a) Let fx=e2x . Find the simplest exact value of...Ch. 5.10 - Express the given quantity as a power of e . (a) 3...Ch. 5.10 - Express the given quantity as a power of e . (a) x...Ch. 5.10 - Find the limits by making appropriate...Ch. 5.10 - Find the limits by making appropriate...Ch. 5.10 - Find gx using Part 2 of the Fundamental Theorem of...Ch. 5.10 - Find gx using Part 2 of the Fundamental Theorem of...Ch. 5.10 - Find the derivative using Formula 18 , and check...Ch. 5.10 - Find the derivative using Formula 18 , and check...Ch. 5.10 - Let Fx=0xsintt2+1dt . Find (a) F0 (b) F0 (c) F0 .Ch. 5.10 - Let Fx=2x3t2+1dt . Find (a) F2 (b) F2 (c) F2 .Ch. 5.10 - True-False Determine whether the equation is true...Ch. 5.10 - True-False Determine whether the equation is true...Ch. 5.10 - True-False Determine whether the equation is true...Ch. 5.10 - True-False Determine whether the equation is true...Ch. 5.10 - (a) Use Formula 18 to find ddx1x2t1+tdt (b) Use a...Ch. 5.10 - Show that (a) ddxxaftdt=fx (b) ddxgxaftdt=fgxgx .Ch. 5.10 - Use the results in Exercise 24 to find the...Ch. 5.10 - Use the results in Exercise 24 to find the...Ch. 5.10 - Find ddx3xx2t1t2+1dt by writing...Ch. 5.10 - Use Exercise 24b and the idea in Exercise 27 to...Ch. 5.10 - Use the result obtained in Exercise 28 to perform...Ch. 5.10 - Prove that the function Fx=x5x1tdt is constant on...Ch. 5.10 - Let Fx=0xftdt , where f is the function whose...Ch. 5.10 - Determine the inflection point(s) for the graph of...Ch. 5.10 - Express Fx in a piecewise form that does not...Ch. 5.10 - Express Fx in a piecewise form that does not...Ch. 5.10 - Use Formula 11 to solve the initial-value problem....Ch. 5.10 - Use Formula 11 to solve the initial-value problem....Ch. 5.10 - Use Formula 11 to solve the initial-value problem....Ch. 5.10 - Use Formula 11 to solve the initial-value problem....Ch. 5.10 - Suppose that at time t=0 there are P0 individuals...Ch. 5.10 - Suppose that t is the velocity function of a...Ch. 5.10 - The accompanying figure shown the graphs of y=fx...Ch. 5.10 - (a) Make a conjecture about the value of the limit...Ch. 5.10 - Let Fx=0xftdt , where f is the function graphed in...Ch. 5.10 - CAS programs have commands for working with most...Ch. 5.10 - The Fresnel sine and cosine functions Sx and Cx...Ch. 5.10 - Find the limit limh01hxx+hlntdtCh. 5.10 - Find a function f and a number a such that...Ch. 5.10 - (a) Give a geometric argument to show that...Ch. 5.10 - Use a graphing utility to generate the graph of...Ch. 5.10 - Prove: If f is continuous on open interval and a...Ch. 5 - Evaluate the integrals. 12x3+4xdxCh. 5 - Evaluate the integrals. u32u+7duCh. 5 - Evaluate the integrals. 4sinx+2cosxdxCh. 5 - Evaluate the integrals. secxtanx+cosxdxCh. 5 - Evaluate the integrals. x2/35exdxCh. 5 - Evaluate the integrals.
Ch. 5 - Evaluate the integrals. 11+x2+21x2dxCh. 5 - Evaluate the integrals. 12xx21+1x41+x2dxCh. 5 - Evaluate the integrals.
(a)
(b)
(c)
(d)
Ch. 5 - The accompanying figure shows the slope field for...Ch. 5 - (a) Show that the substitutions u=secx and u=tanx...Ch. 5 - Use the two substitutions in Exercise to evaluate...Ch. 5 - Evaluate the integral xx21x42x2dx by making the...Ch. 5 - Evaluate the integral
by making the...Ch. 5 - Evaluate the integrals by hand, and check your...Ch. 5 - Evaluate the integrals by hand, and check your...Ch. 5 - Evaluate the integrals by hand, and check your...Ch. 5 - Evaluate the integrals by hand, and check your...Ch. 5 - Express
in sigma notation with
(a) as the...Ch. 5 - (a) Fill in the blank: 1+3+5++2n1=k=1n (b) Use...Ch. 5 - Find the area under the graph of over the...Ch. 5 - Find the area under the graph of fx=5xx2 over the...Ch. 5 - Prob. 23RECh. 5 - Prob. 24RECh. 5 - The definite integral of f over the interval a,b...Ch. 5 - Use a geometric argument to evaluate 012x1dxCh. 5 - Suppose that 01fxdx=12,12fxdx=14,...Ch. 5 - In parts (a)-(d), use the information in Exercise...Ch. 5 - In each part, evaluate the integral. Where...Ch. 5 - In each part, find the limit by interpreting it as...Ch. 5 - Evaluate the integrals using the Fundamental...Ch. 5 - Evaluate the integrals using the Fundamental...Ch. 5 - Evaluate the integrals using the Fundamental...Ch. 5 - Prob. 34RECh. 5 - Evaluate the integrals using the Fundamental...Ch. 5 - Evaluate the integrals using the Fundamental...Ch. 5 - Evaluate the integrals using the Fundamental...Ch. 5 - Prob. 38RECh. 5 - Find the area under the curve y=fx over the stated...Ch. 5 - Find the area under the curve over the stated...Ch. 5 - Find the area under the curve over the stated...Ch. 5 - Find the area under the curve y=fx over the stated...Ch. 5 - Find the area that is above the x-axis but below...Ch. 5 - Use a CAS to find the area of the region in the...Ch. 5 - Sketch the curve and find the total area between...Ch. 5 - Sketch the curve and find the total area between...Ch. 5 - Define Fx by Fx=1xt3+1dt (a) Use part 2 for the...Ch. 5 - Define Fx by Fx=4x1tdt (a) Use Part 2 of the...Ch. 5 - Use Part 2 of the Fundamental Theorem of Calculus...Ch. 5 - Use Part 2 of the Fundamental Theorem of Calculus...Ch. 5 - Use Part 2 of the Fundamental Theorem of Calculus...Ch. 5 - Use Part 2 of the Fundamental Theorem of Calculus...Ch. 5 - Use Part 2 of the Fundamental Theorem of Calculus...Ch. 5 - Use Part 2 of the Fundamental Theorem of Calculus...Ch. 5 - State the two parts of the Fundamental Theorem of...Ch. 5 - Let Fx=0xt23t4+7dt . (a) Find the intervals on...Ch. 5 - (a) Use differentiation to prove that the function...Ch. 5 - What is the natural domain of the function...Ch. 5 - In each part, determine the values of x for which...Ch. 5 - Use a CAS to approximate the largest and smallest...Ch. 5 - Find all values of x in the stated interval that...Ch. 5 - A 10-gram tumor is discovered in a laboratory rat...Ch. 5 - Use the graph of f shown in the accompanying...Ch. 5 - Find the average value of fx=ex+ex over the...Ch. 5 - Derive the formulas for the position and velocity...Ch. 5 - The velocity of a particle moving along an s-axis...Ch. 5 - Prob. 67RECh. 5 - A particle moves along an x-axis . Use the given...Ch. 5 - A particle moves along an x-axis . Use the given...Ch. 5 - A particle moves along an x-axis . Use the given...Ch. 5 - A particle moves with a velocity of tm/s along an...Ch. 5 - A particle moves with a velocity of tm/s along an...Ch. 5 - A particle moves with a velocity of tm/s along an...Ch. 5 - A particle moves with a velocity of tm/s along an...Ch. 5 - A particle moves with acceleration atm/s2 along an...Ch. 5 - A particle moves with acceleration atm/s2 along an...Ch. 5 - A car traveling 60mi/h=88ft/s along a straight...Ch. 5 - Suppose that the velocity function of a particle...Ch. 5 - A ball is thrown vertically upward from a height...Ch. 5 - A rock, dropped from an unknown height, strikes...Ch. 5 - Evaluate the integrals by making an appropriate...Ch. 5 - Evaluate the integrals by making an appropriate...Ch. 5 - Evaluate the integrals by making an appropriate...Ch. 5 - Evaluate the integrals by making an appropriate...Ch. 5 - Evaluate the integrals by making an appropriate...Ch. 5 - Evaluate the integrals by making an appropriate...Ch. 5 - Evaluate the integrals by making an appropriate...Ch. 5 - Evaluate the integrals by making an appropriate...Ch. 5 - Evaluate the limits. (a) limx+1+1x2x (b)...Ch. 5 - Find a function f and a number a such that...
Additional Math Textbook Solutions
Find more solutions based on key concepts
CHECK POINT 1 Write a word description of the set L = {a, b, c, d, e, f}.
Thinking Mathematically (6th Edition)
Simplifying difference quotients Evaluate and simplify the difference quotients f(x+h)f(x)h and f(x)f(a)xa for ...
Calculus: Early Transcendentals (2nd Edition)
Of a group of patients having injuries, 28% visit both a physical therapist and a chiropractor while 8% visit n...
Probability And Statistical Inference (10th Edition)
Houses A real estate agent claims that all things being equal, houses with swimming pools tend to sell for less...
Introductory Statistics
Interpreting a P-Value In Exercises 3–8, the P-value for a hypothesis test is shown. Use the P-value to decide ...
Elementary Statistics: Picturing the World (7th Edition)
For years, telephone area codes in the United States and Canada consisted of a sequence of three digits. The fi...
A First Course in Probability (10th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- The graph of f(x) is given below. Select each true statement about the continuity of f(x) at x = -4. Select all that apply: ☐ f(x) is not continuous at x = -4 because it is not defined at x = −4. ☐ f(x) is not continuous at x = -4 because lim f(x) does not exist. x-4 f(x) is not continuous at x = -4 because lim f(x) = f(−4). ☐ f(x) is continuous at x = -4. x-4 ين من طلب نہ 1 2 3 4 5 6 7arrow_forwardThe graph of f(x) is given below. Select each true statement about the continuity of f(x) at x = -1. -7-6-5 N HT Select all that apply: ☐ f(x) is not continuous at x = -1 because it is not defined at x = -1. ☐ f(x) is not continuous at -1 because lim f(x) does not exist. x-1 ☐ f(x) is not continuous at x = -1 because lim f(x) = f(−1). ☐ f(x) is continuous at x = -1. x-1 5 6 7arrow_forwardUse the shell method to find the volume of the solid generated by revolving the region bounded by the curves and lines about the y-axis. y=x², y=7-6x, x = 0, for x≥0arrow_forward
- The graph of f(x) is given below. Select all of the true statements about the continuity of f(x) at x = −3. -7-6- -5- +1 23456 1 2 3 4 5 67 Select the correct answer below: ○ f(x) is not continuous at x = f(x) is not continuous at x = f(x) is not continuous at x = f(x) is continuous at x = -3 -3 because f(-3) is not defined. -3 because lim f(x) does not exist. 2-3 -3 because lim f(x) = f(−3). 2-3arrow_forwardCould you explain how this was solved, I don’t understand the explanation before the use of the shift property As well as the simplification afterwardsarrow_forwardQuestion The function f(x) is shown in the graph below. Which of the following statements are true? Select all that apply. f(x) 12 10 -16 -14 -12 -10 -8 + -4 " 10 12 14 16 a Select all that apply: ☐ Condition 1 is satisfied. ☐ Condition 2 is satisfied. ☐ Condition 3 is satisfied. ☐ f(x) is continuous.arrow_forward
- Find the equation of the line / in the figure below. Give exact values using the form y = mx + b. m = b = y WebAssign Plot f(x) = 10* log 9 Xarrow_forwardA particle travels along a straight line path given by s=9.5t3-2.2t2-4.5t+9.9 (in meters). What time does it change direction? Report the higher of the answers to the nearest 2 decimal places in seconds.arrow_forwardUse the method of disks to find the volume of the solid that is obtained when the region under the curve y = over the interval [4,17] is rotated about the x-axis.arrow_forward
- 1. Find the area of the region enclosed between the curves y = x and y = x. Sketch the region.arrow_forwardfor the given rectangular coordinates, find two sets of polar coordinates for which 0≤θ<2π, one with r>0 and the other with r<0. (-2sqrt(3),9)arrow_forwardI circled the correct answer, could you show me how to do it using divergence and polar coordinatesarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781305652224
Author:Charles P. McKeague, Mark D. Turner
Publisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...
Algebra
ISBN:9781337111348
Author:Bruce Crauder, Benny Evans, Alan Noell
Publisher:Cengage Learning
Chain Rule dy:dx = dy:du*du:dx; Author: Robert Cappetta;https://www.youtube.com/watch?v=IUYniALwbHs;License: Standard YouTube License, CC-BY
CHAIN RULE Part 1; Author: Btech Maths Hub;https://www.youtube.com/watch?v=TIAw6AJ_5Po;License: Standard YouTube License, CC-BY