Chapter 5, Problem 61P

To determine

Plot the shear diagram, bending moment diagram, axial force diagram, and the qualitative deflected shape of the frame.

Explanation of Solution

Write the condition for static instability, determinacy and indeterminacy of plane frames as follows:

$3m+r<3j+e_c\text{statically}\text{unstable}\text{frame}$        (1)

$3m+r=3j+e_c\text{statically}\text{determinate}\text{frame}$        (2)

$3m+r>3j+e_c\text{statically}\text{indeterminate}\text{frame}$        (3)

Here, number of members is m, number of external reactions is r, the number of joints is j, and the number of elastic hinges is $e_c$.

Find the degree of static indeterminacy (i) using the equation;

$i=\left(3m+r\right)-\left(3j+e_c\right)$        (4)

Refer to the Figure in the question;

The number of members (m) is 2.

The number of external reactions (r) is 3.

The number of joints (j) is 3.

The number of elastic hinges $e_c$ is 0.

Substitute the values in Equation (2);

$\begin{array}{l}3\left(2\right)+3=3\left(3\right)+0\\ 9=9\\ \text{statically}\text{determinate}\text{frame}\end{array}$

Show the free-body diagram of the entire frame as in Figure 1.

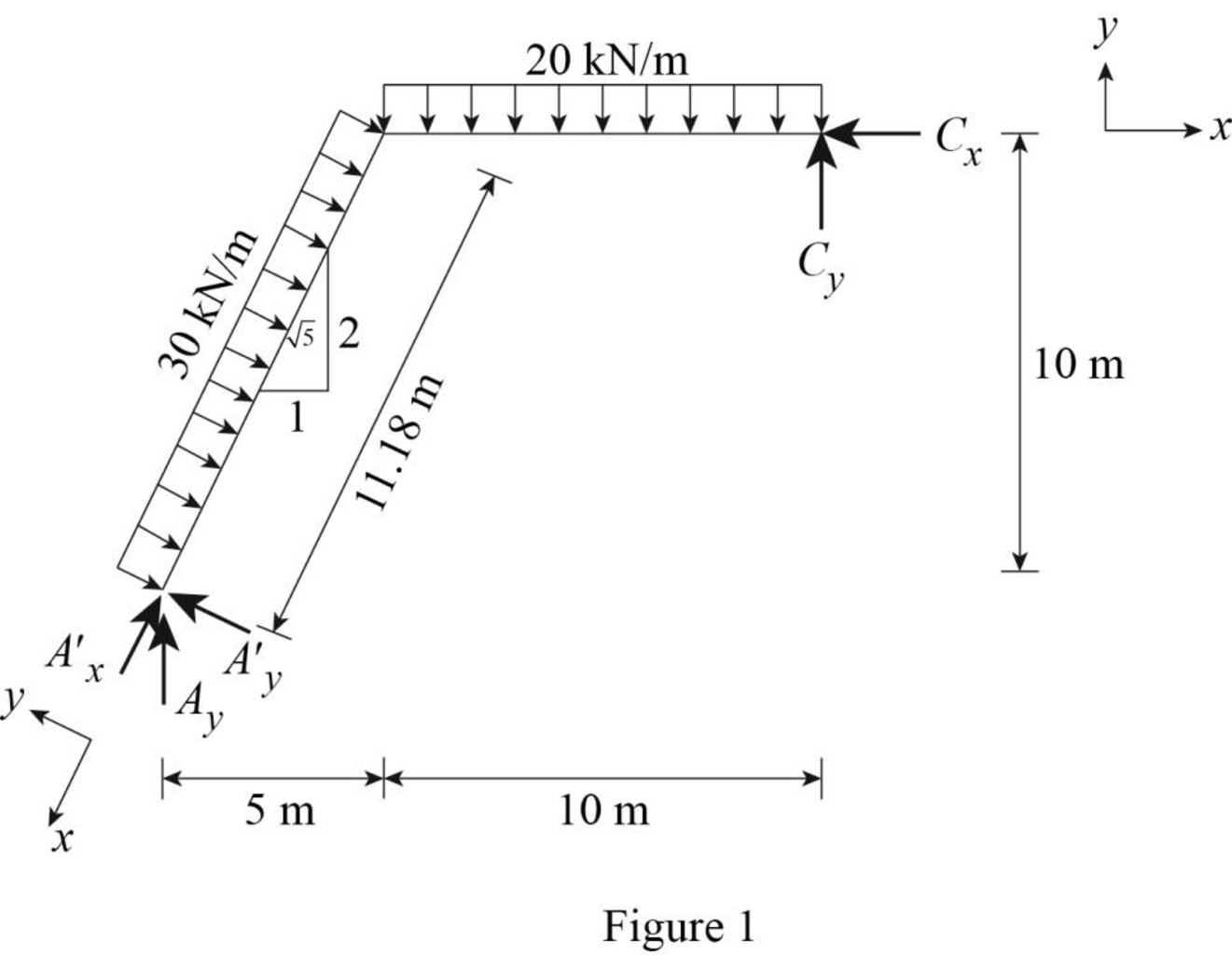
Refer Figure (1).

Find the distance for the beam AB.

$\begin{array}{l}AB\sin \theta =10\\ AB=\frac{10}{\sin 63.43°}\\ AB=11.18\text{m}\end{array}$

Find the horizontal reaction at point C by resolving the horizontal component of forces.

$\begin{array}{l}+\to {\displaystyle \sum F_X=0}\\ -C_x+30\times 11.18\times \frac{2}{\sqrt{5}}=0\\ C_x=300\text{kN}(\leftarrow )\end{array}$

Find the resultant reaction at point C by taking moment about point A.

$\begin{array}{l}+\circlearrowleft {\displaystyle \sum M_A=0}\\ C_y\left(15\right)-20\left(10\right)\left(\frac{10}{2}+5\right)+300\left(10\right)-30\times 11.18\times \frac{11.18}{2}=0\\ 15C_y-2,000+3,000-1,874.886=0\\ C_y=58.33\text{kN}(\uparrow )\end{array}$

Find the vertical reaction at point A by resolving the vertical component of forces.

$\begin{array}{l}+\uparrow {\displaystyle \sum F_Y=0}\\ A_y-20\times 10-30\times 11.18\times \frac{1}{\sqrt{5}}+C_y=0\\ A_y-200-149.99+58.33=0\\ A_y=291.66\text{kN}(\uparrow )\end{array}$

Consider point A:

Resolve the vertical component of forces.

$\begin{array}{c}+\nearrow {\displaystyle \sum F_Y=0}\\ {A^{\prime }}_y=A_y\left(\frac{1}{\sqrt{5}}\right)\\ =291.66\left(\frac{1}{\sqrt{5}}\right)\\ =130.4\text{kN}\nwarrow \end{array}$

Resolve the horizontal component of forces.

$\begin{array}{c}+\nwarrow {\displaystyle \sum F_X=0}\\ {A^{\prime }}_x=A_y\left(\frac{2}{\sqrt{5}}\right)\\ =291.66\left(\frac{2}{\sqrt{5}}\right)\\ =260.87\text{kN}(\nearrow )\end{array}$

Show the free-body diagram of the members and joints of the entire frame as in Figure 2.

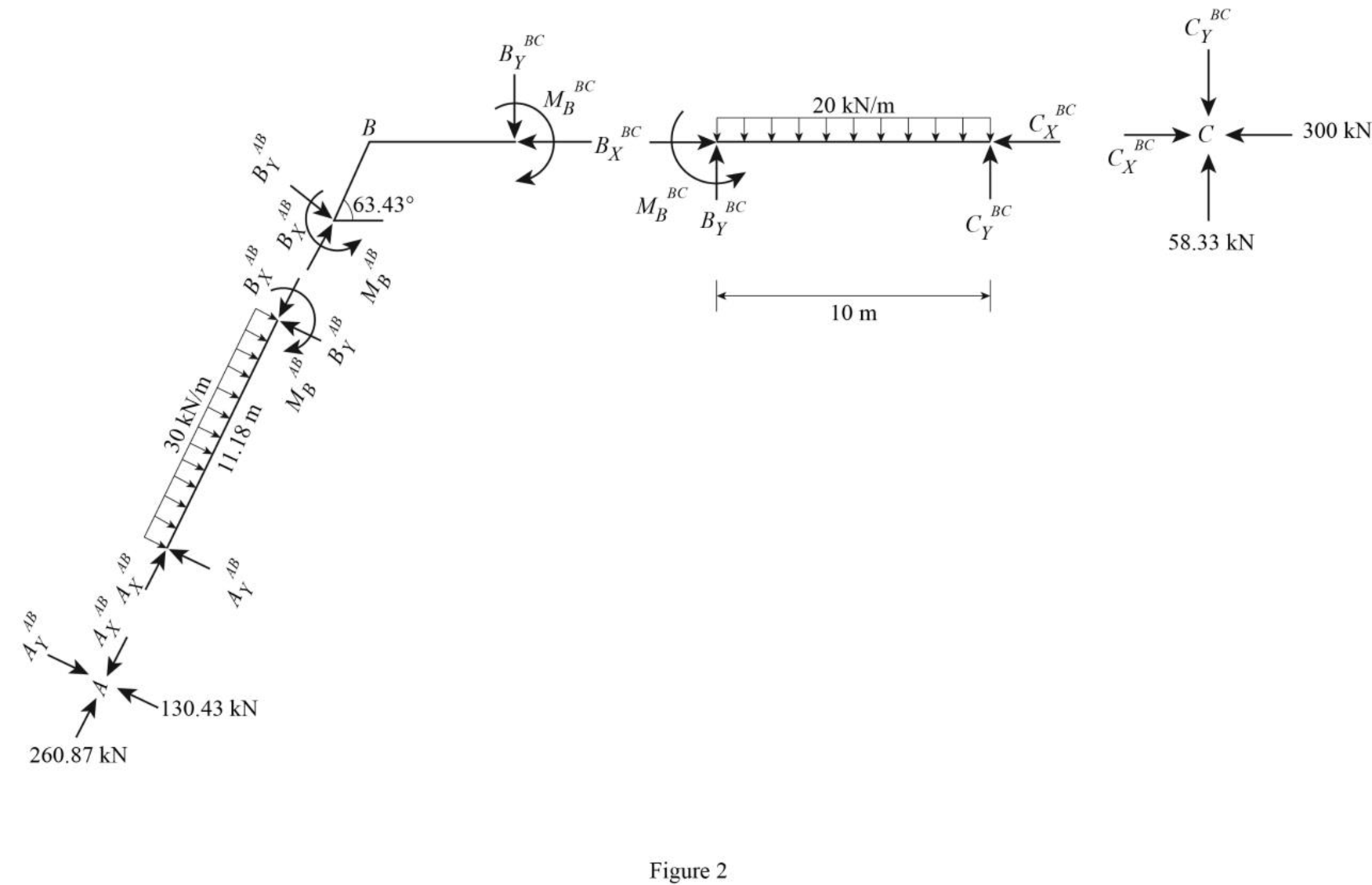
Consider point A:

Resolve the vertical component of forces.

$\begin{array}{l}+\nwarrow {\displaystyle \sum F_Y=0}\\ 130.4-A_Y^{BC}=0\\ A_Y^{BC}=130.4\text{kN}\end{array}$

Resolve the horizontal component of forces.

$\begin{array}{l}+\nearrow {\displaystyle \sum F_X=0}\\ 260.87-A_X^{BC}=0\\ A_X^{BC}=260.87\text{kN}\end{array}$

Consider the member AB:

Resolve the vertical component of forces.

$\begin{array}{l}+\nwarrow {\displaystyle \sum F_Y=0}\\ A_Y^{AB}-30\times 11.18+B_Y^{AB}=0\\ 130.43-335.4+B_Y^{AB}=0\\ B_Y^{AB}=204.97\text{kN}\end{array}$

Resolve the horizontal component of forces.

$\begin{array}{l}+\nearrow {\displaystyle \sum F_X=0}\\ -B_X^{AB}+A_X^{AB}=0\\ -B_X^{BC}+260.87=0\\ B_X^{BC}=260.87\text{kN}\end{array}$

Take moment about the point B.

$\begin{array}{l}+\circlearrowleft {\displaystyle \sum M_B^{AB}=0}\\ -A_Y^{AB}\left(11.18\right)+30\times 11.18\left(\frac{11.18}{2}\right)-M_B^{AB}=0\\ -130.43\left(11.18\right)+450-M_B^{AB}=0\\ M_B^{AB}=416.67\text{kN}\cdot \text{m}\end{array}$

Consider the point C:

Resolve the vertical component of forces.

$\begin{array}{l}+\uparrow {\displaystyle \sum F_Y=0}\\ 58.33-C_Y^{BC}=0\\ C_Y^{BC}=58.33\text{kN}\end{array}$

Resolve the horizontal component of forces.

$\begin{array}{l}+\to {\displaystyle \sum F_X=0}\\ -300+C_X^{BC}=0\\ C_X^{BC}=300\text{kN}\end{array}$

Consider the member BC:

Resolve the vertical component of forces.

$\begin{array}{l}+\uparrow {\displaystyle \sum F_Y=0}\\ B_Y^{BC}-20\times 10+C_Y^{BC}=0\\ B_Y^{BC}-200+58.33=0\\ B_Y^{BC}=141.67\text{kN}\end{array}$

Resolve the horizontal component of forces.

$\begin{array}{l}+\to {\displaystyle \sum F_X=0}\\ B_X^{BC}-C_X^{BC}=0\\ B_X^{BC}-300=0\\ B_X^{BC}=300\text{kN}\end{array}$

Take moment about point B:

$\begin{array}{l}+\circlearrowleft {\displaystyle \sum M_B^{BC}=0}\\ C_Y^{BC}\left(10\right)-20\times 10\left(\frac{10}{2}\right)+M_B^{BC}=0\\ 58.33\left(10\right)-1,000+M_B^{BC}=0\\ M_B^{BC}=416.7\text{kN}\cdot \text{m}\end{array}$

Plot the moment end forces of the frame as in Figure 3.

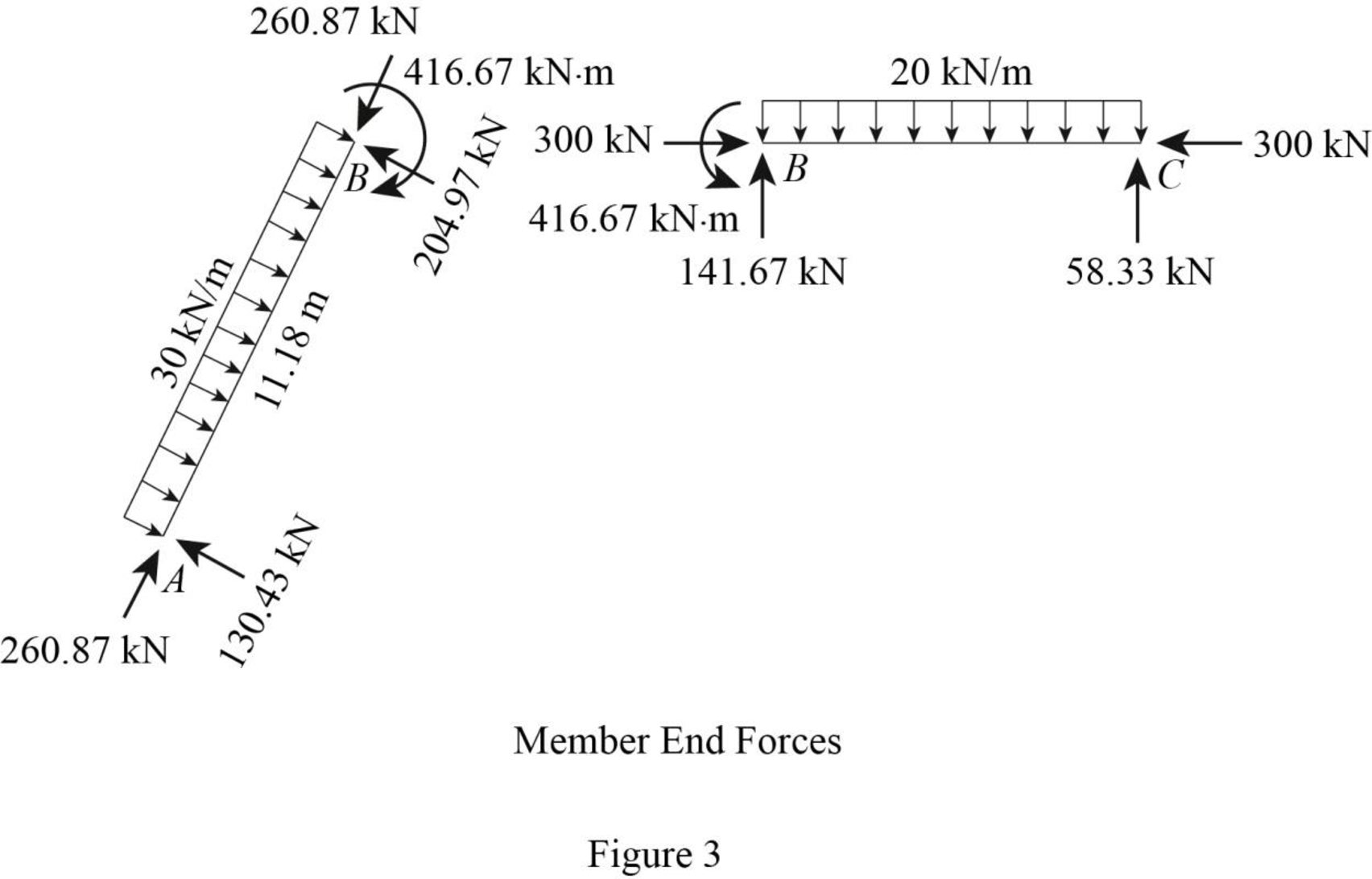
Refer to the moment end force diagram plot the shear diagram, bending moment diagram, and the axial force diagrams.

Plot the shear force diagram as in Figure 4.

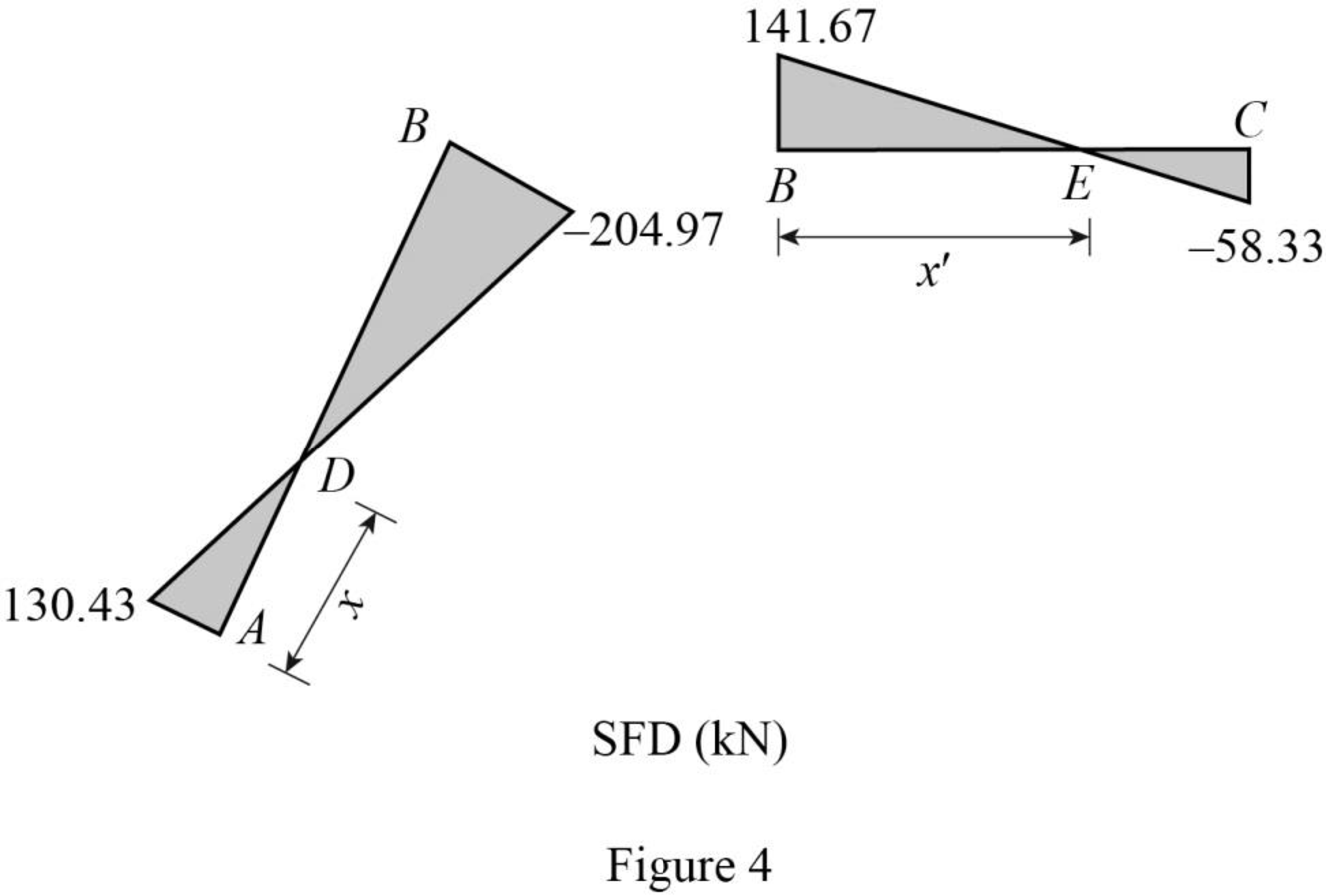
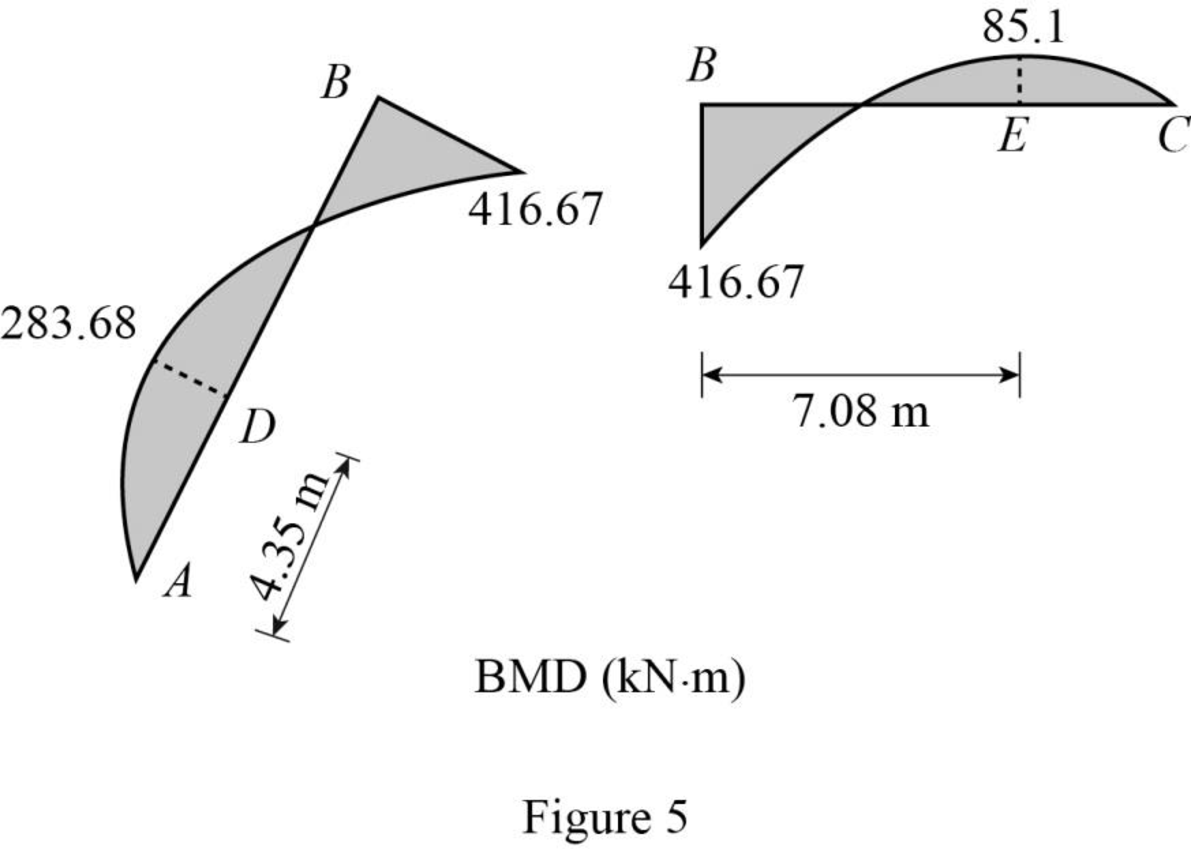
The maximum bending moment occurs where the shear force changes sign.

Consider the section ADB, use the similar triangle concept.

$\begin{array}{l}\frac{130.44}{x}=\frac{204.97}{11.18-x}\\ 1,458.32-130.44x=204.97x\\ 335.41x=1,458.32\\ x=4.35\text{m}\text{from}\text{point }A\end{array}$

Find the maximum bending at point D.

$\begin{array}{c}{M}_D=\frac{130.43\times 4.35}{2}\\ =283.68\text{kN}\cdot \text{m}\end{array}$

Consider the section BEC, use the similar triangle concept.

$\begin{array}{l}\frac{141.67}{x^{\prime }}=\frac{58.33}{10-x^{\prime }}\\ 1,416.7-141.67x^{\prime }=58.33x^{\prime }\\ 200x^{\prime }=1,416.7\\ x^{\prime }=7.08\text{m}\text{from}\text{point }B\end{array}$

Find the maximum bending at point D.

$\begin{array}{c}{M}_D=\frac{58.33\times \left(10-7.08\right)}{2}\\ =85.1\text{kN}\cdot \text{m}\end{array}$

Plot the bending moment diagram as in Figure 5.

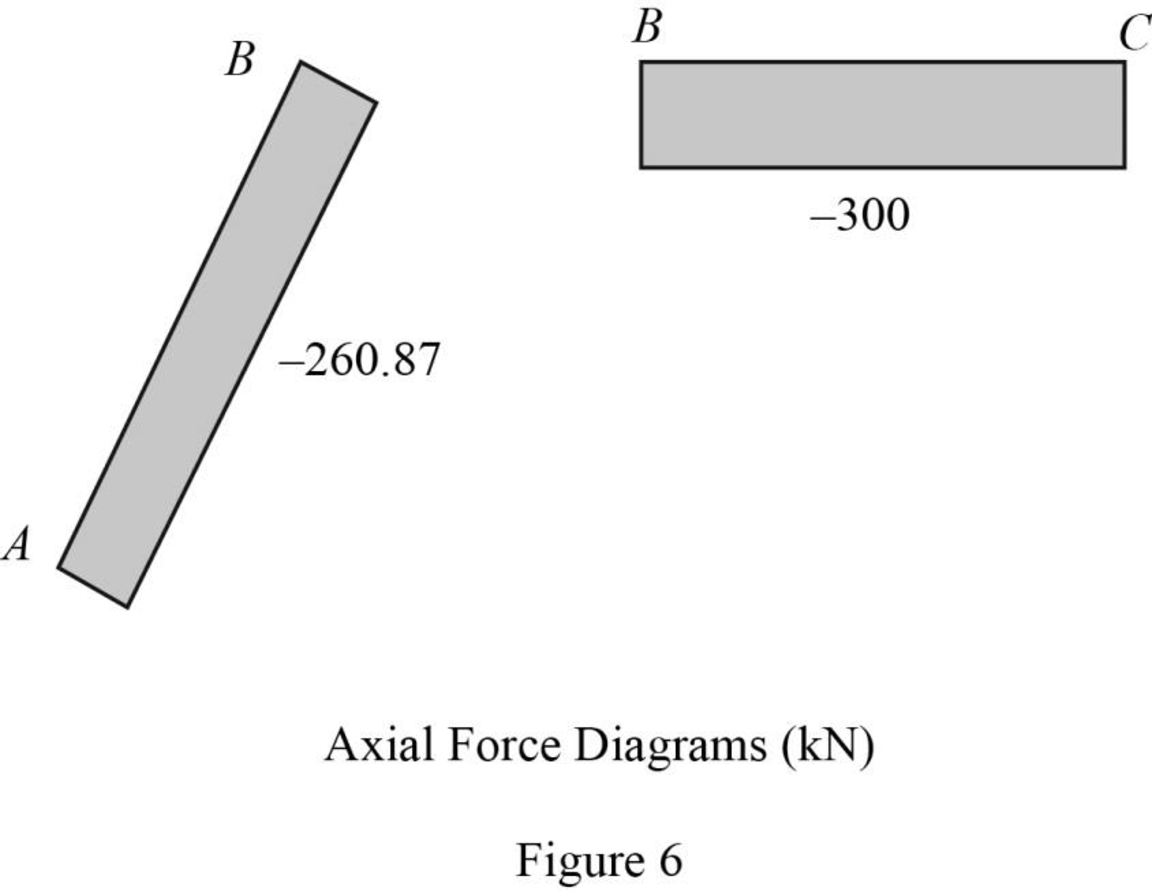
Plot the axial force diagram as in Figure 6.

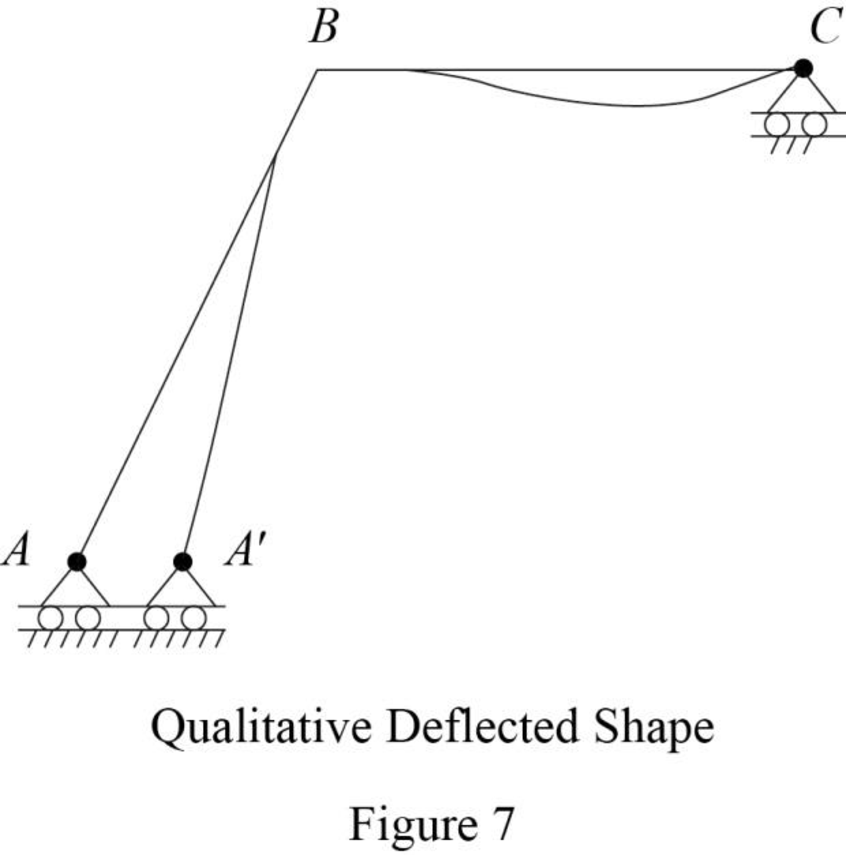
Plot the qualitative deflected shape as in Figure 7.