Chapter 4, Problem 1CQ

Propose a force standard different from the example of a stretched spring discussed in the text. Your standard must be capable of producing the sane force repeatedly.

To determine

To propose: a standard force that is different from the example of a stretched spring.

Explanation of Solution

Introduction:

The force acting on a stretched string can be calculated by calculating the stretch produced in the string. Here, we need to propose a force standard which is different than this stretched spring.

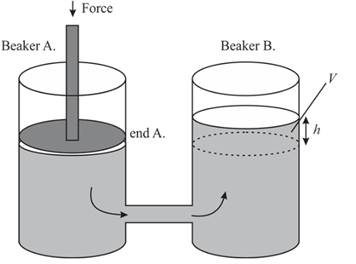
The above figure shows the arrangement of Pascal’s law. When a force is applied on the beaker A, the water level of beaker B rises by a height h .

The pressure acting on the Beaker is given as $P=hdg.$ Where, h is the height of liquid risen, d is the density of liquid and g is acceleration due to gravity.

So, $P=hdg,$

  $\begin{array}{l}\frac{F}{A}=hdg\\ F=hdgA\\ F=(hA)dg\\ F=Vdg\end{array}$

V is the volume of liquid raised in the beaker B. So by using this standard, we can measure the forces acting on beaker A.