Chapter 3.2, Problem 16P

a.

To determine

Find the values ${\displaystyle \sum x},{\displaystyle \sum x^2},{\displaystyle \sum y},\text{ and }{\displaystyle \sum y^2}$ using a calculator.

Answer to Problem 16P

The values of ${\displaystyle \sum x}=\underset{\_}{103}$ and ${\displaystyle \sum x^2}=\underset{\_}{4,607}$.

The values of ${\displaystyle \sum y}=\underset{\_}{90}$ and ${\displaystyle \sum y^2}=\underset{\_}{2,258}$.

Explanation of Solution

Step-by-step procedure to verify ${\displaystyle \sum x}$ and ${\displaystyle \sum x^2}$ using a Ti83 calculator is as given below:

• Press STAT.
• Select Edit.
• Enter the values in L1.
• Press STAT and Choose CALC.
• Select 1-Var Stats.
• To select the variable L1, Press2-nd and then press 1.
• Press Enter.

The output obtained using the Ti83 calculator is as given below:

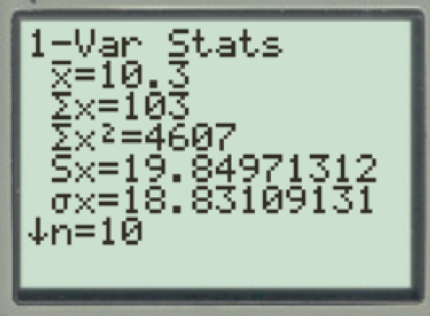
From the output, the values of ${\displaystyle \sum x}=103$ and ${\displaystyle \sum x^2}=4,607$.

Step-by-step procedure to verify ${\displaystyle \sum y}$ and ${\displaystyle \sum y^2}$ using a Ti83 calculator is as given below:

• Press STAT.
• Select Edit.
• Enter the values in L2.
• Press STAT and Choose CALC.
• Select 1-Var Stats.
• To select the variable L2, Press2-nd and then press 2.
• Press Enter.

The output obtained using the Ti83 calculator is as given below:

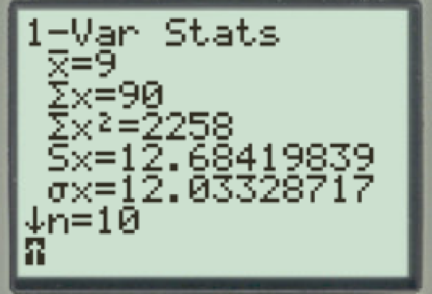
From the output, the values of ${\displaystyle \sum y}=90$ and ${\displaystyle \sum y^2}=2,258$.

b.

To determine

Find the sample mean, variance, and standard deviation for x and y using the computation formula.

Answer to Problem 16P

The values of sample mean, variance, and standard deviation for x using the computation formula are10.3, 394.0, and 19.85, respectively.

The values of sample mean, variance, and standard deviation for y using the computation formula are9, 160.9, and 12.68, respectively.

Explanation of Solution

The sample variance using the computation formula is as follows:

$s^2=\frac{{\displaystyle \sum x^2-\frac{{\left({\displaystyle \sum x}\right)}^2}{n}}}{n-1}$

Where, $\overline{x}$ is the mean, n is the number of values in the data, and x is the values in the data.

The sample standard deviation using the computation formula is as follows:

$s=\sqrt{\frac{{\displaystyle \sum x^2-\frac{{\left({\displaystyle \sum x}\right)}^2}{n}}}{n-1}}$

Where, $\overline{x}$ is the mean, n is the number of values in the data, and x is the values in the data.

The sample mean for x is obtained as given below:

$\begin{array}{c}\overline{x}=\frac{{\displaystyle \sum x}}{n}\\ =\frac{103}{10}\\ =10.3\end{array}$

Thus, the sample mean is 10.3.

The sample variance for x using the computation formula is obtained as given below:

$\begin{array}{c}{s}^2=\frac{4,607-\frac{{\left(103\right)}^2}{10}}{10-1}\\ =394.0\end{array}$

Thus, the sample variance for x using the computation formula is 394.0.

The sample standard deviation for x using the computation formula is as obtained below:

$\begin{array}{c}s=\sqrt{394.0}\\ =19.85\end{array}$

Thus, the sample standard deviation for x using the computation formula is 19.85.

The sample mean for y is obtained as given below:

$\begin{array}{c}\overline{y}=\frac{{\displaystyle \sum y}}{n}\\ =\frac{90}{10}\\ =9\end{array}$

Thus, the sample mean is 9.

The sample variance for y using the computation formula is obtained as given below:

$\begin{array}{c}{s}^2=\frac{2,258-\frac{{\left(90\right)}^2}{10}}{10-1}\\ =160.9\end{array}$

Thus, the sample variance for y using the computation formula is 160.9.

The sample standard deviation for y using the computation formula is obtained as given below:

$\begin{array}{c}s=\sqrt{160.9}\\ =12.68\end{array}$

Thus, the sample standard deviation for y using the computation formula is 12.68.

c.

To determine

Find the 75% Chebyshev interval around the mean for each fund.

Compare the two funds.

Answer to Problem 16P

The 75% Chebyshev interval around the mean for the fund Vanguard Total Stock Index is–29.4 and 50.

The 75% Chebyshev interval around the mean for the fund Vanguard Balanced Index is –16.36 and 34.36.

Explanation of Solution

The 75% Chebyshev interval around the mean for the fund Vanguard Total Stock Index is obtained as given below:

$\begin{array}{c}\overline{x}-2s=10.3-2\left(19.85\right)\\ =10.3-39.7\\ =-29.4\\ \overline{x}+2s=10.3+2\left(19.85\right)\\ =10.3+39.7\\ =50\end{array}$

Thus, the 75% Chebyshev interval around the mean for the fund Vanguard Total Stock Index is –29.4 and 50.

The 75% Chebyshev interval around the mean for the fund Vanguard Balanced Index is obtained as given below:

$\begin{array}{c}\overline{x}-2s=9-2\left(12.68\right)\\ =9-25.36\\ =-16.36\\ \overline{x}+2s=9+2\left(12.68\right)\\ =9+25.36\\ =34.36\end{array}$

Thus, the 75% Chebyshev interval around the mean for the fund Vanguard Balanced Index is –16.36 and 34.36.

From the result, it is observed that the 75% returns lie in the 75% Chebyshev interval for both the funds.

d.

To determine

Find and compare the coefficient of variation for x and y.

Answer to Problem 16P

The coefficient of variation for x is 192.7%.

The coefficient of variation for y is 140.9%.

Explanation of Solution

The formula for the coefficient of variation is as follows:

$CV=\frac{s}{\overline{x}}\times 100\%$

Where, s is the sample standard deviation and $\overline{x}$ is the sample mean.

The coefficient of variation for x is obtained as given below:

$\begin{array}{c}CV=\frac{19.85}{10.3}\times 100\%\\ =192.7\%\end{array}$

Thus, the coefficient of variation for x is 192.7%.

The coefficient of variation for y is obtained as given below:

$\begin{array}{c}CV=\frac{12.68}{9}\times 100\%\\ =140.9\%\end{array}$

Thus, the coefficient of variation for y is 140.9%.

From the results, the coefficient of variation for the fund Vanguard Balanced Index is less when compared to the coefficient of variation for the fund Vanguard Total Stock Index. Hence, the fund Vanguard Balanced Index has less risk per unit of return. Hence, the fund Vanguard Balanced Index appears to be better.