PRECALCULUS W/LIMITS:GRAPH.APPROACH(HS)
8th Edition
ISBN: 9781337904285
Author: Larson
Publisher: CENGAGE L
expand_more
expand_more
format_list_bulleted
Videos
Question
Chapter 3.2, Problem 114E
(a).
To determine
Tograph : the given function and approximate the required rate of ventilation in a room with
(a).
Expert Solution
Explanation of Solution
Given information :
The model:
The model approximates the minimum required rate of ventilation y (in cubic feet per minute per child) in a classroom with x cubic feet of air space per child.
The cubic feet of air space per child,
Graph :
As per the problem,
For the function,
Sketch the graph using graphing utility.
Step 1: Press WINDOW button to access the Window editor.
Step 2: Press
Step 3: Enter the expression,
Step 4: Press GRAPH button to graph the functions.
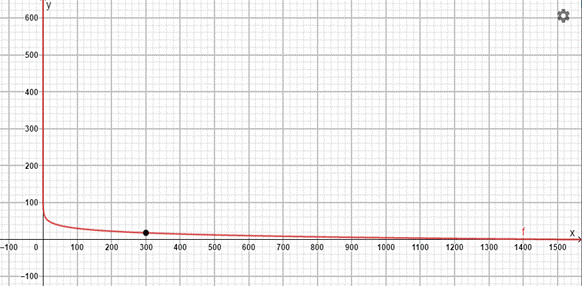
Interpretation:From the graph,
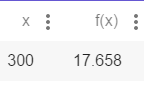
Therefore, for
(b).
To determine
Tocalculate : the rate of ventilation per child.
(b).
Expert Solution
Answer to Problem 114E
The rate of ventilation is
Explanation of Solution
Given information :
The model:
The model approximates the minimum required rate of ventilation y (in cubic feet per minute per child) in a classroom with x cubic feet of air space per child.
Total number of students in the classroom is
The total cubic feet of air per minute is
Calculation :
As per the problem,
Total number of students in the classroom is
The total cubic feet of air per minute is
The rate of ventilation y is the cubic feet of air per minute per child.
Therefore,
(c).
To determine
Toestimate : the minimum required air space per child for the classroom in part(b), using the graph obtained in part(a).
(c).
Expert Solution
Answer to Problem 114E
Explanation of Solution
Given information :
Consider the graph obtained in part (a).
The rate of ventilation per child,
As per the problem,
Consider the graph obtained in part (a):
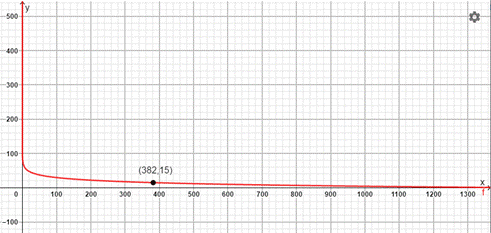
From the graph, for
Therefore, the minimum required air space per child for the classroom, having
(d).
To determine
Toexplain : the rate of ventilation is adequate for the classroom in part (b) having the given volume.
(d).
Expert Solution
Answer to Problem 114E
The rate of ventilation for the classroom,
Explanation of Solution
Given information :
The classroom of
Therate of ventilation for the classroom having
The model:
The model approximates the minimum required rate of ventilation y (in cubic feet per minute per child) in a classroom with x cubic feet of air space per child.
As per the problem,
Total students in the classroom is
The floor space of the classroom is
The height of the ceiling of the classroom is
Therefore, volume of the classroom or the total cubic feet of air spaceis
The cubic feet of air space per child is
Substitute
Therefore, the minimum required rate of ventilation for the classroom is
Thus, the rate of ventilation for the classroom,
Chapter 3 Solutions
PRECALCULUS W/LIMITS:GRAPH.APPROACH(HS)
Ch. 3.1 - Prob. 1ECh. 3.1 - Prob. 2ECh. 3.1 - Prob. 3ECh. 3.1 - Prob. 4ECh. 3.1 - Prob. 5ECh. 3.1 - Prob. 6ECh. 3.1 - Prob. 7ECh. 3.1 - Prob. 8ECh. 3.1 - Prob. 9ECh. 3.1 - Prob. 10E
Ch. 3.1 - Prob. 11ECh. 3.1 - Prob. 12ECh. 3.1 - Prob. 13ECh. 3.1 - Prob. 14ECh. 3.1 - Prob. 15ECh. 3.1 - Prob. 16ECh. 3.1 - Prob. 17ECh. 3.1 - Prob. 18ECh. 3.1 - Prob. 19ECh. 3.1 - Prob. 20ECh. 3.1 - Prob. 21ECh. 3.1 - Prob. 22ECh. 3.1 - Prob. 23ECh. 3.1 - Prob. 24ECh. 3.1 - Prob. 25ECh. 3.1 - Prob. 26ECh. 3.1 - Prob. 27ECh. 3.1 - Prob. 28ECh. 3.1 - Prob. 29ECh. 3.1 - Prob. 30ECh. 3.1 - Prob. 31ECh. 3.1 - Prob. 32ECh. 3.1 - Prob. 33ECh. 3.1 - Prob. 34ECh. 3.1 - Prob. 35ECh. 3.1 - Prob. 36ECh. 3.1 - Prob. 37ECh. 3.1 - Prob. 38ECh. 3.1 - Prob. 39ECh. 3.1 - Prob. 40ECh. 3.1 - Prob. 41ECh. 3.1 - Prob. 42ECh. 3.1 - Prob. 43ECh. 3.1 - Prob. 44ECh. 3.1 - Prob. 45ECh. 3.1 - Prob. 46ECh. 3.1 - Prob. 47ECh. 3.1 - Prob. 48ECh. 3.1 - Prob. 49ECh. 3.1 - Prob. 50ECh. 3.1 - Prob. 51ECh. 3.1 - Prob. 52ECh. 3.1 - Prob. 53ECh. 3.1 - Prob. 54ECh. 3.1 - Prob. 55ECh. 3.1 - Prob. 56ECh. 3.1 - Prob. 57ECh. 3.1 - Prob. 58ECh. 3.1 - Prob. 59ECh. 3.1 - Prob. 60ECh. 3.1 - Prob. 61ECh. 3.1 - Prob. 62ECh. 3.1 - Prob. 63ECh. 3.1 - Prob. 64ECh. 3.1 - Prob. 65ECh. 3.1 - Prob. 66ECh. 3.1 - Prob. 67ECh. 3.1 - Prob. 68ECh. 3.1 - Prob. 69ECh. 3.1 - Prob. 70ECh. 3.1 - Prob. 71ECh. 3.1 - Prob. 72ECh. 3.1 - Prob. 73ECh. 3.1 - Prob. 74ECh. 3.1 - Prob. 75ECh. 3.1 - Prob. 76ECh. 3.1 - Prob. 77ECh. 3.1 - Prob. 78ECh. 3.1 - Prob. 79ECh. 3.1 - Prob. 80ECh. 3.1 - Prob. 81ECh. 3.1 - Prob. 82ECh. 3.1 - Prob. 83ECh. 3.1 - Prob. 84ECh. 3.1 - Prob. 85ECh. 3.1 - Prob. 86ECh. 3.1 - Prob. 87ECh. 3.1 - Prob. 88ECh. 3.1 - Prob. 89ECh. 3.1 - Prob. 90ECh. 3.1 - Prob. 91ECh. 3.1 - Prob. 92ECh. 3.1 - Prob. 93ECh. 3.2 - Prob. 1ECh. 3.2 - Prob. 2ECh. 3.2 - Prob. 3ECh. 3.2 - Prob. 4ECh. 3.2 - Prob. 5ECh. 3.2 - Prob. 6ECh. 3.2 - Prob. 7ECh. 3.2 - Prob. 8ECh. 3.2 - Prob. 9ECh. 3.2 - Prob. 10ECh. 3.2 - Prob. 11ECh. 3.2 - Prob. 12ECh. 3.2 - Prob. 13ECh. 3.2 - Prob. 14ECh. 3.2 - Prob. 15ECh. 3.2 - Prob. 16ECh. 3.2 - Prob. 17ECh. 3.2 - Prob. 18ECh. 3.2 - Prob. 19ECh. 3.2 - Prob. 20ECh. 3.2 - Prob. 21ECh. 3.2 - Prob. 22ECh. 3.2 - Prob. 23ECh. 3.2 - Prob. 24ECh. 3.2 - Prob. 25ECh. 3.2 - Prob. 26ECh. 3.2 - Prob. 27ECh. 3.2 - Prob. 28ECh. 3.2 - Prob. 29ECh. 3.2 - Prob. 30ECh. 3.2 - Prob. 31ECh. 3.2 - Prob. 32ECh. 3.2 - Prob. 33ECh. 3.2 - Prob. 34ECh. 3.2 - Prob. 35ECh. 3.2 - Prob. 36ECh. 3.2 - Prob. 37ECh. 3.2 - Prob. 38ECh. 3.2 - Prob. 39ECh. 3.2 - Prob. 40ECh. 3.2 - Prob. 41ECh. 3.2 - Prob. 42ECh. 3.2 - Prob. 43ECh. 3.2 - Prob. 44ECh. 3.2 - Prob. 45ECh. 3.2 - Prob. 46ECh. 3.2 - Prob. 47ECh. 3.2 - Prob. 48ECh. 3.2 - Prob. 49ECh. 3.2 - Prob. 50ECh. 3.2 - Prob. 51ECh. 3.2 - Prob. 52ECh. 3.2 - Prob. 53ECh. 3.2 - Prob. 54ECh. 3.2 - Prob. 55ECh. 3.2 - Prob. 56ECh. 3.2 - Prob. 57ECh. 3.2 - Prob. 58ECh. 3.2 - Prob. 59ECh. 3.2 - Prob. 60ECh. 3.2 - Prob. 61ECh. 3.2 - Prob. 62ECh. 3.2 - Prob. 63ECh. 3.2 - Prob. 64ECh. 3.2 - Prob. 65ECh. 3.2 - Prob. 66ECh. 3.2 - Prob. 67ECh. 3.2 - Prob. 68ECh. 3.2 - Prob. 69ECh. 3.2 - Prob. 70ECh. 3.2 - Prob. 71ECh. 3.2 - Prob. 72ECh. 3.2 - Prob. 73ECh. 3.2 - Prob. 74ECh. 3.2 - Prob. 75ECh. 3.2 - Prob. 76ECh. 3.2 - Prob. 77ECh. 3.2 - Prob. 78ECh. 3.2 - Prob. 79ECh. 3.2 - Prob. 80ECh. 3.2 - Prob. 81ECh. 3.2 - Prob. 82ECh. 3.2 - Prob. 83ECh. 3.2 - Prob. 84ECh. 3.2 - Prob. 85ECh. 3.2 - Prob. 86ECh. 3.2 - Prob. 87ECh. 3.2 - Prob. 88ECh. 3.2 - Prob. 89ECh. 3.2 - Prob. 90ECh. 3.2 - Prob. 91ECh. 3.2 - Prob. 92ECh. 3.2 - Prob. 93ECh. 3.2 - Prob. 94ECh. 3.2 - Prob. 95ECh. 3.2 - Prob. 96ECh. 3.2 - Prob. 97ECh. 3.2 - Prob. 98ECh. 3.2 - Prob. 99ECh. 3.2 - Prob. 100ECh. 3.2 - Prob. 101ECh. 3.2 - Prob. 102ECh. 3.2 - Prob. 103ECh. 3.2 - Prob. 104ECh. 3.2 - Prob. 105ECh. 3.2 - Prob. 106ECh. 3.2 - Prob. 107ECh. 3.2 - Prob. 108ECh. 3.2 - Prob. 109ECh. 3.2 - Prob. 110ECh. 3.2 - Prob. 111ECh. 3.2 - Prob. 112ECh. 3.2 - Prob. 113ECh. 3.2 - Prob. 114ECh. 3.2 - Prob. 115ECh. 3.2 - Prob. 116ECh. 3.2 - Prob. 117ECh. 3.2 - Prob. 118ECh. 3.2 - Prob. 119ECh. 3.2 - Prob. 120ECh. 3.2 - Prob. 121ECh. 3.2 - Prob. 122ECh. 3.2 - Prob. 123ECh. 3.2 - Prob. 124ECh. 3.2 - Prob. 125ECh. 3.2 - Prob. 126ECh. 3.2 - Prob. 127ECh. 3.2 - Prob. 128ECh. 3.2 - Prob. 129ECh. 3.2 - Prob. 130ECh. 3.2 - Prob. 131ECh. 3.2 - Prob. 132ECh. 3.2 - Prob. 133ECh. 3.2 - Prob. 134ECh. 3.3 - Prob. 1ECh. 3.3 - Prob. 2ECh. 3.3 - Prob. 3ECh. 3.3 - Prob. 4ECh. 3.3 - Prob. 5ECh. 3.3 - Prob. 6ECh. 3.3 - Prob. 7ECh. 3.3 - Prob. 8ECh. 3.3 - Prob. 9ECh. 3.3 - Prob. 10ECh. 3.3 - Prob. 11ECh. 3.3 - Prob. 12ECh. 3.3 - Prob. 13ECh. 3.3 - Prob. 14ECh. 3.3 - Prob. 15ECh. 3.3 - Prob. 16ECh. 3.3 - Prob. 17ECh. 3.3 - Prob. 18ECh. 3.3 - Prob. 19ECh. 3.3 - Prob. 20ECh. 3.3 - Prob. 21ECh. 3.3 - Prob. 22ECh. 3.3 - Prob. 23ECh. 3.3 - Prob. 24ECh. 3.3 - Prob. 25ECh. 3.3 - Prob. 26ECh. 3.3 - Prob. 27ECh. 3.3 - Prob. 28ECh. 3.3 - Prob. 29ECh. 3.3 - Prob. 30ECh. 3.3 - Prob. 31ECh. 3.3 - Prob. 32ECh. 3.3 - Prob. 33ECh. 3.3 - Prob. 34ECh. 3.3 - Prob. 35ECh. 3.3 - Prob. 36ECh. 3.3 - Prob. 37ECh. 3.3 - Prob. 38ECh. 3.3 - Prob. 39ECh. 3.3 - Prob. 40ECh. 3.3 - Prob. 41ECh. 3.3 - Prob. 42ECh. 3.3 - Prob. 43ECh. 3.3 - Prob. 44ECh. 3.3 - Prob. 45ECh. 3.3 - Prob. 46ECh. 3.3 - Prob. 47ECh. 3.3 - Prob. 48ECh. 3.3 - Prob. 49ECh. 3.3 - Prob. 50ECh. 3.3 - Prob. 51ECh. 3.3 - Prob. 52ECh. 3.3 - Prob. 53ECh. 3.3 - Prob. 54ECh. 3.3 - Prob. 55ECh. 3.3 - Prob. 56ECh. 3.3 - Prob. 57ECh. 3.3 - Prob. 58ECh. 3.3 - Prob. 59ECh. 3.3 - Prob. 60ECh. 3.3 - Prob. 61ECh. 3.3 - Prob. 62ECh. 3.3 - Prob. 63ECh. 3.3 - Prob. 64ECh. 3.3 - Prob. 65ECh. 3.3 - Prob. 66ECh. 3.3 - Prob. 67ECh. 3.3 - Prob. 68ECh. 3.3 - Prob. 69ECh. 3.3 - Prob. 70ECh. 3.3 - Prob. 71ECh. 3.3 - Prob. 72ECh. 3.3 - Prob. 73ECh. 3.3 - Prob. 74ECh. 3.3 - Prob. 75ECh. 3.3 - Prob. 76ECh. 3.3 - Prob. 77ECh. 3.3 - Prob. 78ECh. 3.3 - Prob. 79ECh. 3.3 - Prob. 80ECh. 3.3 - Prob. 81ECh. 3.3 - Prob. 82ECh. 3.3 - Prob. 83ECh. 3.3 - Prob. 84ECh. 3.3 - Prob. 85ECh. 3.3 - Prob. 86ECh. 3.3 - Prob. 87ECh. 3.3 - Prob. 88ECh. 3.3 - Prob. 89ECh. 3.3 - Prob. 90ECh. 3.3 - Prob. 91ECh. 3.3 - Prob. 92ECh. 3.3 - Prob. 93ECh. 3.3 - Prob. 94ECh. 3.3 - Prob. 97ECh. 3.3 - Prob. 98ECh. 3.3 - Prob. 99ECh. 3.3 - Prob. 100ECh. 3.3 - Prob. 101ECh. 3.3 - Prob. 102ECh. 3.3 - Prob. 103ECh. 3.3 - Prob. 104ECh. 3.3 - Prob. 105ECh. 3.3 - Prob. 106ECh. 3.3 - Prob. 107ECh. 3.3 - Prob. 108ECh. 3.3 - Prob. 109ECh. 3.3 - Prob. 110ECh. 3.3 - Prob. 111ECh. 3.3 - Prob. 112ECh. 3.4 - Prob. 1ECh. 3.4 - Prob. 2ECh. 3.4 - Prob. 3ECh. 3.4 - Prob. 4ECh. 3.4 - Prob. 5ECh. 3.4 - Prob. 6ECh. 3.4 - Prob. 7ECh. 3.4 - Prob. 8ECh. 3.4 - Prob. 9ECh. 3.4 - Prob. 10ECh. 3.4 - Prob. 11ECh. 3.4 - Prob. 12ECh. 3.4 - Prob. 13ECh. 3.4 - Prob. 14ECh. 3.4 - Prob. 15ECh. 3.4 - Prob. 16ECh. 3.4 - Prob. 17ECh. 3.4 - Prob. 18ECh. 3.4 - Prob. 19ECh. 3.4 - Prob. 20ECh. 3.4 - Prob. 21ECh. 3.4 - Prob. 22ECh. 3.4 - Prob. 23ECh. 3.4 - Prob. 24ECh. 3.4 - Prob. 25ECh. 3.4 - Prob. 26ECh. 3.4 - Prob. 27ECh. 3.4 - Prob. 28ECh. 3.4 - Prob. 29ECh. 3.4 - Prob. 30ECh. 3.4 - Prob. 31ECh. 3.4 - Prob. 32ECh. 3.4 - Prob. 33ECh. 3.4 - Prob. 34ECh. 3.4 - Prob. 35ECh. 3.4 - Prob. 36ECh. 3.4 - Prob. 37ECh. 3.4 - Prob. 38ECh. 3.4 - Prob. 39ECh. 3.4 - Prob. 40ECh. 3.4 - Prob. 41ECh. 3.4 - Prob. 42ECh. 3.4 - Prob. 43ECh. 3.4 - Prob. 44ECh. 3.4 - Prob. 45ECh. 3.4 - Prob. 46ECh. 3.4 - Prob. 47ECh. 3.4 - Prob. 48ECh. 3.4 - Prob. 49ECh. 3.4 - Prob. 50ECh. 3.4 - Prob. 51ECh. 3.4 - Prob. 52ECh. 3.4 - Prob. 53ECh. 3.4 - Prob. 54ECh. 3.4 - Prob. 55ECh. 3.4 - Prob. 56ECh. 3.4 - Prob. 57ECh. 3.4 - Prob. 58ECh. 3.4 - Prob. 59ECh. 3.4 - Prob. 60ECh. 3.4 - Prob. 61ECh. 3.4 - Prob. 62ECh. 3.4 - Prob. 63ECh. 3.4 - Prob. 64ECh. 3.4 - Prob. 65ECh. 3.4 - Prob. 66ECh. 3.4 - Prob. 67ECh. 3.4 - Prob. 68ECh. 3.4 - Prob. 69ECh. 3.4 - Prob. 70ECh. 3.4 - Prob. 71ECh. 3.4 - Prob. 72ECh. 3.4 - Prob. 73ECh. 3.4 - Prob. 74ECh. 3.4 - Prob. 75ECh. 3.4 - Prob. 76ECh. 3.4 - Prob. 77ECh. 3.4 - Prob. 78ECh. 3.4 - Prob. 79ECh. 3.4 - Prob. 80ECh. 3.4 - Prob. 81ECh. 3.4 - Prob. 82ECh. 3.4 - Prob. 83ECh. 3.4 - Prob. 84ECh. 3.4 - Prob. 85ECh. 3.4 - Prob. 86ECh. 3.4 - Prob. 87ECh. 3.4 - Prob. 88ECh. 3.4 - Prob. 89ECh. 3.4 - Prob. 90ECh. 3.4 - Prob. 91ECh. 3.4 - Prob. 92ECh. 3.4 - Prob. 93ECh. 3.4 - Prob. 94ECh. 3.4 - Prob. 95ECh. 3.4 - Prob. 96ECh. 3.4 - Prob. 97ECh. 3.4 - Prob. 98ECh. 3.4 - Prob. 99ECh. 3.4 - Prob. 100ECh. 3.4 - Prob. 101ECh. 3.4 - Prob. 102ECh. 3.4 - Prob. 103ECh. 3.4 - Prob. 104ECh. 3.4 - Prob. 105ECh. 3.4 - Prob. 106ECh. 3.4 - Prob. 107ECh. 3.4 - Prob. 108ECh. 3.4 - Prob. 109ECh. 3.4 - Prob. 110ECh. 3.4 - Prob. 111ECh. 3.4 - Prob. 112ECh. 3.4 - Prob. 113ECh. 3.4 - Prob. 114ECh. 3.4 - Prob. 115ECh. 3.4 - Prob. 116ECh. 3.4 - Prob. 117ECh. 3.4 - Prob. 118ECh. 3.4 - Prob. 119ECh. 3.4 - Prob. 120ECh. 3.4 - Prob. 121ECh. 3.4 - Prob. 122ECh. 3.4 - Prob. 123ECh. 3.4 - Prob. 124ECh. 3.4 - Prob. 125ECh. 3.4 - Prob. 126ECh. 3.4 - Prob. 127ECh. 3.4 - Prob. 128ECh. 3.4 - Prob. 129ECh. 3.4 - Prob. 130ECh. 3.4 - Prob. 131ECh. 3.4 - Prob. 132ECh. 3.4 - Prob. 133ECh. 3.4 - Prob. 134ECh. 3.4 - Prob. 135ECh. 3.4 - Prob. 136ECh. 3.4 - Prob. 137ECh. 3.4 - Prob. 138ECh. 3.4 - Prob. 139ECh. 3.4 - Prob. 140ECh. 3.4 - Prob. 141ECh. 3.4 - Prob. 142ECh. 3.4 - Prob. 143ECh. 3.4 - Prob. 144ECh. 3.4 - Prob. 145ECh. 3.4 - Prob. 146ECh. 3.5 - Prob. 1ECh. 3.5 - Prob. 2ECh. 3.5 - Prob. 3ECh. 3.5 - Prob. 4ECh. 3.5 - Prob. 5ECh. 3.5 - Prob. 6ECh. 3.5 - Prob. 7ECh. 3.5 - Prob. 8ECh. 3.5 - Prob. 9ECh. 3.5 - Prob. 10ECh. 3.5 - Prob. 11ECh. 3.5 - Prob. 12ECh. 3.5 - Prob. 13ECh. 3.5 - Prob. 14ECh. 3.5 - Prob. 15ECh. 3.5 - Prob. 16ECh. 3.5 - Prob. 17ECh. 3.5 - Prob. 18ECh. 3.5 - Prob. 19ECh. 3.5 - Prob. 20ECh. 3.5 - Prob. 21ECh. 3.5 - Prob. 22ECh. 3.5 - Prob. 23ECh. 3.5 - Prob. 24ECh. 3.5 - Prob. 25ECh. 3.5 - Prob. 26ECh. 3.5 - Prob. 27ECh. 3.5 - Prob. 28ECh. 3.5 - Prob. 29ECh. 3.5 - Prob. 30ECh. 3.5 - Prob. 31ECh. 3.5 - Prob. 32ECh. 3.5 - Prob. 33ECh. 3.5 - Prob. 34ECh. 3.5 - Prob. 35ECh. 3.5 - Prob. 36ECh. 3.5 - Prob. 37ECh. 3.5 - Prob. 38ECh. 3.5 - Prob. 39ECh. 3.5 - Prob. 40ECh. 3.5 - Prob. 41ECh. 3.5 - Prob. 42ECh. 3.5 - Prob. 43ECh. 3.5 - Prob. 44ECh. 3.5 - Prob. 45ECh. 3.5 - Prob. 46ECh. 3.5 - Prob. 47ECh. 3.5 - Prob. 48ECh. 3.5 - Prob. 49ECh. 3.5 - Prob. 50ECh. 3.5 - Prob. 51ECh. 3.5 - Prob. 52ECh. 3.5 - Prob. 53ECh. 3.5 - Prob. 54ECh. 3.5 - Prob. 55ECh. 3.5 - Prob. 56ECh. 3.5 - Prob. 57ECh. 3.5 - Prob. 58ECh. 3.5 - Prob. 59ECh. 3.5 - Prob. 60ECh. 3.5 - Prob. 61ECh. 3.5 - Prob. 62ECh. 3.5 - Prob. 63ECh. 3.5 - Prob. 64ECh. 3.5 - Prob. 65ECh. 3.5 - Prob. 66ECh. 3.5 - Prob. 67ECh. 3.5 - Prob. 68ECh. 3.5 - Prob. 69ECh. 3.5 - Prob. 70ECh. 3.5 - Prob. 71ECh. 3.5 - Prob. 72ECh. 3.5 - Prob. 73ECh. 3.5 - Prob. 74ECh. 3.5 - Prob. 75ECh. 3.5 - Prob. 76ECh. 3.5 - Prob. 77ECh. 3.5 - Prob. 78ECh. 3.6 - Prob. 1ECh. 3.6 - Prob. 2ECh. 3.6 - Prob. 3ECh. 3.6 - Prob. 4ECh. 3.6 - Prob. 5ECh. 3.6 - Prob. 6ECh. 3.6 - Prob. 7ECh. 3.6 - Prob. 8ECh. 3.6 - Prob. 9ECh. 3.6 - Prob. 10ECh. 3.6 - Prob. 11ECh. 3.6 - Prob. 12ECh. 3.6 - Prob. 13ECh. 3.6 - Prob. 14ECh. 3.6 - Prob. 15ECh. 3.6 - Prob. 16ECh. 3.6 - Prob. 17ECh. 3.6 - Prob. 18ECh. 3.6 - Prob. 19ECh. 3.6 - Prob. 20ECh. 3.6 - Prob. 21ECh. 3.6 - Prob. 22ECh. 3.6 - Prob. 23ECh. 3.6 - Prob. 24ECh. 3.6 - Prob. 25ECh. 3.6 - Prob. 26ECh. 3.6 - Prob. 27ECh. 3.6 - Prob. 28ECh. 3.6 - Prob. 29ECh. 3.6 - Prob. 30ECh. 3.6 - Prob. 31ECh. 3.6 - Prob. 32ECh. 3.6 - Prob. 33ECh. 3.6 - Prob. 34ECh. 3.6 - Prob. 35ECh. 3.6 - Prob. 36ECh. 3.6 - Prob. 37ECh. 3.6 - Prob. 38ECh. 3.6 - Prob. 39ECh. 3.6 - Prob. 40ECh. 3.6 - Prob. 41ECh. 3.6 - Prob. 42ECh. 3.6 - Prob. 43ECh. 3.6 - Prob. 44ECh. 3.6 - Prob. 45ECh. 3.6 - Prob. 46ECh. 3 - Prob. 1CRCh. 3 - Prob. 2CRCh. 3 - Prob. 3CRCh. 3 - Prob. 4CRCh. 3 - Prob. 5CRCh. 3 - Prob. 6CRCh. 3 - Prob. 7CRCh. 3 - Prob. 8CRCh. 3 - Prob. 9CRCh. 3 - Prob. 10CRCh. 3 - Prob. 11CRCh. 3 - Prob. 12CRCh. 3 - Prob. 13CRCh. 3 - Prob. 14CRCh. 3 - Prob. 15CRCh. 3 - Prob. 16CRCh. 3 - Prob. 17CRCh. 3 - Prob. 18CRCh. 3 - Prob. 19CRCh. 3 - Prob. 20CRCh. 3 - Prob. 21CRCh. 3 - Prob. 22CRCh. 3 - Prob. 23CRCh. 3 - Prob. 24CRCh. 3 - Prob. 25CRCh. 3 - Prob. 26CRCh. 3 - Prob. 27CRCh. 3 - Prob. 28CRCh. 3 - Prob. 29CRCh. 3 - Prob. 30CRCh. 3 - Prob. 31CRCh. 3 - Prob. 32CRCh. 3 - Prob. 33CRCh. 3 - Prob. 34CRCh. 3 - Prob. 35CRCh. 3 - Prob. 36CRCh. 3 - Prob. 37CRCh. 3 - Prob. 38CRCh. 3 - Prob. 39CRCh. 3 - Prob. 40CRCh. 3 - Prob. 41CRCh. 3 - Prob. 42CRCh. 3 - Prob. 43CRCh. 3 - Prob. 44CRCh. 3 - Prob. 45CRCh. 3 - Prob. 46CRCh. 3 - Prob. 47CRCh. 3 - Prob. 48CRCh. 3 - Prob. 49CRCh. 3 - Prob. 50CRCh. 3 - Prob. 51CRCh. 3 - Prob. 52CRCh. 3 - Prob. 53CRCh. 3 - Prob. 54CRCh. 3 - Prob. 55CRCh. 3 - Prob. 56CRCh. 3 - Prob. 57CRCh. 3 - Prob. 58CRCh. 3 - Prob. 59CRCh. 3 - Prob. 60CRCh. 3 - Prob. 61CRCh. 3 - Prob. 62CRCh. 3 - Prob. 63CRCh. 3 - Prob. 64CRCh. 3 - Prob. 65CRCh. 3 - Prob. 66CRCh. 3 - Prob. 67CRCh. 3 - Prob. 68CRCh. 3 - Prob. 69CRCh. 3 - Prob. 70CRCh. 3 - Prob. 71CRCh. 3 - Prob. 72CRCh. 3 - Prob. 73CRCh. 3 - Prob. 74CRCh. 3 - Prob. 75CRCh. 3 - Prob. 76CRCh. 3 - Prob. 77CRCh. 3 - Prob. 78CRCh. 3 - Prob. 79CRCh. 3 - Prob. 80CRCh. 3 - Prob. 81CRCh. 3 - Prob. 82CRCh. 3 - Prob. 83CRCh. 3 - Prob. 84CRCh. 3 - Prob. 85CRCh. 3 - Prob. 86CRCh. 3 - Prob. 87CRCh. 3 - Prob. 88CRCh. 3 - Prob. 89CRCh. 3 - Prob. 90CRCh. 3 - Prob. 91CRCh. 3 - Prob. 92CRCh. 3 - Prob. 93CRCh. 3 - Prob. 94CRCh. 3 - Prob. 95CRCh. 3 - Prob. 96CRCh. 3 - Prob. 97CRCh. 3 - Prob. 98CRCh. 3 - Prob. 99CRCh. 3 - Prob. 100CRCh. 3 - Prob. 101CRCh. 3 - Prob. 102CRCh. 3 - Prob. 103CRCh. 3 - Prob. 104CRCh. 3 - Prob. 105CRCh. 3 - Prob. 106CRCh. 3 - Prob. 107CRCh. 3 - Prob. 108CRCh. 3 - Prob. 109CRCh. 3 - Prob. 110CRCh. 3 - Prob. 111CRCh. 3 - Prob. 112CRCh. 3 - Prob. 113CRCh. 3 - Prob. 114CRCh. 3 - Prob. 115CRCh. 3 - Prob. 116CRCh. 3 - Prob. 117CRCh. 3 - Prob. 118CRCh. 3 - Prob. 119CRCh. 3 - Prob. 120CRCh. 3 - Prob. 121CRCh. 3 - Prob. 122CRCh. 3 - Prob. 123CRCh. 3 - Prob. 124CRCh. 3 - Prob. 125CRCh. 3 - Prob. 126CRCh. 3 - Prob. 1CTCh. 3 - Prob. 2CTCh. 3 - Prob. 3CTCh. 3 - Prob. 4CTCh. 3 - Prob. 5CTCh. 3 - Prob. 6CTCh. 3 - Prob. 7CTCh. 3 - Prob. 8CTCh. 3 - Prob. 9CTCh. 3 - Prob. 10CTCh. 3 - Prob. 11CTCh. 3 - Prob. 12CTCh. 3 - Prob. 13CTCh. 3 - Prob. 14CTCh. 3 - Prob. 15CTCh. 3 - Prob. 16CTCh. 3 - Prob. 17CTCh. 3 - Prob. 18CTCh. 3 - Prob. 19CTCh. 3 - Prob. 20CTCh. 3 - Prob. 21CTCh. 3 - Prob. 22CTCh. 3 - Prob. 23CTCh. 3 - Prob. 24CTCh. 3 - Prob. 25CTCh. 3 - Prob. 26CTCh. 3 - Prob. 27CTCh. 3 - Prob. 28CTCh. 3 - Prob. 29CTCh. 3 - Prob. 30CTCh. 3 - Prob. 1STPCh. 3 - Prob. 2STPCh. 3 - Prob. 3STPCh. 3 - Prob. 4STPCh. 3 - Prob. 5STPCh. 3 - Prob. 6STPCh. 3 - Prob. 7STPCh. 3 - Prob. 8STPCh. 3 - Prob. 9STPCh. 3 - Prob. 10STPCh. 3 - Prob. 11STPCh. 3 - Prob. 12STPCh. 3 - Prob. 13STPCh. 3 - Prob. 14STPCh. 3 - Prob. 15STPCh. 3 - Prob. 16STPCh. 3 - Prob. 17STPCh. 3 - Prob. 18STPCh. 3 - Prob. 19STPCh. 3 - Prob. 20STPCh. 3 - Prob. 21STPCh. 3 - Prob. 22STPCh. 3 - Prob. 23STPCh. 3 - Prob. 24STPCh. 3 - Prob. 25STPCh. 3 - Prob. 26STP
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- Q1. Will you earn more interest amount in two years by depositing $2000 in a simple interest account that pays 6% or in an account that pays 6.15% interest compounded monthly? tarrow_forwardQ4. We want to invest $18000 in an account compounded continuously. How long should the investment be kept so final value of the account reaches $25000 if the annual rate of interest is 5.8%?arrow_forwardQ3. Determine the effective annual yield for each investment below. Then select the better investment. Assume 365 days in a year. a) 5.6% compounded semiannually; b) 5.4% compounded daily.arrow_forward
- Q2. You deposit $22,000 in an account that pays 4.8% interest compounded monthly. a. Find the future value after six years. & b b. Determine the effective annual yield of this account.arrow_forward18. Using the method of variation of parameter, a particular solution to y′′ + 16y = 4 sec(4t) isyp(t) = u1(t) cos(4t) + u2(t) sin(4t). Then u2(t) is equal toA. 1 B. t C. ln | sin 4t| D. ln | cos 4t| E. sec(4t)arrow_forwardQuestion 4. Suppose you need to know an equation of the tangent plane to a surface S at the point P(2, 1, 3). You don't have an equation for S but you know that the curves r1(t) = (2 + 3t, 1 — t², 3 − 4t + t²) r2(u) = (1 + u², 2u³ − 1, 2u + 1) both lie on S. (a) Check that both r₁ and r2 pass through the point P. 1 (b) Give the expression of the 074 in two ways Ət ⚫ in terms of 32 and 33 using the chain rule მყ ⚫ in terms of t using the expression of z(t) in the curve r1 (c) Similarly, give the expression of the 22 in two ways Əz ди ⚫ in terms of oz and oz using the chain rule Əz მყ • in terms of u using the expression of z(u) in the curve r2 (d) Deduce the partial derivative 32 and 33 at the point P and the equation of მე მყ the tangent planearrow_forward
- Coast Guard Patrol Search Mission The pilot of a Coast Guard patrol aircraft on a search mission had just spotted a disabled fishing trawler and decided to go in for a closer look. Flying in a straight line at a constant altitude of 1000 ft and at a steady speed of 256 ft/s, the aircraft passed directly over the trawler. How fast (in ft/s) was the aircraft receding from the trawler when it was 1400 ft from the trawler? (Round your answer to one decimal places.) 1000 ft 180 × ft/s Need Help? Read It SUBMIT ANSWERarrow_forward6. The largest interval in which the solution of (cos t)y′′ +t^2y′ − (5/t)y = e^t/(t−3) , y(1) = 2, y′(1) = 0is guaranteed to exist by the Existence and Uniqueness Theorem is:A. (0, ∞) B. (π/2, 3) C. (0,π/2) D. (0, π) E. (0, 3)arrow_forward12. For the differential equation in the previous question, what is the correct form for a particularsolution?A. yp = Ae^t + Bt^2 B. yp = Ae^t + Bt^2 + Ct + DC. yp = Ate^t + Bt^2 D. yp = Ate^t + Bt^2 + Ct + D Previous differential equation y′′ − 4y′ + 3y = e^t + t^2arrow_forward
- 16. The appropriate form for the particular solution yp(x) of y^(3) − y′′ − 2y′ = x^2 + e^2x isA. yp(x) = Ax^2 + Bx + C + De^2x B. yp(x) = Ax^3 + Bx^2 + Cx + Dxe^2xC. yp(x) = Ax^2 +Be^2x D. yp(x) = A+Be^2x +Ce^−x E. yp(x) = Ax^2 +Bx+C +(Dx+E)e^2xarrow_forwardDistance Between Two Ships Two ships leave the same port at noon. Ship A sails north at 17 mph, and ship B sails east at 11 mph. How fast is the distance between them changing at 1 p.m.? (Round your answer to one decimal place.) 20.3 X mph Need Help? Read It Watch It SUBMIT ANSWERarrow_forwardpractice problem please help!arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendentals
Calculus
ISBN:9781285741550
Author:James Stewart
Publisher:Cengage Learning
Thomas' Calculus (14th Edition)
Calculus
ISBN:9780134438986
Author:Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:9780134763644
Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:PEARSON
Calculus: Early Transcendentals
Calculus
ISBN:9781319050740
Author:Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:W. H. Freeman
Calculus: Early Transcendental Functions
Calculus
ISBN:9781337552516
Author:Ron Larson, Bruce H. Edwards
Publisher:Cengage Learning
Evaluating Indefinite Integrals; Author: Professor Dave Explains;https://www.youtube.com/watch?v=-xHA2RjVkwY;License: Standard YouTube License, CC-BY
Calculus - Lesson 16 | Indefinite and Definite Integrals | Don't Memorise; Author: Don't Memorise;https://www.youtube.com/watch?v=bMnMzNKL9Ks;License: Standard YouTube License, CC-BY