Chapter 19.4, Problem 19.108P

The crude-oil pumping rig shown is driven at 20 rpm. The inside diameter of the well pipe is 2 in., and the diameter of the pump rod is 0.75 in. The length of the pump rod and the length of the column of oil lifted during the stroke are essentially the same, and equal to 6000 ft. During the downward stroke, a valve at the lower end of the pump rod opens to let a quantity of oil into the well pipe, and the column of oil is then lifted to obtain a discharge into the connecting pipeline. Thus, the amount of oil pumped in a given time depends upon the stroke of the lower end of the pump rod. Knowing that the upper end of the rod at D is essentially sinusoidal with a stroke of 45 in. and the specific weight of crude oil is 56.2 lb/ft³, determine (a) the output of the well in ft³/min if the shaft is rigid, (b) the output of the well in ft³/min if the stiffness of the rod is 2210 N/m, the equivalent mass of the oil and shaft is 290 kg, and damping is negligible.

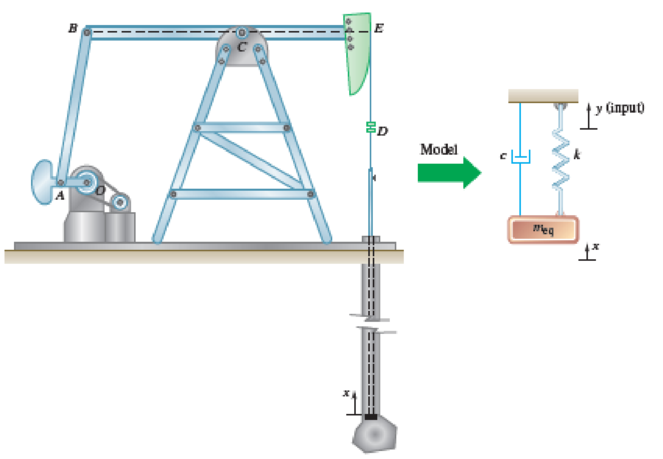
Fig. P19.108

To determine

Find the output of the well $\left(V_{\text{displaced}}\right)$ if the shaft is rigid.

Answer to Problem 19.108P

The output of the well $\left(V_{\text{displaced}}\right)$ if the shaft is rigid is $\underset{\_}{1.406{\text{ft}}^3/\min }$.

Explanation of Solution

Given information:

The forced circular frequency $\left({\omega }_f\right)$ is $20\text{rpm}$.

The diameter of the well pipe $\left(d_{pipe}\right)$ is $2\text{in}\text{.}$.

The diameter of the pump rod $\left(d_{pumprod}\right)$ is $\text{0}\text{.75in}\text{.}$.

The stroke of the upper end of the rod $\left(s_{upperrod}\right)$ is $\text{45in}\text{.}$.

The specific weight of the crude oil $\left({\gamma }_{crude}\right)$ is $56.2{\text{lb/ft}}^{\text{3}}$.

Calculation:

The periodic force frequency is the frequency at which the crude oil is pumped. The speed of the crude oil pump is 20 rpm.

Calculate the periodic force frequency $\left({\omega }_f\right)$ using the relation:

${\omega }_f=\frac{2\pi N}{60}$

Here, N is the speed of the pump.

Substitute $20\text{rpm}$ for N.

$\begin{array}{c}{\omega }_f=\frac{2\pi \left(20\text{rpm}\right)}{60}\\ =2.0944\text{rad}/\text{s}\end{array}$

The oil flows in the pipe between the pump rod and pipe walls. The diameter of the well pipe is 2 in. and that of the pump rod is 0.75 inch. Thus, the oil flow area is the annular area between the pump rod area and pipe area.

Calculate the oil flow area $\left(A_{oil}\right)$ using the formula:

$A_{oil}=\frac{\pi }{4}\left(d_{pipe}^2-d_{pumprod}^2\right)$

Substitute $2\text{in}\text{.}$ for $d_{pipe}$ and $\text{0}\text{.75in}\text{.}$ for $d_{pumprod}$.

$\begin{array}{c}{A}_{oil}=\frac{\pi }{4}\left({\left(\text{2}\text{in}\text{.}\right)}^2-{\left(\text{0}\text{.75}\text{in}\text{.}\right)}^2\right)\\ =2.6998\text{in}{\text{.}}^2\\ =2.6998\text{in}{\text{.}}^2\times \frac{1{\text{ft}}^2}{144\text{in}{\text{.}}^2}\\ =0.0187{\text{ft}}^2\end{array}$

The system is analogous to the forced vibration system where the vibration is due to simple harmonic motion of the support. The stroke of the lower end of the pump is proportional to the amplitude of the vibration motion.

The expression for the relation between stroke of the pump and the amplitude of vibration as follows:

$s=2x_m$ (1)

Here, s is the stroke of the pump and $x_m$ is the amplitude of forced vibration.

Calculate the volume of the oil $\left(V_{oil}\right)$ displaced per revolution using the formula:

$V_{oil}=A_{oil}s$

Substitute $0.0187{\text{ft}}^2$ for $A_{oil}$.

$\begin{array}{c}{V}_{oil}=\left(0.0187{\text{ft}}^2\right)s\\ =0.0187s\end{array}$ (2)

The system is analogous to the forced vibration system where the vibration is due to simple harmonic motion of the support. In the system, the vibration is due to the sinusoidal displacement of the upper end of the rod. The stroke of the sinusoidal motion of the upper end of the rod is 45 inch.

Calculate the magnitude of the static deflection $\left({\delta }_m\right)$ using the relation:

${\delta }_m=\frac{1}{2}{s}_{upperend}$

Substitute $\text{45in}\text{.}$ for $s_{upperrod}$.

$\begin{array}{c}{\delta }_m=\frac{1}{2}\left(45\text{in}\text{.}\right)\\ =22.5\text{in}\text{.}\\ =22.5\text{in}\text{.}\times \frac{1\text{ft}}{12\text{in}\text{.}}\\ =1.875\text{ft}\end{array}$

For rigid shaft:

The expression for the amplitude of the forced vibration $\left(x_m\right)$ as follows:

$x_m=\frac{{\delta }_m}{1-{\left(\frac{{\omega }_f}{{\omega }_n}\right)}^2}$ (3)

Here, ${\omega }_n$ is the natural circular frequency.

For the rigid shaft, the spring constant is infinite and thus the natural frequency of the rod is infinite. The equation (3) implies that the resulting amplitude of forced vibration, when the natural frequency is infinite, is the amplitude of the forced vibration $\left(x_m\right)$ equal to magnitude of static deflection $\left({\delta }_m\right)$. Thus, the amplitude of the forced vibration $\left(x_m\right)$ is $1.875\text{ft}$.

Calculate the stroke (s) using the relation:

Substitute $1.875\text{ft}$ for $x_m$ in equation (1).

$\begin{array}{c}s=2x_m\\ =2\left(1.875\text{ft}\right)\\ =3.75\text{ft}\end{array}$

Calculate the volume of the oil $\left(V_{oil}\right)$ displaced per revolution using the relation:

Substitute $3.75\text{ft}$ for s in equation (2).

$\begin{array}{c}{V}_{oil}=0.0187s\\ =0.0187\left(3.75\text{ft}\right)\\ =0.0703{\text{ft}}^3\end{array}$

The speed of the pump is $20\text{rpm}$ and the volume displaced per revolution is $0.0703{\text{ft}}^3$. Thus, the total volume displaced is the product of pump speed and volume displaced per revolution.

Calculate the total volume displaced $\left(V_{\text{displaced}}\right)$ using the formula:

$V_{\text{displaced}}=N{V}_{oil}$ (4)

Substitute $20\text{rpm}$ for N and $0.0703{\text{ft}}^3$ for $V_{oil}$.

$\begin{array}{c}{V}_{\text{displaced}}=\left(20\text{rpm}\right)\left(0.0703{\text{ft}}^3\right)\\ =1.406{\text{ft}}^3/\min \end{array}$

Therefore, the output of the well $\left(V_{\text{displaced}}\right)$ if the shaft is rigid is $\underset{\_}{1.406{\text{ft}}^3/\min }$.

To determine

Find the output of the well $\left(V_{\text{displaced}}\right)$.

Answer to Problem 19.108P

The output of the well $\left(V_{\text{displaced}}\right)$ is $\underset{\_}{3.31{\text{ft}}^3/\min }$.

Explanation of Solution

Given information:

The forced circular frequency $\left({\omega }_f\right)$ is $20\text{rpm}$.

The diameter of the well pipe $\left(d_{pipe}\right)$ is $2\text{in}\text{.}$.

The diameter of the pump rod $\left(d_{pumprod}\right)$ is $\text{0}\text{.75in}\text{.}$.

The stroke of the upper end of the rod $\left(s_{upperrod}\right)$ is $\text{45in}\text{.}$

The specific weight of the crude oil $\left({\gamma }_{crude}\right)$ is $56.2{\text{lb/ft}}^{\text{3}}$.

The stiffness of the rod (k) is $2210\text{N/m}$.

The mass of the oil (m) is $290\text{kg}$.

Calculation:

Flexible shaft of stiffness:

Calculate the natural circular frequency $\left({\omega }_n\right)$ using the formula:

${\omega }_n=\sqrt{\frac{k}{m}}$

Substitute $\text{2,210}\text{N}/\text{m}$ for k and $290\text{kg}$ for m.

$\begin{array}{c}{\omega }_n=\sqrt{\frac{\text{2210}\text{N}/\text{m}}{290\text{kg}}}\\ =2.7606\text{rad}/\text{s}\end{array}$

Calculate the amplitude of forced vibration $\left(x_m\right)$ using the formula:

Substitute $1.875\text{ft}$ for ${\delta }_m$, $2.0944\text{rad}/\text{s}$ for ${\omega }_f$, and $2.7606\text{rad}/\text{s}$ for ${\omega }_n$ in equation (3).

$\begin{array}{c}{x}_m=\frac{1.875\text{ft}}{1-{\left(\frac{2.0944\text{rad}/\text{s}}{2.7606\text{rad}/\text{s}}\right)}^2}\\ =\frac{1.875}{0.424}\\ =4.42\text{ft}\end{array}$

Calculate the stroke (s) using the relation:

Substitute $1.875\text{ft}$ for $x_m$ in equation (1).

$\begin{array}{c}s=2x_m\\ =2\left(4.42\text{ft}\right)\\ =8.84\text{ft}\end{array}$

Calculate the volume of the oil $\left(V_{oil}\right)$ displaced per revolution using the relation:

Substitute $8.84\text{ft}$ for s in equation (2).

$\begin{array}{c}{V}_{oil}=0.0187s\\ =0.0187\left(8.84\text{ft}\right)\\ =0.1657{\text{ft}}^3\end{array}$

Calculate the total volume displaced $\left(V_{\text{displaced}}\right)$ using the formula:

Substitute $20\text{rpm}$ for N and $0.1657{\text{ft}}^3$ for $V_{oil}$ in equation (4).

$\begin{array}{c}{V}_{\text{displaced}}=N{V}_{oil}\\ =\left(20\text{rpm}\right)\left(0.1657{\text{ft}}^3\right)\\ =3.31{\text{ft}}^3/\min \end{array}$

Therefore, the output of the well $\left(V_{\text{displaced}}\right)$ is $\underset{\_}{3.31{\text{ft}}^3/\min }$.