
(a)
Sketch
(a)
Answer to Problem 9E
The plot
Explanation of Solution
Given data:
Refer to Figure 17.29 in the textbook.
Formula used:
Write the general expression for Fourier series expansion.
Write the general expression for Fourier series coefficient
Write the general expression for Fourier series coefficient
Write the general expression for Fourier series coefficient
Write the expression to calculate the fundamental angular frequency.
Here,
Calculation:s
In the given Figure 17.29, the time period is
The function
Substitute 4 for T in equation (5) to find
Applying equation (6) in equation (2) to find
Simplify the above equation as follows,
Applying equation (6) in equation (3) to finding the Fourier coefficient
Equation (8) is simplified as,
Therefore, equation (8) becomes,
Consider the function,
Consider the following integration formula.
Compare the equations (10) and (11) to simplify the equation (10).
Using the equation (11), the equation (10) can be reduced as,
Simplify the above equation as follows,
Consider the function,
Compare the equations (12) and (11) to simplify the equation (12).
Using the equation (11), the equation (12) can be reduced as,
Substitute the value of x and y in equation (9) as follows,
The above equation becomes,
Applying equation (6) in equation (4) to finding the Fourier coefficient
Equation (14) is simplified as,
Therefore, equation (14) becomes,
Consider the function,
Consider the following integration formula.
Compare the equations (17) and (16) to simplify the equation (16).
Using the equation (17), the equation (16) can be reduced as,
Simplify the above equation as follows,
Consider the function,
Compare the equations (17) and (18) to simplify the equation (18).
Using the equation (17), the equation (18) can be reduced as,
Substitute the values of m and n in equation (15) as follows,
The above equation becomes,
Substitute the values of
The above equation becomes,
For
Therefore, equation (19) will be as follows,
For
Therefore, equation (19) will be as follows,
For
Therefore, equation (19) will be as follows,
Similarly, for
Therefore, equation (19) will be as follows,
Write the MATLAB Code to plot
t=linspace(-3,5,1000);
g0=0;
N=40;
for i=1:1000;
y3=0.193*cos(pi*t/2) + 0.419*cos(pi*t) - 0.167*cos(3*pi*t/2);
y5=0.193*cos(pi*t/2) + 0.419*cos(pi*t) - 0.167*cos(3*pi*t/2) + 0.184*cos(2*pi*t) - 0.143*cos(5*pi*t/2);
end
for i=1:1000;
sum=0;
for n=1:N;
sum=sum+((6*(-1)^n - 2*cos(3*n*pi))/(n*pi)^2)*cos(n*pi*t(i)/2) + (((-1)^n + cos(3*n*pi))/(n*pi))*cos(n*pi*t(i)/2);
end
gt(i)=g0+sum;
end
plot(t,y3,'b', t,y5,'r', t,gt,'g')
legend({'y3','y5','gt'},'Location','best')
xlabel('Time t in sec')
ylabel('The values y3, y5, and gt')
title('Plots for y3, y5, and gt')
Matlab output:
Figure 1 shows the plot
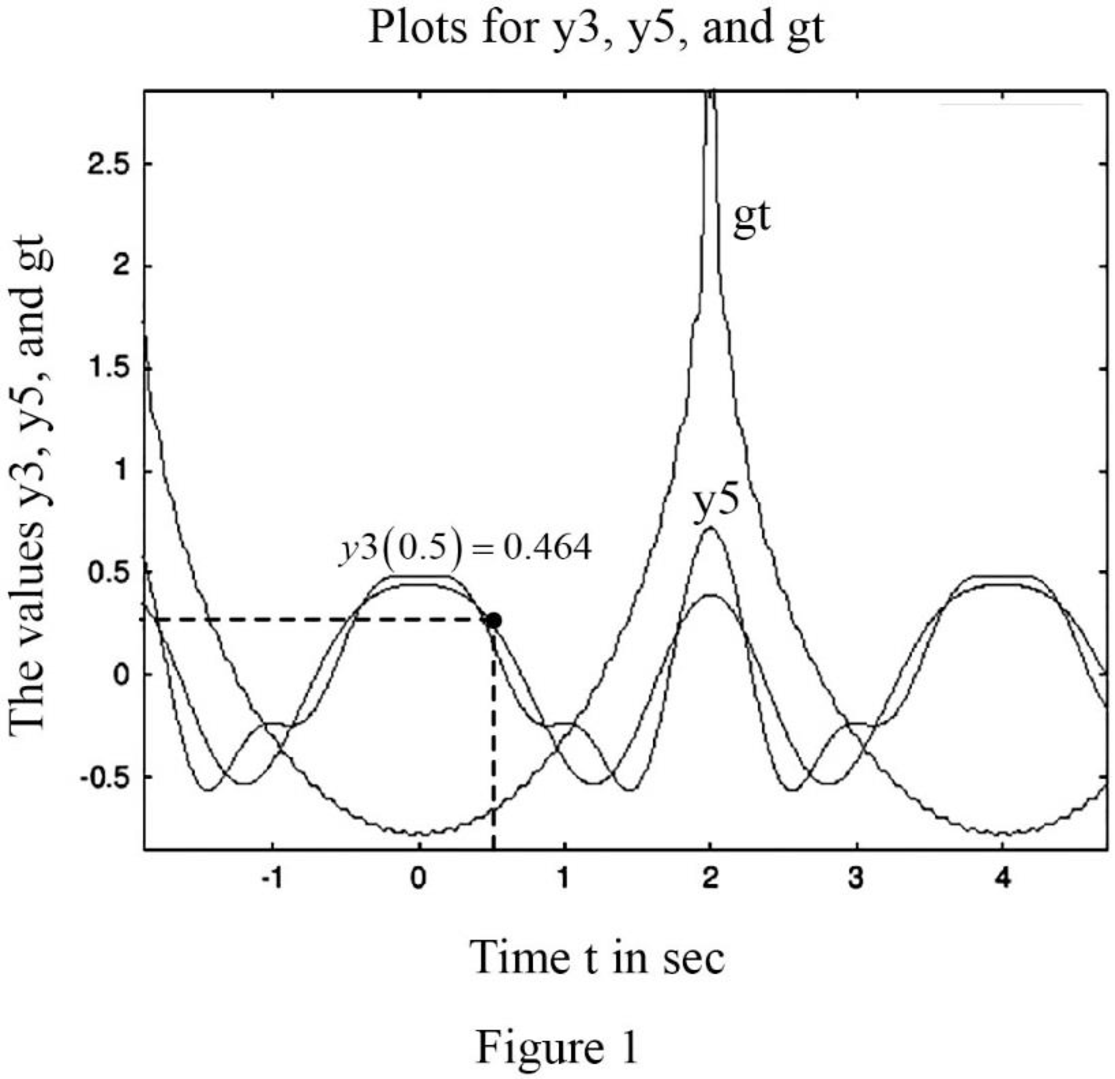
Conclusion:
Thus, the plot
(b)
Find the values of
(b)
Answer to Problem 9E
The values of
Explanation of Solution
Given data:
Refer to Figure 17.29 in the textbook.
Calculation:
From Part (a), the function
Finding
The value of
From Part (a),
Finding
From Part (a),
Finding
From part (a),
Finding
The value of
Finding
Conclusion:
Thus, the values of
Want to see more full solutions like this?
Chapter 17 Solutions
Loose Leaf for Engineering Circuit Analysis Format: Loose-leaf
- Please solve these 3 questions in detailarrow_forward1. Please draw the root locus by hand for the following closed-loop system, where G(s) s+8 S-2 and H(s) = Find the range of K for stability Input R(s) Output C(s) KG(s) H(s) s+6 = S-2arrow_forwardThe state-space Jordan Canonical Form of the following system is: Y(s) 8-5 U(s) (+1)(+3) Select one: O a. -1 0 0 A = 0 -1 0 B: ... ... ... 0 0 C [4 1.5 1.5], D=0 b. -3 1 0 0 A = 0 -3 0 1 B ... 0 0 -1 C -4 -1.5 1.5], D=0 ○ C. -3 1 0 A = 0 -3 0 1 ,B= ... 0 0 ○ d. C [4 1.5 1.5], D=0 -3 1 0 0 A = 0 -3 0 1 , B: ... ... 0 0 -1 C [4 1.5 1.5], D=0 -4 1 If= x and (0): = then 2(t) is: -4 0 Select one: a. x2(t)=4te2t O b. x2(t) = e2t+2te2t Oc. 2(t)=-4te-21 Od. 2(t) e2-2te-2 =arrow_forward
- Three speech signals are TDM multiplexed with a high-quanty music signal. It each speech signal is sampled at 16 kHz and PCM quantized by 8 bits/sample, while the music signal is sampled at 64 kHz with the same PCM quantizer. 1. Draw the block diagram of this TDM. 2. Calculate the output bit rate of this TDM.arrow_forward3- For the network below determine the value of R for maximum power to R (use Thevenin equivalent) and determine the value of maximum power R₁ 1.2Ω E + 12 V I D 10 A R₂60 6Ω Rarrow_forwardPlease solve this problem in detail to understandarrow_forward
- Q3: (40 Marks) Single phase full bridge voltage source inverter has an RLC load with R-1002, L-31.5mH and C=112µF. The inverter frequency is 60Hz and de input voltage is 220V. (a) Express the instantaneous load current in Fourier series to third harmonic. (b) Calculate the RMS load current at the fundamental frequency (n=1). (c) Calculate the load power due to fundamental component (n=1).arrow_forward12.3 Express each of the waveforms in Fig. P12.3 (on page 667) in terms of step functions and then determine its Laplace transform. [Recall that the ramp function is related to the step function by r(t − T) = (t − T) u(t − T).] Assume that all waveforms are zero for t<0. - - -arrow_forwardEvaluate each of the following integraarrow_forward
- With the aid of suitable diagrams, describe the benefits that antenna arrays have over singleelement antennas, with their applicationsarrow_forwardExplain what is meant by an electric dipole antenna, sketch its radiation pattern, state itsdirectivity and describe its main applicationsarrow_forwardEstimate the length required for a half-waveelectric dipole antenna for transmitting/receiving EM waves at 800 MHz (this is in the UHFbandwidth of 470 to 860 MHz, used for UK TV transmissions).arrow_forward
 Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON
Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning
Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education
Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education
Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON
Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,
Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,





