
Disk B is at rest when it is brought into contact with disk A, which has an initial angular velocity ω0. (a) Show that the final angular velocities of the disks are independent of the coefficient of friction μk between the disks as long as μk ≠ 0. (b) Express the final angular velocity of disk A in terms of ω0 and the ratio of the masses of the two disks, mA/mB.
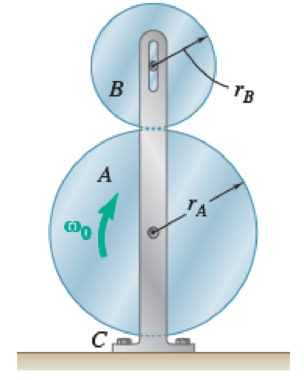
Fig. P16.43 and P16.44
(a)
Show that the final velocities of disk A and B are independent of coefficient of kinetic friction
Explanation of Solution
The mass of the disk A is
The mass of the disk B is
The initial angular velocity of the disk A is
The coefficient of the kinetic friction is
The radius of the disk A is
The radius of the disk B is
The acceleration due to gravity is g.
The time required for the disk to come to rest is t.
Calculation:
Calculate the mass moment of inertia of the disk A
Calculate the mass moment of inertia of the disk B
Calculate the load of the disk A
Calculate the load of the disk B
Show the free body diagram of the disk B as in Figure 1.
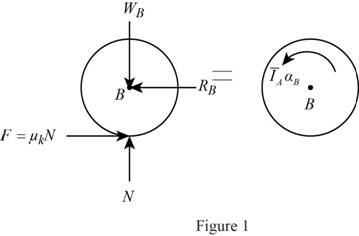
Here,
Refer to Figure 1.
Calculate the vertical forces by applying the equation of equilibrium:
Sum of vertical forces is equal to 0.
Substitute
Calculate the magnitude of the friction force
Substitute
Calculate the horizontal forces by applying the equation of equilibrium:
Sum of horizontal forces is equal to 0.
Substitute
Calculate the angular acceleration of the disk B
Calculate the moment about point B by applying the equation of equilibrium:
Substitute
Show the free body diagram of the disk A as in Figure 2.
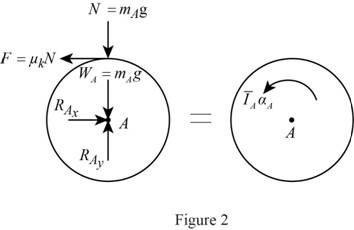
Here,
Refer to Figure 2.
Calculate the horizontal forces by applying the equation of equilibrium:
Sum of horizontal forces is equal to 0.
Substitute
Calculate the vertical forces by applying the equation of equilibrium:
Sum of vertical forces is equal to 0.
Substitute
Calculate the angular acceleration of the disk A
Calculate the moment about point A by applying the equation of equilibrium:
Substitute
The angular velocity of the disk A
Substitute
The angular velocity of the disk B
Substitute
While there is no slipping between disk A and B, their velocity ratio is same.
Show the free body diagram of the system as in Figure 3.
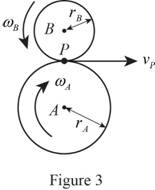
Refer to Figure 3.
The velocity of pinion
Substitute
Calculate the angular velocity of the disk A
Substitute
Calculate the angular velocity of the disk B
Substitute
From Equation (5) and (6), it is clear that the final velocities of disk A and B are independent of coefficient of kinetic friction
(b)
Express the final angular velocity of disk A in terms of
Answer to Problem 16.44P
The final angular velocity of disk A in terms of
Explanation of Solution
The mass of the disk A is
The mass of the disk B is
The initial angular velocity of the disk A is
The coefficient of the kinetic friction is
The radius of the disk A is
The radius of the disk B is
The acceleration due to gravity is g.
The time required for the disk to come to rest is t.
Calculation:
Refer to part (a).
Calculate the final angular velocity of disk A in terms of
Refer Equation (5).
Hence, the final angular velocity of disk A in terms of
Want to see more full solutions like this?
Chapter 16 Solutions
VECTOR MECH...,STAT.+DYN.(LL)-W/ACCESS
Additional Engineering Textbook Solutions
Elementary Surveying: An Introduction To Geomatics (15th Edition)
Thermodynamics: An Engineering Approach
Electric Circuits. (11th Edition)
Database Concepts (8th Edition)
Modern Database Management
Automotive Technology: Principles, Diagnosis, And Service (6th Edition) (halderman Automotive Series)
- Auto Controls A union feedback control system has the following open loop transfer function where k>0 is a variable proportional gain i. for K = 1 , derive the exact magnitude and phase expressions of G(jw). ii) for K = 1 , identify the gaincross-over frequency (Wgc) [where IG(jo))| 1] and phase cross-overfrequency [where <G(jw) = - 180]. You can use MATLAB command "margin" to obtain there quantities. iii) Calculate gain margin (in dB) and phase margin (in degrees) ·State whether the closed-loop is stable for K = 1 and briefly justify your answer based on the margin . (Gain marginPhase margin) iv. what happens to the gain margin and Phase margin when you increase the value of K?you You can use for loop in MATLAB to check that.Helpful matlab commands : if, bode, margin, rlocus NO COPIED SOLUTIONSarrow_forwardAuto Controls Hand sketch the root Focus of the following transfer function How many asymptotes are there ?what are the angles of the asymptotes?Does the system remain stable for all values of K NO COPIED SOLUTIONSarrow_forward-400" 150" in Datum 80" 90" -280"arrow_forward
- 7) Please draw the front, top and side view for the following object. Please cross this line outarrow_forwardA 10-kg box is pulled along P,Na rough surface by a force P, as shown in thefigure. The pulling force linearly increaseswith time, while the particle is motionless att = 0s untilit reaches a maximum force of100 Nattimet = 4s. If the ground has staticand kinetic friction coefficients of u, = 0.6 andHU, = 0.4 respectively, determine the velocityof the A 1 0 - kg box is pulled along P , N a rough surface by a force P , as shown in the figure. The pulling force linearly increases with time, while the particle is motionless at t = 0 s untilit reaches a maximum force of 1 0 0 Nattimet = 4 s . If the ground has static and kinetic friction coefficients of u , = 0 . 6 and HU , = 0 . 4 respectively, determine the velocity of the particle att = 4 s .arrow_forwardCalculate the speed of the driven member with the following conditions: Diameter of the motor pulley: 4 in Diameter of the driven pulley: 12 in Speed of the motor pulley: 1800 rpmarrow_forward
- 4. In the figure, shaft A made of AISI 1010 hot-rolled steel, is welded to a fixed support and is subjected to loading by equal and opposite Forces F via shaft B. Stress concentration factors K₁ (1.7) and Kts (1.6) are induced by the 3mm fillet. Notch sensitivities are q₁=0.9 and qts=1. The length of shaft A from the fixed support to the connection at shaft B is 1m. The load F cycles from 0.5 to 2kN and a static load P is 100N. For shaft A, find the factor of safety (for infinite life) using the modified Goodman fatigue failure criterion. 3 mm fillet Shaft A 20 mm 25 mm Shaft B 25 mmarrow_forwardPlease sovle this for me and please don't use aiarrow_forwardPlease sovle this for me and please don't use aiarrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





