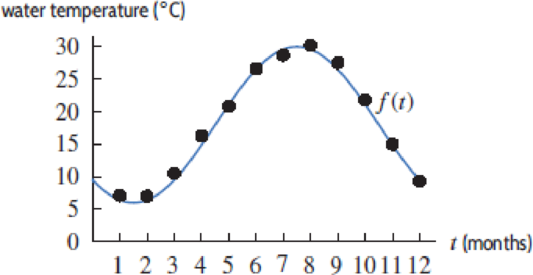
Problem 1E: For Exercises 19, draw the angle using a ray through the origin, and determine whether the sine,... Problem 2E: For Exercises 19, draw the angle using a ray through the origin, and determine whether the sine,... Problem 3E: For Exercises 19, draw the angle using a ray through the origin, and determine whether the sine,... Problem 4E: For Exercises 19, draw the angle using a ray through the origin, and determine whether the sine,... Problem 5E: For Exercises 19, draw the angle using a ray through the origin, and determine whether the sine,... Problem 6E: For Exercises 19, draw the angle using a ray through the origin, and determine whether the sine,... Problem 7E: For Exercises 19, draw the angle using a ray through the origin, and determine whether the sine,... Problem 8E: For Exercises 19, draw the angle using a ray through the origin, and determine whether the sine,... Problem 9E: For Exercises 19, draw the angle using a ray through the origin, and determine whether the sine,... Problem 10E: Find the period and amplitude in Exercises 1013. y=7sin(3t) Problem 11E: Find the period and amplitude in Exercises 1013. z=3cos(u/4)+5 Problem 12E: Find the period and amplitude in Exercises 1013. w=84sin(2x+) Problem 13E: Find the period and amplitude in Exercises 1013. r=0.1sin(t)+2 Problem 14E: For Exercises 1423, find a possible formula for each graph. Problem 15E: For Exercises 1423, find a possible formula for each graph. Problem 16E: For Exercises 1423, find a possible formula for each graph. Problem 17E: For Exercises 1423, find a possible formula for each graph. Problem 18E: For Exercises 1423, find a possible formula for each graph. Problem 19E: For Exercises 1423, find a possible formula for each graph. Problem 20E: For Exercises 1423, find a possible formula for each graph. Problem 21E: For Exercises 1423, find a possible formula for each graph. Problem 22E: For Exercises 1423, find a possible formula for each graph. Problem 23E: For Exercises 1423, find a possible formula for each graph. Problem 24E: In Exercises 2429, calculate the quantity without using the the trigonometric functions on a... Problem 25E: In Exercises 2429, calculate the quantity without using the the trigonometric functions on a... Problem 26E: In Exercises 2429, calculate the quantity without using the the trigonometric functions on a... Problem 27E: In Exercises 2429, calculate the quantity without using the the trigonometric functions on a... Problem 28E: In Exercises 2429, calculate the quantity without using the the trigonometric functions on a... Problem 29E: In Exercises 2429, calculate the quantity without using the the trigonometric functions on a... Problem 30E: In Exercises 3034, find a solution to the equation if possible. Give the answer in exact form and in... Problem 31E: In Exercises 3034, find a solution to the equation if possible. Give the answer in exact form and in... Problem 32E: In Exercises 3034, find a solution to the equation if possible. Give the answer in exact form and in... Problem 33E: In Exercises 3034, find a solution to the equation if possible. Give the answer in exact form and in... Problem 34E: In Exercises 3034, find a solution to the equation if possible. Give the answer in exact form and in... Problem 35E: What is the period of the earths revolution around the sun? Problem 36E: What is the approximate period of the moons revolution around the earth? Problem 37E: When a cars engine makes less than about 200 revolutions per minute, it stalls. What is the period... Problem 38E: A compact disc spins at a rate of 200 to 500 revolutions per minute. What are the equivalent rates... Problem 39E: Find the angle, in degrees, that a wheelchair ramp makes with the ground if the ramp rises 1 foot... Problem 40E: (a) Use a graphing calculator or computer to estimate the period of 2 sin + 3 cos(2). (b) Explain... Problem 41E: Without a calculator or computer, match the formulas with the graphs in Figure 1.72.... Problem 42E: Figure 1.73 shows four periodic functions of the family f(t)=Acos(B(th)), all with the same... Problem 43E: In Problems 4349, graph the given function on the axes in Figure 1.74. Figure 1.74 y=ksinx Problem 44E: In Problems 4349, graph the given function on the axes in Figure 1.74. Figure 1.74 y=kcosx Problem 45E: In Problems 4349, graph the given function on the axes in Figure 1.74. Figure 1.74 y=k(cosx)+k Problem 46E: In Problems 4349, graph the given function on the axes in Figure 1.74. Figure 1.74 y=k(sinx)k Problem 47E: In Problems 4349, graph the given function on the axes in Figure 1.74. Figure 1.74 y=ksin(xk) Problem 48E: In Problems 4349, graph the given function on the axes in Figure 1.74. Figure 1.74 y=kcos(x+k) Problem 49E: In Problems 4349, graph the given function on the axes in Figure 1.74. Figure 1.74 y=ksin(2x/k) Problem 50E: For Problems 5053, use Figure 1.75 to estimate the given value for f(t)=Acos(B(th))+C, which... Problem 51E: For Problems 5053, use Figure 1.75 to estimate the given value for f(t)=Acos(B(th))+C, which... Problem 52E: For Problems 5053, use Figure 1.75 to estimate the given value for f(t)=Acos(B(th))+C, which... Problem 53E: For Problems 5053, use Figure 1.75 to estimate the given value for f(t)=Acos(B(th))+C, which... Problem 54E: For Problems 5457, use Figure 1.75 to estimate the given value for f(t) = A sin(B(t )) + C, which... Problem 55E: For Problems 5457, use Figure 1.75 to estimate the given value for f(t) = A sin(B(t )) + C, which... Problem 56E: For Problems 5457, use Figure 1.75 to estimate the given value for f(t) = A sin(B(t )) + C, which... Problem 57E: For Problems 5457, use Figure 1.75 to estimate the given value for f(t) = A sin(B(t )) + C, which... Problem 58E: The visitors guide to St. Petersburg, Florida, contains the chart shown in Figure 1.77 to advertise... Problem 59E: What is the difference between sin x2, sin2 x, and sin(sin x)? Express each of the three as a... Problem 60E: On the graph of y = sin x, points P and Q are at consecutive lowest and highest points. Find the... Problem 61E: A population of animals oscillates sinusoidally between a low of 700 on January 1 and a high of 900... Problem 62E: The desert temperature, H, oscillates daily between 40F at 5 am and 80F at 5 pm. Write a possible... Problem 63E: The depth of water in a tank oscillates sinusoidally once every 6 hours. If the smallest depth is... Problem 64E: The voltage, V, of an electrical outlet in a home as a function of time, t (in seconds), is... Problem 65E: The power output, P, of a solar panel varies with the position of the sun. Let P = 10 sin watts,... Problem 66E: A baseball hit at an angle of to the horizontal with initial velocity v0 has horizontal range, R,... Problem 67E: (a) Match the functions = f(t), = g(t), = (t), = k(t), whose values are in the table, with the... Problem 68E: For a boat to float in a tidal bay, the water must be at least 2.5 meters deep. The depth of water... Problem 69E: The Bay of Fundy in Canada has the largest tides in the world. The difference between low and high... Problem 70E: Match graphs A-D in Figure 1.78 with the functions below. Assume a, b, c and d are positive... Problem 71E: In Figure 1.79, the blue curve shows monthly mean carbon dioxide (CO2) concentration, in parts per... Problem 72E: Find the area of the trapezoidal cross-section of the irrigation canal shown in Figure 1.80. Figure... Problem 73E: Graph y = sin x, y = 0.4, and y = 0.4. (a) From the graph, estimate to one decimal place all the... Problem 74E: This problem introduces the arccosine function, or inverse cosine, denoted by cos1 on most... Problem 75E: In Problems 7578, explain what is wrong with the statement. The functions f(x) = 3 cos x and g(x) =... Problem 76E: In Problems 7578, explain what is wrong with the statement. For the function f(x) = sin(Bx) with B ... Problem 77E: In Problems 7578, explain what is wrong with the statement. The function y = sin x cos x is periodic... Problem 78E: In Problems 7578, explain what is wrong with the statement. For positive A, B, C, the maximum value... Problem 79E: In Problems 7980, give an example of: A sine function with period 23. Problem 80E: In Problems 7980, give an example of: A cosine function which oscillates between values of 1200 and... Problem 81E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. The family... Problem 82E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. The family... Problem 83E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. The function... Problem 84E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. The function... Problem 85E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. If t is in... Problem 86E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. The function... Problem 87E: Are the statements in Problems 8197 true or false? Give an explanation for your answer.... Problem 88E: Are the statements in Problems 8197 true or false? Give an explanation for your answer.... Problem 89E: Are the statements in Problems 8197 true or false? Give an explanation for your answer.... Problem 90E: Are the statements in Problems 8197 true or false? Give an explanation for your answer.... Problem 91E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. The function... Problem 92E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. The function... Problem 93E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. If f(x) is a... Problem 94E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. If g(x) is a... Problem 95E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. The function... Problem 96E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. sin1(sint)=t... Problem 97E: Are the statements in Problems 8197 true or false? Give an explanation for your answer. The function... format_list_bulleted


 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning


