Thinking Like an Engineer: An Active Learning Approach (4th Edition)
4th Edition
ISBN: 9780134639673
Author: Elizabeth A. Stephan, David R. Bowman, William J. Park, Benjamin L. Sill, Matthew W. Ohland
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 13, Problem 5RQ
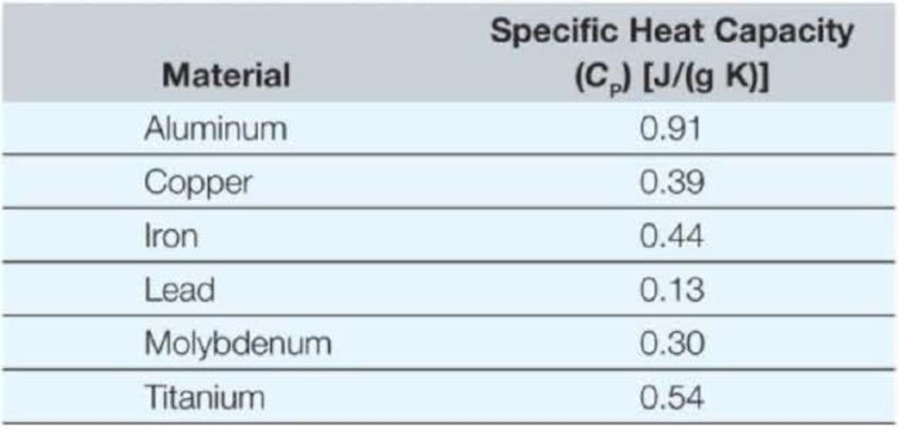
If an object is heated, the temperature of the object will increase. The thermal energy (Q) associated with a change in temperature (ΔT) is a function of the mass of the object (m) and the specific heat (C2). Specific heat is a material property, and values are available in literature. In an experiment, heat is applied to the end of an object, and the temperature change at the other end of the object recorded. An unknown material is tested in the lab, yielding the following results.
- a. Show the resulting data and trendline, with equation and R2 value, on the appropriate graph type (xy scatter, semilog, or log–log) to make the data appear linear.
- b. If the material was titanium, what mass of sample was tested?
- c. If a 4-gram sample was used which of the following materials was tested?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Please can you help with the attached question? Many thanks
Which of the following sequences converge and which diverge?
20) an
=
21) a =
n!
106
1/(Inn)
3n+1
"
22) a =
3n-1
1/n
x"
23) a =
, x>0
2n+1
3" x 6"
24) an
25) a, = tanh(n)
=
2" xn!
n²
1
26) a =
sin
2n-1
n
27) a = tan(n)
1
28) a =
1
3
++
(Inn) 200
2"
29) an
n
30)
=n-√√n²-n
1"1
31) a ==
dx
nix
Which of the following sequences converge and which diverge?
n+1
6)
a =
1-
2n
(-1)+1
7)
a =
2n-1
2n
8)
an
=
n+1
1
9)
a = sin
+
2
n
sin n
10) a =
n
11) an
=
12) a =
13) an
14) an
15) an
16) an
n
2"
In(n+1)
= 81/n
n
n
=(1+7)"
=
=
10n
3
n
1/n
17) an
=
In n
1/n
n'
18) a =√4"n
Chapter 13 Solutions
Thinking Like an Engineer: An Active Learning Approach (4th Edition)
Ch. 13.2 - An unknown amount of oxygen, kept in 8 piston-type...Ch. 13.2 - The data shown graphically in the figure describe...Ch. 13.5 - Prob. 3CCCh. 13.5 - Prob. 4CCCh. 13 - Capillary action draws liquid up a narrow tube...Ch. 13 - Several reactions are carried out in a closed...Ch. 13 - An environmental engineer has obtained a bacteria...Ch. 13 - In a turbine a device used for mixing the power...Ch. 13 - Being quite interested in obsolete electronics,...Ch. 13 - Referring to the previous ICA 13-5, Angus is also...
Ch. 13 - Prob. 7ICACh. 13 - The following instructions apply to ICA 13-7 to...Ch. 13 - The following instructions apply to ICA 13-7 to...Ch. 13 - The following instructions will apply to ICA 13-10...Ch. 13 - The following instructions will apply to ICA 13-10...Ch. 13 - The following instructions will apply to ICA 13-10...Ch. 13 - The following instructions will apply to ICA 13-10...Ch. 13 - The following instructions will apply to ICA 13-10...Ch. 13 - The following instructions will apply to ICA 13-10...Ch. 13 - The following instructions will apply to ICA 13-10...Ch. 13 - The following instructions will apply to ICA 13-10...Ch. 13 - The following instructions will apply to ICA 13-10...Ch. 13 - Prob. 21ICACh. 13 - As a reminder, the Reynolds number is discussed in...Ch. 13 - As a reminder, the Reynolds number is discussed in...Ch. 13 - An environmental engineer has obtained a bacteria...Ch. 13 - An environmental engineer has obtained a bacteria...Ch. 13 - An environmental engineer has obtained a bacteria...Ch. 13 - A growing field of inquiry that poses both great...Ch. 13 - If an object is heated, the temperature of the...Ch. 13 - The Volcanic Explosivity Index (VEI) is based...Ch. 13 - You are an engineer for a plastics manufacturing...Ch. 13 - A Pitot tube is a device used to measure the...Ch. 13 - As part of an electronic music synthesizer you...Ch. 13 - The following data were collected during testing...Ch. 13 - The relationship of the power required by a...Ch. 13 - When a fluid flows around an object, it creates a...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
What types of coolant are used in vehicles?
Automotive Technology: Principles, Diagnosis, And Service (6th Edition) (halderman Automotive Series)
How are relationships between tables expressed in a relational database?
Modern Database Management
Why is the study of database technology important?
Database Concepts (8th Edition)
17–1C A high-speed aircraft is cruising in still air. How does the temperature of air at the nose of the aircra...
Thermodynamics: An Engineering Approach
What is an uninitialized variable?
Starting Out with Programming Logic and Design (5th Edition) (What's New in Computer Science)
How is the hydrodynamic entry length defined for flow in a pipe? Is the entry length longer in laminar or turbu...
Fluid Mechanics: Fundamentals and Applications
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Qu 3 Nickel (Ni) single crystal turbine blades burn less fuel at higher temperatures because blades are grown on [110] closed packed direction. Nickel (Ni) at 20°C is FCC, and has an atomic radius, R, of 0.125 nm. Draw a reduced-sphere unit cell for this crystal and draw and label the vector [I 10], starting from the origin (0, 0, 0). a) Calculate the length of the vector [| 10] in nanometers. Express your answer in nanometers to one significant figure. b) Calculate the linear density of Nickel in the [| 1 0] direction in [atom/nm]. Express your answer in atoms/nm to one significant figure. show all work problemsarrow_forwardhandwritten-solutions, please!arrow_forwardhandwritten-solutions, please!arrow_forward
- Required information An eccentric force P is applied as shown to a steel bar of 25 × 90-mm cross section. The strains at A and B have been measured and found to be εΑ = +490 μ εB=-70 μ Know that E = 200 GPa. 25 mm 30 mm 90 mm 45 mm B Determine the distance d. The distance dis 15 mm mm.arrow_forwardhandwritten-solutions, please!arrow_forwardhandwritten-solutions, please!arrow_forward
- ! Required information Assume that the couple shown acts in a vertical plane. Take M = 25 kip.in. r = 0.75 in. A B 4.8 in. M 1.2 in. [1.2 in. Determine the stress at point B. The stress at point B is ksi.arrow_forwardhandwritten-solutions, please!arrow_forwardhandwritten-solutions, please!arrow_forward
- No use chatgptarrow_forwardProblem 6 (Optional, extra 6 points) 150 mm 150 mm 120 mm 80 mm 60 mm PROBLEM 18.103 A 2.5 kg homogeneous disk of radius 80 mm rotates with an angular velocity ₁ with respect to arm ABC, which is welded to a shaft DCE rotating as shown at the constant rate w212 rad/s. Friction in the bearing at A causes ₁ to decrease at the rate of 15 rad/s². Determine the dynamic reactions at D and E at a time when ₁ has decreased to 50 rad/s. Answer: 5=-22.01 +26.8} N E=-21.2-5.20Ĵ Narrow_forwardProblem 1. Two uniform rods AB and CE, each of weight 3 lb and length 2 ft, are welded to each other at their midpoints. Knowing that this assembly has an angular velocity of constant magnitude c = 12 rad/s, determine: (1). the magnitude and direction of the angular momentum HD of the assembly about D. (2). the dynamic reactions (ignore mg) at the bearings at A and B. 9 in. 3 in. 03 9 in. 3 in. Answers: HD = 0.162 i +0.184 j slug-ft²/s HG = 2.21 k Ay =-1.1 lb; Az = 0; By = 1.1 lb; B₂ = 0.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Principles of Heat Transfer (Activate Learning wi...Mechanical EngineeringISBN:9781305387102Author:Kreith, Frank; Manglik, Raj M.Publisher:Cengage Learning
Principles of Heat Transfer (Activate Learning wi...
Mechanical Engineering
ISBN:9781305387102
Author:Kreith, Frank; Manglik, Raj M.
Publisher:Cengage Learning
Heat Transfer – Conduction, Convection and Radiation; Author: NG Science;https://www.youtube.com/watch?v=Me60Ti0E_rY;License: Standard youtube license