Chapter 11.10, Problem 113RP

Consider a two-stage compression refrigeration system operating between the pressure limits of 1.4 and 0.12 MPa. The working fluid is refrigerant-134a. The refrigerant leaves the condenser as a saturated liquid and is throttled to a flash chamber operating at 0.5 MPa. Part of the refrigerant evaporates during this flashing process, and this vapor is mixed with the refrigerant leaving the low-pressure compressor. The mixture is then compressed to the condenser pressure by the high-pressure compressor. The liquid in the flash chamber is throttled to the evaporator pressure, and it cools the refrigerated space as it vaporizes in the evaporator. Assuming the refrigerant leaves the evaporator as saturated vapor and both compressors are isentropic, determine (a) the fraction of the refrigerant that evaporates as it is throttled to the flash chamber, (b) the amount of heat removed from the refrigerated space and the compressor work per unit mass of refrigerant flowing through the condenser, and (c) the coefficient of performance.

To determine

The fraction of the refrigerant that evaporates as it is throttled to the flash chamber.

Answer to Problem 113RP

The fraction of the refrigerant that evaporates as it is throttled to the flash chamber is $\text{0}\text{.290}$.

Explanation of Solution

Show the T-s diagram as in Figure (1).

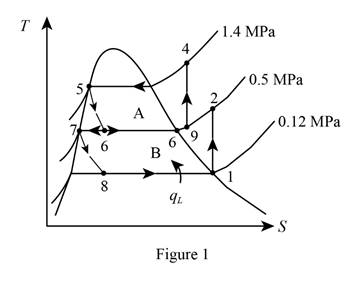
From Figure (1), write the specific enthalpy at state 5 is equal to state 6 due to throttling process.

$h_5\cong h_6$ (I)

Here, specific enthalpy at state 5 and 6 is $h_5\text{and}{h}_6$ respectively.

From Figure (1), write the specific enthalpy at state 7 is equal to state 8 due to throttling process.

$h_7\cong h_8$ (II)

Here, specific enthalpy at state 7 and 8 is $h_7\text{and}{h}_8$ respectively.

Express the fraction of the refrigerant that evaporates as it is throttled to the flash chamber

$x_6=\frac{h_6-h_8}{h_{fg@500\text{kPa}}}$ (III)

Here, specific enthalpy at saturated vapor is $h_g$ and specific enthalpy at evaporation and pressure of $\text{500}\text{kPa}\left(\text{0}\text{.5}\text{MPa}\right)$ is $h_{fg@500\text{kPa}}$.

Conclusion:

Perform unit conversion of pressure at state 1 from $\text{kPa}\text{to}\text{MPa}$.

$\begin{array}{c}{P}_1=0.12\text{MPa}\left[\frac{\text{1000}\text{kPa}}{\text{MPa}}\right]\\ =120\text{kPa}\end{array}$

Refer Table A-12, “saturated refrigerant-134a-pressure table”, and write the properties corresponding to pressure at state 1 $\left(P_1\right)$ of $\text{120}\text{kPa}$.

$\begin{array}{c}{h}_1=h_g\\ =236.99\text{kJ}/\text{kg}\\ s_1=s_g\\ =0.94789\text{kJ}/\text{kg}\cdot \text{K}\end{array}$

Here, specific entropy and enthalpy at state 1 is $s_1\text{and}{h}_1$ respectively,  specific enthalpy and entropy at saturated vapor is $h_g\text{and}{s}_g$ respectively.

Refer Table A-13, “superheated refrigerant 134a”, and write the specific enthalpy at state 2 corresponding to pressure at state 2 of $0.5\text{MPa}$ and specific entropy at state 2 $\left(s_2=s_1\right)$ of $0.94789\text{kJ}/\text{kg}\cdot \text{K}$ using interpolation method.

Write the formula of interpolation method of two variables.

$y_2=\frac{\left(x_2-x_1\right)\left(y_3-y_1\right)}{\left(x_3-x_1\right)}+y_1$ (IV)

Here, the variables denote by x and y is specific entropy at state 2 and specific enthalpy at state 2 respectively.

Show the specific enthalpy at state 2 corresponding to specific entropy as in Table (1).

Specific entropy at state 2 $s_2\left(\text{kJ}/\text{kg}\cdot \text{K}\right)$	Specific enthalpy at state 2 $h_2\left(\text{kJ}/\text{kg}\right)$
0.9384 $\left(x_1\right)$	263.48 $\left(y_1\right)$
0.94789 $\left(x_2\right)$	$\left(y_2=?\right)$
0.9704 $\left(x_3\right)$	273.03 $\left(y_3\right)$

Substitute $\text{0}\text{.9384}\text{kJ}/\text{kg}\cdot \text{K}\text{,}0.94789\text{kJ}/\text{kg}\cdot \text{K}\text{and}\text{0}\text{.9704}\text{kJ}/\text{kg}\cdot \text{K}$ for $x_1,x_2\text{and}{x}_3$ respectively, $263.48\text{kJ}/\text{kg}$ for $y_1$ and $273.03\text{kJ}/\text{kg}$ for $y_3$ in Equation (IV).

$\begin{array}{c}{y}_2=\frac{\left[\left(\text{0}\text{.94789}-\text{0}\text{.9384}\right)\text{kJ}/\text{kg}\cdot \text{K}\right]\left[\left(273.03-263.48\right)\text{kJ}/\text{kg}\right]}{\left(\text{0}\text{.9704}-\text{0}\text{.9384}\right)\text{kJ}/\text{kg}\cdot \text{K}}+263.48\text{kJ}/\text{kg}\\ =266.29\text{kJ}/\text{kg}\\ =h_2\end{array}$

Thus, the specific enthalpy at state 2 is,

$h_2=266.29\text{kJ}/\text{kg}$

Perform unit conversion of pressure at state 3 from $\text{kPa}\text{to}\text{MPa}$.

$\begin{array}{c}{P}_3=0.5\text{MPa}\left[\frac{\text{1000}\text{kPa}}{\text{MPa}}\right]\\ =500\text{kPa}\end{array}$

Refer Table A-12, “saturated refrigerant-134a-pressure table”, and write the property corresponding to pressure at state 3 $\left(P_3\right)$ of $\text{500}\text{kPa}$.

$\begin{array}{c}{h}_3=h_g\\ =259.36\text{kJ}/\text{kg}\end{array}$

Perform unit conversion of pressure at state 5 from $\text{kPa}\text{to}\text{MPa}$.

$\begin{array}{c}{P}_5=1.4\text{MPa}\left[\frac{\text{1000}\text{kPa}}{\text{MPa}}\right]\\ =1400\text{kPa}\end{array}$

Refer Table A-12, “saturated refrigerant-134a-pressure table”, and write the property corresponding to pressure at state 5 $\left(P_5\right)$ of $\text{1400}\text{kPa}$.

$\begin{array}{c}{h}_5=h_f\\ =127.25\text{kJ}/\text{kg}\end{array}$

Here, specific enthalpy at saturated liquid is $h_f$.

Substitute $127.25\text{kJ}/\text{kg}$ for $h_5$ in Equation (I).

$h_6=127.25\text{kJ}/\text{kg}$

Refer Table A-12, “saturated refrigerant-134a-pressure table”, and write the property corresponding to pressure at state 8 $\left(P_8\right)$ of $\text{500}\text{kPa}$.

$\begin{array}{c}{h}_8=h_f\\ =73.32\text{kJ}/\text{kg}\end{array}$

Substitute $73.32\text{kJ}/\text{kg}$ for $h_8$ in Equation (II).

$h_7=63.92\text{kJ}/\text{kg}$

Refer Table A-12, “saturated refrigerant-134a-pressure table”, and write the specific enthalpy at evaporation and pressure of $\text{500}\text{kPa}$.

$h_{fg@500\text{kPa}}=186.04\text{kJ}/\text{kg}$

Substitute $127.25\text{kJ}/\text{kg}$ for $h_6$, $73.32\text{kJ}/\text{kg}$ for $h_8$ and $186.04\text{kJ}/\text{kg}$ for $h_{fg@500\text{kPa}}$ in Equation (III).

$\begin{array}{c}{x}_6=\frac{127.25\text{kJ}/\text{kg}-73.32\text{kJ}/\text{kg}}{186.04\text{kJ}/\text{kg}}\\ =0.2899\\ \simeq 0.290\end{array}$

Hence, the fraction of the refrigerant that evaporates as it is throttled to the flash chamber is $\text{0}\text{.290}$.

To determine

The amount of heat removed from the refrigerated space and the compressor work per unit mass of refrigerant flowing through the condenser.

Answer to Problem 113RP

The amount of heat removed from the refrigerated space is $\text{116}\text{kJ}/\text{kg}$ and the compressor work per unit mass of refrigerant flowing through the condenser is $\text{42}\text{.7}\text{kJ}/\text{kg}$.

Explanation of Solution

Express the enthalpy at state 9 by using an energy balance on the mixing chamber.

$\begin{array}{l}{\dot{E}}_{\text{in}}-{\dot{E}}_{\text{out}}=\Delta {\dot{E}}_{\text{system}}\\ {\dot{E}}_{\text{in}}-{\dot{E}}_{\text{out}}=0\\ {\displaystyle \sum {\dot{m}}_e{h}_e=}{\displaystyle \sum {\dot{m}}_i{h}_i}\end{array}$

$\begin{array}{l}{\dot{E}}_{\text{in}}={\dot{E}}_{\text{out}}\\ \left(1\right)h_9=x_6{h}_3+\left(1-x_6\right)h_2\end{array}$ (V)

Here, the rate of total energy entering the system is ${\dot{E}}_{\text{in}}$, the rate of total energy leaving the system is ${\dot{E}}_{\text{out}}$, the rate of change in the total energy of the system is $\Delta {\dot{E}}_{\text{system}}$, mass flow rate at exit and inlet is ${\dot{m}}_e\text{and}{\dot{m}}_i$ respectively, and specific enthalpy at exit and inlet is $h_e\text{and}{h}_i$ respectively.

Express the amount of heat removed from the refrigerated space.

$q_L=\left(1-x_6\right)\left(h_1-h_8\right)$ (VI)

Express the compressor work per unit mass of refrigerant flowing through the condenser.

$w_{\text{in}}=\left(1-x_6\right)\left(h_2-h_1\right)+\left(h_4-h_9\right)$ (VII)

Conclusion:

Substitute $0.2899$ for $x_6$, $\text{259}\text{.36}\text{kJ}/\text{kg}$ for $h_3$, and $\text{266}\text{.29}\text{kJ}/\text{kg}$ for $h_2$ in Equation (V).

$\begin{array}{l}\left(1\right)h_9=\left(0.2899\right)\left(\text{259}\text{.36}\text{kJ}/\text{kg}\right)+\left(1-0.2899\right)\left(\text{266}\text{.29}\text{kJ}/\text{kg}\right)\\ h_9=\text{264}\text{.28}\text{kJ}/\text{kg}\end{array}$

Refer Table A-13, “superheated refrigerant 134a”, and write the specific entropy at state 9 corresponding to pressure at state 9 of $0.5\text{MPa}$ and specific enthalpy at state 9 of $\text{264}\text{.28}\text{kJ}/\text{kg}$ using interpolation method.

Show the specific enthropy at state 9 corresponding to specific enthalpy as in Table (2).

Specific enthalpy at state 9 $h_9\left(\text{kJ}/\text{kg}\right)$	Specific entropy at state 9 $s_9\left(\text{kJ}/\text{kg}\cdot \text{K}\right)$
263.48 $\left(x_1\right)$	0.9384 $\left(y_1\right)$
264.28 $\left(x_2\right)$	$\left(y_2=?\right)$
273.03 $\left(x_3\right)$	0.9704 $\left(y_3\right)$

Use excels and tabulates the values of Table (2) in Equation (IV) to get,

$\begin{array}{c}{y}_2=0.94108\text{kJ}/\text{kg}\cdot \text{K}\\ =s_9\end{array}$

Thus, the specific entropy at state 9 is,

$s_9=0.94108\text{kJ}/\text{kg}\cdot \text{K}$

Refer Table A-13, “superheated refrigerant 134a”, and write the specific enthalpy at state 4 corresponding to pressure at state 4 of $1.4\text{MPa}$ and specific entropy at state 2 $\left(s_9=s_4\right)$ of $0.94108\text{kJ}/\text{kg}\cdot \text{K}$ using interpolation method.

$h_4=286.19\text{kJ}/\text{kg}$

Substitute $0.2899$ for $x_6$, $\text{236}\text{.99}\text{kJ}/\text{kg}\text{and}\text{73}\text{.32}\text{kJ}/\text{kg}$ for $h_1\text{and}{h}_8$ respectively in Equation (VI).

$\begin{array}{c}{q}_L=\left(1-0.2899\right)\left(\text{236}\text{.99}\text{kJ}/\text{kg}-\text{73}\text{.32}\text{kJ}/\text{kg}\right)\\ =116.2\text{kJ}/\text{kg}\\ \simeq 116\text{kJ}/\text{kg}\end{array}$

Hence, the amount of heat removed from the refrigerated space is $\text{116}\text{kJ}/\text{kg}$.

Substitute $0.2899$ for $x_6$, $\text{266}\text{.29}\text{kJ}/\text{kg}\text{and}\text{236}\text{.99}\text{kJ}/\text{kg}$ for $h_2\text{and}{h}_1$ respectively and $\text{286}\text{.19}\text{kJ}/\text{kg}\text{and}\text{264}\text{.28}\text{kJ}/\text{kg}$ for $h_4\text{and}{h}_9$ respectively in Equation (VII).

$\begin{array}{c}{w}_{\text{in}}=\left(1-0.2899\right)\left(\text{266}\text{.29}\text{kJ}/\text{kg}-\text{236}\text{.99}\text{kJ}/\text{kg}\right)+\left(\text{286}\text{.19}\text{kJ}/\text{kg}-\text{264}\text{.28}\text{kJ}/\text{kg}\right)\\ =\text{42}\text{.7}\text{kJ}/\text{kg}\end{array}$

Hence, the compressor work per unit mass of refrigerant flowing through the condenser is $\text{42}\text{.7}\text{kJ}/\text{kg}$.

To determine

The coefficient of performance of the system.

Answer to Problem 113RP

The coefficient of performance of the system is $\text{2}\text{.72}$.

Explanation of Solution

Express the coefficient of performance of the system.

$\text{COP}=\frac{q_L}{w_{\text{in}}}$ (VIII)

Conclusion:

Substitute $116.2\text{kJ}/\text{kg}$ for $q_L$ and $\text{42}\text{.7}\text{kJ}/\text{kg}$ for $w_{\text{in}}$ in Equation (VIII).

$\begin{array}{c}\text{COP}=\frac{116.2\text{kJ}/\text{kg}}{\text{42}\text{.7}\text{kJ}/\text{kg}}\\ =2.72\end{array}$

Hence, the coefficient of performance of the system is $\text{2}\text{.72}$.