Chapter 1.1, Problem 59E

To determine

To graph: the given set.

Explanation of Solution

Given information:

An interval is given as

  $\left(-2,0\right)\cup \left(-1,1\right)$

Concept used:

An interval $\left(a,b\right)$ in terms of an inequality can be expressed as $a<x<b$ .

An interval $\left[a,b\right]$ in terms of an inequality can be expressed as $a\le x\le b$ .

An interval $\left[a,b\right)$ in terms of an inequality can be expressed as $a\le x<b$ .

An interval $\left(a,b\right]$ in terms of an inequality can be expressed as $a<x\le b$ .

An interval $\left(-\infty ,b\right]$ in terms of an inequality can be expressed as $x\le b$ .

An interval $\left(-\infty ,b\right)$ in terms of an inequality can be expressed as $x<b$ .

An interval $\left[a,\infty \right)$ in terms of an inequality can be expressed as $x\ge a$ .

An interval $\left(a,\infty \right)$ in terms of an inequality can be expressed as $x>a$ .

For any two or more sets, union of the sets gives the elements which are in the either one of the sets and the all elements of all sets. Common elements are considered once. Union is represented by the symbol $\cup$ .

Calculation:

Consider the given interval.

  $\left(-2,0\right)\cup \left(-1,1\right)$

Let us consider a variable x o express the inequality.

So, the interval can be expressed as an inequality as $-2<x<0\cup -1<x<1$ .

Combining the interval will be $-2<x<1=\left(-2,1\right)$

Graph:

Now, graph the interval on number line as shown:

  

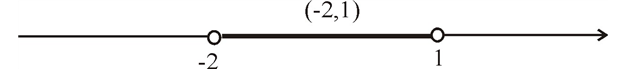
Here, $-2\text{and}1$ both the points are excluded from the interval so, open balls are placed for $-2\text{and}1$ on the number line.