
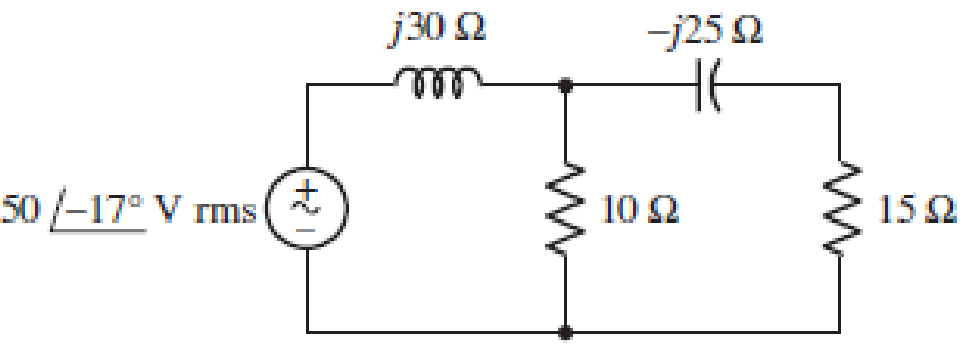
■ FIGURE 11.49
Instead of including a capacitor as indicated in Fig. 11.49, the circuit is erroneously constructed using two identical inductors, each having an impedance of j30 W at the operating frequency of 50 Hz. (a) Compute the complex power delivered to each passive component. (b) Verify your solution by calculating the complex power supplied by the source. (c) At what power factor is the source operating?
(a)
Find the complex power delivered to each passive element when
Answer to Problem 53E
The complex power delivered to
Explanation of Solution
Given data:
Refer to Figure 11.49 in the textbook for the given circuit.
Operating frequency is 50 Hz.
Formula used:
Write the expression for complex power delivered to the element as follows:
Here,
Write the expression for current in terms of voltage and impedance as follows:
Calculation:
Consider
Use the expression in Equation (2) and find the source current as follows:
Substitute
Use voltage division rule and find the voltage across
Substitute
Modify the expression in Equation (1) for the complex power delivered to
Substitute
Consider the node voltage across the shunt branches as
Substitute
Use current division rule and find the current through
Substitute
Modify the expression in Equation (1) for the complex power delivered to the
Substitute
Use voltage division rule and find the voltage across
Substitute
Use current division rule and find the current through
Substitute
Modify the expression in Equation (1) for the complex power delivered to the
Substitute
Use voltage division rule and find the voltage across
Substitute
Modify the expression in Equation (1) for the complex power delivered to the
Substitute
Conclusion:
Thus, the complex power delivered to
(b)
Verify the solution obtained in Part (a) by calculating the complex power supplied by the source.
Explanation of Solution
Calculation:
Modify the expression in Equation (1) for the complex power supplied by the source as follows:
Substitute
Write the expression for sum of complex delivered to (or absorbed by) each passive element as follows:
From Part (a), substitute
From the calculation, it is clear that, the complex power supplied by the source is equal to the complex power delivered to each passive element.
Conclusion:
Thus, the solution obtained in Part (a) is verified.
(c)
Find the power factor of the source.
Answer to Problem 53E
The power factor of the source is 0.253 lagging.
Explanation of Solution
Formula used:
Write the expression for complex power in the rectangular form as follows:
Here,
Write the expression for power factor as follows:
Calculation:
Rewrite the expression for complex power supplied by the source in rectangular form as follows:
Compare the complex power supplied by the source with the expression in Equation (3) and write the average and reactive power supplied by the source as follows:
Substitute 19.1482 W for
If the imaginary part of the complex power (reactive power) is positive value, then the load has lagging power factor. If the imaginary part is negative value, then the load has leading power factor.
As the imaginary part of the given complex power is positive value, the power factor is lagging power factor.
Conclusion:
Thus, the power factor of the source is 0.253 lagging.
Want to see more full solutions like this?
Chapter 11 Solutions
ENGINEERING CIRCUIT...(LL)>CUSTOM PKG.<
- 2.a. Simplify and determine Zk+ for: 2.x. 60 [Hz] ⚫ 2.y. 180 [Hz] a.x. 60[Hz] a.y. 180 [Hz] Joo (127 2[H] w 240 [√]arrow_forwardP3. Given the following network, determine: ⚫ 3.a. Equivalent Y ⚫ 3.b. Equivalent A 2 R[2] 10 8 b 20 30 5arrow_forward[Electrical Circuits] P1. Using the mesh current method, calculate the magnitude and direction of: 1.a. I and I (mesh currents) 1.b. I10 (test current in R10 = 1082) 1.c. (Calculate the magnitude and signs of V10) 6[A] 12 [√] بي 10 38 20 4A] Iw -800arrow_forward
- Need handwritten solution do not use chatgptarrow_forward[07/01, 16:59] C P: Question: Calculate the following for 100Hz and 500Hz (express all answers in phasor form). Show all work. A) Xc and ZTB) VR1 and VC1 C) IT Handwritten Solution Pleasearrow_forward1. Sketch the root loci of a system with the following characteristic equation: s²+2s+2+K(s+2)=0 2. Sketch the root loci for the following loop transfer function: KG(s)H(s)=- K(s+1) s(s+2)(s²+2s+4)arrow_forward
- 3. For the unity feedback system with forward path transfer function, G(s), below: G(s)= K(s² +8) (s+4)(s+5) Sketch the root locus and show the breakaway/break-in point(s) and jo-axis crossing. Determine the angle of arrival and K value at the breakaway/break- in point(s). Give your comment the system is stable or unstable.arrow_forwardFind the step response of each of the transfer functions shown in Eqs. (4.62) through (4.64) and compare them. [Shown in the image]Book: Norman S. Nise - Control Systems Engineering, 6th EditionTopic: Chapter-4: Time Response, Example 4.8Solve the math with proper explanation. Please don't give AI response. Asking for a expert verified answer.arrow_forward2. With respect to the circuit shown in Figure 2 below V2 -R1 R2 R4 w R3 R5 Figure 2: DC Circuit 2 a. Using Ohm's and Kirchhoff's laws calculate the current flowing through R3 and so determine wattage rating of R3. b. Verify your results with simulations. Note: you must use the values for the components in Table 2. Table 2 V2 (Volts) R1 (KQ) R2 (KQ) R3 (KQ) R4 (KQ) R5 (KQ) 9 3.3 5 10 6 1 3.3arrow_forward
