College Physics: A Strategic Approach (3rd Edition)

3rd Edition

ISBN: 9780321879721

Author: Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field

Publisher: PEARSON

See similar textbooks

Concept explainers

Kinematics

A machine is a device that accepts energy in some available form and utilizes it to do a type of work. Energy, work, or power has to be transferred from one mechanical part to another to run a machine. While the transfer of energy between two machine par…

Kinetic Energy and Work-Energy Theorem

In physics, work is the product of the net force in direction of the displacement and the magnitude of this displacement or it can also be defined as the energy transfer of an object when it is moved for a distance due to the forces acting on it in the d…

Videos

Textbook Question

Chapter 10, Problem 20CQ

A solid cylinder and a hollow cylinder have the same mass, same radius, and turn on frictionless, horizontal axles. (The hollow cylinder has lightweight spokes connecting it to the axle.) A rope is wrapped around each cylinder and tied to a block. The blocks have the same mass and are held the same height above the ground as shown in Figure Q10.20. Both blocks are released simultaneously. The ropes do not slip. Which block hits the ground first? Or is it a tie? Explain.

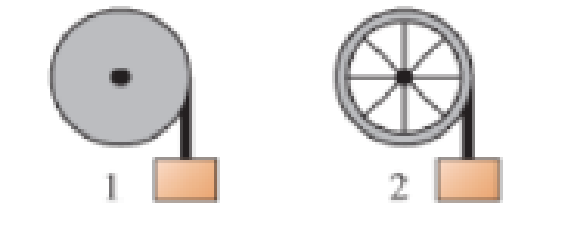
Chapter 10, Problem 20CQ, A solid cylinder and a hollow cylinder have the same mass, same radius, and turn on frictionless,

Figure Q10.20

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 10, Problem 19CQ

Chapter 10, Problem 21CQ

Students have asked these similar questions

No chatgpt pls will upvote

suggest a reason ultrasound cleaning is better than cleaning by hand?

Checkpoint 4 The figure shows four orientations of an electric di- pole in an external electric field. Rank the orienta- tions according to (a) the magnitude of the torque on the dipole and (b) the potential energy of the di- pole, greatest first. (1) (2) E (4)

Chapter 10 Solutions

College Physics: A Strategic Approach (3rd Edition)

Ch. 10 - A ball of putty is dropped from a height of 2 m...Ch. 10 - A 0.5 kg mass on a 1-m-long string swings in a...Ch. 10 - Particle A has less mass than particle B. Both are...Ch. 10 - Puck B has twice the mass of puck A. Starting from...Ch. 10 - To change a tire, you need to use a jack to raise...Ch. 10 - Prob. 16CQ Ch. 10 - A roller coaster car rolls down a frictionless...Ch. 10 - A spring gun shoots out a plastic ball at speed v....Ch. 10 - Sandy and Chris stand on the edge of a cliff and...Ch. 10 - A solid cylinder and a hollow cylinder have the...Ch. 10 - You are much more likely to be injured if you fall...Ch. 10 - A roller coaster starts from rest at its highest...Ch. 10 - You and a friend each carry a 15 kg suitcase up...Ch. 10 - A woman uses a pulley and a rope to raise a 20 kg...Ch. 10 - A hockey puck sliding along frictionless ice with...Ch. 10 - A block slides down a smooth ramp, starting from...Ch. 10 - A wrecking ball is suspended from a 5.0-m-long...Ch. 10 - Prob. 1P Ch. 10 - The two ropes seen in Figure P10.2 are used to...Ch. 10 - The two ropes shown in the bird's-eye view of...Ch. 10 - Prob. 4P Ch. 10 - A boy flies a kite with the string at a 30 angle...Ch. 10 - A crate slides down a ramp that makes a 20 angle...Ch. 10 - Which has the larger kinetic energy, a 10 g bullet...Ch. 10 - At what speed does a 1000 kg compact car have the...Ch. 10 - Prob. 9P Ch. 10 - The cheetah is the fastest land animal, reaching...Ch. 10 - How fast would an 80 kg man need to run in order...Ch. 10 - Sams job at the amusement park is to slow down and...Ch. 10 - A 20 g plastic ball is moving to the left at 30...Ch. 10 - Prob. 14P Ch. 10 - An energy storage system based on a flywheel (a...Ch. 10 - The lowest point in death Valley is 85.0 m below...Ch. 10 - Prob. 17P Ch. 10 - The world's fastest humans can reach speeds of...Ch. 10 - A 72 kg bike racer climbs a 1200-m-long section of...Ch. 10 - A 1000 kg wrecking ball hangs from a 15-m-long...Ch. 10 - How far must you stretch a spring with k = 1000...Ch. 10 - How much energy can be stored in a spring with a...Ch. 10 - The elastic energy stored in your tendons can...Ch. 10 - Marissa drags a 23 kg duffel bag 14 m across the...Ch. 10 - Mark pushes his broken car 150 m down the block to...Ch. 10 - A 900 N crate slides 12m down a ramp that makes an...Ch. 10 - A 25 kg child slides down a playground slide at a...Ch. 10 - A boy reaches out of a window and tosses a ball...Ch. 10 - Prob. 29P Ch. 10 - What minimum speed does a 100 g puck need to make...Ch. 10 - A car is parked at the top of a 50-m-high hill....Ch. 10 - A 1500 kg car is approaching the hill shown in...Ch. 10 - A 10 kg runaway grocery cart runs into a spring,...Ch. 10 - As a 15,000 kg jet lands on an aircraft carrier,...Ch. 10 - Your friend's Frisbee has become stuck 16m above...Ch. 10 - A fireman of mass 80 kg slides down a pole. When...Ch. 10 - Prob. 37P Ch. 10 - Prob. 38P Ch. 10 - In the winter activity of tubing, riders slide...Ch. 10 - A cyclist is coasting at 12 m/s when she starts...Ch. 10 - A 50 g marble moving at 2.0 m/s strikes a 20 g...Ch. 10 - Ball 1, with a mass of 100 g and traveling at 10...Ch. 10 - Prob. 43P Ch. 10 - Two balls undergo a perfectly elastic head-on...Ch. 10 - Prob. 45P Ch. 10 - Prob. 46P Ch. 10 - A 1000 kg sports car accelerates from 0 to 30m/sin...Ch. 10 - In just 0.30 s, you compress a spring (spring...Ch. 10 - An elite Tour de France cyclist can maintain an...Ch. 10 - A 710 kg car drives at a constant speed of 23 m/s....Ch. 10 - An elevator weighing 2500 N ascends at a constant...Ch. 10 - How much work does Scott do to push a 80 kg sofa...Ch. 10 - A 550 kg elevator accelerates upward at 1.2 m/s2...Ch. 10 - Prob. 54GP Ch. 10 - Prob. 55GP Ch. 10 - Prob. 56GP Ch. 10 - You are driving your 1500 kg car at 20 m/s down a...Ch. 10 - A 20 kg child is on a swing that hangs from...Ch. 10 - Prob. 59GP Ch. 10 - A cannon tilted up at a 30 angle fires a cannon...Ch. 10 - The sledder shown in Figure P10.61 starts from the...Ch. 10 - A 50 g ice cube can slide without friction up and...Ch. 10 - The maximum energy a bone can absorb without...Ch. 10 - In an amusement park water slide, people slide...Ch. 10 - Boxes A and B in Figure P10.69 have masses of 12.0...Ch. 10 - What would be the speed of the boxes in Problem 69...Ch. 10 - A 20 g ball is fired horizontally with initial...Ch. 10 - Two coupled boxcars are rolling along at 2.5 m/s...Ch. 10 - A fish scale, consisting of a spring with spring...Ch. 10 - A 70 kg human sprinter can accelerate from rest to...Ch. 10 - A 50 g ball of clay traveling at 6.5 m/s hits and...Ch. 10 - Prob. 78GP Ch. 10 - The mass of an elevator and its occupants is 1200...Ch. 10 - Prob. 80GP Ch. 10 - Tennis Ball Testing A tennis ball bouncing on a...Ch. 10 - Tennis Ball Testing A tennis ball bouncing on a...Ch. 10 - Tennis Ball Testing A tennis ball bouncing on a...Ch. 10 - Prob. 84MSPP Ch. 10 - Tennis Ball Testing A tennis ball bouncing on a...Ch. 10 - Work and Power in Cycling When you ride a bicycle...Ch. 10 - Work and Power in Cycling When you ride a bicycle...Ch. 10 - Work and Power in Cycling When you ride a bicycle...Ch. 10 - Work and Power in Cycling When you ride a bicycle...Ch. 10 - Work and Power in Cycling When you ride a bicycle...

Additional Science Textbook Solutions

Find more solutions based on key concepts

10.71 Identify each of the following as an acid or a base: (10.1) H2SO4 RbOH Ca(OH)2 HI ...

Chemistry: An Introduction to General, Organic, and Biological Chemistry (13th Edition)

Fibrous connective tissue consists of ground substance and fibers that provide strength, support, and flexibili...

Human Biology: Concepts and Current Issues (8th Edition)

If isomer A is heated to about 100 C, a mixture of isomers A and B is formed. Explain why there is no trace of ...

Organic Chemistry (8th Edition)

1. In uniform circular motion, which of the following are constant: speed, velocity, angular velocity, centripe...

Physics for Scientists and Engineers: A Strategic Approach, Vol. 1 (Chs 1-21) (4th Edition)

Plants use the process of photosynthesis to convert the energy in sunlight to chemical energy in the form of su...

Campbell Essential Biology with Physiology (5th Edition)

1.14 Classify each of the following as a pure substance or a mixture. If a mixture, indicate whether it is homo...

Chemistry: The Central Science (14th Edition)

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.