
EBK FUNDAMENTALS OF APPLIED ELECTROMAGN
7th Edition
ISBN: 8220100663659
Author: ULABY
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Textbook Question
Chapter 1, Problem 7P
A wave traveling along a string in the +x direction is given by
y1(x, t) = A cos(ωt − βx),
where x = 0 is the end of the string, which is tied rigidly to a wall, as shown in Fig. P1.7.
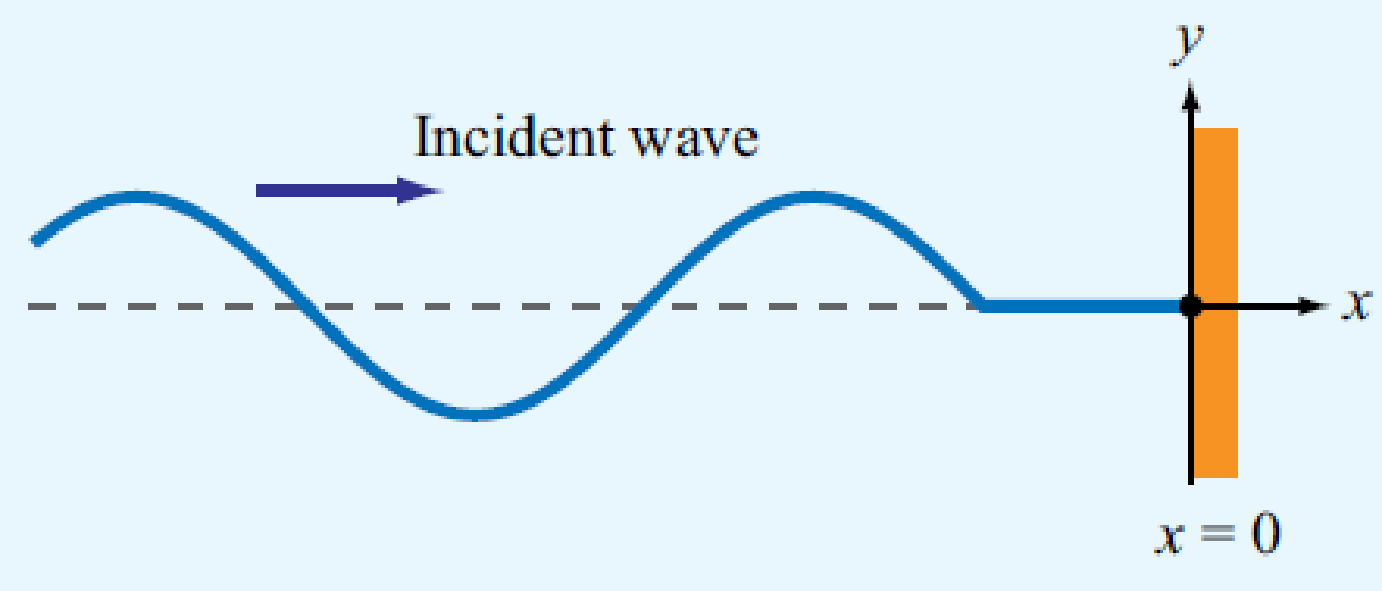
Figure P1.7 Wave on a string tied to a wall at x = 0 (Problem 1.7).
When wave y1(x, t) arrives at the wall, a reflected wave y2(x, t) is generated. Hence, at any location on the string, the vertical displacement ys is the sum of the incident and reflected waves:
ys(x, t) = y1(x, t) + y2(x, t).
- (a) Write an expression for y2(x, t), keeping in mind its direction of travel and the fact that the end of the string cannot move.
- (b) Generate plots of y1(x, t), y2(x, t) and ys(x, t) versus x over the range −2λ ≤ x ≤ 0 at ωt = π/4 and at ωt = π/2.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Question 2
A transistor is used as a switch and the waveforms are shown in Figure 2. The parameters are
Vcc = 225 V, VBE(sat) = 3 V, IB = 8 A, VCE(sat) = 2 V, Ics = 90 A, td = 0.5 µs, tr = 1 µs, ts = 3 µs, tƒ
= 2 μs, and f
10 kHz. The duty cycle is k 50%. The collector- emitter leakage current is
ICEO = 2 mA. Determine the power loss due to the collector current:
=
=
=
(a) during turn-on ton = td + tr
VCE
Vcc
(b) during conduction period tn
V CE(sat)
0
toff"
ton
Ics
0.9 Ics
(c) during turn-off toff = ts + tf
(d) during off-time tot
(e) the total average power losses PT
ICEO
0
IBS
0
Figure 2
V BE(sat)
0
主
*
td
tr
In
Is
If
to
iB
VBE
T= 1/fs
Question 1:
The beta (B) of the bipolar transistor shown in Figure 1 varies from 12 to 60. The load resistance
is Rc = 5. The dc supply voltage is VCC = 40 V and the input voltage to the base circuit is
VB = 5 V. If VCE(sat) = 1.2 V, VBE(sat) = 1.6 V, and RB = 0.8 2, calculate:
(a) the overdrive factor ODF.
(b) the forced ẞ
(c) the power loss in the transistor PT.
IB
VB
RB
+
V BE
RC
Vcc'
Ic
+
IE
Figure 1
VCE
I need help in creating a matlab code to find the currents
Chapter 1 Solutions
EBK FUNDAMENTALS OF APPLIED ELECTROMAGN
Ch. 1.3 - Prob. 1CQCh. 1.3 - What is Coulombs law? State its properties.Ch. 1.3 - What are the two important properties of electric...Ch. 1.3 - What do the electrical permittivity and magnetic...Ch. 1.3 - What are the three branches and associated...Ch. 1.4 - How can you tell if a wave is traveling in the...Ch. 1.4 - Prob. 7CQCh. 1.4 - Why does a negative value of 0 signify a phase...Ch. 1.4 - Consider the red wave shown in Fig. E1.1. What is...Ch. 1.4 - The wave shown in red in Fig. E1.2 is given by...
Ch. 1.4 - The electric field of a traveling electromagnetic...Ch. 1.4 - Prob. 4ECh. 1.4 - The red wave shown in Fig. E1.5 is given by...Ch. 1.4 - An electromagnetic wave is propagating in the z...Ch. 1.5 - What are the three fundamental properties of EM...Ch. 1.5 - What is the range of frequencies covered by the...Ch. 1.5 - Prob. 11CQCh. 1.6 - Express the following complex functions in polar...Ch. 1.6 - Show that 2j=(1+j). (See EM.)Ch. 1.7 - Prob. 12CQCh. 1.7 - How is the phasor technique used when the forcing...Ch. 1.7 - A series RL circuit is connected to a voltage...Ch. 1.7 - A phasor voltage is given by V=j5V. Find (t).Ch. 1 - A 2 kHz sound wave traveling in the x direction in...Ch. 1 - For the pressure wave described in Example 1-1,...Ch. 1 - A harmonic wave traveling along a string is...Ch. 1 - A wave traveling along a string is given by...Ch. 1 - Two waves, y1(t) and y2(t), have identical...Ch. 1 - The height of an ocean wave is described by the...Ch. 1 - A wave traveling along a string in the +x...Ch. 1 - Two waves on a string are given by the following...Ch. 1 - Give expressions for y(x, t) for a sinusoidal wave...Ch. 1 - An oscillator that generates a sinusoidal wave on...Ch. 1 - Prob. 11PCh. 1 - Given two waves characterized by...Ch. 1 - The voltage of an electromagnetic wave traveling...Ch. 1 - A certain electromagnetic wave traveling in...Ch. 1 - Prob. 15PCh. 1 - Prob. 16PCh. 1 - Complex numbers z1 and z2 are given z1=3j2z2=4+j3...Ch. 1 - Complex numbers z1 and z2 are given by...Ch. 1 - If z=2+j4, determine the following quantities in...Ch. 1 - Find complex numbers t=z1+z2 and s=z1z2, both in...Ch. 1 - Complex numbers z1 and z2 are given by...Ch. 1 - If z=3j5, find the value of ln(z).Ch. 1 - If z = 3 j4. find the value of ez.Ch. 1 - Prob. 24PCh. 1 - A voltage source given by s(t)=25cos(2103t30)(V)...Ch. 1 - Find the phasors of the following time functions:...Ch. 1 - Find the instantaneous time sinusoidal functions...Ch. 1 - A series RLC circuit is connected to a generator...Ch. 1 - The voltage source of the circuit shown in Fig....
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, electrical-engineering and related others by exploring similar questions and additional content below.Similar questions
- I need help fixing this MATLAB code: as I try to get it working there were some problems:arrow_forwardI need help in construct a matlab code to find the voltage of VR1 to VR4, the currents, and the watts based on that circuit.arrow_forwardQ2: Using D flip-flops, design a synchronous counter. The counter counts in the sequence 1,3,5,7, 1,7,5,3,1,3,5,7,.... when its enable input x is equal to 1; otherwise, the counter count 0.arrow_forward
- From the collector characteristic curves and the dc load line given below, determine the following: (a) Maximum collector current for linear operation (b) Base current at the maximum collector current (c) VCE at maximum collector current. lc (mA) 600 ΜΑ 60- 500 με 50- 400 με 40- 300 μ Α 30- Q-point 200 ΜΑ 20- 10- 100 μ Α 0 VCE (V) 1 2 3 4 5 6 7 8 9 10 [6 Paarrow_forwardProcedure:- 1- Connect the cct. shown in fig.(2). a ADDS DS Fig.(2) 2-For resistive load, measure le output voltage by using oscilloscope ;then sketch this wave. 3- Measure the average values ::f VL and IL: 4- Repeat steps 2 & 3 but for RL load. Report:- 1- Calculate the D.C. output vcl age theoretically and compare it with the test value. 2- Calculate the harmonic cont :nts of the load voltage, and explain how filter components may be selected. 3- Compare between the three-phase half & full-wave uncontrolled bridge rectifier. 4- Draw the waveform for the c:t. shown in fig.(2) but after replaced Di and D3 by thyristors with a 30° and a2 = 90° 5- Draw the waveform for the cct. shown in fig.(2) but after replace the 6-diodes by 6- thyristor. 6- Discuss your results. Please solve No. 4 and 5arrow_forwardPlease I want solution by handwrittenarrow_forward
- 8 00 ! Required information Consider the circuit given below. 0/2 points awarded 3 ΚΩ www t=0 6kM Scored R 1.5i Vc 1 μF 10 V If R = 5.00 kQ, determine vao+). The value of va(0) is 1.4545 V.arrow_forwardI want to know what does it look in a breadboard circuit, because I want to created it but I not sure it is build properly, can you give me an illustuation base on this image, it do need to real, something like virutal examplearrow_forwardCharge neutrality Since doped semiconductor remains electroneutral, the concentration of negative charges equals the concentration of positive charges. n+ Na,ionized p+Nd,ionized np = n; 2 2 N-Na N N d d р + 2 2 n = Nd-Na 2 + Na - 2 Na +n₁ 2 71/2 1/2 2 2 +n Concentration of electrons and holes 1. Calculate concentrations of electrons and holes at room temperature in Si and Ge with donor concentration of 1.5x10¹7 cm³ and acceptor concentration of 8x1016 cm-3. 2. Will these concentrations change much with the temperature increase to 100°C?arrow_forward
- Answer the questions on the end of the image pleasearrow_forwardAnswer these two questions on the end of the image, please 1.Calculate intrinsic carrier concentration for Si, Ge and GaAs at temperatures -20°C, 20°C (room temperature) and 120°C 2.Compare the obtained data with n and p shown on previous slide 25arrow_forwardCan you help me achieve the requirements using Arduino? I have encountered some issues with these requirements. Q.2: Suppose you have two push buttons connected to ports (0 & 1) and four LED's connected to ports (6-9). Write a program to flash ON the odd LED's if we press the switch 0 for 4s, flash ON the even LED's if we press the switch 1 for 5s and flash ON all the LED's otherwise for 6s.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON
Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning
Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education
Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education
Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON
Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,
Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,

Introductory Circuit Analysis (13th Edition)
Electrical Engineering
ISBN:9780133923605
Author:Robert L. Boylestad
Publisher:PEARSON

Delmar's Standard Textbook Of Electricity
Electrical Engineering
ISBN:9781337900348
Author:Stephen L. Herman
Publisher:Cengage Learning

Programmable Logic Controllers
Electrical Engineering
ISBN:9780073373843
Author:Frank D. Petruzella
Publisher:McGraw-Hill Education

Fundamentals of Electric Circuits
Electrical Engineering
ISBN:9780078028229
Author:Charles K Alexander, Matthew Sadiku
Publisher:McGraw-Hill Education

Electric Circuits. (11th Edition)
Electrical Engineering
ISBN:9780134746968
Author:James W. Nilsson, Susan Riedel
Publisher:PEARSON

Engineering Electromagnetics
Electrical Engineering
ISBN:9780078028151
Author:Hayt, William H. (william Hart), Jr, BUCK, John A.
Publisher:Mcgraw-hill Education,
What Is a Plane Wave? — Lesson 2; Author: EMViso;https://www.youtube.com/watch?v=ES2WFevGM0g;License: Standard Youtube License