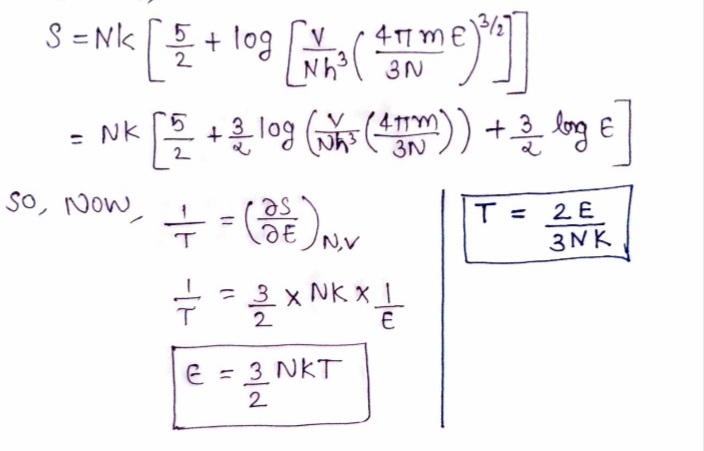
V From the Sackur-Tetrode formula S(E, V, N) = Nk ln 3/27 +-Nk, derive Απm E %3D 3H3 N s many properties of the monotonic ideal classical gas as you can.
Q: A room temperature gas of non interacting HF molecules are confined to a bottle with dimensions of…
A:
Q: The surface temperature of the Sun is 5800 K. The mean radiuses of the Earth and the Sun are…
A: To calculate the steady-state surface temperature of the Earth, we can use the Stefan-Boltzmann law,…
Q: The diameter of a gas molecule is 2:4 x 1-10 m. Calculate the mean free path at NTP. Given Boltzmann…
A:
Q: Show that for an ideal Fermi-Dirac gas that (kT\ 4 57? (kT 1+ 12 2 2 Νμο P-5 V 16 \Ho)
A:
Q: Problem 2: Consider four independent magnetic atoms in a magnetic field B parallel to z axis at…
A: The partition function of single magnetic atom is, Z1=e-m0BkT+em0BkT For four of such magnetic atoms…
Q: The probability of a molecule of mass (m) in a gas at temp (7) having a speed of (c) is given by:…
A: Solution:-Given thatp=c2×m2πkT32×e-mc22kTmaximum probability is found when c=2kTm
Q: An approximate partition function for a gas of hard spheres can be obtained from the partition…
A:
Q: we shall approximate the partition function of a crystal by bv/2kT-ק 3N e'olkT e-hv/kT where hv/k=…
A: The partition function for a crystal is Q, its internal energy is U and then specific heat is given…
Q: How would I derive the product PV for a gas of photons in relation to their total internal energy?…
A:
Q: Answer in 90 minutes please.
A: Step 1:The probability of occupying states i and k, respectively…
Q: 1- a) Consider a physical system composed of N identical particles confined to a space of volume V.…
A:
Q: Real gases For helium gas, the critical temperature is Tk=5.2K and the critical pressure is…
A:
Q: (a) Consider nodal configuration shown below. (a) Derive the finite-difference equations under…
A: In this question we have to find the value of T(m,n). Please give positive feedback if the answer…
Q: The characteristic vibrational temperature for chlorine (Cl₂) gas is 805 K. Calculate the fraction…
A: The vibrational first excited state is reached by any diatomic when it gets excited and leaves its…
Q: Consider a system consisting of N independent indistinguishable identical molecules, each of which…
A: Given data, Energy of state no = Eo = 0 Energy of state n1 = E1 Temperature of the system = T
Q: According to the energy according to the equipartition theorem of degrees of freedom, what is the…
A:
Q: Problem 1: Consider a classical ideal gas in three dimensions, with N indistinguishable atoms…
A: Consider a classical ideal gas in three dimensions, with N indistinguishable atoms confined in a box…
Q: A 0.825 mol sample of NO,(g) initially at 298 K and 1.00 atm is held at constant volume while enough…
A: NO2 is linear molecule. Since the heat is supplied at constant volume Work done W = P∆V = 0 All the…
Q: For 3D free electron gas, the density of states counts the number of degenerate electron states dn…
A:
Q: When developing the partition functions for each degree of freedom in a diatomic ideal gas, we made…
A: Since we know that partition function is the sum of all boltzman constants. Z= Σgne-(βEn) for…
Q: The variation of the molar Gibbs free pn- ergy of a substance with temperature and pressure is given…
A:

Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 1 images

- Imagine a photon gas at an initial temperature of T = 1.4 K. What is the temperature of the photon gas (in K) after it has undergone a reversible adiabatic expansion to 2 times its original volume?Consider a system with 1000 particles that can only have two energies, ɛ, and with ɛ, > E,. The difference between these two values is Aɛ = ɛ, -& . Assume that gi = g2 = 1. Using the %3D %3D equation for the Boltzmann distribution graph the number of particles, ni and m, in states & n2, E and E, as a function of temperature for a Aɛ = 1×10-2' J and for a temperature range from 2 to 300 K. (Note: kg = 1.380x10-23 J K-!. %3D %3D (s,-s,) gLe Aɛ/ n2 or = e n,Consider a classical ideal gas of N diatomic heterogeneous molecules at temperature T. The charac- teristic rotational energy parameter is € = 1 and the natural frequency of vibrations is wo. Consider the temperature region where T≫er/kB, but T is of the order of ħwo/kB. Ignore contributions from all other internal modes. Calculate the canonical partition function, the average energy, and the heat capacity at constant volume, Cv.
- Show that the one-particle partition function Z₁ for a 2D ideal gas confined to area A is: A 2²/1 Z₁ = SThe exact differential for the Gibbs energy is given by dG = -SdT + VdP. The form of this differential implies which of the following relationships? O (7),- (), ƏG Әт ƏG др P T (37), - - (-), P T as ( x) - (*), = др T (327), = -(0)₁ == P TA diatomic gas molecule can be in one of two vibrational energy levels, separated by 0.1 eV. Give the probabilities to be in either state and use these to calculate their relative populations at room temperature, T≈ 300 K. [You may use that kB ≈ 8.6 × 10−5 K eV−1]