Concept explainers
Size and Life
Physicists look for simple models and general principles that underlie and explain diverse physical phenomena. In the first 13 chapters of this textbook, you’ve seen that just a handful of general principles and laws can be used to solve a wide range of problems. Can this approach have any relevance to a subject like biology? It may seem surprising, but there are general 'laws of biology“’ that apply, with quantitative accuracy, to organisms as diverse as elephants and mice.
Let’s look at an example. An elephant uses more metabolic power than a mouse. This is not surprising, as an elephant is much bigger. But recasting the data shows an interesting trend. When we looked at the energy required to raise the temperature of different substances, we considered specific heat. The “specific” meant that we considered the heat required for 1 kilogram. For animals, rather than metabolic rate, we can look at the specific metabolic rate, the metabolic power used per kilogram of tissue. If we factor out the mass difference between a mouse and an elephant, are their specific metabolic powers the same?
In fact, the specific metabolic rate varies quite a bit among mammals, as the graph of specific metabolic rate versus mass shows. But there is an interesting trend: All of the data points lie on a single smooth curve. In other words, there really is a biological law we can use to predict a mammal’s metabolic rate knowing only its mass M. In particular, the specific metabolic rate is proportional to M –0.25. Because a 4000 kg elephant is 160,000 times more massive than a 25 g mouse, the mouse’s specific metabolic power is (160,000)0.25 = 20 times that of the elephant. A law that shows how a property scales with the size of a system is called a scaling law.
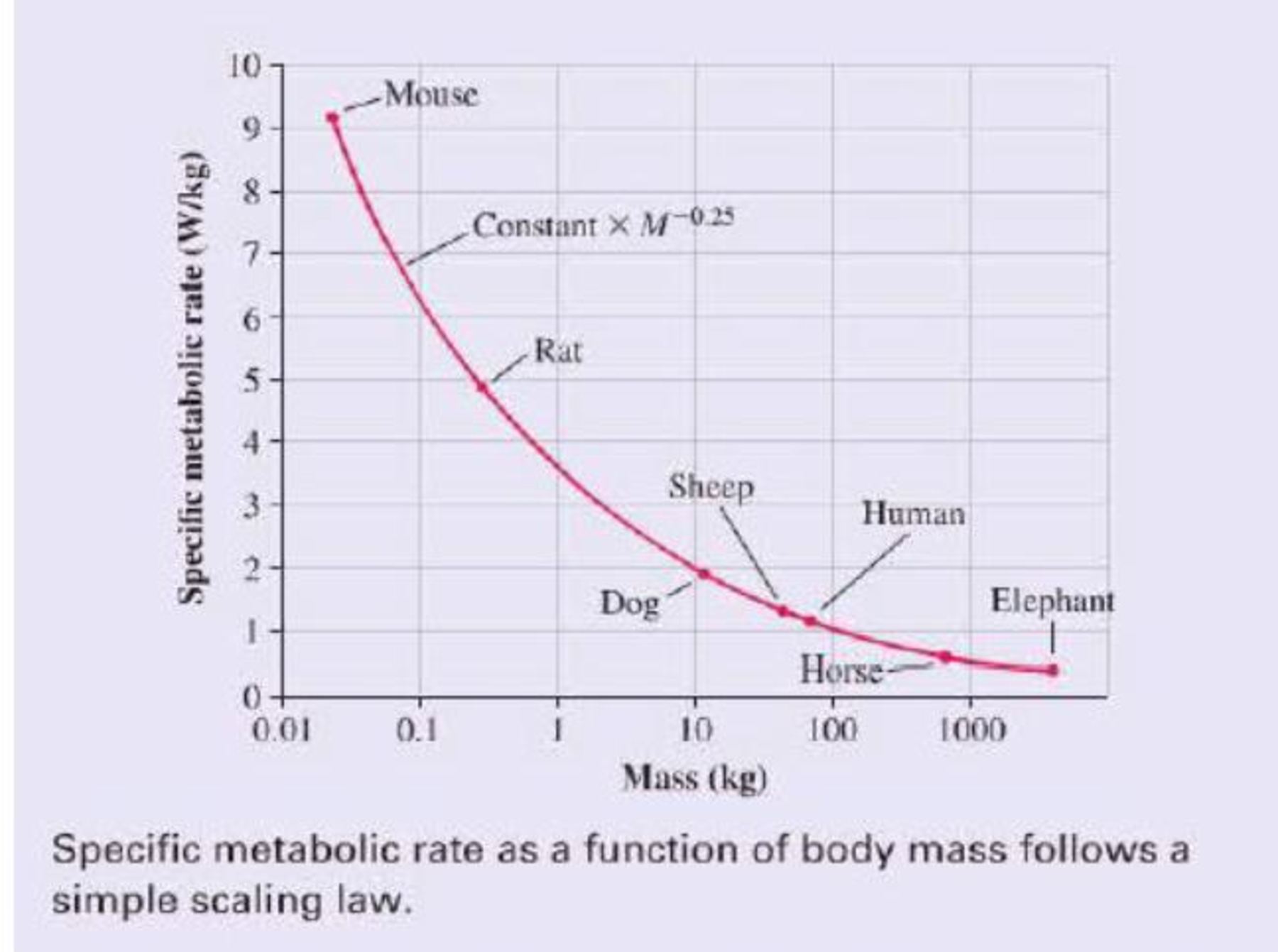
A similar scaling law holds for birds, reptiles, and even bacteria. Why should a single simple relationship hold true for organisms that range in size from a single cell to a 100 ton blue whale? Interestingly, no one knows for sure. It is a matter of current research to find out just what this and other scaling laws tell us about the nature of life.
Perhaps the metabolic-power scaling law is a result of
If heat dissipation were the only factor limiting metabolism, we can show that the specific metabolic rate should scale as M–0.33quite different from the M–0.25 scaling observed. Clearly, another factor is at work. Exactly what underlies the M–0.25 scaling is still a matter of debate, but some recent analysis suggests the scaling is due to limitations not of heat transfer but of fluid flow. Cells in mice, elephants, and all mammals receive nutrients and oxygen for metabolism from the bloodstream. Because the minimum size of a capillary is about the same for all mammals, the structure of the circulatory system must vary from animal to animal. The human aorta has a diameter of about 1 inch; in a mouse, the diameter is approximately l/20th of this. Thus a mouse has fewer levels of branching to smaller and smaller blood vessels as we move from the aorta to the capillaries. The smaller blood vessels in mice mean that viscosity is more of a factor throughout the circulatory system. The circulatory system of a mouse is quite different from that of ail elephant.
A model of specific metabolic rate based on blood-flow limitations predicts a M–0.25 law, exactly as observed. The model also makes other testable predictions. For example, the model predicts that the smallest possible mammal should have a body mass of about 1 gram—exactly the size of the smallest shrew. Even smaller animals have different types of circulatory' systems; in the smallest animals, nutrient transport is by diffusion alone. But the model can be extended to predict that the specific metabolic rate for these animals will follow a scaling law similar to that for mammals, exactly as observed. It is too soon to know if this model will ultimately prove to be correct, but it’s indisputable that there are large-scale regularities in biology that follow mathematical relationships based on the laws of physics.
The following questions are related to the passage "Size and Life" on the previous page.
A typical timber wolf has a mass of 40 kg, a typical jackrabbit a mass of 2.5 kg. Given the scaling law presented in the passage, we’d expect the specific metabolic rate of the jackrabbit to be higher by a factor of
- A. 2
- B. 4
- C. 8
- D. 16
Want to see the full answer?
Check out a sample textbook solution
Chapter P Solutions
College Physics: A Strategic Approach (4th Edition)
Additional Science Textbook Solutions
An Introduction to Thermal Physics
Essential University Physics: Volume 1 (3rd Edition)
University Physics with Modern Physics (14th Edition)
Physics for Scientists and Engineers: A Strategic Approach with Modern Physics (4th Edition)
Lecture- Tutorials for Introductory Astronomy
Physics for Scientists and Engineers: A Strategic Approach, Vol. 1 (Chs 1-21) (4th Edition)
- A typical bathroom sink faucet releases about two gallons of water every minute. a) If it takes you 2.5 minutes to brush your teeth and you leave the water run for the entire time, how much water would be used? b) If you only run the water for 30 seconds, how much water would be used?arrow_forwardNumerical and engineering analysis. I need a solution as soon as possiblearrow_forwardUPS ships a lot of boxes. There are different ways to measure how big is a box. For the following two boxes which box - box 1 or box 2 - do you think is "bigger" and why. You must pick only one of the boxes as the bigger box. Box 1: Dimensions: 18"×24"×12" that weighs 7.7 lbs Box 2: Dimensions: 24"×36"×6" that weighs 7.7 Ibs.arrow_forward
- I believe the solution to the problem at this url is incorrect and that the problem requires integration. I asked this problem once before and got a very different answer (64 gallons versus 11 gallons as provided in this answer). The previous solution was much more involved but I had a hard time following the solution so I asked the question again. I would like this problem solved by a different expert please. https://www.bartleby.com/questions-and-answers/5.-oil-flows-into-a-tank-according-to-the-rate-ft11-and-at-the-same-time-empties-out-at-the-rate-et-/cdf8b9dc-0b1c-4bfc-bb53-ad200f80692barrow_forwardThe average life expectancy in Japan is 81 years. What is this time in SI units?arrow_forwardRoughly how many floating-point operations can a supercomputer perform in a human lifetime?arrow_forward
- A scientist performs an experiment on all 8 planets in the solar system by comparing the lengths of the strips of paper tape required to record 10 data points. Therefore, which would we find that: A) The longest strip of paper is required for the planet: ? B) The shortest strip of paper is required for the planet: ? 8 Planets are Saturn, Earth, Venus, Uranus, Mars, Neptune, Jupiter, Mercuryarrow_forwardFor the remaining questions, you need to use Figure 1.4 to obtain the necessary orders of magnitude of lengths, masses, and times.About how many floating-point operations can a supercomputer perform each year?arrow_forwardDensity Here is a simple problem. Then we'll do one that requires a little more thought. The shape of a city is roughly a circle with a radius of 5 miles. If the population density for the city is 10,000 people per square mile, what is the population of the city? 11111 Now here's a problem that will stretch your problem solving skills. This time the city is also in the shape of a circle, but the population density is higher towards the center of the city and lower towards the outskirts. The numbers in the diagram represent miles. The population density is 10,000 people per square mile in the inner circle. In the first ring out from the center the density is 8,000 people per square mile, the second ring out it's 6,000, in the third ring out it's 4,000 and in the largest ring it's only 2,000 people per square mile. Finally, here is a three dimensional problem. Density is defined as weight per unit of volume. So which weighs more, a ball of zinc with a radius of 3 cm, or a ball of…arrow_forward
- UPVOTE WILL BE GIVEN! ANSWER CLEAR AND DETAILED. A cell of baker’s yeast is typically 4.0 mm in diameter. (Assume that it is spherical, even if they have a variety of shapes.) If you had 18 mL of yeast (i.e., about one tablespoon), about how many cells would it contain?arrow_forwardPlease begin with a fundamental equation and system definitions. and steps by steps Thank You!arrow_forwardCan you please help me with this question? I really appreciate your help! thank you!arrow_forward
 University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning


