Calculus: Early Transcendentals, 2nd Edition
2nd Edition
ISBN: 9780321965165
Author: William L. Briggs, Lyle Cochran, Bernard Gillett
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter D2.4, Problem 45E
Applications
43–46. Horizontal oscillators The equation of motion for a spring-block system that lies on a horizontal surface (see figure) is the same as the equation of motion for a vertically suspended system. As before, m is the mass of the block, k is the spring constant, c is the damping coefficient (perhaps due to friction as the block slides on the surface), and Fext is an external force. We let x(t) be the position of the block at time t, where x increases to the right and x = 0 is the position of the block at which the spring is neither stretched nor compressed.
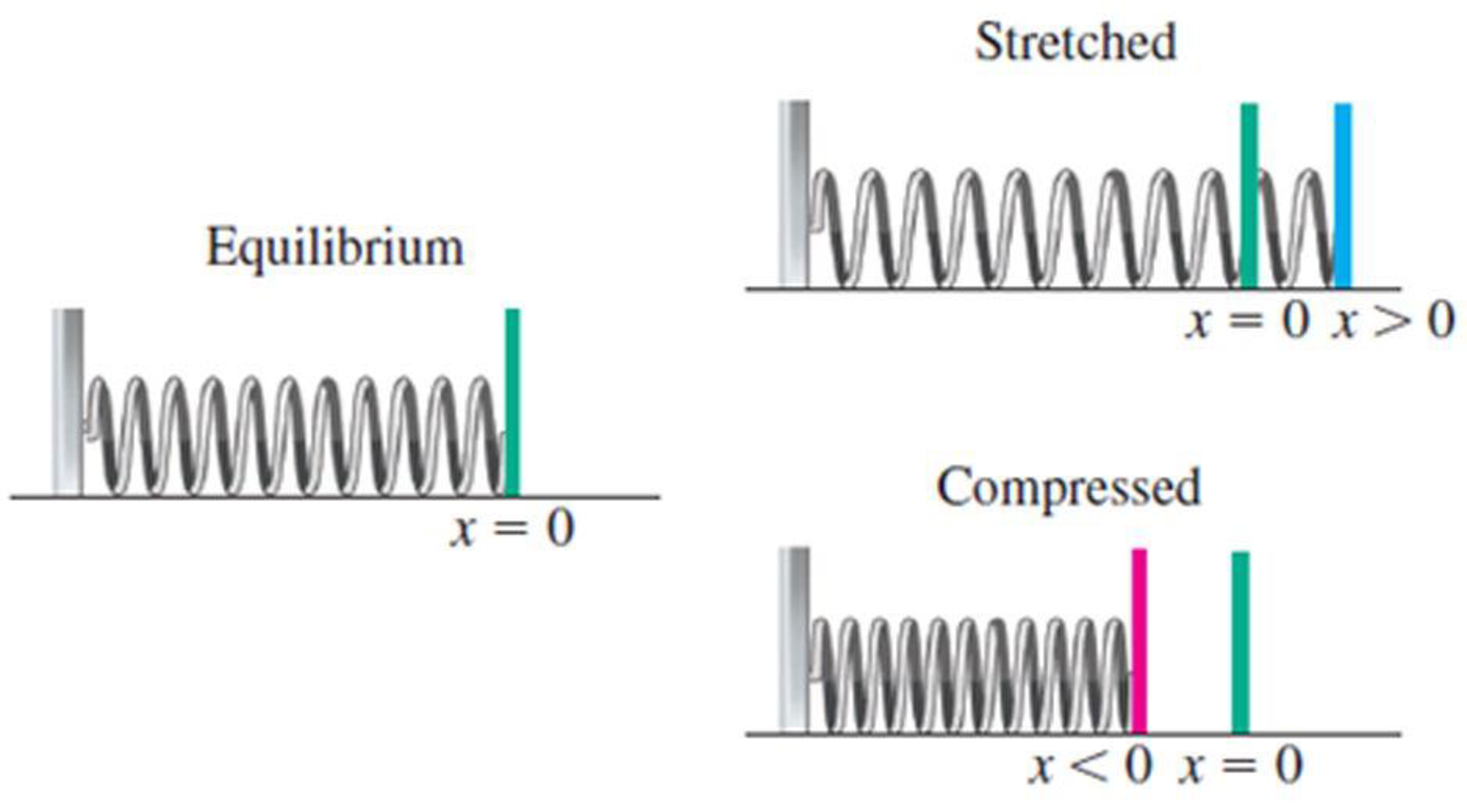
a. Find the position of the block in the following situations.
b. Graph the position function.
c. Describe the type of motion you observe.
45. m = 4 kg, c = 4 kg/s, k = 17 N/m,
Fext = 148 sin t, x(0) = 0, x′(0) = 0
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Q2/
verify that
f
grad = (h grad f- f grad h)
h
h₂
where and h are scalar factions.
Solve in paper
This question is a previous exam question. I am using it for practice but am stuck
in
Q. A firm
price of 501: If the Total cast is given by
perfect competition sells its products at the
TTC = 3Q² +2Q+5.
level of output will
will be the level of profit at
What
What
Devive the
Consumer
Curve
approach.
demand
the function
maximize
this firm's,
that
using
putput level.
the indifference
prpfit.
Q₂. The Total Cost equation in the production of bacon has
hypothetical factor
a
2
A
C=
"TC 1000+ 159" +03 ; Where ç. Kash, Bacao - metric bone
Compute
and
11" tonnes the
and
average
cost at output level of 10.
Stretch theme marginal cost of the
the
shope
Carve an
the production
average,
Cost arve
12 tonnes
and explain, the relationship between
Marginal Cost
product es tamen op d
Galaxy A71
01
Curve
in
Chapter D2 Solutions
Calculus: Early Transcendentals, 2nd Edition
Ch. D2.1 - Describe how to find the order of a differential...Ch. D2.1 - Prob. 2ECh. D2.1 - Prob. 3ECh. D2.1 - Give a general form of a second-order linear...Ch. D2.1 - Prob. 5ECh. D2.1 - Prob. 6ECh. D2.1 - Prob. 7ECh. D2.1 - Prob. 8ECh. D2.1 - Prob. 9ECh. D2.1 - Prob. 10E
Ch. D2.1 - Prob. 11ECh. D2.1 - Prob. 12ECh. D2.1 - Prob. 13ECh. D2.1 - Verifying solutions Verify by substitution that...Ch. D2.1 - Prob. 15ECh. D2.1 - Prob. 16ECh. D2.1 - Prob. 17ECh. D2.1 - Prob. 18ECh. D2.1 - Prob. 19ECh. D2.1 - Prob. 20ECh. D2.1 - Prob. 21ECh. D2.1 - Prob. 22ECh. D2.1 - Prob. 23ECh. D2.1 - Prob. 24ECh. D2.1 - Prob. 25ECh. D2.1 - Prob. 26ECh. D2.1 - Prob. 27ECh. D2.1 - Prob. 28ECh. D2.1 - Prob. 29ECh. D2.1 - Prob. 30ECh. D2.1 - Prob. 31ECh. D2.1 - Prob. 32ECh. D2.1 - Prob. 33ECh. D2.1 - Prob. 34ECh. D2.1 - Prob. 35ECh. D2.1 - Prob. 36ECh. D2.1 - Prob. 37ECh. D2.1 - Prob. 38ECh. D2.1 - Prob. 39ECh. D2.1 - Prob. 40ECh. D2.1 - Prob. 41ECh. D2.1 - Prob. 42ECh. D2.1 - Prob. 43ECh. D2.1 - Initial value problems Solve the following initial...Ch. D2.1 - Prob. 45ECh. D2.1 - Prob. 46ECh. D2.1 - Explain why or why not Determine whether the...Ch. D2.1 - Prob. 48ECh. D2.1 - Solution verification Verify by substitution that...Ch. D2.1 - Prob. 50ECh. D2.1 - Prob. 51ECh. D2.1 - Prob. 52ECh. D2.1 - Prob. 53ECh. D2.1 - Prob. 54ECh. D2.1 - Prob. 55ECh. D2.1 - Prob. 56ECh. D2.1 - Prob. 57ECh. D2.1 - Prob. 58ECh. D2.1 - Prob. 59ECh. D2.1 - Prob. 60ECh. D2.1 - Prob. 61ECh. D2.1 - Prob. 62ECh. D2.1 - Prob. 63ECh. D2.1 - Prob. 64ECh. D2.1 - Prob. 65ECh. D2.1 - Prob. 66ECh. D2.1 - Prob. 67ECh. D2.1 - Prob. 68ECh. D2.1 - Prob. 69ECh. D2.1 - Reduction of order Suppose you are solving a...Ch. D2.2 - Prob. 1ECh. D2.2 - Prob. 2ECh. D2.2 - Prob. 3ECh. D2.2 - Prob. 4ECh. D2.2 - Prob. 5ECh. D2.2 - Prob. 6ECh. D2.2 - Prob. 7ECh. D2.2 - Give the trial solution used to solve a...Ch. D2.2 - Prob. 9ECh. D2.2 - Prob. 10ECh. D2.2 - General solutions with distinct real roots Find...Ch. D2.2 - Prob. 12ECh. D2.2 - Prob. 13ECh. D2.2 - Prob. 14ECh. D2.2 - Initial value problems with distinct real roots...Ch. D2.2 - Prob. 16ECh. D2.2 - Prob. 17ECh. D2.2 - Prob. 18ECh. D2.2 - Prob. 19ECh. D2.2 - Prob. 20ECh. D2.2 - Prob. 21ECh. D2.2 - Prob. 22ECh. D2.2 - Prob. 23ECh. D2.2 - Prob. 24ECh. D2.2 - Prob. 25ECh. D2.2 - Prob. 26ECh. D2.2 - Prob. 27ECh. D2.2 - Prob. 28ECh. D2.2 - Prob. 29ECh. D2.2 - Prob. 30ECh. D2.2 - Prob. 31ECh. D2.2 - Prob. 32ECh. D2.2 - Prob. 33ECh. D2.2 - Prob. 34ECh. D2.2 - Initial value problems with Cauchy-Euler equations...Ch. D2.2 - Prob. 36ECh. D2.2 - Prob. 37ECh. D2.2 - Initial value problems with Cauchy-Euler equations...Ch. D2.2 - Prob. 39ECh. D2.2 - Prob. 42ECh. D2.2 - Prob. 43ECh. D2.2 - Prob. 44ECh. D2.2 - Prob. 45ECh. D2.2 - Prob. 46ECh. D2.2 - Prob. 47ECh. D2.2 - Prob. 48ECh. D2.2 - Prob. 49ECh. D2.2 - Prob. 50ECh. D2.2 - Prob. 51ECh. D2.2 - Cauchy-Euler equation with repeated roots It can...Ch. D2.2 - Prob. 53ECh. D2.2 - Prob. 54ECh. D2.2 - Prob. 55ECh. D2.2 - Prob. 56ECh. D2.2 - Prob. 57ECh. D2.2 - Prob. 58ECh. D2.2 - Prob. 59ECh. D2.2 - Prob. 60ECh. D2.2 - Prob. 61ECh. D2.2 - Cauchy-Euler equation with repeated roots One of...Ch. D2.2 - Prob. 63ECh. D2.2 - Prob. 64ECh. D2.2 - Prob. 65ECh. D2.2 - Prob. 66ECh. D2.3 - Explain how to find the general solution of the...Ch. D2.3 - Prob. 2ECh. D2.3 - Prob. 3ECh. D2.3 - Prob. 4ECh. D2.3 - Prob. 5ECh. D2.3 - Prob. 6ECh. D2.3 - Prob. 7ECh. D2.3 - Prob. 8ECh. D2.3 - Prob. 9ECh. D2.3 - Prob. 10ECh. D2.3 - Prob. 11ECh. D2.3 - Prob. 12ECh. D2.3 - Prob. 13ECh. D2.3 - Undetermined coefficients with exponentials Find a...Ch. D2.3 - Prob. 15ECh. D2.3 - Prob. 16ECh. D2.3 - Prob. 17ECh. D2.3 - Prob. 18ECh. D2.3 - Prob. 19ECh. D2.3 - Prob. 20ECh. D2.3 - Prob. 21ECh. D2.3 - Prob. 22ECh. D2.3 - Prob. 23ECh. D2.3 - Prob. 24ECh. D2.3 - Prob. 25ECh. D2.3 - Prob. 26ECh. D2.3 - Prob. 27ECh. D2.3 - Prob. 28ECh. D2.3 - Prob. 29ECh. D2.3 - Prob. 30ECh. D2.3 - Prob. 31ECh. D2.3 - Prob. 32ECh. D2.3 - Prob. 33ECh. D2.3 - Prob. 34ECh. D2.3 - Prob. 35ECh. D2.3 - Prob. 36ECh. D2.3 - Prob. 37ECh. D2.3 - Initial value problems Find the general solution...Ch. D2.3 - Prob. 39ECh. D2.3 - Prob. 40ECh. D2.3 - Prob. 41ECh. D2.3 - Prob. 42ECh. D2.3 - Prob. 43ECh. D2.3 - Prob. 44ECh. D2.3 - Prob. 45ECh. D2.3 - Prob. 46ECh. D2.3 - Prob. 47ECh. D2.3 - Prob. 48ECh. D2.3 - Prob. 49ECh. D2.3 - Prob. 50ECh. D2.3 - Prob. 51ECh. D2.3 - Variation of parameters Finding a particular...Ch. D2.4 - Explain the meaning of the words damped, undamped,...Ch. D2.4 - In the models discussed in this section, under...Ch. D2.4 - Prob. 3ECh. D2.4 - Prob. 4ECh. D2.4 - Prob. 5ECh. D2.4 - Prob. 6ECh. D2.4 - Prob. 7ECh. D2.4 - Prob. 8ECh. D2.4 - Prob. 9ECh. D2.4 - Free undamped oscillations Solve the initial value...Ch. D2.4 - Prob. 11ECh. D2.4 - Prob. 12ECh. D2.4 - Prob. 13ECh. D2.4 - Prob. 14ECh. D2.4 - Prob. 15ECh. D2.4 - Prob. 16ECh. D2.4 - Free damped oscillations Solve the initial value...Ch. D2.4 - Free damped oscillations Solve the initial value...Ch. D2.4 - Designing a shock absorber A shock absorber must...Ch. D2.4 - Designing a suspension system A spring in a...Ch. D2.4 - Forced damped oscillations 21.A 1-kg block hangs...Ch. D2.4 - Forced damped oscillations 22.A 20-kg block hangs...Ch. D2.4 - Prob. 23ECh. D2.4 - Prob. 24ECh. D2.4 - Prob. 25ECh. D2.4 - Prob. 26ECh. D2.4 - Prob. 27ECh. D2.4 - LCR circuits 28.The circuit in Exercise 27 (10-ohm...Ch. D2.4 - Prob. 29ECh. D2.4 - Prob. 30ECh. D2.4 - Prob. 31ECh. D2.4 - LCR circuits 32.Find the charge on the capacitor...Ch. D2.4 - Explain why or why not Determine whether the...Ch. D2.4 - Prob. 34ECh. D2.4 - Prob. 35ECh. D2.4 - Prob. 36ECh. D2.4 - Prob. 37ECh. D2.4 - Prob. 38ECh. D2.4 - Prob. 39ECh. D2.4 - Prob. 41ECh. D2.4 - Prob. 42ECh. D2.4 - Prob. 43ECh. D2.4 - Prob. 44ECh. D2.4 - Applications 4346.Horizontal oscillators The...Ch. D2.4 - Prob. 46ECh. D2.4 - Prob. 47ECh. D2.4 - Prob. 48ECh. D2.4 - Prob. 49ECh. D2.4 - Prob. 51ECh. D2.4 - Prob. 52ECh. D2.5 - Prob. 1ECh. D2.5 - Prob. 2ECh. D2.5 - Prob. 3ECh. D2.5 - Prob. 4ECh. D2.5 - Prob. 5ECh. D2.5 - Prob. 6ECh. D2.5 - Prob. 7ECh. D2.5 - Prob. 8ECh. D2.5 - Gain and phase lag functions Consider the...Ch. D2.5 - Prob. 10ECh. D2.5 - Prob. 11ECh. D2.5 - Solutions to oscillator equations Consider the...Ch. D2.5 - Prob. 13ECh. D2.5 - Solutions to oscillator equations Consider the...Ch. D2.5 - Prob. 15ECh. D2.5 - Prob. 16ECh. D2.5 - Prob. 17ECh. D2.5 - Prob. 18ECh. D2.5 - Analyzing circuit equations Consider the circuit...Ch. D2.5 - Prob. 20ECh. D2.5 - Prob. 21ECh. D2.5 - Prob. 22ECh. D2.5 - Prob. 23ECh. D2.5 - A high-pass filter Consider the LCR circuit shown...Ch. D2.5 - High-pass filters Consider the high-pass filter...Ch. D2.5 - Prob. 26ECh. D2.5 - High-pass filters Consider the high-pass filter...Ch. D2.5 - Prob. 28ECh. D2 - Prob. 1RECh. D2 - Prob. 2RECh. D2 - Prob. 3RECh. D2 - Prob. 4RECh. D2 - Solving homogeneous equations Find the general...Ch. D2 - Prob. 6RECh. D2 - Prob. 7RECh. D2 - Prob. 8RECh. D2 - Prob. 9RECh. D2 - Prob. 10RECh. D2 - Prob. 11RECh. D2 - Prob. 12RECh. D2 - Prob. 13RECh. D2 - Prob. 14RECh. D2 - Prob. 15RECh. D2 - Prob. 16RECh. D2 - Prob. 17RECh. D2 - Prob. 18RECh. D2 - Prob. 19RECh. D2 - Prob. 20RECh. D2 - Prob. 21RECh. D2 - Forced undamped oscillations A 4-kg block hangs on...Ch. D2 - Free damped oscillations A 0.2-kg block hangs on a...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- (b) For each of the following sets, 6 is an element of that set. (i) {x ER|x is an integer greater than 1} (ii) {x ЄR|x is the cube of an integer} (iii) {6, {6}} (iv) {{6},{6, {6}}} (v) {{{2}}}arrow_forwardQuestion 1 Reverse the order of integration to calculate .8 .2 A = = So² Son y1/3 cos² (x²) dx dy. Then the value of sin(A) is -0.952 0.894 0.914 0.811 0.154 -0.134 -0.583 O 0.686 1 ptsarrow_forward3 Calculate the integral approximations T and M6 for 2 x dx. Your answers must be accurate to 8 decimal places. T6= e to search M6- Submit answer Next item Answers Answer # m 0 T F4 F5 The Weather Channel UP DELL F6 F7 % 5 olo in 0 W E R T A S D F G ZX C F8 Score & 7 H FO F10 8 の K B N Marrow_forward
- Start with a circle of radius r=9. Form the two shaded regions pictured below. Let f(6) be the area of the shaded region on the left which has an arc and two straight line sides. Let g(6) be the area of the shaded region on the right which is a right triangle. Note that the areas of these two regions will be functions of 6; r=9 is fixed in the problem. 0 f(0) (a) Find a formula for f(6)= | | (b)Find a formula for g(6)= lim ƒ (6) (c) 80 = lim g (0) (d) 80 = lim (e) [f(8)/g(6)]= 0 g(0)arrow_forwardMy teacher's answer is: 4a⁷b⁶arrow_forwardExplain to me why the answer is: 4a⁷b⁶ if not then defend yoursarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Trigonometry - Harmonic Motion - Equation Setup; Author: David Hays;https://www.youtube.com/watch?v=BPrZnn3DJ6Q;License: Standard YouTube License, CC-BY
Simple Harmonic Motion - An introduction : ExamSolutions Maths Revision; Author: ExamSolutions;https://www.youtube.com/watch?v=tH2vldyP5OE;License: Standard YouTube License, CC-BY