Chapter 9.4, Problem 69P

Use the method of superposition to solve the following problems and assume that the flexural rigidity El of each beam is constant.

9.69 through 9.72 For the beam and loading shown, determine (a) the deflection at point C, (b) the slope at end A.

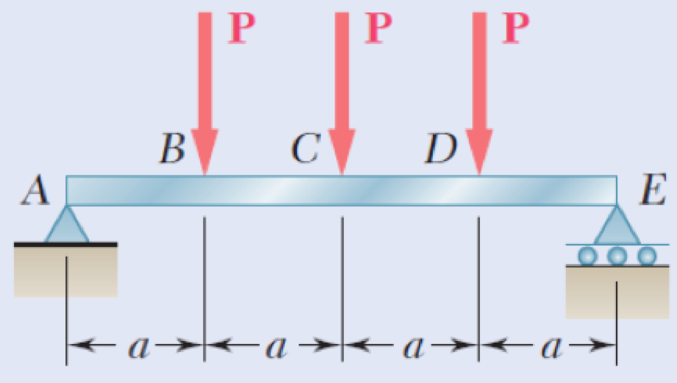
Fig. P9.69

To determine

Find the deflection at point C of the beam using superposition method.

Answer to Problem 69P

The deflection at point C of the beam is $\underset{\_}{y_C=\frac{19P{a}^3}{6EI}(\downarrow )}$.

Explanation of Solution

The flexural rigidity of the beam is EI.

Show the free-body diagram of the superimposed beam as in Figure 1.

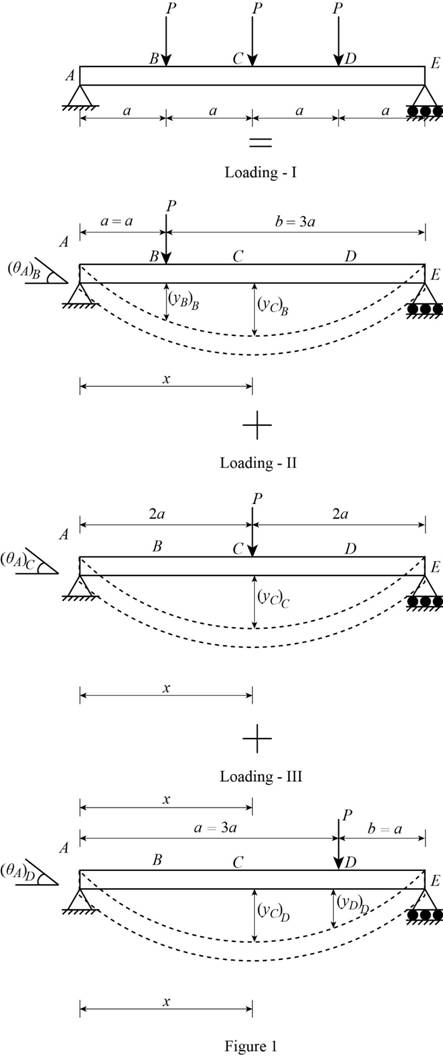
Loading I:

The downward load P is acting at point B of the beam.

Refer to case 5 in Appendix D “Beam Deflections and Slopes” in the textbook.

Write the deflection equation for concentrated load acting at any point in the simply supported beam.

$y=\frac{Pb}{6EIL}\left[x^3-\left(L^2-b^2\right)x\right];\text{when}x<a$

Consider $L=4a;a=a;b=3a;x=2a$.

When $x>a$, consider x as $\left(L-x\right)$ and interchange the notation of a and b.

Find the deflection at point C due to point load P at point B of the beam as follows;

$\begin{array}{c}{\left(y_C\right)}_B=\frac{Pa}{6EIL}\left[{\left(L-x\right)}^3-\left(L^2-a^2\right)\left(L-x\right)\right]\\ =\frac{Pa}{6EI\left(4a\right)}\left[{\left(4a-2a\right)}^3-\left({\left(4a\right)}^2-a^2\right)\left(4a-2a\right)\right]\\ =\frac{P}{24EI}\left[8a^3-\left(15a^2\right)\left(2a\right)\right]\\ =\frac{P}{24EI}\left[8a^3-30a^3\right]\end{array}$

$\begin{array}{c}{\left(y_C\right)}_B=-\frac{22P{a}^3}{24EI}\\ =-\frac{11P{a}^3}{12EI}\end{array}$

Loading II:

The downward point load P is acting at point C.

Refer to case 4 in Appendix D “Beam Deflections and Slopes” in the textbook.

Write the deflection equation for concentrated load acting at mid-point in the simply supported beam.

$y=-\frac{P{L}^3}{48EI}$

Consider $L=4a$.

Find the deflection at point C due to load P at point C as follows;

$\begin{array}{c}{\left(y_C\right)}_C=-\frac{P{\left(4a\right)}^3}{48EI}\\ =-\frac{64P{a}^3}{48EI}\\ =-\frac{4P{a}^3}{3EI}\end{array}$

Loading III:

The downward load P is acting at point D of the beam.

Refer to case 5 in Appendix D “Beam Deflections and Slopes” in the textbook.

Write the deflection equation for concentrated load acting at any point in the simply supported beam.

$y=\frac{Pb}{6EIL}\left[x^3-\left(L^2-b^2\right)x\right];\text{when}x<a$

Consider $L=4a;a=3a;b=a;x=2a$.

Find the deflection at point C due to point load P at point D of the beam as follows;

$\begin{array}{c}{\left(y_C\right)}_D=\frac{P\left(a\right)}{6EI\left(4a\right)}\left[{\left(2a\right)}^3-\left({\left(4a\right)}^2-a^2\right)\left(2a\right)\right]\\ =\frac{P}{24EI}\left[8a^3-\left(15a^2\right)\left(2a\right)\right]\\ =\frac{P}{24EI}\left[8a^3-30a^3\right]\\ =-\frac{11P{a}^3}{12EI}\end{array}$

Apply the superimposition concept.

Find the deflection at point C $\left(y_C\right)$ of the beam using the relation.

$y_C={\left(y_C\right)}_B+{\left(y_C\right)}_C+{\left(y_C\right)}_D$

Substitute $-\frac{11P{a}^3}{12EI}$ for ${\left(y_C\right)}_B$, $-\frac{4P{a}^3}{3EI}$ for ${\left(y_C\right)}_C$, and $-\frac{11P{a}^3}{12EI}$ for ${\left(y_C\right)}_D$.

$\begin{array}{c}{y}_C=-\frac{11P{a}^3}{12EI}-\frac{4P{a}^3}{3EI}-\frac{11P{a}^3}{12EI}\\ =-\frac{11P{a}^3}{12EI}-\frac{16P{a}^3}{12EI}-\frac{11P{a}^3}{12EI}\\ =-\frac{38P{a}^3}{12EI}\\ =\frac{19P{a}^3}{6EI}(\downarrow )\end{array}$

Therefore, the deflection at point C of the beam is $\underset{\_}{y_C=\frac{19P{a}^3}{6EI}(\downarrow )}$.

To determine

Find the slope at point A of the beam using superposition method.

Answer to Problem 69P

The slope at point A of the beam is $\underset{\_}{{\theta }_A=\frac{5P{a}^2}{2EI}\left(\text{Anticlockwise}\right)}$.

Explanation of Solution

The flexural rigidity of the beam is EI.

Refer to Figure (1) in Part (a);

Loading I:

The downward load P is acting at point B of the beam.

Refer to case 5 in Appendix D “Beam Deflections and Slopes” in the textbook.

Write the slope equation for concentrated load acting at any point in the simply supported beam.

${\theta }_A=-\frac{Pb\left(L^2-b^2\right)}{6EIL}$

Consider $L=4a;a=a;b=3a$.

Find the slope at point A due to point load P at point B of the beam as follows;

$\begin{array}{c}{\left({\theta }_A\right)}_B=-\frac{P\left(3a\right)\left({\left(4a\right)}^2-{\left(3a\right)}^2\right)}{6EI\left(4a\right)}\\ =-\frac{P\left(7a^2\right)}{8EI}\\ =-\frac{7P{a}^2}{8EI}\end{array}$

Loading II:

The downward point load P is acting at point C.

Refer to case 4 in Appendix D “Beam Deflections and Slopes” in the textbook.

Write the slope equation for concentrated load acting at mid-point in the simply supported beam.

$\theta =-\frac{P{L}^2}{16EI}$

Consider $L=4a;$.

Find the slope at point A due to load P at point C is;

$\begin{array}{c}{\left({\theta }_A\right)}_C=-\frac{P{\left(4a\right)}^2}{16EI}\\ =-\frac{P{a}^2}{EI}\end{array}$

Loading III:

The downward load P is acting at point D of the beam.

Refer to case 5 in Appendix D “Beam Deflections and Slopes” in the textbook.

Write the slope equation for concentrated load acting at any point in the simply supported beam.

${\theta }_A=-\frac{Pb\left(L^2-b^2\right)}{6EIL}$

Consider $L=4a;a=3a;b=a;x=2a$.

Find the slope at point A due to point load P at point D of the beam is;

$\begin{array}{c}{\left({\theta }_A\right)}_D=-\frac{Pa\left({\left(4a\right)}^2-a^2\right)}{6EI\left(4a\right)}\\ =-\frac{P\left(15a^2\right)}{24EI}\\ =-\frac{15P{a}^2}{24EI}\end{array}$

Apply the superimposition concept.

Find the slope at point A $\left({\theta }_A\right)$ of the beam using the relation.

${\theta }_A={\left({\theta }_A\right)}_B+{\left({\theta }_A\right)}_C+{\left({\theta }_A\right)}_D$

Substitute $-\frac{7P{a}^2}{8EI}$ for ${\left({\theta }_A\right)}_B$, $-\frac{P{a}^2}{EI}$ for ${\left({\theta }_A\right)}_C$, and $-\frac{15P{a}^2}{24EI}$ for ${\left({\theta }_A\right)}_D$.

$\begin{array}{c}{\theta }_A=-\frac{7P{a}^2}{8EI}-\frac{P{a}^2}{EI}-\frac{15P{a}^2}{24EI}\\ =-\frac{21P{a}^2}{24EI}-\frac{24P{a}^2}{24EI}-\frac{15P{a}^2}{24EI}\\ =-\frac{5P{a}^2}{2EI}\\ =\frac{5P{a}^2}{2EI}\left(\text{Anticlockwise}\right)\end{array}$

Therefore, the slope at point A of the beam is $\underset{\_}{{\theta }_A=\frac{5P{a}^2}{2EI}\left(\text{Anticlockwise}\right)}$.