Chapter 9.2, Problem 33PPS

(a)

To determine

To sketch: The graph for two options for the dish one that opens up and one that opens left.

Answer to Problem 33PPS

The graphs of the dish that opens up and one that opens left are shown in figure (1) and (2).

Explanation of Solution

Given:

The diameter of the dish is 146 feet.

The focus is 48 feet from the vertex.

Calculation:

Assume that the vertex of the parabola is at origin $\left(0,0\right)$ .

The sketch of the dish opening up is as follows.

  

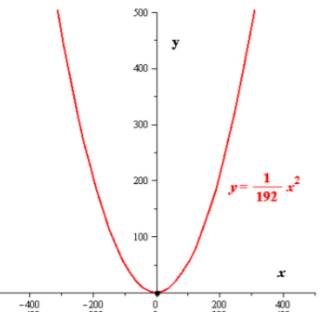
Figure (1)

The sketch of the dish opening left is as follows.

  

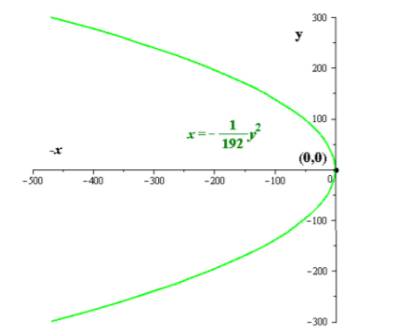
Figure (2)

Therefore, the graphs of the dish that opens up and one that opens left are shown in figure (1) and (2).

(b)

To determine

To write: The two equations that model the sketches in previous part.

Answer to Problem 33PPS

The equations are $y=\frac{1}{192}{x}^2$ and $x=-\frac{1}{192}{y}^2$ .

Explanation of Solution

Given:

The diameter of the dish is 146 feet.

The focus is 48 feet from the vertex.

Calculation:

Assume that the vertex of the parabola is at origin $\left(0,0\right)$ . So, $h=0$ and $k=0$ .

The distance between focus and vertex is equal to 48 feet. Then

  $\begin{array}{c}\left|\frac{1}{4a}\right|=48\\ \left|a\right|=\frac{1}{\left(4\right)\left(48\right)}\\ =\frac{1}{192}\end{array}$

The parabola opening up will be of the form $y=a{x}^2$ .

Substitute $a=\frac{1}{192}$ in the standard form $y=a{x}^2$ to model the equation opening up.

  $y=\frac{1}{192}{x}^2$

The parabola opening left will be of the form $x=-a{y}^2$ .

Substitute $a=\frac{1}{192}$ in the standard form $x=-a{y}^2$ to model the equation opening up.

  $x=-\frac{1}{192}{y}^2$

Therefore, the equations are $y=\frac{1}{192}{x}^2$ and $x=-\frac{1}{192}{y}^2$ .

(c)

To determine

To find: Whether it is matter to find the equation that must be used to find the depth of the dish.

Answer to Problem 33PPS

No, it does not matter which equation is used to find the depth of the dish.

Explanation of Solution

Given:

The diameter of the dish is 146 feet.

The focus is 48 feet from the vertex.

Calculation:

Two graphs are identical in all respect except for the direction in which they open.

Therefore, no, it does not matter which equation is used to find the depth of the dish.