
Concept explainers
Consider dimensionless velocity distribution in Couette flow (which is also called generalized Couette flow) with an applied pressure gradient which is obtained in the following from in Example 9-16 as
where
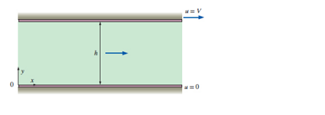
FIGURE P9-102
(a)
The velocity distribution is a superposition of Couette flow with a linear velocity distribution and Poiseuille flow with a parabolic velocity distribution.
Explanation of Solution
Given information:
The following figure shows that two infinite plates.
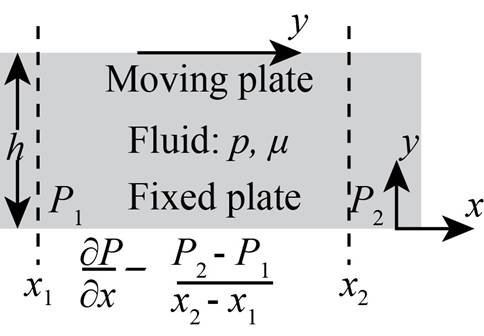
Figure-(1)
At the point of wall and fluid, the velocity of the fluid is equal to zero.
Write the expression for the pressure gradient.
Here, the arbitrary locations along the
Write the expression for
Here, the viscosity is
Write the expression for
Here, the density of the fluid is
Integrate Equation (II) with respect to
Here, the constant is
Integrate Equation (IV) with respect to
Here, the constant is
Integrate Equation (III) with respect to
Here, the constant is
The following figure represents planar Poiseuile flow.
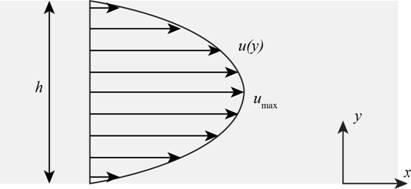
Figure (2)
Write the expression for dimensionless form of velocity field.
Here, the non- dimensional velocity along the arbitrary location along the
The Equation (VII) indicates that the pressure gradient is positive. If the both walls are stationary and pressure gradient is also there, then the flow should be planar Poiseuile flow.
Write the expression for non- dimensional velocity.
Write the expression for non- dimensional pressure.
Write the expression for non -dimensional distance
Calculation:
Substitute
Substitute
Substitute
The Equations (VII) indicates super position of the linear velocity profile
Substitute
(b)
The plot the
Answer to Problem 102P
The following Figure (4) represents the
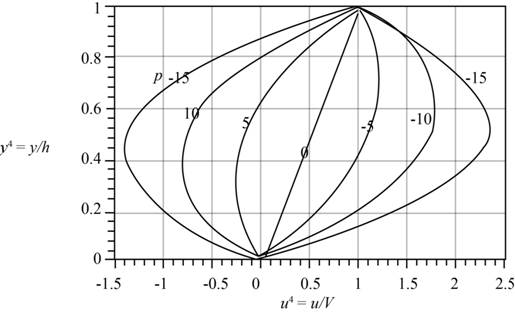
Explanation of Solution
At the point of wall and fluid, the velocity of the fluid is equal to zero.
Write the expression for the pressure gradient.
Here, the arbitrary locations along the
The following figure represents planar Poiseuile flow.
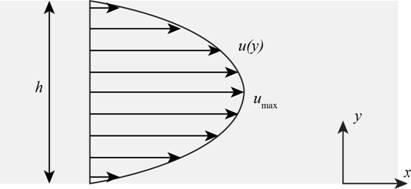
Figure (2)
Write the expression for dimensionless form of velocity field.
Here, the non dimensional velocities is
The Equation (VII) indicates that the pressure gradient is positive. If the both walls are stationary and pressure gradient is also there, then the flow should be planar Poiseuile flow.
Write the expression for non -dimensional velocity.
Write the expression for non -dimensional pressure.
The following figure represents the Couette flow between two parallel plates.
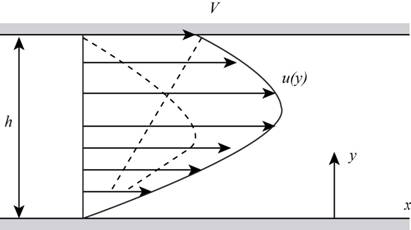
Figure-(3)
The pressure gradient is less than two, then pressure is decreasing in
Write the expression for non dimensional distance
Substitute
The following table represents the pressure gradient and x or y non- dimensional distance.
| | | |
| | | |
| | | |
| | | |
The following figure represents between
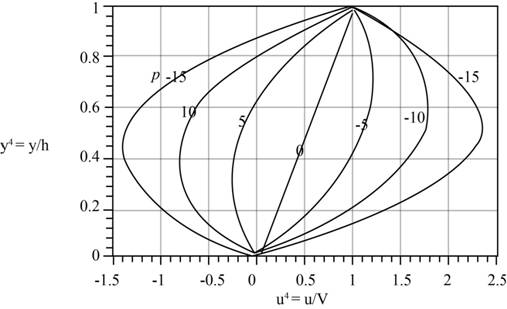
Figure (4)
The Figure (4) indicates that the pressure gradient is positive. If the both walls are stationary and pressure gradient is also there, then the flow should be planar Poiseuile flow.
Conclusion:
The following Figure (4) represents the
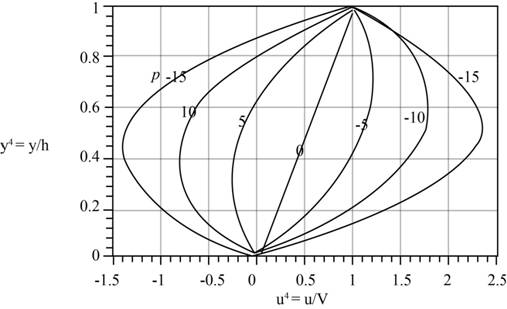
(c)
The position and magnitude of maximum dimensionless velocity.
Answer to Problem 102P
The expression for the pressure of fluid 1 is
The expression for the pressure of fluid
Explanation of Solution
Assume, at the point of wall and fluid, the velocity of the fluid is equal to zero.
Write the expression for velocity of the fluid 1,
Here, the velocity of fluid 1 is
Assume, the velocity of the fluid 2 at the free surface of the wall is equal to the velocity of the moving plates.
Write the expressions for the velocity of fluid 2.
Here, the velocity of fluid 2 is
Write the expression for velocity at interface.
Write the expression for rate of shear stress.
Here, the kinematic coefficient of fluid is
Write the expression for the shear stress acting on fluid
Here, the kinematic coefficient of fluid 1 is
Write the expression for the shear stress acting on fluid 2.
Here, the kinematic coefficient of fluid 2 is
Write the expression for the rate of shear stress at interface.
Write the expression for pressure at the bottom of the flow,
Here, the pressure is
Write the expression for the pressure at the interface of fluid 1.
Here, the pressure at the fluid 1 is
Write the expression for the pressure at the interface of fluid 2.
Here, the pressure at the fluid 1 is
At the interface of the fluid the pressure cannot have discontinuity and the surface is ignored.
Write the expression for the pressure at interface of fluid.
Here, the velocity of flow for fluid
Write the expression for
Here, the density of the fluid 1 is
Write the expression for
Here, the density of the fluid 2 is
Calculation:
Integrate Equation (XXVII) with respect to
Here, the constant is
Substitute
Substitute
Integrate Equation (XXVIII) with respect to
Here, the constant is
Substitute
Substitute
Substitute
Conclusion:
The expression for the pressure of fluid 1 is
The expression for the pressure of fluid 2 is
(d)
The position and magnitude of the maximum dimensionless velocity.
Answer to Problem 102P
The position of the maximum dimensionless velocity is
The magnitude of the maximum dimensionless velocity is
Explanation of Solution
Given information:
Write the expression for the position of the velocity.
Here, the viscosity of the fluid is
The following figure represents positions of the velocities.
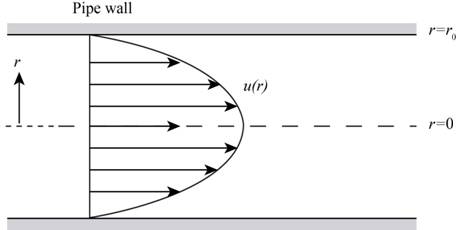
Figure-(5)
Write the expression for the radial velocity
Here, the maximum velocity is
The following figure represents the magnitude of the velocity.
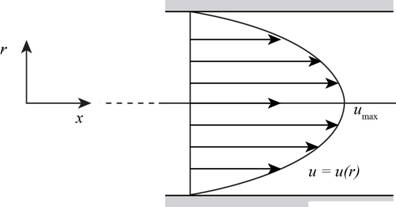
Figure-(6)
Write the expression for magnitude.
Here the magnitude is
Substitute
Conclusion:
The position of the maximum dimensionless velocity is
The magnitude of the maximum dimensionless velocity is
Want to see more full solutions like this?
Chapter 9 Solutions
Fluid Mechanics: Fundamentals and Applications
- auto controlsarrow_forward1 Pleasearrow_forwardA spring cylinder system measures the pressure. Determine which spring can measure pressure between 0-1 MPa with a large excursion. The plate has a diameter of 20 mm. Also determine the displacement of each 0.1 MPa step.Spring power F=c x fF=Springpower(N)c=Spring constant (N/mm)f=Suspension (mm) How do I come up with right answer?arrow_forward
- A lift with a counterweight is attached to the ceiling. The attachment is with 6 stainless and oiled screws. What screw size is required? What tightening torque? - The lift weighs 500 kg and can carry 800 kg. - Counterweight weight 600 kg - Durability class 12.8 = 960 MPa- Safety factor ns=5+-Sr/Fm= 0.29Gr =0.55arrow_forwardKnowing that a force P of magnitude 750 N is applied to the pedal shown, determine (a) the diameter of the pin at C for which the average shearing stress in the pin is 40 MPa, (b) the corresponding bearing stress in the pedal at C, (c) the corresponding bearing stress in each support bracket at C. 75 mm 300 mm- mm A B P 125 mm 5 mm C Darrow_forwardAssume the B frame differs from the N frame through a 90 degree rotation about the second N base vector. The corresponding DCM description is: 1 2 3 4 5 6 9 # adjust the return matrix values as needed def result(): dcm = [0, 0, 0, 0, 0, 0, 0, 0, 0] return dcmarrow_forward
- Find the reaction at A and B The other response I got was not too accurate,I need expert solved answer, don't use Artificial intelligence or screen shot it solvingarrow_forwardNo chatgpt plsarrow_forwardSolve for the reaction of all the forces Don't use artificial intelligence or screen shot it, only expert should solvearrow_forward
- No chatgpt plsarrow_forwardA six cylinder petrol engine has a compression ratio of 5:1. The clearance volume of each cylinder is 110CC. It operates on the four-stroke constant volume cycle and the indicated efficiency ratio referred to air standard efficiency is 0.56. At the speed of 2400 rpm. 44000KJ/kg. Determine the consumes 10kg of fuel per hour. The calorific value of fuel average indicated mean effective pressure.arrow_forwardThe members of a truss are connected to the gusset plate as shown in (Figure 1). The forces are concurrent at point O. Take = 90° and T₁ = 7.5 kN. Part A Determine the magnitude of F for equilibrium. Express your answer to three significant figures and include the appropriate units. F= 7.03 Submit ? kN Previous Answers Request Answer × Incorrect; Try Again; 21 attempts remaining ▾ Part B Determine the magnitude of T2 for equilibrium. Express your answer to three significant figures and include the appropriate units. Figure T₂ = 7.03 C T2 |? KN Submit Previous Answers Request Answer × Incorrect; Try Again; 23 attempts remaining Provide Feedbackarrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





