A Problem Solving Approach To Mathematics For Elementary School Teachers, Loose Leaf Edition Plus Mylab Math With Pearson Etext -- 18 Week Access Card Package (13th Edition)
13th Edition
ISBN: 9780136209409
Author: Rick Billstein, Shlomo Libeskind, Johnny Lott, Barbara Boschmans
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 8.1A, Problem 7A
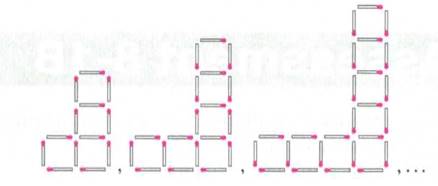
Ryan is building matchstick sequences as shown. How many matchsticks will he use for the nth figure if the pattern of adding one new square horizontally and one vertically continues?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
these are solutions to a tutorial that was done and im a little lost. can someone please explain to me how these iterations function, for example i Do not know how each set of matrices produces a number if someine could explain how its done and provide steps it would be greatly appreciated thanks.
Q1) Classify the following statements as a true or false statements
a. Any ring with identity is a finitely generated right R module.-
b. An ideal 22 is small ideal in Z
c. A nontrivial direct summand of a module cannot be large or small submodule
d. The sum of a finite family of small submodules of a module M is small in M
A module M 0 is called directly indecomposable if and only if 0 and M are
the only direct summands of M
f. A monomorphism a: M-N is said to split if and only if Ker(a) is a direct-
summand in M
& Z₂ contains no minimal submodules
h. Qz is a finitely generated module
i. Every divisible Z-module is injective
j. Every free module is a projective module
Q4) Give an example and explain your claim in each case
a) A module M which has two composition senes 7
b) A free subset of a modale
c) A free module
24
d) A module contains a direct summand submodule 7,
e) A short exact sequence of modules 74.
*************
*********************************
Q.1) Classify the following statements as a true or false statements:
a. If M is a module, then every proper submodule of M is contained in a maximal
submodule of M.
b. The sum of a finite family of small submodules of a module M is small in M.
c. Zz is directly indecomposable.
d. An epimorphism a: M→ N is called solit iff Ker(a) is a direct summand in M.
e. The Z-module has two composition series.
Z
6Z
f. Zz does not have a composition series.
g. Any finitely generated module is a free module.
h. If O→A MW→ 0 is short exact sequence then f is epimorphism.
i. If f is a homomorphism then f-1 is also a homomorphism.
Maximal C≤A if and only if is simple.
Sup
Q.4) Give an example and explain your claim in each case:
Monomorphism not split.
b) A finite free module.
c) Semisimple module.
d) A small submodule A of a module N and a homomorphism op: MN, but
(A) is not small in M.
Chapter 8 Solutions
A Problem Solving Approach To Mathematics For Elementary School Teachers, Loose Leaf Edition Plus Mylab Math With Pearson Etext -- 18 Week Access Card Package (13th Edition)
Ch. 8.1 - Student were asked to write an algebraic...Ch. 8.1 - a. Choose seven consecutive term in any arithmetic...Ch. 8.1 - A teacher instructed her class as follows: Take...Ch. 8.1 - A teacher instructed her class to take any number...Ch. 8.1 - Give an example of geometric sequence whose first...Ch. 8.1 - Joel says that there is no reason to have more...Ch. 8.1 - A student wonders if sets can ever be considered...Ch. 8.1 - A student wants to know how can she prove that a...Ch. 8.1 - Prob. 9MCCh. 8.1 - Prob. 11MC
Ch. 8.1 - Prob. 1NAEPCh. 8.1 - 2+n 5 3+n 6 4+n 7 5+n 8 What number does n...Ch. 8.1 - Prob. 3NAEPCh. 8.1 - Melissa saves money for six weeks to buy a...Ch. 8.1A - Translate the following phrases into algebraic...Ch. 8.1A - Prob. 2ACh. 8.1A - In the tile pattern for an arithmetic sequence of...Ch. 8.1A - Prob. 4ACh. 8.1A - In a college, there are P professors and S...Ch. 8.1A - Suppose a class has g girls and b boys, and there...Ch. 8.1A - Ryan is building matchstick sequences as shown....Ch. 8.1A - Prob. 8ACh. 8.1A - For a particular event, a student pays 5 per...Ch. 8.1A - Prob. 10ACh. 8.1A - Prob. 11ACh. 8.1A - Prob. 12ACh. 8.1A - Find the first term and the difference in an...Ch. 8.1A - Prob. 14ACh. 8.1A - A ball is dropped from a height of 10 ft and...Ch. 8.1A - Jake bought contact lenses and was told to wear...Ch. 8.1A - Construct a Fibonacci-like sequence that is also...Ch. 8.1A - Prob. 18ACh. 8.1B - Translate the following phrases into algebraic...Ch. 8.1B - Prob. 2ACh. 8.1B - Discover a possible tile pattern in the following...Ch. 8.1B - In the following descriptions, write an expression...Ch. 8.1B - Prob. 5ACh. 8.1B - Suppose there are c chairs and t tables in a...Ch. 8.1B - Prob. 7ACh. 8.1B - Prob. 8ACh. 8.1B - Prob. 9ACh. 8.1B - Matt has twice as many as stickers as David. If...Ch. 8.1B - Prob. 11ACh. 8.1B - Prob. 12ACh. 8.1B - Find the first term and the difference in an...Ch. 8.1B - Find the first term in a geometric sequence if the...Ch. 8.1B - A ball is dropped from a height of 20 meters and...Ch. 8.1B - Consider the following arithmetic sequences....Ch. 8.1B - Prob. 18ACh. 8.2 - Students were asked to find three consecutive...Ch. 8.2 - Prob. 2MCCh. 8.2 - Create an equation with x on both sides of the...Ch. 8.2 - Prob. 4MCCh. 8.2 - Prob. 5MCCh. 8.2 - Prob. 6MCCh. 8.2 - Prob. 7MCCh. 8.2 - Prob. 8MCCh. 8.2 - A student was told that in order to check a...Ch. 8.2 - Prob. 10MCCh. 8.2 - Olivier said that his teacher would not accept 5=x...Ch. 8.2 - A student claimed that the equation 2x=3y has...Ch. 8.2 - Prob. 13MCCh. 8.2 - Prob. 14MCCh. 8.2 - Prob. 15MCCh. 8.2 - Prob. 16MCCh. 8.2 - Prob. 17MCCh. 8.2 - Prob. 18MCCh. 8.2 - Write an algebraic equation relating the variables...Ch. 8.2 - a. Show that the sum of every three consecutive...Ch. 8.2 - Prob. 21MCCh. 8.2 - Prob. 22MCCh. 8.2 - Prob. 1NAEPCh. 8.2 - Prob. 2NAEPCh. 8.2 - Archaeologists measure the lengths of certain...Ch. 8.2 - Jessica makes clay bowls. The following equation...Ch. 8.2A - Consider the balances: What is the value of each...Ch. 8.2A - Prob. 2ACh. 8.2A - Solve each of the following if x is a real number....Ch. 8.2A - Ryan is building a matchstick sequence so that one...Ch. 8.2A - For a particular event, 812 tickets were sold for...Ch. 8.2A - An estate of 486,000 is left to three siblings....Ch. 8.2A - A 10-ft board is to be cut into three pieces: two...Ch. 8.2A - Prob. 8ACh. 8.2A - Miriam is 10 years older than Ricardo. Two years...Ch. 8.2A - In a college, 20 times as many undergraduate...Ch. 8.2A - A farmer has 1200 yd of fencing to enclose a...Ch. 8.2A - The sum of three consecutive terms in the...Ch. 8.2A - Carlos bought 430 feet of fencing. With this, he...Ch. 8.2A - The sum of four consecutive natural numbers is...Ch. 8.2B - Consider the following balances: a. Which shape...Ch. 8.2B - Prob. 2ACh. 8.2B - Prob. 3ACh. 8.2B - Prob. 4ACh. 8.2B - Prob. 5ACh. 8.2B - Prob. 6ACh. 8.2B - Prob. 7ACh. 8.2B - Matt has twice as many stickers as David. How many...Ch. 8.2B - Prob. 9ACh. 8.2B - Prob. 10ACh. 8.2B - Prob. 11ACh. 8.2B - Prob. 12ACh. 8.2B - Prob. 13ACh. 8.3 - Prob. 1MCCh. 8.3 - Prob. 2MCCh. 8.3 - Prob. 4MCCh. 8.3 - Prob. 7MCCh. 8.3 - Prob. 8MCCh. 8.3 - Prob. 9MCCh. 8.3 - Prob. 10MCCh. 8.3 - Prob. 11MCCh. 8.3 - Prob. 12MCCh. 8.3 - Prob. 13MCCh. 8.3 - Prob. 14MCCh. 8.3 - Prob. 15MCCh. 8.3 - Prob. 16MCCh. 8.3 - Prob. 17MCCh. 8.3 - Prob. 18MCCh. 8.3 - Refer to the School Book page on page 456 and...Ch. 8.3 - Prob. 20MCCh. 8.3 - Prob. 21MCCh. 8.3 - Prob. 22MCCh. 8.3 - Prob. 23MCCh. 8.3 - Prob. 24MCCh. 8.3 - Prob. 1NAEPCh. 8.3 - Prob. 2NAEPCh. 8.3 - Prob. 3NAEPCh. 8.3A - Prob. 1ACh. 8.3A - Which of the following are function from the set...Ch. 8.3A - a. Draw an arrow diagram of a function with domain...Ch. 8.3A - Suppose f(x)=2x+1 and the domain {0,1,2,3,4}....Ch. 8.3A - Prob. 5ACh. 8.3A - a. Make an arrow diagram for each of the...Ch. 8.3A - The dosage of a certain drug is related to the...Ch. 8.3A - For each of the following, guess what might be...Ch. 8.3A - Quick-Talk advertises monthly cellualr...Ch. 8.3A - For each of the following sequenceseither...Ch. 8.3A - Consider the following two function machines. Find...Ch. 8.3A - In problem 11, find the all inputs for which the...Ch. 8.3A - a. Repeat exercise 11 when the bottom machine...Ch. 8.3A - Consider the function f(x)=x+b, where b is a...Ch. 8.3A - Let t(n) be the function that assigns the nth term...Ch. 8.3A - a. If f(x)=x2 and the output is 2, find all...Ch. 8.3A - Consider a function machine that accepts inputs as...Ch. 8.3A - The following graph shows the relationship between...Ch. 8.3A - A ball is shot out of a cannon at ground level....Ch. 8.3A - Prob. 20ACh. 8.3A - Prob. 21ACh. 8.3A - Which of the following equations or inequalities...Ch. 8.3A - Which of the following are graphs of fucntions and...Ch. 8.3B - The following sets of ordered pairs are functions....Ch. 8.3B - Prob. 2ACh. 8.3B - Prob. 4ACh. 8.3B - Prob. 5ACh. 8.3B - Prob. 6ACh. 8.3B - Prob. 7ACh. 8.3B - Prob. 8ACh. 8.3B - Prob. 9ACh. 8.3B - Prob. 10ACh. 8.3B - Prob. 11ACh. 8.3B - In problem 11, find the all inputs for which the...Ch. 8.3B - Prob. 13ACh. 8.3B - Prob. 14ACh. 8.3B - Prob. 15ACh. 8.3B - Prob. 16ACh. 8.3B - Prob. 17ACh. 8.3B - Prob. 18ACh. 8.3B - Prob. 19ACh. 8.3B - Prob. 20ACh. 8.3B - Prob. 21ACh. 8.3B - Prob. 22ACh. 8.3B - Prob. 23ACh. 8.4 - In this chapter, an arithmetic sequence was...Ch. 8.4 - Prob. 2MCCh. 8.4 - Prob. 3MCCh. 8.4 - Prob. 4MCCh. 8.4 - Prob. 5MCCh. 8.4 - Prob. 6MCCh. 8.4 - Prob. 7MCCh. 8.4 - Prob. 8MCCh. 8.4 - Prob. 9MCCh. 8.4 - Prob. 10MCCh. 8.4 - Janis noticed that in the simultaneous equations...Ch. 8.4 - Prob. 12MCCh. 8.4 - Prob. 13MCCh. 8.4 - Prob. 14MCCh. 8.4 - Prob. 15MCCh. 8.4 - Prob. 16MCCh. 8.4 - Prob. 17MCCh. 8.4 - Prob. 18MCCh. 8.4 - Prob. 19MCCh. 8.4 - Prob. 20MCCh. 8.4 - Prob. 1NAEPCh. 8.4 - Prob. 2NAEPCh. 8.4 - Prob. 3NAEPCh. 8.4 - Prob. 4NAEPCh. 8.4A - The graph of y=mx is given in the following...Ch. 8.4A - Prob. 2ACh. 8.4A - Find the x-intercept and y-intercept for the...Ch. 8.4A - Prob. 4ACh. 8.4A - Write each of the following equations in...Ch. 8.4A - For each of the following, write the equation of...Ch. 8.4A - Find the coordinates of two other collinear points...Ch. 8.4A - Prob. 8ACh. 8.4A - Prob. 9ACh. 8.4A - Find the equation for each of the following lines....Ch. 8.4A - For each of the following, find the slope, if...Ch. 8.4A - Write the equation of each line in exercise 11....Ch. 8.4A - Wildlife experts found that the number of chirps C...Ch. 8.4A - The lines AB and BC shown in the figure are graph...Ch. 8.4A - What do the graphs of the following equations have...Ch. 8.4A - Suppose a car is travelling at constant speed of...Ch. 8.4A - Solve each of the following systems, if possible....Ch. 8.4A - The owner of a 5000-gal oil truck loads the truck...Ch. 8.4A - Josephines bank contains 27 coins. If all the...Ch. 8.4B - Prob. 2ACh. 8.4B - ASSESSMENT 8-4B Find the x-intercept and...Ch. 8.4B - Prob. 4ACh. 8.4B - Prob. 5ACh. 8.4B - Prob. 6ACh. 8.4B - ASSESSMENT 8-4B Find the coordinates of two other...Ch. 8.4B - Prob. 8ACh. 8.4B - Prob. 9ACh. 8.4B - ASSESSMENT 8-4B Find the equation for each of the...Ch. 8.4B - ASSESSMENT 8-4B For each of the following, find...Ch. 8.4B - ASSESSMENT 8-4B Write the equation of each line in...Ch. 8.4B - Prob. 13ACh. 8.4B - Prob. 14ACh. 8.4B - Prob. 15ACh. 8.4B - Prob. 16ACh. 8.4B - Solve each of the following systems, if possible....Ch. 8.CR - Each of the following is a geometric sequence....Ch. 8.CR - Prob. 2CRCh. 8.CR - a. If the first two terms of an arithmetic...Ch. 8.CR - There are 13 times as many students as professors...Ch. 8.CR - Write a sentence that gives the same information...Ch. 8.CR - Write an equation to find the number of feet given...Ch. 8.CR - The sum of n whole numbers is S. If each number is...Ch. 8.CR - I am thinking a whole number. If I divide it by...Ch. 8.CR - a. Think of a number. Add 17. Double the result....Ch. 8.CR - Find all the values of x that satisfy the...Ch. 8.CR - Mike has 3 times as many baseball cards as Jordan,...Ch. 8.CR - Jeannie has 10 books overdue at the library. She...Ch. 8.CR - Three children deliver all the newspapers in a...Ch. 8.CR - Which of the following sets of ordered pairs are...Ch. 8.CR - Prob. 15CRCh. 8.CR - Prob. 16CRCh. 8.CR - Prob. 17CRCh. 8.CR - Prob. 18CRCh. 8.CR - Prob. 19CRCh. 8.CR - Prob. 20CRCh. 8.CR - Graph each of the following equations: a. y=-2x+5...Ch. 8.CR - Prob. 22CRCh. 8.CR - Solve the following equations if possible: a....Ch. 8 - NOW TRY THIS Noah has some white square tiles and...Ch. 8 - Prob. 2NTCh. 8 - NOW TRY THIS What are the values of and in...Ch. 8 - NOW TRY THIS Solve the Newspaper Delivery problem...Ch. 8 - NOW TRY THIS Determine which of the following are...Ch. 8 - NOW TRY THIS a. Use the slope formula to find the...
Additional Math Textbook Solutions
Find more solutions based on key concepts
1. How much money is Joe earning when he’s 30?
Pathways To Math Literacy (looseleaf)
23. A plant nursery sells two sizes of oak trees to landscapers. Large trees cost the nursery $120 from the gro...
College Algebra (Collegiate Math)
Students in a Listening Responses class bought 40 tickets for a piano concert. The number of tickets purchased ...
Elementary and Intermediate Algebra: Concepts and Applications (7th Edition)
153. A rain gutter is made from sheets of aluminum that are 20 inches wide. As shown in the figure, the edges ...
College Algebra (7th Edition)
(a) Make a stem-and-leaf plot for these 24 observations on the number of customers who used a down-town CitiBan...
APPLIED STAT.IN BUS.+ECONOMICS
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- Prove that Σ prime p≤x p=3 (mod 10) 1 Ρ = for some constant A. log log x + A+O 1 log x "arrow_forwardProve that, for x ≥ 2, d(n) n2 log x = B ― +0 X (금) n≤x where B is a constant that you should determine.arrow_forwardProve that, for x ≥ 2, > narrow_forwardI need diagram with solutionsarrow_forwardT. Determine the least common denominator and the domain for the 2x-3 10 problem: + x²+6x+8 x²+x-12 3 2x 2. Add: + Simplify and 5x+10 x²-2x-8 state the domain. 7 3. Add/Subtract: x+2 1 + x+6 2x+2 4 Simplify and state the domain. x+1 4 4. Subtract: - Simplify 3x-3 x²-3x+2 and state the domain. 1 15 3x-5 5. Add/Subtract: + 2 2x-14 x²-7x Simplify and state the domain.arrow_forwardQ.1) Classify the following statements as a true or false statements: Q a. A simple ring R is simple as a right R-module. b. Every ideal of ZZ is small ideal. very den to is lovaginz c. A nontrivial direct summand of a module cannot be large or small submodule. d. The sum of a finite family of small submodules of a module M is small in M. e. The direct product of a finite family of projective modules is projective f. The sum of a finite family of large submodules of a module M is large in M. g. Zz contains no minimal submodules. h. Qz has no minimal and no maximal submodules. i. Every divisible Z-module is injective. j. Every projective module is a free module. a homomorp cements Q.4) Give an example and explain your claim in each case: a) A module M which has a largest proper submodule, is directly indecomposable. b) A free subset of a module. c) A finite free module. d) A module contains no a direct summand. e) A short split exact sequence of modules.arrow_forward1 2 21. For the matrix A = 3 4 find AT (the transpose of A). 22. Determine whether the vector @ 1 3 2 is perpendicular to -6 3 2 23. If v1 = (2) 3 and v2 = compute V1 V2 (dot product). .arrow_forward7. Find the eigenvalues of the matrix (69) 8. Determine whether the vector (£) 23 is in the span of the vectors -0-0 and 2 2arrow_forward1. Solve for x: 2. Simplify: 2x+5=15. (x+3)² − (x − 2)². - b 3. If a = 3 and 6 = 4, find (a + b)² − (a² + b²). 4. Solve for x in 3x² - 12 = 0. -arrow_forward5. Find the derivative of f(x) = 6. Evaluate the integral: 3x3 2x²+x— 5. - [dz. x² dx.arrow_forward5. Find the greatest common divisor (GCD) of 24 and 36. 6. Is 121 a prime number? If not, find its factors.arrow_forward13. If a fair coin is flipped, what is the probability of getting heads? 14. A bag contains 3 red balls and 2 blue balls. If one ball is picked at random, what is the probability of picking a red ball?arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Elementary AlgebraAlgebraISBN:9780998625713Author:Lynn Marecek, MaryAnne Anthony-SmithPublisher:OpenStax - Rice University
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Elementary Algebra
Algebra
ISBN:9780998625713
Author:Lynn Marecek, MaryAnne Anthony-Smith
Publisher:OpenStax - Rice University
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Sequences and Series Introduction; Author: Mario's Math Tutoring;https://www.youtube.com/watch?v=m5Yn4BdpOV0;License: Standard YouTube License, CC-BY
Introduction to sequences; Author: Dr. Trefor Bazett;https://www.youtube.com/watch?v=VG9ft4_dK24;License: Standard YouTube License, CC-BY