
Single Variable Calculus: Early Transcendentals (2nd Edition) - Standalone book
2nd Edition
ISBN: 9780321954237
Author: William L. Briggs, Lyle Cochran, Bernard Gillett
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Textbook Question
Chapter 7.9, Problem 45E
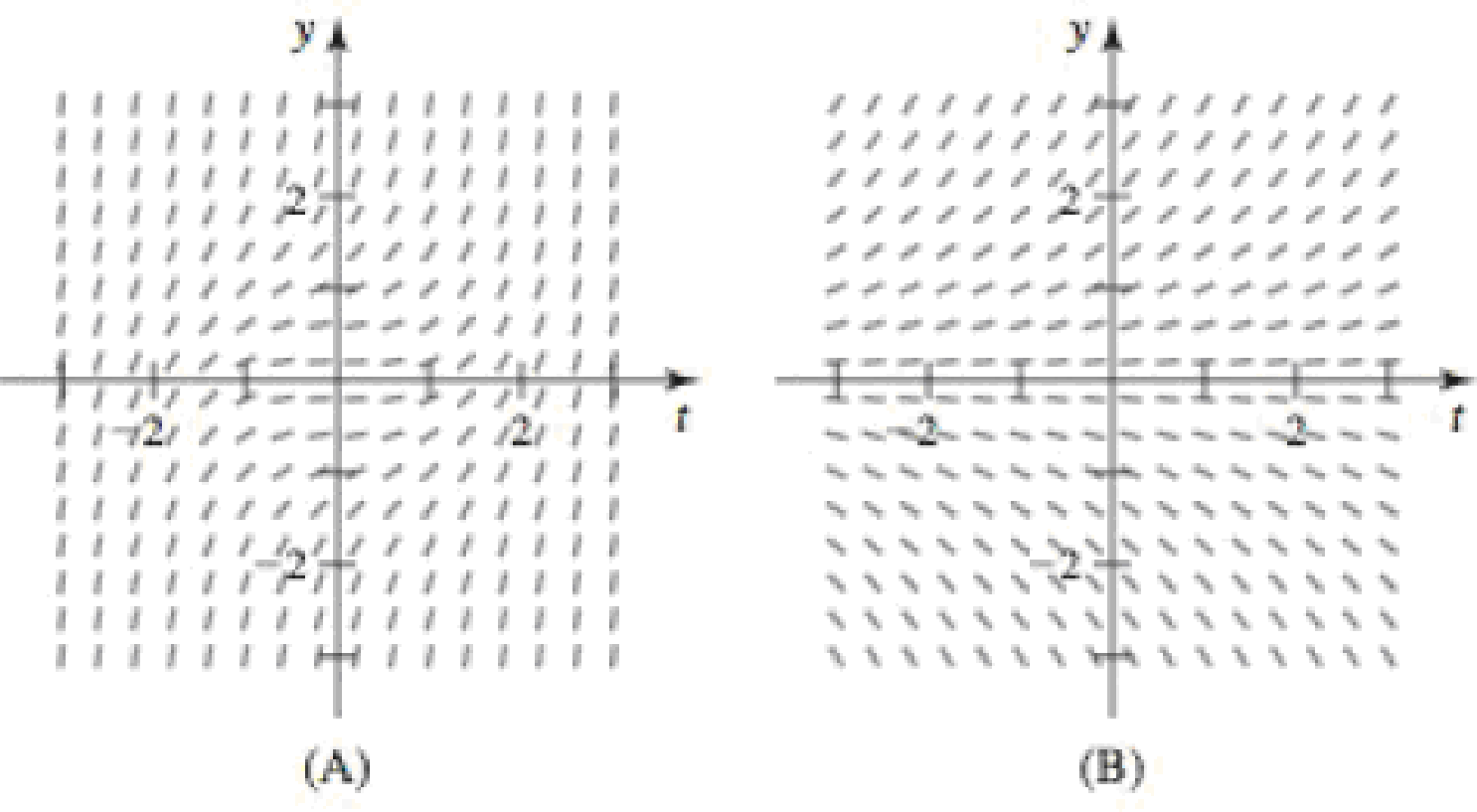
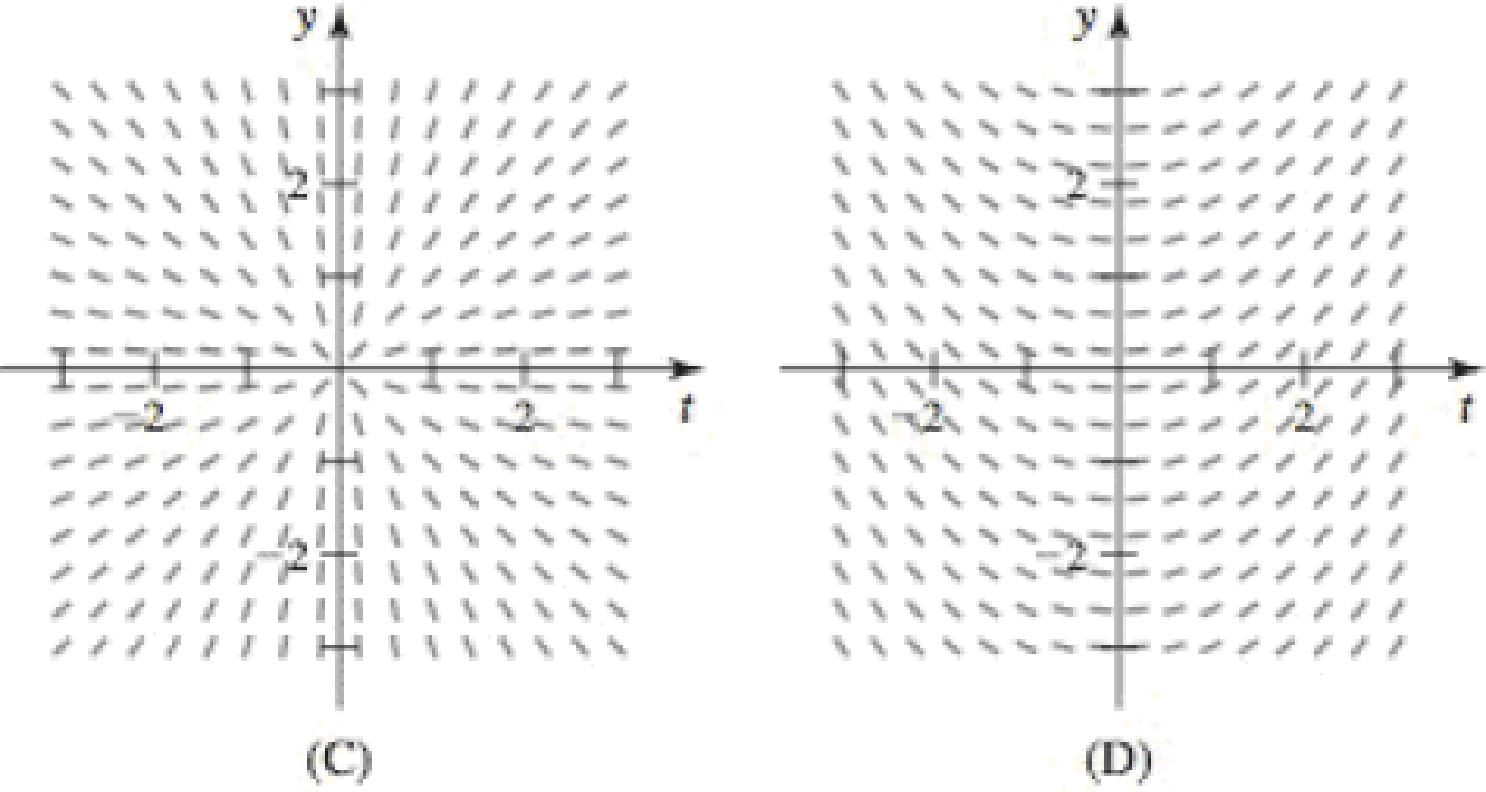
Matching direction fields Match equations a–d with the direction fields A–D.
- a. y′(t) = t/2
- b. y′(t) = y/2
- c. y′(t) = (t2 + y2)/2
- d. y′(t) = y/t
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
4. Consider the initial value problem
y' = 3x(y-1) 1/3,
y(xo) = yo.
(a) For what points (co, yo) does the IVP have a solution?
(b) For what points (xo, yo) does the IVP have a unique solution on some open interval that contains 20?
(c) Solve the IVP
y' = 3x(y-1) 1/3,
y(0) = 9
and determine the largest open interval on which this solution is unique.
Find the limit. (If the limit is infinite, enter 'oo' or '-o', as appropriate. If the limit does not otherwise exist, enter DNE.)
lim
X→ ∞
(✓
81x2
-
81x + x
9x)
2) Compute the following anti-derivative.
√1x4 dx
Chapter 7 Solutions
Single Variable Calculus: Early Transcendentals (2nd Edition) - Standalone book
Ch. 7.1 - Prob. 1QCCh. 7.1 - Prob. 2QCCh. 7.1 - Prob. 3QCCh. 7.1 - Prob. 4QCCh. 7.1 - What change of variables would you use for the...Ch. 7.1 - Prob. 2ECh. 7.1 - What trigonometric identity is useful in...Ch. 7.1 - Describe a first step in integrating x32x+4x1dx.Ch. 7.1 - Prob. 5ECh. 7.1 - Prob. 6E
Ch. 7.1 - Substitution Review Evaluate the following...Ch. 7.1 - Substitution Review Evaluate the following...Ch. 7.1 - Substitution Review Evaluate the following...Ch. 7.1 - Substitution Review Evaluate the following...Ch. 7.1 - Substitution Review Evaluate the following...Ch. 7.1 - Substitution Review Evaluate the following...Ch. 7.1 - Substitution Review Evaluate the following...Ch. 7.1 - Substitution Review Evaluate the following...Ch. 7.1 - Subtle substitutions Evaluate the following...Ch. 7.1 - Subtle substitutions Evaluate the following...Ch. 7.1 - Subtle substitutions Evaluate the following...Ch. 7.1 - Prob. 18ECh. 7.1 - Subtle substitutions Evaluate the following...Ch. 7.1 - Subtle substitutions Evaluate the following...Ch. 7.1 - Subtle substitutions Evaluate the following...Ch. 7.1 - Prob. 22ECh. 7.1 - Splitting fractions Evaluate the following...Ch. 7.1 - Splitting fractions Evaluate the following...Ch. 7.1 - Splitting fractions Evaluate the following...Ch. 7.1 - Splitting fractions Evaluate the following...Ch. 7.1 - Splitting fractions Evaluate the following...Ch. 7.1 - Splitting fractions Evaluate the following...Ch. 7.1 - Division with rational functions Evaluate the...Ch. 7.1 - Division with rational functions Evaluate the...Ch. 7.1 - Division with rational functions Evaluate the...Ch. 7.1 - Prob. 32ECh. 7.1 - Completing the square Evaluate the following...Ch. 7.1 - Completing the square Evaluate the following...Ch. 7.1 - Completing the square Evaluate the following...Ch. 7.1 - Completing the square Evaluate the following...Ch. 7.1 - Multiply by 1 Evaluate the following integrals....Ch. 7.1 - Multiply by 1 Evaluate the following integrals....Ch. 7.1 - Multiply by 1 Evaluate the following integrals....Ch. 7.1 - Multiply by 1 Evaluate the following integrals....Ch. 7.1 - Further Explorations 41. Explain why or why not...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Prob. 52ECh. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Miscellaneous integrals Use the approaches...Ch. 7.1 - Different substitutions a. Evaluate tanxsec2xdx...Ch. 7.1 - Different methods a. Evaluate cotxcsc2xdx using...Ch. 7.1 - Different methods a. Evaluate x2x+1dx using the...Ch. 7.1 - Different substitutions a. Show that...Ch. 7.1 - Area of a region between curves Find the area of...Ch. 7.1 - Area of a region between curves Find the area of...Ch. 7.1 - Prob. 61ECh. 7.1 - Prob. 62ECh. 7.1 - Arc length Find the length of the curve y = x5/4...Ch. 7.1 - Surface area Find the area of the surface...Ch. 7.1 - Surface area Let f(x)=x+1. Find the area of the...Ch. 7.1 - Skydiving A skydiver in free fall subject to...Ch. 7.2 - Prob. 1QCCh. 7.2 - Prob. 2QCCh. 7.2 - Prob. 3QCCh. 7.2 - On which derivative rule is integration by parts...Ch. 7.2 - How would you choose dv when evaluating xneaxdx...Ch. 7.2 - Prob. 3ECh. 7.2 - Explain how integration by parts is used to...Ch. 7.2 - Prob. 5ECh. 7.2 - Prob. 6ECh. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Prob. 20ECh. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Integration by parts Evaluate the following...Ch. 7.2 - Repeated integration by parts Evaluate the...Ch. 7.2 - Repeated integration by parts Evaluate the...Ch. 7.2 - Repeated integration by parts Evaluate the...Ch. 7.2 - Repeated integration by parts Evaluate the...Ch. 7.2 - Repeated integration by parts Evaluate the...Ch. 7.2 - Repeated integration by parts Evaluate the...Ch. 7.2 - Repeated integration by parts Evaluate the...Ch. 7.2 - Repeated integration by parts Evaluate the...Ch. 7.2 - Definite integrals Evaluate the following definite...Ch. 7.2 - Definite integrals Evaluate the following definite...Ch. 7.2 - Definite integrals Evaluate the following definite...Ch. 7.2 - Definite integrals Evaluate the following definite...Ch. 7.2 - Definite integrals Evaluate the following definite...Ch. 7.2 - Definite integrals Evaluate the following definite...Ch. 7.2 - Definite integrals Evaluate the following definite...Ch. 7.2 - Prob. 38ECh. 7.2 - Volumes of solids Find the volume of the solid...Ch. 7.2 - Volumes of solids Find the volume of the solid...Ch. 7.2 - Volumes of solids Find the volume of the solid...Ch. 7.2 - Volumes of solids Find the volume of the solid...Ch. 7.2 - Reduction formulas Use integration by parts to...Ch. 7.2 - Reduction formulas Use integration by parts to...Ch. 7.2 - Reduction formulas Use integration by parts to...Ch. 7.2 - Reduction formulas Use integration by parts to...Ch. 7.2 - Prob. 48ECh. 7.2 - Prob. 49ECh. 7.2 - Prob. 50ECh. 7.2 - Prob. 51ECh. 7.2 - Integrals involving lnxdx Use a substitution to...Ch. 7.2 - Integrals involving lnxdx Use a substitution to...Ch. 7.2 - Two methods a. Evaluate xlnx2dx using the...Ch. 7.2 - Logarithm base b Prove that logbxdx=1lnb(xlnxx)+C.Ch. 7.2 - Two integration methods Evaluate sinxcosxdx using...Ch. 7.2 - Combining two integration methods Evaluate cosxdx...Ch. 7.2 - Prob. 58ECh. 7.2 - Function defined as an integral Find the arc...Ch. 7.2 - A family of exponentials The curves y = xeax are...Ch. 7.2 - Solid of revolution Find the volume of the solid...Ch. 7.2 - Prob. 62ECh. 7.2 - Comparing volumes Let R be the region bounded by y...Ch. 7.2 - Log integrals Use integration by parts to show...Ch. 7.2 - A useful integral a. Use integration by parts to...Ch. 7.2 - Integrating inverse functions Assume that f has an...Ch. 7.2 - Integral of sec3 x Use integration by parts to...Ch. 7.2 - Two useful exponential integrals Use integration...Ch. 7.2 - Prob. 69ECh. 7.2 - Find the error Suppose you evaluate dxx using...Ch. 7.2 - Prob. 71ECh. 7.2 - Practice with tabular integration Evaluate the...Ch. 7.2 - Prob. 73ECh. 7.2 - Integrating derivatives Use integration by parts...Ch. 7.2 - An identity Show that if f has a continuous second...Ch. 7.2 - An identity Show that if f and g have continuous...Ch. 7.2 - Possible and impossible integrals Let In=xnex2dx,...Ch. 7.2 - Looking ahead (to Chapter 9) Suppose that a...Ch. 7.3 - Prob. 1QCCh. 7.3 - Prob. 2QCCh. 7.3 - State the half-angle identities used to integrate...Ch. 7.3 - State the three Pythagorean identities.Ch. 7.3 - Describe the method used to integrate sin3 x.Ch. 7.3 - Describe the method used to integrate sinm x cosn...Ch. 7.3 - What is a reduction formula?Ch. 7.3 - How would you evaluate cos2xsin3xdx?Ch. 7.3 - How would you evaluate tan10xsec2xdx?Ch. 7.3 - How would you evaluate sec12xtanxdx?Ch. 7.3 - Integrals of sin x or cos x Evaluate the following...Ch. 7.3 - Integrals of sin x or cos x Evaluate the following...Ch. 7.3 - Integrals of sin x or cos x Evaluate the following...Ch. 7.3 - Integrals of sin x or cos x Evaluate the following...Ch. 7.3 - Integrals of sin x or cos x Evaluate the following...Ch. 7.3 - Integrals of sin x or cos x Evaluate the following...Ch. 7.3 - Integrals of sin x and cos x Evaluate the...Ch. 7.3 - Integrals of sin x and cos x Evaluate the...Ch. 7.3 - Integrals of sin x and cos x Evaluate the...Ch. 7.3 - Integrals of sin x and cos x Evaluate the...Ch. 7.3 - Integrals of sin x and cos x Evaluate the...Ch. 7.3 - Integrals of sin x and cos x Evaluate the...Ch. 7.3 - Prob. 21ECh. 7.3 - Integrals of sin x and cos x Evaluate the...Ch. 7.3 - Integrals of sin x and cos x Evaluate the...Ch. 7.3 - Integrals of sin x and cos x Evaluate the...Ch. 7.3 - Integrals of tan x or cot x Evaluate the following...Ch. 7.3 - Integrals of tan x or cot x Evaluate the following...Ch. 7.3 - Integrals of tan x or cot x Evaluate the following...Ch. 7.3 - Integrals of tan x or cot x Evaluate the following...Ch. 7.3 - Integrals of tan x or cot x Evaluate the following...Ch. 7.3 - Integrals of tan x or cot x Evaluate the following...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Integrals involving tan x and sec x Evaluate the...Ch. 7.3 - Explain why or why not Determine whether the...Ch. 7.3 - Prob. 46ECh. 7.3 - Prob. 47ECh. 7.3 - Prob. 48ECh. 7.3 - Prob. 49ECh. 7.3 - Additional integrals Evaluate the following...Ch. 7.3 - Additional integrals Evaluate the following...Ch. 7.3 - Prob. 52ECh. 7.3 - Additional integrals Evaluate the following...Ch. 7.3 - Prob. 54ECh. 7.3 - Additional integrals Evaluate the following...Ch. 7.3 - Prob. 56ECh. 7.3 - Additional integrals Evaluate the following...Ch. 7.3 - Prob. 58ECh. 7.3 - Square roots Evaluate the following integrals. 59....Ch. 7.3 - Square roots Evaluate the following integrals. 60....Ch. 7.3 - Square roots Evaluate the following integrals. 61....Ch. 7.3 - Sine football Find the volume of the solid...Ch. 7.3 - Arc length Find the length of the curve y = ln...Ch. 7.3 - Prob. 64ECh. 7.3 - A tangent reduction formula Prove that for...Ch. 7.3 - A secant reduction formula Prove that for positive...Ch. 7.3 - Integrals of the form sinmxcosnxdx Use the...Ch. 7.3 - Integrals of the form sinmxcosnxdx Use the...Ch. 7.3 - Integrals of the form sinmxcosnxdx Use the...Ch. 7.3 - Integrals of the form sinmxcosnxdx Use the...Ch. 7.3 - Integrals of the form sinmxcosnxdx Use the...Ch. 7.3 - Mercator map projection The Mercator map...Ch. 7.3 - Prob. 73ECh. 7.4 - Prob. 1QCCh. 7.4 - Prob. 2QCCh. 7.4 - Prob. 3QCCh. 7.4 - What change of variables is suggested by an...Ch. 7.4 - What change of variables is suggested by an...Ch. 7.4 - What change of variables is suggested by an...Ch. 7.4 - If x = 4 tan , express sin in terms of x.Ch. 7.4 - If x = 2 sin , express cot in terms of x.Ch. 7.4 - If x = 8 sec , express tan in terms of x.Ch. 7.4 - Sine substitution Evaluate the following...Ch. 7.4 - Sine substitution Evaluate the following...Ch. 7.4 - Sine substitution Evaluate the following...Ch. 7.4 - Sine substitution Evaluate the following...Ch. 7.4 - Sine substitution Evaluate the following...Ch. 7.4 - Sine substitution Evaluate the following...Ch. 7.4 - Sine substitution Evaluate the following...Ch. 7.4 - Sine substitution Evaluate the following...Ch. 7.4 - Prob. 15ECh. 7.4 - Sine substitution Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Prob. 19ECh. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Prob. 23ECh. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Prob. 26ECh. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Prob. 30ECh. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Prob. 34ECh. 7.4 - Prob. 35ECh. 7.4 - Prob. 36ECh. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Prob. 41ECh. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Trigonometric substitutions Evaluate the following...Ch. 7.4 - Prob. 46ECh. 7.4 - Prob. 47ECh. 7.4 - Evaluating definite integrals Evaluate the...Ch. 7.4 - Evaluating definite integrals Evaluate the...Ch. 7.4 - Evaluating definite integrals Evaluate the...Ch. 7.4 - Evaluating definite integrals Evaluate the...Ch. 7.4 - Evaluating definite integrals Evaluate the...Ch. 7.4 - Prob. 53ECh. 7.4 - Evaluating definite integrals Evaluate the...Ch. 7.4 - Evaluating definite integrals Evaluate the...Ch. 7.4 - Prob. 56ECh. 7.4 - Explain why or why not Determine whether the...Ch. 7.4 - Completing the square Evaluate the following...Ch. 7.4 - Completing the square Evaluate the following...Ch. 7.4 - Completing the square Evaluate the following...Ch. 7.4 - Completing the square Evaluate the following...Ch. 7.4 - Prob. 62ECh. 7.4 - Completing the square Evaluate the following...Ch. 7.4 - Completing the square Evaluate the following...Ch. 7.4 - Completing the square Evaluate the following...Ch. 7.4 - Area of an ellipse The upper half of the ellipse...Ch. 7.4 - Area of a segment of a circle Use two approaches...Ch. 7.4 - Area of a lune A lune is a crescent-shaped region...Ch. 7.4 - Area and volume Consider the function f(x) = (9 +...Ch. 7.4 - Prob. 70ECh. 7.4 - Arc length of a parabola Find the length of the...Ch. 7.4 - Prob. 72ECh. 7.4 - Using the integral of sec3 u By reduction formula...Ch. 7.4 - Using the integral of sec3 u By reduction formula...Ch. 7.4 - Prob. 75ECh. 7.4 - Asymmetric integrands Evaluate the following...Ch. 7.4 - Asymmetric integrands Evaluate the following...Ch. 7.4 - Prob. 78ECh. 7.4 - Prob. 79ECh. 7.4 - Prob. 80ECh. 7.4 - Prob. 81ECh. 7.4 - Magnetic field due to current in a straight wire A...Ch. 7.4 - Prob. 83ECh. 7.4 - Show that...Ch. 7.4 - Evaluate for x21x3dx, for x 1 and for x 1.Ch. 7.4 - Prob. 87ECh. 7.4 - Prob. 88ECh. 7.4 - Prob. 89ECh. 7.5 - Prob. 1QCCh. 7.5 - Prob. 2QCCh. 7.5 - Prob. 3QCCh. 7.5 - Prob. 4QCCh. 7.5 - What kinds of functions can be integrated using...Ch. 7.5 - Give an example of each of the following. a. A...Ch. 7.5 - What term(s) should appear in the partial fraction...Ch. 7.5 - Prob. 4ECh. 7.5 - Prob. 5ECh. 7.5 - Setting up partial fraction decomposition Give the...Ch. 7.5 - Setting up partial fraction decomposition Give the...Ch. 7.5 - Setting up partial fraction decomposition Give the...Ch. 7.5 - Setting up partial fraction decomposition Give the...Ch. 7.5 - Setting up partial fraction decomposition Give the...Ch. 7.5 - Setting up partial fraction decomposition Give the...Ch. 7.5 - Setting up partial fraction decomposition Give the...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Simple linear factors Evaluate the following...Ch. 7.5 - Repeated linear factors Evaluate the following...Ch. 7.5 - Repeated linear factors Evaluate the following...Ch. 7.5 - Repeated linear factors Evaluate the following...Ch. 7.5 - Repeated linear factors Evaluate the following...Ch. 7.5 - Repeated linear factors Evaluate the following...Ch. 7.5 - Repeated linear factors Evaluate the following...Ch. 7.5 - Repeated linear factors Evaluate the following...Ch. 7.5 - Repeated linear factors Evaluate the following...Ch. 7.5 - Repeated linear factors Evaluate the following...Ch. 7.5 - Prob. 36ECh. 7.5 - Repeated linear factors Evaluate the following...Ch. 7.5 - Prob. 38ECh. 7.5 - Setting up partial fraction decompositions Give...Ch. 7.5 - Prob. 40ECh. 7.5 - Setting up partial fraction decompositions Give...Ch. 7.5 - Prob. 42ECh. 7.5 - Simple irreducible quadratic factors Evaluate the...Ch. 7.5 - Simple irreducible quadratic factors Evaluate the...Ch. 7.5 - Simple irreducible quadratic factors Evaluate the...Ch. 7.5 - Simple irreducible quadratic factors Evaluate the...Ch. 7.5 - Simple irreducible quadratic factors Evaluate the...Ch. 7.5 - Prob. 48ECh. 7.5 - Prob. 49ECh. 7.5 - Simple irreducible quadratic factors Evaluate the...Ch. 7.5 - Explain why or why not Determine whether the...Ch. 7.5 - Prob. 52ECh. 7.5 - Areas of regions Find the area of the following...Ch. 7.5 - Prob. 54ECh. 7.5 - Prob. 55ECh. 7.5 - Prob. 56ECh. 7.5 - Volumes of solids Find the volume of the following...Ch. 7.5 - Prob. 58ECh. 7.5 - Volumes of solids Find the volume of the following...Ch. 7.5 - Prob. 60ECh. 7.5 - Prob. 61ECh. 7.5 - Whats wrong? Why are there no constants A and B...Ch. 7.5 - Preliminary steps The following integrals require...Ch. 7.5 - Preliminary steps The following integrals require...Ch. 7.5 - Prob. 65ECh. 7.5 - Prob. 66ECh. 7.5 - Prob. 67ECh. 7.5 - Prob. 68ECh. 7.5 - Preliminary steps The following integrals require...Ch. 7.5 - Preliminary steps The following integrals require...Ch. 7.5 - Preliminary steps The following integrals require...Ch. 7.5 - Preliminary steps The following integrals require...Ch. 7.5 - Prob. 73ECh. 7.5 - Preliminary steps The following integrals require...Ch. 7.5 - Prob. 75ECh. 7.5 - Prob. 76ECh. 7.5 - Prob. 77ECh. 7.5 - Prob. 78ECh. 7.5 - Prob. 79ECh. 7.5 - Fractional powers Use the indicated substitution...Ch. 7.5 - Prob. 81ECh. 7.5 - Prob. 82ECh. 7.5 - Repeated quadratic factors Refer to the summary...Ch. 7.5 - Repeated quadratic factors Refer to the summary...Ch. 7.5 - Prob. 85ECh. 7.5 - Prob. 86ECh. 7.5 - Two methods Evaluate dxx21, for x l, in two ways;...Ch. 7.5 - Rational functions of trigonometric functions An...Ch. 7.5 - Prob. 89ECh. 7.5 - Rational functions of trigonometric functions An...Ch. 7.5 - Rational functions of trigonometric functions An...Ch. 7.5 - Prob. 92ECh. 7.5 - Prob. 93ECh. 7.5 - Prob. 94ECh. 7.5 - Three start-ups Three cars. A, B, and C, start...Ch. 7.5 - Prob. 96ECh. 7.5 - Prob. 97ECh. 7.5 - Prob. 98ECh. 7.6 - Prob. 1QCCh. 7.6 - Prob. 2QCCh. 7.6 - Prob. 3QCCh. 7.6 - Give some examples of analytical methods for...Ch. 7.6 - Prob. 2ECh. 7.6 - Prob. 3ECh. 7.6 - Is a reduction formula an analytical method or a...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Prob. 18ECh. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Table lookup integrals Use a table of integrals to...Ch. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Prob. 26ECh. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Prob. 28ECh. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Prob. 30ECh. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Preliminary work Use a table of integrals to...Ch. 7.6 - Geometry problems Use a table of integrals to...Ch. 7.6 - Prob. 40ECh. 7.6 - Prob. 41ECh. 7.6 - Geometry problems Use a table of integrals to...Ch. 7.6 - Prob. 43ECh. 7.6 - Geometry problems Use a table of integrals to...Ch. 7.6 - Prob. 45ECh. 7.6 - Geometry problems Use a table of integrals to...Ch. 7.6 - Apparent discrepancy Resolve the apparent...Ch. 7.6 - Reduction formulas Use the reduction formulas in a...Ch. 7.6 - Reduction formulas Use the reduction formulas in a...Ch. 7.6 - Reduction formulas Use the reduction formulas in a...Ch. 7.6 - Reduction formulas Use the reduction formulas in a...Ch. 7.6 - Evaluating an integral without the Fundamental...Ch. 7.6 - Two integration approaches Evaluate cos(lnx)dx two...Ch. 7.6 - Arc length of a parabola Let L(c) be the length of...Ch. 7.6 - Deriving formulas Evaluate the following...Ch. 7.6 - Deriving formulas Evaluate the following...Ch. 7.6 - Deriving formulas Evaluate the following...Ch. 7.6 - Deriving formulas Evaluate the following...Ch. 7.7 - Prob. 1QCCh. 7.7 - Prob. 2QCCh. 7.7 - Prob. 3QCCh. 7.7 - Prob. 4QCCh. 7.7 - If the interval [4, 18] is partitioned into n = 28...Ch. 7.7 - Explain geometrically how the Midpoint Rule is...Ch. 7.7 - Prob. 3ECh. 7.7 - If the Midpoint Rule is used on the interval [1,...Ch. 7.7 - If the Trapezoid Rule is used on the interval [1,...Ch. 7.7 - Prob. 6ECh. 7.7 - Absolute and relative error Compute the absolute...Ch. 7.7 - Absolute and relative error Compute the absolute...Ch. 7.7 - Midpoint Rule approximations Find the indicated...Ch. 7.7 - Midpoint Rule approximations Find the indicated...Ch. 7.7 - Midpoint Rule approximations Find the indicated...Ch. 7.7 - Midpoint Rule approximations Find the indicated...Ch. 7.7 - Trapezoid Rule approximations Find the indicated...Ch. 7.7 - Prob. 16ECh. 7.7 - Trapezoid Rule approximations Find the indicated...Ch. 7.7 - Trapezoid Rule approximations Find the indicated...Ch. 7.7 - Midpoint Rule, Trapezoid Rule, and relative error...Ch. 7.7 - Midpoint Rule, Trapezoid Rule, and relative error...Ch. 7.7 - Comparing the Midpoint and Trapezoid Rules Apply...Ch. 7.7 - Comparing the Midpoint and Trapezoid Rules Apply...Ch. 7.7 - Prob. 23ECh. 7.7 - Prob. 24ECh. 7.7 - Prob. 25ECh. 7.7 - Comparing the Midpoint and Trapezoid Rules Apply...Ch. 7.7 - Temperature data Hourly temperature data for...Ch. 7.7 - Temperature data Hourly temperature data for...Ch. 7.7 - Temperature data Hourly temperature data for...Ch. 7.7 - Temperature data Hourly temperature data for...Ch. 7.7 - Nonuniform grids Use the indicated methods to...Ch. 7.7 - Nonuniform grids Use the indicated methods to...Ch. 7.7 - Nonuniform grids Use the indicated methods to...Ch. 7.7 - Nonuniform grids Use the indicated methods to...Ch. 7.7 - Trapezoid Rule and Simpsons Rule Consider the...Ch. 7.7 - Trapezoid Rule and Simpsons Rule Consider the...Ch. 7.7 - Trapezoid Rule and Simpsons Rule Consider the...Ch. 7.7 - Prob. 38ECh. 7.7 - Simpsons Rule Apply Simpsons Rule to the following...Ch. 7.7 - Prob. 40ECh. 7.7 - Simpsons Rule Apply Simpsons Rule to the following...Ch. 7.7 - Prob. 42ECh. 7.7 - Explain why or why not Determine whether the...Ch. 7.7 - Comparing the Midpoint and Trapezoid Rules Compare...Ch. 7.7 - Comparing the Midpoint and Trapezoid Rules Compare...Ch. 7.7 - Prob. 46ECh. 7.7 - Prob. 47ECh. 7.7 - Prob. 48ECh. 7.7 - Prob. 49ECh. 7.7 - Using Simpsons Rule Approximate the following...Ch. 7.7 - Prob. 51ECh. 7.7 - Period of a pendulum A standard pendulum of length...Ch. 7.7 - Prob. 53ECh. 7.7 - Prob. 54ECh. 7.7 - Normal distribution of heights The heights of U.S....Ch. 7.7 - Prob. 56ECh. 7.7 - U.S. oil produced and imported The figure shows...Ch. 7.7 - Estimating error Refer to Theorem 7.2 and let...Ch. 7.7 - Estimating error Refer to Theorem 7.2 and let f(x)...Ch. 7.7 - Exact Trapezoid Rule Prove that the Trapezoid Rule...Ch. 7.7 - Prob. 61ECh. 7.7 - Shortcut for the Trapezoid Rule Given a Midpoint...Ch. 7.7 - Prob. 63ECh. 7.7 - Shortcut for Simpsons Rule Using the notation of...Ch. 7.7 - Another Simpsons Rule formula Another Simpsons...Ch. 7.8 - Prob. 1QCCh. 7.8 - Prob. 2QCCh. 7.8 - Prob. 3QCCh. 7.8 - What are the two general ways in which an improper...Ch. 7.8 - Explain how to evaluate af(x)dx.Ch. 7.8 - Prob. 3ECh. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Prob. 16ECh. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Prob. 20ECh. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Prob. 24ECh. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Infinite intervals of integration Evaluate the...Ch. 7.8 - Volumes on infinite intervals Find the volume of...Ch. 7.8 - Volumes on infinite intervals Find the volume of...Ch. 7.8 - Volumes on infinite intervals Find the volume of...Ch. 7.8 - Volumes on infinite intervals Find the volume of...Ch. 7.8 - Volumes on infinite intervals Find the volume of...Ch. 7.8 - Volumes on infinite intervals Find the volume of...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Prob. 36ECh. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Integrals with unbounded integrands Evaluate the...Ch. 7.8 - Volumes with infinite integrands Find the volume...Ch. 7.8 - Volumes with infinite integrands Find the volume...Ch. 7.8 - Volumes with infinite integrands Find the volume...Ch. 7.8 - Volumes with infinite integrands Find the volume...Ch. 7.8 - Volumes with infinite integrands Find the volume...Ch. 7.8 - Volumes with infinite integrands Find the volume...Ch. 7.8 - Bioavailability When a drug is given...Ch. 7.8 - Draining a pool Water is drained from a swimming...Ch. 7.8 - Maximum distance An object moves on a line with...Ch. 7.8 - Prob. 60ECh. 7.8 - Explain why or why not Determine whether the...Ch. 7.8 - Prob. 62ECh. 7.8 - Prob. 63ECh. 7.8 - Prob. 64ECh. 7.8 - Prob. 65ECh. 7.8 - Prob. 66ECh. 7.8 - Integration by parts Use integration by parts to...Ch. 7.8 - Prob. 68ECh. 7.8 - A close comparison Graph the integrands and then...Ch. 7.8 - Area between curves Let R be the region bounded by...Ch. 7.8 - Area between curves Let R be the region bounded by...Ch. 7.8 - An area function Let A(a) denote the area of the...Ch. 7.8 - Regions bounded by exponentials Let a 0 and let R...Ch. 7.8 - Prob. 74ECh. 7.8 - Prob. 75ECh. 7.8 - Prob. 76ECh. 7.8 - Prob. 77ECh. 7.8 - Prob. 78ECh. 7.8 - Prob. 79ECh. 7.8 - Prob. 80ECh. 7.8 - Perpetual annuity Imagine that today you deposit B...Ch. 7.8 - Draining a tank Water is drained from a 3000-gal...Ch. 7.8 - Decaying oscillations Let a 0 and b be real...Ch. 7.8 - Electronic chips Suppose the probability that a...Ch. 7.8 - Prob. 85ECh. 7.8 - The Eiffel Tower property Let R be the region...Ch. 7.8 - Escape velocity and black holes The work required...Ch. 7.8 - Adding a proton to a nucleus The nucleus of an...Ch. 7.8 - Prob. 89ECh. 7.8 - Laplace transforms A powerful tool in solving...Ch. 7.8 - Laplace transforms A powerful tool in solving...Ch. 7.8 - Laplace transforms A powerful tool in solving...Ch. 7.8 - Laplace transforms A powerful tool in solving...Ch. 7.8 - Laplace transforms A powerful tool in solving...Ch. 7.8 - Improper integrals Evaluate the following improper...Ch. 7.8 - A better way Compute 01lnxdx using integration by...Ch. 7.8 - Prob. 97ECh. 7.8 - Gamma function The gamma function is defined by...Ch. 7.8 - Many methods needed Show that 0xlnx(1+x)2dx= in...Ch. 7.8 - Prob. 100ECh. 7.8 - Prob. 101ECh. 7.8 - Prob. 102ECh. 7.9 - Prob. 1QCCh. 7.9 - Prob. 2QCCh. 7.9 - Prob. 3QCCh. 7.9 - Prob. 4QCCh. 7.9 - Prob. 1ECh. 7.9 - Is y(t) + 9y(t) = 10 linear or nonlinear?Ch. 7.9 - Prob. 3ECh. 7.9 - Prob. 4ECh. 7.9 - Prob. 5ECh. 7.9 - Prob. 6ECh. 7.9 - Prob. 7ECh. 7.9 - Prob. 8ECh. 7.9 - Verifying general solutions Verify that the given...Ch. 7.9 - Verifying general solutions Verify that the given...Ch. 7.9 - Verifying general solutions Verify that the given...Ch. 7.9 - Verifying general solutions Verify that the given...Ch. 7.9 - Prob. 13ECh. 7.9 - Prob. 14ECh. 7.9 - Prob. 15ECh. 7.9 - Prob. 16ECh. 7.9 - Prob. 17ECh. 7.9 - Prob. 18ECh. 7.9 - Prob. 19ECh. 7.9 - Prob. 20ECh. 7.9 - First-order linear equations Find the general...Ch. 7.9 - First-order linear equations Find the general...Ch. 7.9 - Prob. 23ECh. 7.9 - Prob. 24ECh. 7.9 - Initial value problems Solve the following...Ch. 7.9 - Initial value problems Solve the following...Ch. 7.9 - Initial value problems Solve the following...Ch. 7.9 - Prob. 28ECh. 7.9 - Prob. 29ECh. 7.9 - Prob. 30ECh. 7.9 - Separable differential equations Find the general...Ch. 7.9 - Separable differential equations Find the general...Ch. 7.9 - Separable differential equations Find the general...Ch. 7.9 - Separable differential equations Find the general...Ch. 7.9 - Separable differential equations Determine whether...Ch. 7.9 - Separable differential equations Determine whether...Ch. 7.9 - Separable differential equations Determine whether...Ch. 7.9 - Separable differential equations Determine whether...Ch. 7.9 - Separable differential equations Determine whether...Ch. 7.9 - Prob. 40ECh. 7.9 - Prob. 41ECh. 7.9 - Prob. 42ECh. 7.9 - Prob. 43ECh. 7.9 - Direction fields A differential equation and its...Ch. 7.9 - Matching direction fields Match equations ad with...Ch. 7.9 - Sketching direction fields Use the window [2, 2] ...Ch. 7.9 - Sketching direction fields Use the window [2, 2] ...Ch. 7.9 - Prob. 48ECh. 7.9 - Prob. 49ECh. 7.9 - Prob. 50ECh. 7.9 - Prob. 51ECh. 7.9 - Prob. 52ECh. 7.9 - Prob. 53ECh. 7.9 - Prob. 54ECh. 7.9 - Prob. 55ECh. 7.9 - Prob. 56ECh. 7.9 - Prob. 57ECh. 7.9 - Prob. 58ECh. 7.9 - Prob. 59ECh. 7.9 - Prob. 60ECh. 7.9 - Logistic equation for spread of rumors...Ch. 7.9 - Prob. 62ECh. 7.9 - Prob. 63ECh. 7.9 - Prob. 64ECh. 7.9 - Chemical rate equations The reaction of chemical...Ch. 7.9 - Prob. 66ECh. 7.9 - Prob. 67ECh. 7.9 - Prob. 68ECh. 7.9 - Prob. 69ECh. 7.9 - Prob. 70ECh. 7 - Explain why or why not Determine whether the...Ch. 7 - Basic integration techniques Use the methods...Ch. 7 - Basic integration techniques Use the methods...Ch. 7 - Basic integration techniques Use the methods...Ch. 7 - Basic integration techniques Use the methods...Ch. 7 - Basic integration techniques Use the methods...Ch. 7 - Basic integration techniques Use the methods...Ch. 7 - Integration by parts Use integration by parts to...Ch. 7 - Integration by parts Use integration by parts to...Ch. 7 - Prob. 10RECh. 7 - Prob. 11RECh. 7 - Trigonometric integrals Evaluate the following...Ch. 7 - Trigonometric integrals Evaluate the following...Ch. 7 - Prob. 14RECh. 7 - Trigonometric integrals Evaluate the following...Ch. 7 - Prob. 16RECh. 7 - Prob. 17RECh. 7 - Prob. 18RECh. 7 - Trigonometric substitutions Evaluate the following...Ch. 7 - Prob. 20RECh. 7 - Prob. 21RECh. 7 - Partial fractions Use partial fractions to...Ch. 7 - Partial fractions Use partial fractions to...Ch. 7 - Partial fractions Use partial fractions to...Ch. 7 - Partial fractions Use partial fractions to...Ch. 7 - Table of integrals Use a table of integrals to...Ch. 7 - Table of integrals Use a table of integrals to...Ch. 7 - Table of integrals Use a table of integrals to...Ch. 7 - Table of integrals Use a table of integrals to...Ch. 7 - Errors in numerical integration Let...Ch. 7 - Prob. 33RECh. 7 - Improper integrals Evaluate the following...Ch. 7 - Improper integrals Evaluate the following...Ch. 7 - Improper integrals Evaluate the following...Ch. 7 - Improper integrals Evaluate the following...Ch. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Prob. 43RECh. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Prob. 45RECh. 7 - Prob. 46RECh. 7 - Prob. 47RECh. 7 - Prob. 48RECh. 7 - Prob. 49RECh. 7 - Prob. 50RECh. 7 - Prob. 51RECh. 7 - Prob. 52RECh. 7 - Prob. 53RECh. 7 - Prob. 54RECh. 7 - Prob. 55RECh. 7 - Prob. 56RECh. 7 - Prob. 57RECh. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Miscellaneous Integrals Evaluate the following...Ch. 7 - Preliminary work Make a change of variables or use...Ch. 7 - Preliminary work Make a change of variables or use...Ch. 7 - Preliminary work Make a change of variables or use...Ch. 7 - Preliminary work Make a change of variables or use...Ch. 7 - Preliminary work Make a change of variables or use...Ch. 7 - Preliminary work Make a change of variables or use...Ch. 7 - Prob. 70RECh. 7 - Volumes The region R is bounded by the curve y =...Ch. 7 - Volumes The region R is bounded by the curve y =...Ch. 7 - Volumes The region R is bounded by the curve y =...Ch. 7 - Volumes The region R is bounded by the curve y =...Ch. 7 - Comparing volumes Let R be the region bounded by...Ch. 7 - Comparing areas Show that the area of the region...Ch. 7 - Zero log integral It is evident from the graph of...Ch. 7 - Arc length Find the length of the curve y = ln x...Ch. 7 - Average velocity Find the average velocity of a...Ch. 7 - Comparing distances Starting at the same time and...Ch. 7 - Traffic flow When data from a traffic study are...Ch. 7 - Comparing integrals Graph the functions f(x) = ...Ch. 7 - A family of logarithm integrals Let...Ch. 7 - Arc length Find the length of the curve...Ch. 7 - Best approximation Let I=01x2xlnxdx. Use any...Ch. 7 - Numerical integration Use a calculator to...Ch. 7 - Numerical integration Use a calculator to...Ch. 7 - Two worthy integrals a. Let I(a)=0dx(1+xa)(1+x2),...Ch. 7 - Comparing volumes Let R be the region bounded by y...Ch. 7 - Equal volumes a. Let R be the region bounded by...Ch. 7 - Equal volumes Let R1 be the region bounded by the...Ch. 7 - Prob. 92RECh. 7 - Prob. 93RECh. 7 - Prob. 94RECh. 7 - Prob. 95RECh. 7 - Prob. 96RECh. 7 - Prob. 97RECh. 7 - Prob. 98RECh. 7 - Prob. 99RECh. 7 - Prob. 100RECh. 7 - Prob. 101RECh. 7 - Prob. 102RE
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- Question 3 (5pt): A chemical reaction. In an elementary chemical reaction, single molecules of two reactants A and B form a molecule of the product C : ABC. The law of mass action states that the rate of reaction is proportional to the product of the concentrations of A and B: d[C] dt = k[A][B] (where k is a constant positive number). Thus, if the initial concentrations are [A] = = a moles/L and [B] = b moles/L we write x = [C], then we have (E): dx dt = k(ax)(b-x) 1 (a) Write the differential equation (E) with separate variables, i.e. of the form f(x)dx = g(t)dt. (b) Assume first that a b. Show that 1 1 1 1 = (a - x) (b - x) - a) a - x b - x b) (c) Find an antiderivative for the function f(x) = (a-x) (b-x) using the previous question. (d) Solve the differentiel equation (E), i.e. find x as a function of t. Use the fact that the initial concentration of C is 0. (e) Now assume that a = b. Find x(t) assuming that a = b. How does this expression for x(t) simplify if it is known that [C] =…arrow_forward3) Find the volume of the solid that lies inside both the sphere x² + y² + z² cylinder x²+y² = 1. = 4 and thearrow_forward1) Compute the following limit. lim x-0 2 cos(x) 2x² - x4arrow_forward
- y = f(x) b C The graph of y = f(x) is shown in the figure above. On which of the following intervals are dy > 0 and dx d²y dx2 <0? I. aarrow_forward3 2 1 y O a The graph of the function f is shown in the figure above. Which of the following statements about f is true? о limb f(x) = 2 Olima f(x) = 2 о lima f (x) = lim x →b f(x) → f (x) = 1 limb. lima f(x) does not existarrow_forwardQuestion 1 (1pt). The graph below shows the velocity (in m/s) of an electric autonomous vehicle moving along a straight track. At t = 0 the vehicle is at the charging station. 1 8 10 12 0 2 4 6 (a) How far is the vehicle from the charging station when t = 2, 4, 6, 8, 10, 12? (b) At what times is the vehicle farthest from the charging station? (c) What is the total distance traveled by the vehicle?arrow_forwardQuestion 2 (1pt). Evaluate the following (definite and indefinite) integrals (a) / (e² + ½) dx (b) S (3u 2)(u+1)du (c) [ cos³ (9) sin(9)do .3 (d) L³ (₂ + 1 dzarrow_forward= Question 4 (5pt): The Orchard Problem. Below is the graph y f(t) of the annual harvest (assumed continuous) in kg/year from my cranapple orchard t years after planting. The trees take about 25 years to get established, and from that point on, for the next 25 years, they give a fairly good yield. But after 50 years, age and disease are taking their toll, and the annual yield is falling off. 40 35 30 。 ៣៩ ថា8 8 8 8 6 25 20 15 10 y 5 0 0 5 10 15 20 25 30 35 40 45 50 55 60 The orchard problem is this: when should the orchard be cut down and re- planted, thus starting the cycle again? What you want to do is to maximize your average harvest per year over a full cycle. Of course there are costs to cutting the orchard down and replanting, but it turns out that we can ignore these. The first cost is the time it takes to cut the trees down and replant but we assume that this can effectively be done in a week, and the loss of time is negligible. Secondly there is the cost of the labour to cut…arrow_forwardnd ave a ction and ave an 48. The domain of f y=f'(x) x 1 2 (= x<0 x<0 = f(x) possible. Group Activity In Exercises 49 and 50, do the following. (a) Find the absolute extrema of f and where they occur. (b) Find any points of inflection. (c) Sketch a possible graph of f. 49. f is continuous on [0,3] and satisfies the following. X 0 1 2 3 f 0 2 0 -2 f' 3 0 does not exist -3 f" 0 -1 does not exist 0 ve tes where X 0 < x <1 1< x <2 2arrow_forwardNumerically estimate the value of limx→2+x3−83x−9, rounded correctly to one decimal place. In the provided table below, you must enter your answers rounded exactly to the correct number of decimals, based on the Numerical Conventions for MATH1044 (see lecture notes 1.3 Actions page 3). If there are more rows provided in the table than you need, enter NA for those output values in the table that should not be used. x→2+ x3−83x−9 2.1 2.01 2.001 2.0001 2.00001 2.000001arrow_forwardFind the general solution of the given differential equation. (1+x)dy/dx - xy = x +x2arrow_forwardEstimate the instantaneous rate of change of the function f(x) = 2x² - 3x − 4 at x = -2 using the average rate of change over successively smaller intervals.arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Basic Differentiation Rules For Derivatives; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=IvLpN1G1Ncg;License: Standard YouTube License, CC-BY
