
Concept explainers
Future scientists: Education professionals refer to science, technology, engineering and mathematics as the STEM disciplines. A recent ACT Condition and Career Readiness Report states that 47% of high school graduates have expressed interest in a STEM discipline. A random sample of 85 freshmen is selected.
- Is it appropriate to use the normal approximation to find the
probability that less than 45% of the freshmen in the sample have expressed interest in a STEM discipline? If so, find the probability. If not, explain why not. - A new sample of 150 freshmen is selected. Find the probability that less than of the freshmen in this sample have expressed interest in a STEM discipline.
- Find the probability that the proportion of freshmen in the sample of 150 who have expressed interest in a STEM discipline is between 0.40 and 0.45.
- Find the probability that more than 38% of the freshmen in the sample of 150 have expressed interest in a STEM discipline.
- Would it be unusual if less than 42% of the freshmen in the sample of 150 have expressed interest in a STEM discipline?
a.
To find:Whether it is appropriate to use the normal approximation to find the probability that less than 45% of freshmenhave expressed interest in a STEM discipline.
Answer to Problem 21E
It is possible to use the normal distribution. The probability that less than 45% of freshmen have expressed interest in a STEM discipline is 0.3557.
Explanation of Solution
Given information:Educational professionals refer to science, technology, engineering and mathematics as the STEM disciplines. A recent ACT Condition and Career Readiness Report states that 47% of high school graduates have expressed interest in a STEM discipline. A randomsample of 85 freshmen is selected.
Formula used:A random variable is normally distributed when
Where n is the number of sample and p is the success probability.
The mean is
The standard deviation is
Calculation:
47% of high school graduates have expressed interest in a STEM discipline and a random sample of 85 freshmen is selected. (Given)
Then
Therefore, it is possible to use thenormal distribution.
Let
The mean is
The standard deviation is
We need to find
The z-score is given by
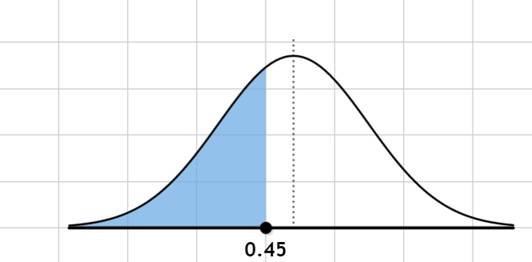
Therefore, from the standardize normal distribution table, the area to the left of
Hence, the probability that less than 45% of freshmen have expressed interest in a STEM discipline is 0.3557.
b.
To find:The probability that less than 45% of freshmen have expressed interest in a STEM discipline.
Answer to Problem 21E
The probability less than 45% of freshmen have expressed interest in a STEM discipline is 0.3085.
Explanation of Solution
Given information: Educational professionals refer to science, technology, engineering and mathematics as the STEM disciplines. A recent ACT Condition and Career Readiness Report states that 47% of high school graduates have expressed interest in a STEM discipline. A random sample of 150 freshmen is selected.
Formula used:
The mean is
The standard deviation is
The z-score is given by
Calculation:
Let
The mean is
The standard deviation is
We need to find
The z-score is given by
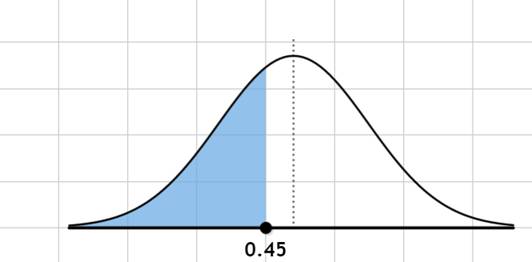
Therefore, from the standardize normal distribution table, the area to the left of
Hence, the probability that less than 45% of freshmen have expressed interest in a STEM discipline is 0.3085.
c.
To find: The probability that the sample proportion of the freshmen who have expressed interest in a STEM discipline is between 0.40 and 0.45.
Answer to Problem 21E
The probability that the sample proportion of freshmen who have expressed interest in a STEM discipline is between 0.40 and 0.45 is 0.2684.
Explanation of Solution
Given information:Educational professionals refer to science, technology, engineering and mathematics as the STEM disciplines. A recent ACT Condition and Career Readiness Report states that 47% of high school graduates have expressed interest in a STEM discipline. A random sample of 150 freshmen is selected.
Formula used:The z-score is given by
Calculation:
Let
The mean is
The standard deviation is
We need to find
The z-score is given by
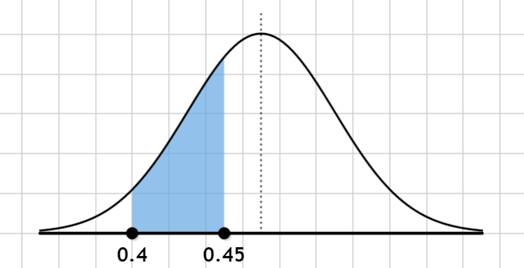
Therefore, from the standardize normal distribution table, the area to
Hence, the probability that the sample proportion of the freshmen who have expressed interest in a STEM discipline is between 0.40 and 0.45 is 0.2684.
d.
To find: The probability that more than 38% of freshmen in the sample of 150 have expressed interest in a STEM discipline.
Answer to Problem 21E
The probability that more than 38% of freshmen in the sample of 150 have expressed interest in a STEM discipline is 0.9878.
Explanation of Solution
Given information:Educational professionals refer to science, technology, engineering and mathematics as the STEM disciplines. A recent ACT Condition and Career Readiness Report states that 47% of high school graduates have expressed interest in a STEM discipline. A random sample of 150 freshmen is selected.
Formula used:The z-score is given by
Calculation:
Let
The mean is
The standard deviation is
We need to find
The z-score is given by
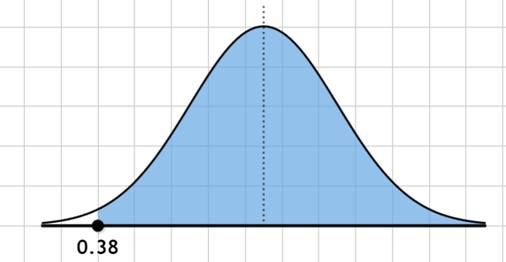
Therefore, from the standardize normal distribution table, the area to the right of
Hence, the probability that more than 38% of freshmen in the sample of 150 have expressed interest in a STEM discipline is 0.9878.
e.
To find: Whether it is unusual if less than 42% of the freshmen in the sample of 150have expressed interest in a STEM discipline.
Answer to Problem 21E
Less than 42% of the freshmen in the sample of 150have expressed interest in a STEM discipline is not unusual.
Explanation of Solution
Given information:Educational professionals refer to science, technology, engineering and mathematics as the STEM disciplines. A recent ACT Condition and Career Readiness Report states that 47% of high school graduates have expressed interest in a STEM discipline. A random sample of 150 freshmen is selected.
Formula used:The z-score is given by
Calculation:
Let
The mean is
The standard deviation is
We will compute the probability that sample proportion is less than 0.42. If the probability is less than 0.05, then the event is unusual.
We need to find
The z-score is given by
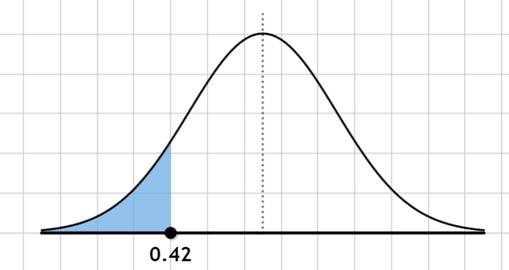
Therefore, from the standardize normal distribution table, the area to the right of
Thus, the probability that less than 42% of the freshmen in the sample of 150have expressed interest in a STEM discipline is 0.1056.
Since the probability is greater than 0.05, the given event isnot unusual.
Hence,less than 42% of the freshmen in the sample of 150have expressed interest in a STEM discipline is not unusual.
Want to see more full solutions like this?
Chapter 7 Solutions
ELEMENTARY STATISTICS-ALEKS ACCESS CODE
- 19. Let X be a non-negative random variable. Show that lim nE (IX >n)) = 0. E lim (x)-0. = >arrow_forward(c) Utilize Fubini's Theorem to demonstrate that E(X)= = (1- F(x))dx.arrow_forward(c) Describe the positive and negative parts of a random variable. How is the integral defined for a general random variable using these components?arrow_forward
- 26. (a) Provide an example where X, X but E(X,) does not converge to E(X).arrow_forward(b) Demonstrate that if X and Y are independent, then it follows that E(XY) E(X)E(Y);arrow_forward(d) Under what conditions do we say that a random variable X is integrable, specifically when (i) X is a non-negative random variable and (ii) when X is a general random variable?arrow_forward
 Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
 Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning



