Chapter 7.2, Problem 86E

To determine

To test: The comparison of means of the two samples using significance test of pooled methods.

Answer to Problem 86E

Solution: The data strongly suggests that there is no significant difference between the means of early eaters and late eaters in terms of consumption of fat.

Explanation of Solution

Given: The data on total fats for early eaters and late eaters are provided. The summary of statistics fats consumption by the two groups are provided as:

$\begin{array}{c}{\overline{x}}_1=23.1\\ {\overline{x}}_2=21.4\\ s_1=12.5\\ s_2=8.2\end{array}$

$\begin{array}{c}{n}_1=202\\ n_2=200\end{array}$

Explanation:

Calculation: The significance test is to compare the means of two groups in terms of consumption of fats. Hence, the null hypothesis is that the consumption of fats in the two groups is the same as against the alternative that the consumption of fats in the two groups is not same.

Therefore, the hypotheses are formulated as:

$\begin{array}{l}{H}_0:{\mu }_{\text{Early}}={\mu }_{\text{Late}}\\ H_a:{\mu }_{\text{Early}}\ne {\mu }_{\text{Late}}\end{array}$

In the above hypothesis, ${\mu }_{\text{Early}}$ represents the average fat consumption of early eaters and ${\mu }_{\text{Late}}$ represents the average fat consumption of late eaters.

The two-sample t- test statistic for pooled methods is defined as:

$t=\frac{\left({\overline{x}}_1-{\overline{x}}_2\right)-\left({\mu }_1-{\mu }_2\right)}{s_p\sqrt{\frac{1}{n_1}+\frac{1}{n_2}}}$

where,

$\begin{array}{c}{\overline{x}}_1=\text{Sample mean of the fat consumption by early eaters}\\ {\overline{x}}_2=\text{Sample mean of the fat consumption by late eaters}\\ s_1=\text{Sample standard deviation of fat consumption by early eaters}\\ s_2=\text{Sample standard deviation of fat consumption by late eaters}\end{array}$

$\begin{array}{c}{n}_1=\text{Sample size of fat consumption by early eaters}\\ n_2=\text{Sample size of fat consumption by late eaters}\\ {\mu }_1-{\mu }_2=\text{Difference of true population means}\\ s_p=\text{The pooled sample standard deviation}\end{array}$

First, determine the pooled sample standard deviation. The formula for pooled sample standard deviation is defined as:

$s_p=\sqrt{\frac{\left(n_1-1\right)s_1^2+\left(n_2-1\right)s_2^2}{n_1+n_2-2}}$

Substitute the provided values and determine the pooled sample variance:

$\begin{array}{c}{s}_p=\sqrt{\frac{\left[\left(202-1\right)\times \left({12.5}^2\right)\right]+\left[\left(200-1\right)\times \left({8.2}^2\right)\right]}{202+200-2}}\\ =\sqrt{\frac{44787.01}{400}}\\ =10.58\end{array}$

Now, determine the t- statistic. The difference of means is assumed as 0 in the null hypothesis. Substitute the provided values in the above-defined formula to compute the two sample t-statistic. So,

$\begin{array}{c}t=\frac{\left({\overline{x}}_1-{\overline{x}}_2\right)-\left({\mu }_1-{\mu }_2\right)}{s_p\sqrt{\frac{1}{n_1}+\frac{1}{n_2}}}\\ =\frac{\left(23.1-21.4\right)-0}{\left(10.58\right)\times \sqrt{\frac{1}{202}+\frac{1}{200}}}\\ =\frac{1.7}{1.06}\\ =1.60\end{array}$

The p-value for the provided two-sided test is calculated as $P\left(T\ge 1.60\right)$. For the pooled two-sample t-statistic, the degrees of freedom are $n_1+n_2-2$. So, the degrees of freedom are calculated as:

$\begin{array}{c}\text{Degrees of freedom}=n_1+n_2-2\\ =202+200-2\\ =400\end{array}$

So, the degrees of freedom are 400.

The Excel function to determine the p- value from t-test statistic is displayed in the screenshot below:

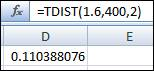
Conclusion: Therefore, the p-value is obtained as 0.1104, which is more than 0.05. So, do not reject the null hypothesis and hence it is concluded that the data strongly suggests that there is no significant difference between the means of the two groups in terms of fats consumption.

To determine

To find: A 95% confidence interval for the difference of means between early eaters and late eaters in terms of consumption of fats using pooled methods.

Answer to Problem 86E

Solution: A 95% confidence interval is $\underset{\_}{\left(-0.3747,3.7747\right)}$.

Explanation of Solution

Calculation: The confidence interval is calculated as:

$\text{Confidence interval}=\left({\overline{x}}_1-{\overline{x}}_2\right)\pm t^{*}\left(s_p\sqrt{\frac{1}{n_1}+\frac{1}{n_2}}\right)$

where,

$\begin{array}{c}{\overline{x}}_1=\text{Sample mean of the fat consumption by early eaters}\\ {\overline{x}}_2=\text{Sample mean of the fat consumption by late eaters}\\ s_1=\text{Sample standard deviation of fat consumption by early eaters}\\ s_2=\text{Sample standard deviation of fat consumption by late eaters}\end{array}$

$\begin{array}{c}{n}_1=\text{Sample size of fat consumption by early eaters}\\ n_2=\text{Sample size of fat consumption by late eaters}\\ {\mu }_1-{\mu }_2=\text{Difference of true population means}\\ s_p=\text{The pooled sample standard deviation}\end{array}$

The pooled sample variance is obtained as 10.58 in the previous part. The critical value of t for 95% confidence level and 400 degrees of freedom is 1.9659. Substitute the provided values in the above-defined formula to determine the 95% confidence interval for the difference between the mean consumption of fats of two groups. So,

$\begin{array}{c}\left({\overline{x}}_1-{\overline{x}}_2\right)\pm t^{*}\left(s_p\sqrt{\frac{1}{n_1}+\frac{1}{n_2}}\right)=\left(23.1-21.4\right)\pm \left[\left(1.9659\right)\times \left(10.58\right)\times \sqrt{\frac{1}{202}+\frac{1}{200}}\right]\\ =\left(1.7\pm 2.0747\right)\\ =\left(1.7-2.0747,1.7+2.0747\right)\\ =\left(-0.3747,3.7747\right)\end{array}$

Interpretation: Therefore, the 95% confidence interval for the difference between the means is obtained as $\left(-0.3747,3.7747\right)$.

To determine

To explain: The results obtained in the provided problem with those obtained in Exercise 7.71.

Answer to Problem 86E

Solution: The results are almost similar to those obtained in Exercise 7.71.

Explanation of Solution

The results of Exercise 7.71 are obtained as:

$\begin{array}{c}t=1.62\\ p-\text{value}=0.1081\end{array}$.

The results obtained in this provided problem are:

$\begin{array}{c}t=1.62\\ p-\text{value}=0.1104\end{array}$

Therefore, the results are almost similar.