Chapter 7, Problem 7.3P

Two identical chairs, each weighing 14 lb, are stacked as shown. The center of gravity of each chair is denoted by G. The coefficient of static friction is 0.2 at B (the contact point between the chairs) and 0.35 at A, C, and D. Determine the smallest force P that would cause sliding.

To determine

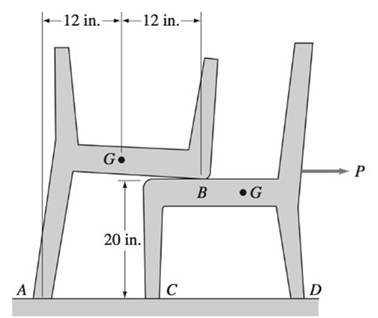
Smallest force 'P' that would cause sliding.

Answer to Problem 7.3P

Smallest force 'P' that would cause sliding is $9.52lb$.

Explanation of Solution

Given information:

Weight of each chair is $14lb$.

Co-efficient of static friction at point B is $0.2$.

Co-efficient of static friction at point A,C and D is $0.35$.

If there is no relative motion between two surfaces that are in contact, The relationship between normal force $N$ and friction force $F$ would be:

  $F={\mu }_{s}N$

  ${\mu }_s$ - Co-efficient of static friction

Steps to follow in the equilibrium analysis of a body are:

1. Draw the free body diagram.

2. Write the equilibrium equations.

3. Solve the equations for the unknowns.

Calculation:

Assume impending sliding at point B, C and D.

Assume $F_B,F_C,F_D$ as the friction force at point B, C and D.

Assume $N_B,N_C,N_D$ as the reaction force at point B, C and D.

  $\begin{array}{l}{F}_B=0.2N_B\\ F_C=0.35N_C\\ F_D=0.35N_D\end{array}$

FBD of left chair

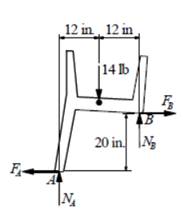
For the equilibrium of above section, the bending moment about point A is equal to zero.

  ${\displaystyle \sum M_A=0}$

  $\begin{array}{l}0.2N_B\left(20in\right)-N_B\left(24in\right)+\left(14lb\right)\left(12in\right)=0\\ N_B=8.4lb\end{array}$

FBD of right chair

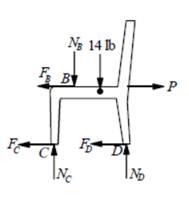
Write equilibrium equation in vertical direction.

  $\uparrow {\displaystyle \sum F_y}=0$

  $\begin{array}{l}{N}_C+N_D-N_B-14lb=0\\ N_C+N_D-8.4lb-14lb=0\\ N_C+N_D=22.4lb\end{array}$

Write equilibrium equation in horizontal direction.

  $\to {\displaystyle \sum F_x}=0$

  $\begin{array}{l}P-0.35\left(N_C+N_D\right)-0.2N_B=0\\ P-0.35\left(22.4lb\right)-0.2\left(8.4lb\right)=0\end{array}$

Solve

  $P=9.52lb$

Check for sliding at point A.

FBD of left chair

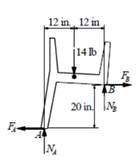
Write equilibrium equation in vertical direction.

  $\uparrow {\displaystyle \sum F_y}=0$

  $\begin{array}{l}{N}_A+N_B-14lb=0\\ N_A+8.4lb-14lb=0\\ N_A=5.6lb\end{array}$

Write equilibrium equation in horizontal direction.

  $\leftarrow {\displaystyle \sum F_x}=0$

  $\begin{array}{l}{F}_A-0.2N_B=0\\ F_A=0.2\left(8.4lb\right)\\ F_A=1.68lb\end{array}$

Coefficient friction ${\mu }_A$ at point A

  ${\mu }_A=\frac{F_A}{N_A}=\frac{1.68lb}{5.6lb}=0.3<{\left({\mu }_A\right)}_S$

Conclusion:

Smallest force 'P' that would cause sliding is $9.52lb$.