Finite Mathematics for Business, Economics, Life Sciences and Social Sciences

14th Edition

ISBN: 9780134677972

Author: Barnett

Publisher: PEARSON

See similar textbooks

Videos

Textbook Question

Chapter 6.4, Problem 43E

In Problems 39-47, construct a mathematical model in the form of a linear programming problem. Do not solve.

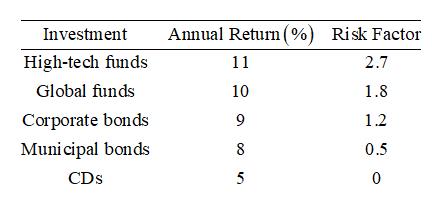
Investment strategy. An investor is planning to divide her investments among high-tech mutual funds, global mutual funds, corporate bonds, municipal bonds, and CDs. Each of these investments has an estimated annual return and a risk factor (see the table). The risk level for each choice is the product of its risk factor and the percentage of the total funds invested in that choice. The total risk level is the sum of the risk levels for all the investments. The investor wants at least 20 % of her investments to be in CDs and does not want the risk level to exceed 1.8 . What percentage of her total investments should be invested in each choice to maximize the return?

Chapter 6.4, Problem 43E, In Problems 39-47, construct a mathematical model in the form of a linear programming problem. Do

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 6.4, Problem 42E

Chapter 6.4, Problem 44E

Students have asked these similar questions

First Securities, Inc., an investment firm, has $380,000 on account. The chief investment officer would like to reinvest the $380,000 in a portfolio that would maximize return on investment while at the same time maintaining a relatively conservative mix of stocks and bonds. The following table shows the investment opportunities and rates of return. Investment Opportunity Rate of Return Municipal Bonds (X1) 0.095 High Tech Stock (X2) 0.146 Blue Chip Stock (X3) 0.075 Federal Bonds (X4) 0.070 a) The Board of Directors has mandated that at least 60 percent of the investment consist of a combination of municipal and federal bonds, at least 25 percent Blue Chip Stock, no more than 30 percent High Tech and Blue Chip Stock, and no more than 15 percent High Tech Stock. Based on above problem, how many constraints are in this problem? A) 3 B) 4 C) 5 D) 2 b) Based on part a, which of the…

Investments: Financial Stocks At the end of September 2021, Toronto Dominion Bank (TD) stock cost $66 per share, was expected to yield 4% per year in dividends (calculated on the total value of TD stock you bought), and had a risk index of 3.0 per share, while CNA Financial Corp. (CNA) stock cost $42 per share, was expected to yield 3.5% per year in dividends, and had a risk index of 2.0 per share.† You have up to $25,800 to invest in these stocks, and would like to earn at least $958 in dividends over the course of a year. (Assume the dividends to be unchanged for the year.) How many shares (to the nearest tenth of a unit) of each stock should you purchase to meet your requirements and minimize the total risk index for your portfolio? Toronto Dominion Bank _____shares CNA Financial Corp. ___shares What is the minimum total risk index? (Round your answer to two decimal places.)

Investment A woman has $1,400,000 to purchasethree investments. One investment earns 8%, asecond earns 7.5%, and the third earns 10%.a. What investment strategies will give annual earnings of $120,000 from these three investments?b. What one investment strategy will minimize herrisk and return $120,000 if the 10% investmenthas the most risk?

Chapter 6 Solutions

Finite Mathematics for Business, Economics, Life Sciences and Social Sciences

Ch. 6.1 - The following linear programming problem has only...Ch. 6.1 - Use the table method to solve the following linear...Ch. 6.1 - Use the table method to solve the following linear...Ch. 6.1 - Refer to Example 1. Find the basic solution for...Ch. 6.1 - Construct the table of basic solutions and use it...Ch. 6.1 - Construct the table of basic solutions and use it...Ch. 6.1 - Refer to Table 5. For the basic solution...Ch. 6.1 - In Problems 1-8, evaluate the expression. (If...Ch. 6.1 - In Problems 1-8, evaluate the expression. (If...Ch. 6.1 - In Problems 1-8, evaluate the expression. (If...

Additional Math Textbook Solutions

Find more solutions based on key concepts

13. Water Taxi Safety Passengers died when a water taxi sank in Baltimore’s Inner Harbor. Men are typically hea...

Elementary Statistics (13th Edition)

CHECK POINT 1 Find a counterexample to show that the statement The product of two two-digit numbers is a three-...

Thinking Mathematically (6th Edition)

The following set of data is from sample of n=5: a. Compute the mean, median, and mode. b. Compute the range, v...

Basic Business Statistics, Student Value Edition

In Exercises 5-36, express all probabilities as fractions. 23. Combination Lock The typical combination lock us...

Elementary Statistics

In Problems 13-22, for the given functions f and g , find: a. ( fg )( 4 ) b. ( gf )( 2 ) c. ( ff )( 1 ) d. ( gg...

Precalculus

Product of the given expression

Pre-Algebra Student Edition

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.