The Heart of Mathematics: An Invitation to Effective Thinking
4th Edition
ISBN: 9781118156599
Author: Edward B. Burger, Michael Starbird
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 6.2, Problem 36MS
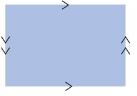
Klein bottle. Using the diagram here for building a Klein bottle (as described in Scction 5.2), count the number of vertices, edges, and faces. Use this data to conjecture the Euler Characteristic for the Klein bottle.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
(d) Consider the sequences (xn), (yn) defined recursively as follows:
Xn+1 = xn2yn,
i.
ii.
n,
Yn+1=Yn2xn for n ≥ 1,
x1 = 1, y₁ = 2.
Calculate x2, y2 and x3, Y3.
-
Show using induction or otherwise that for any natural number
-
Xn+Yni =
(1+2i)".
Hence or otherwise, show that for any natural number n,
iii.
Zn = (V5)” cos(n arctan2),
n = (V5)” sin(n arctan 2).
2.
(a) For each of the following functions, decide whether it is injective, and whether it
is surjective. Justify your answers.
i.
f: Z →
22
Z
3z +1
ii.
9: C→
22
Re(z) + Im(z)
Not use ai please
Chapter 6 Solutions
The Heart of Mathematics: An Invitation to Effective Thinking
Ch. 6.1 - Map maker, map maker make me a graph. Represent...Ch. 6.1 - Unabridged list. Represent cach landmass from...Ch. 6.1 - Will the walk work? Does your graph from...Ch. 6.1 - Walk around the house. Is it possibel to traverse...Ch. 6.1 - Walk the line. Does this graph above have an Euler...Ch. 6.1 - Walkabout. Does this graph have an Euler circuit?...Ch. 6.1 - Linking the loops. In this map, the following...Ch. 6.1 - Scenic drive. (S) Here is a map of Rockystone...Ch. 6.1 - Under-edged. (H) Does this graph have an Euler...Ch. 6.1 - No man is an island. The country of Pelago...
Ch. 6.1 - Path-o-rama. For each graph below, determine if...Ch. 6.1 - Walk around the block. Create a graph of the...Ch. 6.1 - Walking the dogs. Your dogs, Abbey and Bear, love...Ch. 6.1 - Delivery query. The next time you see a postal...Ch. 6.1 - Snow job. (ExH) Shown here is a map of the tiny...Ch. 6.1 - Special delivery. (ExH) Julia is the letter...Ch. 6.1 - Draw this old house. Suppose you wanted to trace...Ch. 6.1 - Path of no return. Consider this map showing a...Ch. 6.1 - Without a trace. Is it possibel to trace out...Ch. 6.1 - New Euler. In the three previous Mindscapes, you...Ch. 6.1 - New edge—new circuit. Look at the graph for...Ch. 6.1 - New edge—new path. Review your work for...Ch. 6.1 - Path to proof. Suppose you have a connected graph...Ch. 6.1 - No Euler no how. Look at graph (a) for Mindscape...Ch. 6.1 - Degree day. (S) For cach graph below, determine...Ch. 6.1 - degrees of proof. Review your work for Mindscape...Ch. 6.1 - Degrees in sequence. Can you draw a graph that has...Ch. 6.1 - Even Steven. Review your work in Mindscape 28 to...Ch. 6.1 - Little League lesson. (H) You are in charge of...Ch. 6.1 - With a group of folks. In a small group, discuss...Ch. 6.1 - Power beyond the mathematics. Provide several...Ch. 6.1 - Here we celebrate the power of algebra as a...Ch. 6.1 - Here we celebrate the power of algebra as a...Ch. 6.1 - Here we celebrate the power of algebra as a...Ch. 6.1 - Here we celebrate the power of algebra as a...Ch. 6.1 - Here we celebrate the power of algebra as a...Ch. 6.2 - What a character! What expression gives the Euler...Ch. 6.2 - Count, then verify. What are the values of V, E,...Ch. 6.2 - Sneeze, then verify. Look at an unopened tissue...Ch. 6.2 - Blow, then verify. Inflate a ballon and use a...Ch. 6.2 - Add one. Find the values V, E, and F for the graph...Ch. 6.2 - Bowling. What is the Euler Characteristic of the...Ch. 6.2 - Making change. We begin with the graph pictured at...Ch. 6.2 - Making a point. Take a connected graph and add a...Ch. 6.2 - On the edge (H). Is it possible to add an edge to...Ch. 6.2 - Soap films. Consider the following sequence of...Ch. 6.2 - Dualing. What is the relationship between the...Ch. 6.2 - Prob. 12MSCh. 6.2 - Lots of separation. Suppose we are told that a...Ch. 6.2 - Prob. 14MSCh. 6.2 - Psychic readings. Someone is thinking of a...Ch. 6.2 - Prob. 16MSCh. 6.2 - Prob. 17MSCh. 6.2 - Circular reasoning. Create a connected graph as...Ch. 6.2 - Prob. 19MSCh. 6.2 - More circles. Consider the sphere described in...Ch. 6.2 - In the rough (S). Count the number of facets,...Ch. 6.2 - Cutting corners (H). The following collection of...Ch. 6.2 - Stellar. The following collection of pictures...Ch. 6.2 - A torus graph (ExH). The Euler Characteristic...Ch. 6.2 - Regular unfolding. Each graph below represents...Ch. 6.2 - A tale of two graphs. Suppose we draw a graph that...Ch. 6.2 - Two graph conjectures (S). Can you conjecture a...Ch. 6.2 - Lots of graphs conjecture. Can you conjecture a...Ch. 6.2 - Torus count. Three hollowed, triangular prisms...Ch. 6.2 - Torus two count (H). Carefully count the number of...Ch. 6.2 - Torus many count. Using the preceding calculations...Ch. 6.2 - Prob. 32MSCh. 6.2 - Tell the truth. Someone said that she made a...Ch. 6.2 - No sphere. Suppose we have a sphere built out of...Ch. 6.2 - Soccer ball. A soccer ball is made of pentagons...Ch. 6.2 - Klein bottle. Using the diagram here for building...Ch. 6.2 - Not many neighbors. Show that every map has at...Ch. 6.2 - Infinite edges. Suppose we consider a conn ected...Ch. 6.2 - Here we celebrate the power of algebra as a...Ch. 6.2 - Prob. 44MSCh. 6.2 - Prob. 45MSCh. 6.2 - Here we celebrate the power of algebra as a...Ch. 6.2 - Here we celebrate the power of algebra as a...Ch. 6.3 - Dont be cross. Here is a drawing of a graph with...Ch. 6.3 - De Plane! De Plane! (S) Is the graph given in...Ch. 6.3 - Countdown (H). For the graph drawing shown, count...Ch. 6.3 - Prob. 4MSCh. 6.3 - Criss-Cross. Is it possible to redraw the graph...Ch. 6.3 - Dont cross in the edge. Each of the graphs drawn...Ch. 6.3 - Hot crossed buns. Each of the graphs drawn below...Ch. 6.3 - Prob. 8MSCh. 6.3 - Spider on a mirror. Is it possible to redraw the...Ch. 6.3 - One more vertex. The graph here is drawn to show...Ch. 6.3 - Yet one more vertex (H). The graph shown is drawn...Ch. 6.3 - Familiar freckles. Is it possible to redraw the...Ch. 6.3 - Remind you of anyone you know? Is it possible to...Ch. 6.3 - Final countdown. For this graph drawing, count the...Ch. 6.3 - Euler check-up. Use your answer to the previous...Ch. 6.3 - Euler second opinion. For the graph drawing shown...Ch. 6.3 - Prob. 17MSCh. 6.3 - Prob. 18MSCh. 6.3 - A colorful museum. This figure shows the floor...Ch. 6.3 - Limit of 5. Start drawing a planar graph. Keep...Ch. 6.3 - Starring the hexagon. Is it possible to redraw...Ch. 6.3 - Prob. 22MSCh. 6.3 - Prob. 23MSCh. 6.3 - Getting greedy. (H) Suppose you are asked to color...Ch. 6.3 - Stingy rather than greedy. By coloring the...Ch. 6.3 - Getting more colorful. Graphs dont have to be...Ch. 6.3 - Prob. 27MSCh. 6.3 - Prob. 28MSCh. 6.3 - Chromatically applied. There are eight radio...Ch. 6.3 - Prob. 30MSCh. 6.3 - Personal perspectives. Write a short essay...Ch. 6.3 - Here we celebrate the power of algebra as a...Ch. 6.3 - Here we celebrate the power of algebra as a...Ch. 6.3 - Prob. 37MSCh. 6.3 - Here we celebrate the power of algebra as a...Ch. 6.3 - Here we celebrate the power of algebra as a...Ch. 6.4 - Up close and personal. Create a graph to model...Ch. 6.4 - Network lookout. Find an examle of a network...Ch. 6.4 - Prob. 3MSCh. 6.4 - Hamiltonian holiday (S). You are interning for a...Ch. 6.4 - Home style. Create a graph to model the rooms in...Ch. 6.4 - Six degrees or less. Suppose this graph is a model...Ch. 6.4 - Degrees of you. Find ten willing friends or...Ch. 6.4 - Campus shortcut. Find a map of your campus and...Ch. 6.4 - Arborist lesson. Which of the graphs below are...Ch. 6.4 - Prob. 10MSCh. 6.4 - Prob. 11MSCh. 6.4 - Prob. 12MSCh. 6.4 - Prob. 13MSCh. 6.4 - Prob. 14MSCh. 6.4 - Prob. 15MSCh. 6.4 - Hamilton Study. Look at the graph you drew to...Ch. 6.4 - Business trip redux. Look back in the section and...Ch. 6.4 - Handling Hamiltons. For each graph below, find a...Ch. 6.4 - Road trip. You are checking out gradua te programs...Ch. 6.4 - Back to Hatties trip. Look back in this section...Ch. 6.4 - Solve the Icosian Game. Find a Hamiltonian circuit...Ch. 6.4 - Hunt for Hamilton (S). A large island country has...Ch. 6.4 - Has no Hamilton. Give some characteristics that...Ch. 6.4 - Cubing Hamilton (ExH). Can you find a Hamihonian...Ch. 6.4 - Hamiltonian path. A Hamiltonian path is a path in...Ch. 6.4 - Sorry, no path. Give some characteristics that...Ch. 6.4 - Prob. 27MSCh. 6.4 - Prob. 28MSCh. 6.4 - Prob. 29MSCh. 6.4 - Prob. 30MSCh. 6.4 - Edge count. Look at all the trees you drew in the...Ch. 6.4 - Personal perspecthes. Write a short essay...Ch. 6.4 - Prob. 33MSCh. 6.4 - Prob. 34MSCh. 6.4 - Dollars and cents. Your spanning tree has three...Ch. 6.4 - Adding up. Your spanning tree has four edges with...Ch. 6.4 - Prob. 38MSCh. 6.4 - Vertex search (H). Your graph has a Hamiltonian...Ch. 6.4 - Binary gossip tree. You told a secret to two of...
Additional Math Textbook Solutions
Find more solutions based on key concepts
CHECK POINT 1 In a survey on musical tastes, respondents were asked: Do you listed to classical music? Do you l...
Thinking Mathematically (6th Edition)
Constructing and Graphing Discrete Probability Distributions In Exercises 19 and 20, (a) construct a probabilit...
Elementary Statistics: Picturing the World (7th Edition)
Limits of sequences Find the limit of the following sequences or determine that the limit does not exist. 29. {...
Calculus: Early Transcendentals (2nd Edition)
Fill in each blank so that the resulting statement is true. If n is a counting number, bn, read ______, indicat...
College Algebra (7th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- 2. Given f(0) = (2 cos 0)² - 3sin²0. 1 a. Show that f(0) = ½ + ½ 7 + - cos cos 20. 2 2 b. Hence, find the exact value of √² 0 ƒ (0) do.arrow_forward2. Given f(0) = (2 cos 0)² - 3sin²0. 1 a. Show that f(0) = ½ + ½ 7 +-cos cos 20. 2 2 b. Hence, find the exact value of √ ƒ (0) d0.arrow_forwardNot use ai pleasearrow_forward
- In a crossover trial comparing a new drug to a standard, π denotes the probabilitythat the new one is judged better. It is desired to estimate π and test H0 : π = 0.5against H1 : π = 0.5. In 20 independent observations, the new drug is better eachtime.(a) Find and plot the likelihood function. Give the ML estimate of π (Hint: youmay use the plot function in R)arrow_forwardQ9. If A and B are two events, prove that P(ANB) ≥ 1 − P(Ā) – P(B). [Note: This is a simplified version of the Bonferroni inequality.] -arrow_forwardCan you explain what this analysis means in layman's terms? - We calculated that a target sample size of 3626, which was based on anticipated baseline 90-day mortality of 22% and a noninferiority margin of no more than 4 percentage points, would give the trial 80% power, at a one-sided alpha level of 2.5%, accounting for a maximum of 5% loss to follow-up and for early stopping rules for three interim analyses.-arrow_forward
- (x)=2x-x2 2 a=2, b = 1/2, C=0 b) Vertex v F(x)=ax 2 + bx + c x= Za V=2.0L YEF(- =) = 4 b (글) JANUARY 17, 2025 WORKSHEET 1 Solve the following four problems on a separate sheet. Fully justify your answers to MATH 122 ล T earn full credit. 1. Let f(x) = 2x- 1x2 2 (a) Rewrite this quadratic function in standard form: f(x) = ax² + bx + c and indicate the values of the coefficients: a, b and c. (b) Find the vertex V, focus F, focal width, directrix D, and the axis of symmetry for the graph of y = f(x). (c) Plot a graph of y = f(x) and indicate all quantities found in part (b) on your graph. (d) Specify the domain and range of the function f. OUR 2. Let g(x) = f(x) u(x) where f is the quadratic function from problem 1 and u is the unit step function: u(x) = { 0 1 if x ≥0 0 if x<0 y = u(x) 0 (a) Write a piecewise formula for the function g. (b) Sketch a graph of y = g(x). (c) Indicate the domain and range of the function g. X фирм where u is the unit step function defined in problem 2. 3. Let…arrow_forwardClasswork for Geometry 1st X S Savvas Realize * MARYIA DASHUTSINA-Ba → CA savvasrealize.com/dashboard/classes/49ec9fc00d8f48ec9a4b05b30c9ee0ba A > SIS © = =Wauconda Middle S... 31 WMS 8th Grade Tea... SIS Grades and Attenda.... esc GEOMETRY 1ST < Study Guide T6 K 18 L 63° 9 N M Quadrilateral JKLM is a parallelogram. What is the m ZKJN? mZKJN = Review Progress acerarrow_forwardQ7. A business office orders paper supplies from one of three vendors, V₁, V2, or V3. Orders are to be placed on two successive days, one order per day. Thus, (V2, V3) might denote that vendor V2 gets the order on the first day and vendor V3 gets the order on the second day. (a) List the sample points in this experiment of ordering paper on two successive days. (b) Assume the vendors are selected at random each day and assign a probability to each sample point. (c) Let A denote the event that the same vendor gets both orders and B the event that V2 gets at least one order. Find P(A), P(B), P(AUB), and P(An B) by summing the probabilities of the sample points in these events.arrow_forward
- Question 1arrow_forward- Q5. Extend Theorem 5 (P(AUB) = P(A) + P(B) = P(ANB)), proved in class, to three events, A, B and C, by finding an expression for P(AUBUC) in terms of the probabilities of A, B and C, of their pair-wise intersections, and the intersection of all three events. (Hint: Begin by considering AUB as a single event).arrow_forwardCan you help me understand this analysis? A 95.7% confidence interval is shown for the intention-to-treat analysis (accounting for alpha spending in interim analyses), and 95% confidence intervals are shown for the other two analyses. The widths of the confidence intervals have not been adjusted for multiplicity. The dashed line indicates the noninferiority margin of 4 percentage points.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Area Between The Curve Problem No 1 - Applications Of Definite Integration - Diploma Maths II; Author: Ekeeda;https://www.youtube.com/watch?v=q3ZU0GnGaxA;License: Standard YouTube License, CC-BY