
Concept explainers
The truss shown consists of nine members and is support by a ball-and-socket at B, a short link at C, and two short links at D. (a) Check that this truss is a simple truss, that completely constrained, and that the reactions at its suppo are statically determinate. (b) Determine the force in each member for P = (−1200 N)j and Q = 0.
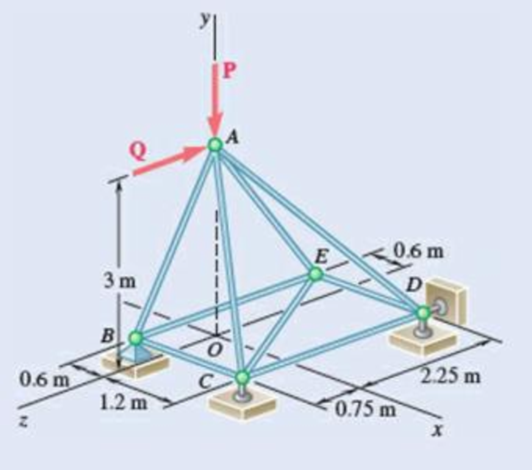
Fig. P6.39
(a)
Verify that the truss is a simple truss, completely constrained, and the reactions at the supports are statically determinate.
Answer to Problem 6.39P
The reactions at supports B, C, and D is
Explanation of Solution
Given information:
The value of the force P is
The value of the force Q is zero.
Calculation:
Show the free-body diagram of the truss as in Figure 1.
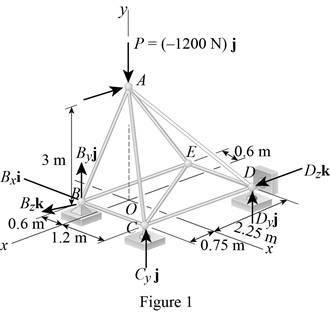
Find the vector coordinates by taking moment about point B.
Equate the coefficients of i to zero.
Equate the coefficients of j to zero.
Equate the coefficients of k to zero.
Substitute 300 N for
Resolve the force components in y-axis.
Substitute 300 N for
The unknown reactions can be calculated with the equilibrium equations. Therefore, the truss is statically determinate, completely constrained and simple truss.
Thus, the reactions at supports B, C, and D is
(b)
Find the force in each member of the truss.
Answer to Problem 6.39P
The force in the member AB is
The force in the member BC is
The force in the member BE is
The force in the member AC is
The force in the member CE is
The force in the member CD is
The force in the member AD is
The force in the member DE is
The force in the member AE is
Explanation of Solution
Show the free-body diagram of the joint B as in Figure 2.
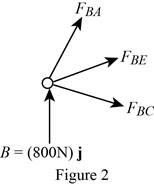
Resolve the force components as follows;
Write the vector value of
Find the scalar quantity of BA using the relation.
Find the force in the member AB as follows;
Substitute
Find the force in the member BC as follows;
Find the force in the member BE as follows;
Equate the coefficients of j to zero.
Equate the coefficients of i to zero.
Substitute –840 N for
Equate the coefficients of k to zero.
Substitute –840 N for
Therefore,
The force in the member AB is
The force in the member BC is
The force in the member BE is
Show the free-body diagram of the joint C as in Figure 3.
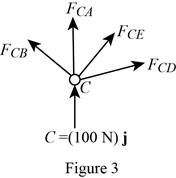
Resolve the force components as follows;
Write the vector value of
Find the scalar quantity of CA using the relation.
Find the force in the member AC as follows;
Substitute
Find the force in the member CB as follows;
Find the force in the member CD as follows;
Write the vector value of
Find the scalar quantity of CE using the relation.
Find the force in the member CE as follows;
Substitute
Equate the coefficients of j to zero.
Equate the coefficients of i to zero.
Substitute –110.6 N for
Equate the coefficients of k to zero.
Substitute –110.6 N for
Therefore,
The force in the member AC is
The force in the member CE is
The force in the member CD is
Show the free-body diagram of the joint D as in Figure 4.
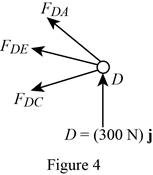
Resolve the force components as follows;
Write the vector value of
Find the scalar quantity of DA using the relation.
Find the force in the member AD as follows;
Substitute
Find the force in the member DC as follows;
Find the force in the member DE as follows;
Equate the coefficients of j to zero.
Equate the coefficients of i to zero.
Substitute –394 N for
Therefore,
The force in the member AD is
The force in the member DE is
Show the free-body diagram of the joint E as in figure 5.
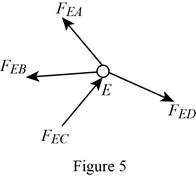
The member AE is not in the xz plane.
Therefore, the force in the member AE is
Want to see more full solutions like this?
Chapter 6 Solutions
VEC MECH 180-DAT EBOOK ACCESS(STAT+DYNA)
- Staring with an energy balance on a cylindirical shell volume element, derive the steady one dimensional heat conduction equation for a long cylinder with constant thermal conductivity in which heat is generated at a rate of egen.arrow_forwardConsider a round potato being baked in an oven. Would you model the heat transfer to the potato as one, two, or three dimensional? Would the heat transfer be steady or transient? Also, which coordinate system would you use to solve this problem, and where would you place the origin? Explain.arrow_forward0 = 6 a = 25 t = 3 Y b = 30 xarrow_forward
- Solve this problem and show all of the workarrow_forwardNo chatgpt pls will upvotearrow_forwardreading is 0.4 mas SHOWN. Assume h₁ = 0.4 m, h₂ = 0.5 m. (a) Do you know the specific weight of mercury? (b) Do you know the specific weight of gasoline? (c) Do you know the specific weight of oil? (a) YHg = 133,000 (b) Ygas = 6867 (c) Yoil = 8829 eTextbook and Media Part 2 N/m³ N/m³ N/m³ A+ Gasoline t +B Oil -Mercury Attempts: unlimited Did you calculate the pressure difference between two locations using the correct specific weight? Did you assume that the pressures in fluid are the same in a horizontal plane even though they are in different tubes? Are the calculated pressures in a column of fluid always higher at lower elevations? Did you account for the fact that the two horizontal tubes of the U-tube are above the ground? Concepts: The pressure in a fluid is a function of the specific weight of the fluid and the height relative to a reference. Pressure is constant in a horizontal plane of a continuous mass of fluid. (a) What is the initial pressure difference? (PA-PB) (b) What is…arrow_forward
- Find the solution of the following Differential Equations 1) "-4y+3y=0 3) "+16y=0 2) y"-16y=0 4) y"-y-6y=0 5) y"+2y=0 7) y"+y=0, (#0) 9) y"-y=0, y(0) = 6, y'(0) = -4 11) y"-4y+3y=0, y(0)=-1, 13) y'(0) = -5 "+2y+2y=0 15) y"-9y=0 17) y"-4y=0 6) y"-2y+2y=0 8) "+4y+5y=0 10) y"-9y=0, y(0) = 2, y'(0) = 0 12) y"-3y+2y= 0, y(0)=-1, y'(0) = 0 14) 4y+4y+y=0 16) "+6y+12y=0 18) 4y+4y+17y=0arrow_forwardAccess Pearson Mastering Engineering Back to my courses Course Home Course Home Scoresarrow_forwardAccess Pearson Mastering Engineering Back to my courses Course Home Course Home Scores Review Next >arrow_forward
 International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
