Chapter 5.4, Problem 64E

a.

To determine

The particle’s velocity at time $t=5$ .

Answer to Problem 64E

The velocity of particle at time $t=5$ is 2 m/sec.

Explanation of Solution

Given:

Graph of function $y=f(x)$

  

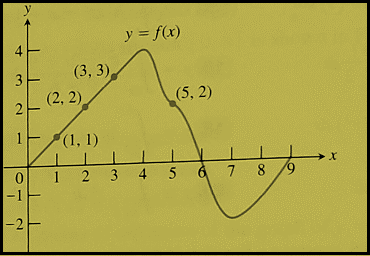
Position of particle at any time $t$ is $s={\displaystyle {\int }_0^{t}f(x)dx}$.

Concept Used:

Velocity: The velocity of a particle is the change in its position over time.

Also, $x-$ axis denotes time $t$ .

Calculation:

According to graph of function,

Point $(5,2)$ lie on the graph then,

  $\begin{array}{c}\text{Put }x=5,y=2\\ y=f(x)\\ 2=f(5)\\ f(5)=2\end{array}$

The velocity of a particle is the change in its position over time then

  $\begin{array}{c}v(t)=\frac{ds}{dt}\\ =\frac{d}{dt}{\displaystyle {\int }_0^{t}f(x)dx}\\ =f(t)\end{array}$

So, $v(t)=f(t)$ .

Now, velocity of particle at time $t=5$ :

Put $t=x=5$ ,

  $\begin{array}{l}v(5)=f(5)\\ v(5)=2\\ v(5)=2\text{ m/sec}\end{array}$

Conclusion:

The velocity of particle at time $t=5$ is 2 m/sec.

b.

To determine

The acceleration of the particle at time $t=5$ is positive or negative.

Answer to Problem 64E

The acceleration of the particle at time $t=5$ is negative.

Explanation of Solution

Given:

Graph of function $y=f(x)$

  

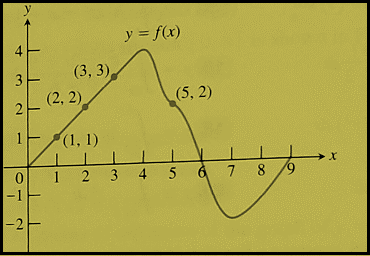
Concept Used:

Theorem: If the derivative of a function is positive on an interval then the function is increasing on that interval; if derivative is negative on that interval then the function is decreasing.

Also, $x-$ axis denotes time $t$ .

Calculation:

The acceleration of particle is slope of the graph.

Now, according to graph

The graph is negative in the neighborhood of $x=5\left(t=x=5\right)$ then slope is negative.

So, the acceleration of the particle at time $t=5$ is negative.

Conclusion:

The acceleration of the particle at time $t=5$ is negative.

c.

To determine

The particle’s position at time $t=3.$

Answer to Problem 64E

The particle’s position is $s=4.5{\text{ units}}^2$ .

Explanation of Solution

Given:

Position of particle at any time $t$ is

  $s={\displaystyle {\int }_0^{t}f(x)dx}$.

Calculation:

Position of particle at any time $t=3$ is

  $s={\displaystyle {\int }_0^{3}f(x)dx}$.

This is the area of the region from $x=0\text{ to }x=3.$

Graph is

  

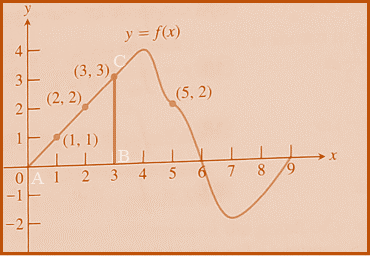
Now, according to graph

  $\text{The area of region }=\text{ area of triangle,}$

Base of triangle $ABC=AB$ ,

And, $AB=3\text{ units}$ .

Height of triangle $ABC=BC$ ,

  $BC=3\text{ units}$ .

Now,

Area of triangle $ABC$ ,

  $\begin{array}{l}\text{Area}=\frac{1}{2}\times \text{Base}\times \text{Height}\\ \text{ =}\frac{1}{2}\times AB\times BC\\ \text{ =}\frac{1}{2}\times 3\times 3=\frac{9}{2}\\ =4.5{\text{ units}}^2\end{array}$

So, $s=4.5{\text{ units}}^2$ .

The particle’s position at time $t=3\text{ is }s=4.5{\text{ units}}^2$ .

Conclusion:

The particle’s position at time $t=3\text{ is }s=4.5{\text{ units}}^2$ .

d.

To determine

The particle’s position has maximum value in time interval $0\le t\le 9$ .

Answer to Problem 64E

The position function $s$ has its largest value at $t=6$ .

Explanation of Solution

Given:

Graph of the function is

  

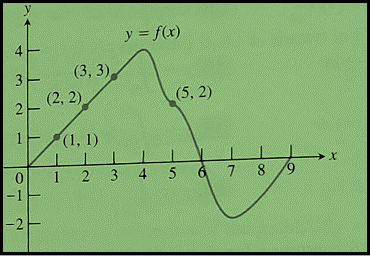
Position of particle at any time $t$ is

  $s={\displaystyle {\int }_0^{t}f(x)dx}$.

And, $x-$ axis denotes time $t$ .

Calculation:

A function $h(x)$ has maximum minimum value when $h\text{'}(x)=0$ .

Now, first derivative of position function $s$ is

  $s\text{'}=v=f(x)$ .

For maxima minima put $s\text{'}=0$ .

  $\begin{array}{c}s\text{'}=0\\ f(x)=0\end{array}$

According to graph, at $x=6$ the value of function $f(6)=0$ .

Also, function changes its values from positive to negative so, position function $s$ has maximum value at $x=6$ .

Hence, $s$ has its largest value at $t=6$ .

Conclusion:

The position function $s$ has its largest value at $t=6$ .

e.

To determine

Find the approximate value of $t$ when acceleration is zero.

Answer to Problem 64E

The acceleration is zero at $t=4\text{ and }t=7$ .

Explanation of Solution

Given:

Graph of the function is

  

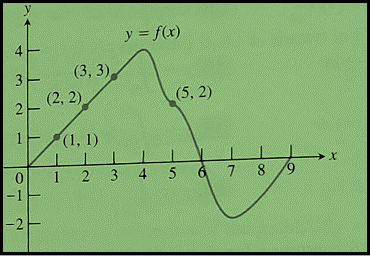
Also, $x-$ axis denotes time $t$ .

Calculation:

According to graph, function has maxima and minima at $t=4\text{ and }t=7$ respectively.

And, acceleration $a(t)=v\text{'}(t)$ (velocity of particle).

Now, $v\text{'}(t)=0$ at $t=4\text{ and }t=7$ because the velocity function has maxima and minima at $t=4\text{ and }t=7$ respectively.

So, at $t=4$ and $t=7$ ,

  $\begin{array}{l}a(t)=v\text{'}(t)\\ a(t)=0.\end{array}$

Hence, the acceleration is zero at $t=4\text{ and }t=7$ .

Conclusion:

The acceleration is zero at $t=4\text{ and }t=7$ .

f.

To determine

Find the value of $t$ when the particle is moving toward the origin and away from the origin.

Answer to Problem 64E

The particle moves away from the origin in time interval $\left[0,\text{ 6}\right]$ .

The particle moves toward to the origin in time interval $\left[6,9\right]$ .

Explanation of Solution

Given:

Graph of the function is

  

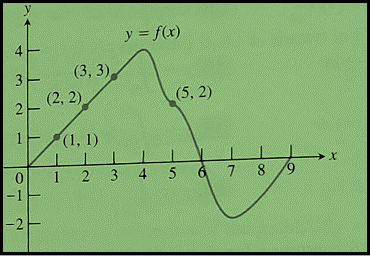
Position of particle at any time $t$ is

  $s={\displaystyle {\int }_0^{t}f(x)dx}$.

Also, $x-$ axis denotes time $t$ .

Calculation:

The particle moves toward to the origin when the area of region is negative and moves away from the origin when the area of region is positive.

Now,

Position of particle during time $0\le t\le 6$

  $s={\displaystyle {\int }_0^{6}f(x)dx}>0$because the graph lies above the $x-$ axis.

So, the particle moves away from the origin in time interval $\left[0,\text{ 6}\right]$ .

And, position of particle during time $0\le t\le 6$

  $s={\displaystyle {\int }_6^{9}f(x)dx}<0$because the graph lies below the $x-$ axis.

So, the particle moves toward to the origin in time interval $\left[6,9\right]$ .

Conclusion:

The particle moves away from the origin in time interval $\left[0,\text{ 6}\right]$ .

The particle moves toward to the origin in time interval $\left[6,9\right]$ .

g.

To determine

The particle lie on which side of the origin at time $t=9$ .

Answer to Problem 64E

The particle lie on the right side of the origin at time $t=9$ .

Explanation of Solution

Given:

Graph of the function is

  

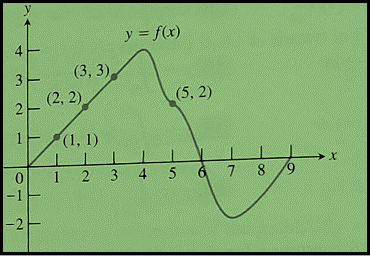
Also, $x-$ axis denotes time $t$ .

Calculation:

According to the graph, the area of the region lie above the $x-$ axis is more than the area of the region lie below the $x-$ axis. So, the particle lie on the right side of the origin at time $t=9$ .

Conclusion:

The particle lie on the right side of the origin at time $t=9$ .