Chapter 5.4, Problem 63E

(a.)

To determine

The value of $H\left(0\right)$ .

Answer to Problem 63E

It has been determined that $H\left(0\right)=0$ .

Explanation of Solution

Given:

  $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ , where $f$ is a continuous function with domain $\left[0,12\right]$

graphed as follows:

  

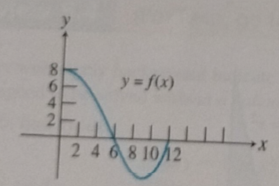
Concept used:

  ${\displaystyle {\int }_a^{a}f\left(t\right)dt}=0$

Calculation:

It is given that $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ .

Put $x=0$ in $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ to get,

  $H\left(0\right)={\displaystyle {\int }_0^{0}f\left(t\right)dt}$

As discussed, ${\displaystyle {\int }_a^{a}f\left(t\right)dt}=0$ .

Put $a=0$ in ${\displaystyle {\int }_a^{a}f\left(t\right)dt}=0$ to get,

  ${\displaystyle {\int }_0^{0}f\left(t\right)dt}=0$

Put ${\displaystyle {\int }_0^{0}f\left(t\right)dt}=0$ in $H\left(0\right)={\displaystyle {\int }_0^{0}f\left(t\right)dt}$ to get,

  $H\left(0\right)=0$

Conclusion:

It has been determined that $H\left(0\right)=0$ .

(b.)

To determine

The interval in which the function $H$ is increasing.

Answer to Problem 63E

It has been determined that the interval in which the function $H$ is increasing is $\left[0,6\right]$ .

Explanation of Solution

Given:

  $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ , where $f$ is a continuous function with domain $\left[0,12\right]$

graphed as follows:

  

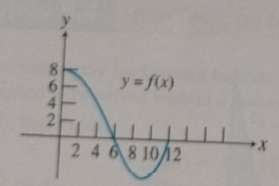
Concept used:

A function $f\left(x\right)$ is said to be increasing in an interval if the value of the function increases when $x$ increases.

Calculation:

It is given that $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ .

According to the definition of definite integral, $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ is nothing but the area under $f$ from $0$ to $x$ .

Moreover, according to convention, the area above the $x$ axis is considered as positive area and area below the $x$ axis is considered as negative area.

It can be seen that the area under $f$ is positive from $0$ to $6$ and is negative from $6$ to $12$ .

This implies that $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ , which evaluates the area under $f$ from $0$ to $x$ , is increasing in the interval $\left[0,6\right]$ .

Conclusion:

It has been determined that the interval in which the function $H$ is increasing is $\left[0,6\right]$ .

(c.)

To determine

The interval in which the graph of $H$ is concave up.

Answer to Problem 63E

It has been determined that the graph of $H$ is concave up in the interval $\left[9,12\right]$ .

Explanation of Solution

Given:

  $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ , where $f$ is a continuous function with domain $\left[0,12\right]$

graphed as follows:

  

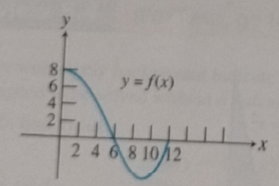
Concept used:

A function is concave up in the interval where its second derivative is positive.

Calculation:

It is given that $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ .

According to the Fundamental Theorem of Calculus,

  $H^{\prime }\left(x\right)=f\left(x\right)$

Differentiating,

  $H^{″}\left(x\right)=f^{\prime }\left(x\right)$

Now, the graph of $H$ is concave up in the interval where $H^{″}\left(x\right)>0$ .

Equivalently, the graph of $H$ is concave up in the interval where $f^{\prime }\left(x\right)>0$ .

So, the graph of $H$ is concave up in the interval where $f$ is increasing.

It can be seen from the given graph that $f$ is increasing in the interval $\left[9,12\right]$ .

Then, the graph of $H$ is concave up in the interval $\left[9,12\right]$ .

Conclusion:

It has been determined that the graph of $H$ is concave up in the interval $\left[9,12\right]$ .

(d.)

To determine

If $H\left(12\right)$ is positive or negative.

Answer to Problem 63E

It has been determined that $H\left(12\right)$ must be positive.

Explanation of Solution

Given:

  $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ , where $f$ is a continuous function with domain $\left[0,12\right]$

graphed as follows:

  

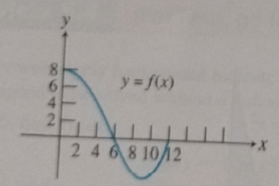
Concept used:

A definite integral of a function is the area under the curve of the function in the given interval.

Calculation:

It is given that $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ .

Put $x=12$ in $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ to get,

  $H\left(12\right)={\displaystyle {\int }_0^{12}f\left(t\right)dt}$

This implies that $H\left(12\right)$ is the area under the curve of $f$ from $x=0$ to $x=12$ .

Now, the area above the $x$ axis is considered to be positive and the area under the $x$ axis is considered to be negative.

It can be seen from the given graph that the area under the curve of $f$ is positive from $x=0$ to $x=6$ and negative from $x=6$ to $x=12$ .

However, it can also be seen that the magnitude of the positive area under the curve of $f$ from $x=0$ to $x=6$ is greater than the magnitude of the negative area under the curve of $f$ from $x=6$ to $x=12$ .

Then, the sum of these areas, which is the area under the curve of $f$ from $x=0$ to $x=12$ must be positive.

Hence, $H\left(12\right)$ must be positive.

Conclusion:

It has been determined that $H\left(12\right)$ must be positive.

(e.)

To determine

The point where $H$ achieves its maximum value.

Answer to Problem 63E

It has been determined that $H$ achieves its maximum value at $x=6$ .

Explanation of Solution

Given:

  $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ , where $f$ is a continuous function with domain $\left[0,12\right]$

graphed as follows:

  

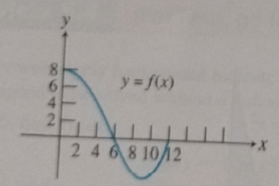
Concept used:

A function attains its maximum value at the point where it changes from being increasing to decreasing.

Calculation:

It is given that $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ .

According to the definition of definite integral, $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ is nothing but the area under $f$ from $0$ to $x$ .

Moreover, according to convention, the area above the $x$ axis is considered as positive area and area below the $x$ axis is considered as negative area.

It can be seen that the area under $f$ is positive from $0$ to $6$ and is negative from $6$ to $12$ .

This implies that $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ , which evaluates the area under $f$ from $0$ to $x$ , is increasing in the interval $\left[0,6\right]$ and decreasing in the interval $\left[6,12\right]$ .

As determined previously,

  $H\left(0\right)=0$

As determined previously, $H\left(12\right)$ is positive.

Combining, it follows that $H$ achieves its maximum value at $x=6$ .

Conclusion:

It has been determined that $H$ achieves its maximum value at $x=6$ .

(f.)

To determine

The point where $H$ achieves its minimum value.

Answer to Problem 63E

It has been determined that $H$ achieves its minimum value at $x=0$ .

Explanation of Solution

Given:

  $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ , where $f$ is a continuous function with domain $\left[0,12\right]$

graphed as follows:

  

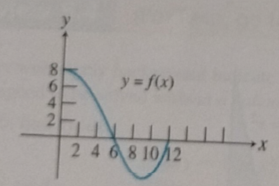
Concept used:

A function attains its minimum value either at the end points or at the point where it stops being a decreasing function.

Calculation:

It is given that $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ .

According to the definition of definite integral, $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ is nothing but the area under $f$ from $0$ to $x$ .

Moreover, according to convention, the area above the $x$ axis is considered as positive area and area below the $x$ axis is considered as negative area.

It can be seen that the area under $f$ is positive from $0$ to $6$ and is negative from $6$ to $12$ .

This implies that $H\left(x\right)={\displaystyle {\int }_0^{x}f\left(t\right)dt}$ , which evaluates the area under $f$ from $0$ to $x$ , is increasing in the interval $\left[0,6\right]$ and decreasing in the interval $\left[6,12\right]$ .

As determined previously,

  $H\left(0\right)=0$

As determined previously, $H\left(12\right)$ is positive.

Combining, it follows that $H$ achieves its minimum value at $x=0$ .

Conclusion:

It has been determined that $H$ achieves its minimum value at $x=0$ .