Algebra 1
1st Edition
ISBN: 9780078884801
Author: McGraw-Hill/Glencoe
Publisher: Glencoe/McGraw-Hill School Pub Co
expand_more
expand_more
format_list_bulleted
Videos
Question
Chapter 5.1, Problem 45PPS
(a)
To determine
Draw a picture to represent this situation.
(a)
Expert Solution
Answer to Problem 45PPS
The right side must be down than the right side
Explanation of Solution
Given:
In a balance have 12 pounds on the left side and 18 pounds on the right side.
Concept Used:
As 12 lbs is less than 18 lbs. So the right side must be down than the right side.
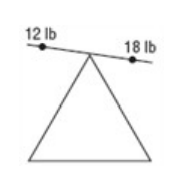
Thus, the right side must be down than the right side as 12pounds on the left side and 18 pounds on the right side
(b)
To determine
Write an inequality.
(b)
Expert Solution
Answer to Problem 45PPS
12 lbs < 18lbs
Explanation of Solution
Given:
In a balance have 12 pounds on the left side and 18 pounds on the tight side.
Concept Used:
Left side 12 lbs is less than the right side 18 lbs.
Inequality: 12 lbs < 18lbs
Calculation:
Inequality: 12 lbs < 18lbs
Thus, we can write the inequality 12 lbs < 18lbs
(c)
To determine
Create a table showing the result of doubling, tripling or quadrupling the weight of each side of the balance.
Create a second table showing the result of reducing the weight on each side of the balance by a factor of 12 ; 13 and 14 . Include a column in each table for the inequality representing each situation.
(c)
Expert Solution
Answer to Problem 45PPS
Explanation of Solution
Given: In a balance have 12 pounds on the left side and 18 pounds on the tight side.
Concept Used:
Make two tables to represent the situation doubling, tripling or quadrupling the weight of each side of the balance and by a factor of 12 ; 13 and 14 .
Calculation:
Create a table showing the result of doubling, tripling or quadrupling the weight of each side of the balance.
| x | Original | 12 Pound | < | 18 Pound |
| x2 | Doubling | 24 Pound | < | 36 Pound |
| x3 | Tripling | 36 Pound | < | 54 Pound |
| x4 | Quadrupling | 48 Pound | < | 72 Pound |
Create a second table showing the result of reducing the weight on each side of the balance by a factor of 12 ; 13 and 14 . Include a column in each table for the inequality representing each situation.
| x | Original | 12 Pound | < | 18 Pound |
| x12 | by a factor of 12 | 6 Pound | < | 9 Pound |
| x13 | by a factor of 13 | 4 Pound | < | 6 Pound |
| x14 | by a factor of 14 | 3 Pound | < | 4.5 Pound |
Thus, the two tables represent the situations.
(d)
To determine
Describe the effect multiplying or dividing each side of an inequality by the same positive value has on the inequality.
(d)
Expert Solution
Explanation of Solution
Given: In a balance have 12 pounds on the left side and 18 pounds on the tight side.
Concept Used:
If a true inequality is multiplied by a positive number, the resulting inequality is also true.
If a true inequality is divided by a positive number, the resulting inequality is also true.
Thus, if a true inequality is multiplied by a positive number, the resulting inequality is also true and if a true inequality is divided by a positive number, the resulting inequality is also true.
Chapter 5 Solutions
Algebra 1
Ch. 5.1 - Prob. 1ACYPCh. 5.1 - Prob. 1BCYPCh. 5.1 - Prob. 2CYPCh. 5.1 - Prob. 3ACYPCh. 5.1 - Prob. 3BCYPCh. 5.1 - Prob. 4CYPCh. 5.1 - Prob. 1CYUCh. 5.1 - Prob. 2CYUCh. 5.1 - Prob. 3CYUCh. 5.1 - Prob. 4CYU
Ch. 5.1 - Prob. 5CYUCh. 5.1 - Prob. 6CYUCh. 5.1 - Prob. 7CYUCh. 5.1 - Prob. 8CYUCh. 5.1 - Prob. 9CYUCh. 5.1 - Prob. 10CYUCh. 5.1 - Prob. 11CYUCh. 5.1 - Prob. 12PPSCh. 5.1 - Prob. 13PPSCh. 5.1 - Prob. 14PPSCh. 5.1 - Prob. 15PPSCh. 5.1 - Prob. 16PPSCh. 5.1 - Prob. 17PPSCh. 5.1 - Prob. 18PPSCh. 5.1 - Prob. 19PPSCh. 5.1 - Prob. 20PPSCh. 5.1 - Prob. 21PPSCh. 5.1 - Prob. 22PPSCh. 5.1 - Prob. 23PPSCh. 5.1 - Prob. 24PPSCh. 5.1 - Prob. 25PPSCh. 5.1 - Prob. 26PPSCh. 5.1 - Prob. 27PPSCh. 5.1 - Prob. 28PPSCh. 5.1 - Prob. 29PPSCh. 5.1 - Prob. 30PPSCh. 5.1 - Prob. 31PPSCh. 5.1 - Prob. 32PPSCh. 5.1 - Prob. 33PPSCh. 5.1 - Prob. 34PPSCh. 5.1 - Prob. 35PPSCh. 5.1 - Prob. 36PPSCh. 5.1 - Prob. 37PPSCh. 5.1 - Prob. 38PPSCh. 5.1 - Prob. 39PPSCh. 5.1 - Prob. 40PPSCh. 5.1 - Prob. 41PPSCh. 5.1 - Prob. 42PPSCh. 5.1 - Prob. 43PPSCh. 5.1 - Prob. 44PPSCh. 5.1 - Prob. 45PPSCh. 5.1 - Prob. 46PPSCh. 5.1 - Prob. 47PPSCh. 5.1 - Prob. 48PPSCh. 5.1 - Prob. 49PPSCh. 5.1 - Prob. 50PPSCh. 5.1 - Prob. 51PPSCh. 5.1 - Prob. 52HPCh. 5.1 - Prob. 53HPCh. 5.1 - Prob. 54HPCh. 5.1 - Prob. 55HPCh. 5.1 - Prob. 56HPCh. 5.1 - Prob. 57STPCh. 5.1 - Prob. 58STPCh. 5.1 - Prob. 59STPCh. 5.1 - Prob. 60STPCh. 5.1 - Prob. 61SRCh. 5.1 - Prob. 62SRCh. 5.1 - Prob. 63SRCh. 5.1 - Prob. 64SRCh. 5.1 - Prob. 65SRCh. 5.1 - Prob. 66SRCh. 5.1 - Prob. 67SRCh. 5.1 - Prob. 68SRCh. 5.1 - Prob. 69SRCh. 5.1 - Prob. 70SRCh. 5.1 - Prob. 71SRCh. 5.1 - Prob. 72SRCh. 5.1 - Prob. 73SRCh. 5.1 - Prob. 74SCh. 5.1 - Prob. 75SCh. 5.1 - Prob. 76SCh. 5.1 - Prob. 77SCh. 5.1 - Prob. 78SCh. 5.1 - Prob. 79SCh. 5.1 - Prob. 80SCh. 5.1 - Prob. 81SCh. 5.2 - Prob. 1CYPCh. 5.2 - Prob. 2ACYPCh. 5.2 - Prob. 2BCYPCh. 5.2 - Prob. 2CCYPCh. 5.2 - Prob. 2DCYPCh. 5.2 - Prob. 3ACYPCh. 5.2 - Prob. 3BCYPCh. 5.2 - Prob. 3CCYPCh. 5.2 - Prob. 3DCYPCh. 5.2 - Prob. 1CYUCh. 5.2 - Prob. 2CYUCh. 5.2 - Prob. 3CYUCh. 5.2 - Prob. 4CYUCh. 5.2 - Prob. 5CYUCh. 5.2 - Prob. 6CYUCh. 5.2 - Prob. 7CYUCh. 5.2 - Prob. 8CYUCh. 5.2 - Prob. 9CYUCh. 5.2 - Prob. 10PPSCh. 5.2 - Prob. 11PPSCh. 5.2 - Prob. 12PPSCh. 5.2 - Prob. 13PPSCh. 5.2 - Prob. 14PPSCh. 5.2 - Prob. 15PPSCh. 5.2 - Prob. 16PPSCh. 5.2 - Prob. 17PPSCh. 5.2 - Prob. 18PPSCh. 5.2 - Prob. 19PPSCh. 5.2 - Prob. 20PPSCh. 5.2 - Prob. 21PPSCh. 5.2 - Prob. 22PPSCh. 5.2 - Prob. 23PPSCh. 5.2 - Prob. 24PPSCh. 5.2 - Prob. 25PPSCh. 5.2 - Prob. 26PPSCh. 5.2 - Prob. 27PPSCh. 5.2 - Prob. 28PPSCh. 5.2 - Prob. 29PPSCh. 5.2 - Prob. 30PPSCh. 5.2 - Prob. 31PPSCh. 5.2 - Prob. 32PPSCh. 5.2 - Prob. 33PPSCh. 5.2 - Prob. 34PPSCh. 5.2 - Prob. 35PPSCh. 5.2 - Prob. 36PPSCh. 5.2 - Prob. 37PPSCh. 5.2 - Prob. 38PPSCh. 5.2 - Prob. 39PPSCh. 5.2 - Prob. 40PPSCh. 5.2 - Prob. 41PPSCh. 5.2 - Prob. 42HPCh. 5.2 - Prob. 43HPCh. 5.2 - Prob. 44HPCh. 5.2 - Prob. 45HPCh. 5.2 - Prob. 46HPCh. 5.2 - Prob. 47HPCh. 5.2 - Prob. 48STPCh. 5.2 - Prob. 49STPCh. 5.2 - Prob. 50STPCh. 5.2 - Prob. 51STPCh. 5.2 - Prob. 52SRCh. 5.2 - Prob. 53SRCh. 5.2 - Prob. 54SRCh. 5.2 - Prob. 55SRCh. 5.2 - Prob. 56SRCh. 5.2 - Prob. 57SRCh. 5.2 - Prob. 58SRCh. 5.2 - Prob. 59SRCh. 5.2 - Prob. 60SRCh. 5.2 - Prob. 61SRCh. 5.2 - Prob. 62SRCh. 5.2 - Prob. 63SCh. 5.2 - Prob. 64SCh. 5.2 - Prob. 65SCh. 5.2 - Prob. 66SCh. 5.2 - Prob. 67SCh. 5.2 - Prob. 68SCh. 5.3 - Prob. 1CYPCh. 5.3 - Prob. 2ACYPCh. 5.3 - Prob. 2BCYPCh. 5.3 - Prob. 3CYPCh. 5.3 - Prob. 4ACYPCh. 5.3 - Prob. 4BCYPCh. 5.3 - Prob. 5ACYPCh. 5.3 - Prob. 5BCYPCh. 5.3 - Prob. 1CYUCh. 5.3 - Prob. 2CYUCh. 5.3 - Prob. 3CYUCh. 5.3 - Prob. 4CYUCh. 5.3 - Prob. 5CYUCh. 5.3 - Prob. 6CYUCh. 5.3 - Prob. 7CYUCh. 5.3 - Prob. 8CYUCh. 5.3 - Prob. 9CYUCh. 5.3 - Prob. 10CYUCh. 5.3 - Prob. 11CYUCh. 5.3 - Prob. 12PPSCh. 5.3 - Prob. 13PPSCh. 5.3 - Prob. 14PPSCh. 5.3 - Prob. 15PPSCh. 5.3 - Prob. 16PPSCh. 5.3 - Prob. 17PPSCh. 5.3 - Prob. 18PPSCh. 5.3 - Prob. 19PPSCh. 5.3 - Prob. 20PPSCh. 5.3 - Prob. 21PPSCh. 5.3 - Prob. 22PPSCh. 5.3 - Prob. 23PPSCh. 5.3 - Prob. 24PPSCh. 5.3 - Prob. 25PPSCh. 5.3 - Prob. 26PPSCh. 5.3 - Prob. 27PPSCh. 5.3 - Prob. 28PPSCh. 5.3 - Prob. 29PPSCh. 5.3 - Prob. 30PPSCh. 5.3 - Prob. 31PPSCh. 5.3 - Prob. 32PPSCh. 5.3 - Prob. 33PPSCh. 5.3 - Prob. 34PPSCh. 5.3 - Prob. 35PPSCh. 5.3 - Prob. 36PPSCh. 5.3 - Prob. 37PPSCh. 5.3 - Prob. 38PPSCh. 5.3 - Prob. 39PPSCh. 5.3 - Prob. 40PPSCh. 5.3 - Prob. 41PPSCh. 5.3 - Prob. 42PPSCh. 5.3 - Prob. 43PPSCh. 5.3 - Prob. 44PPSCh. 5.3 - Prob. 45PPSCh. 5.3 - Prob. 46PPSCh. 5.3 - Prob. 47PPSCh. 5.3 - Prob. 48PPSCh. 5.3 - Prob. 49PPSCh. 5.3 - Prob. 50PPSCh. 5.3 - Prob. 51PPSCh. 5.3 - Prob. 52PPSCh. 5.3 - Prob. 53PPSCh. 5.3 - Prob. 54PPSCh. 5.3 - Prob. 55HPCh. 5.3 - Prob. 56HPCh. 5.3 - Prob. 57HPCh. 5.3 - Prob. 58HPCh. 5.3 - Prob. 59HPCh. 5.3 - Prob. 60STPCh. 5.3 - Prob. 61STPCh. 5.3 - Prob. 62STPCh. 5.3 - Prob. 63STPCh. 5.3 - Prob. 64SRCh. 5.3 - Prob. 65SRCh. 5.3 - Prob. 66SRCh. 5.3 - Prob. 67SRCh. 5.3 - Prob. 68SRCh. 5.3 - Prob. 69SRCh. 5.3 - Prob. 70SRCh. 5.3 - Prob. 71SRCh. 5.3 - Prob. 72SRCh. 5.3 - Prob. 73SRCh. 5.3 - Prob. 74SRCh. 5.3 - Prob. 75SRCh. 5.3 - Prob. 76SRCh. 5.3 - Prob. 77SRCh. 5.3 - Prob. 78SCh. 5.3 - Prob. 79SCh. 5.3 - Prob. 80SCh. 5.3 - Prob. 81SCh. 5.3 - Prob. 82SCh. 5.3 - Prob. 83SCh. 5.4 - Prob. 1ACYPCh. 5.4 - Prob. 1BCYPCh. 5.4 - Prob. 2BCYPCh. 5.4 - Prob. 3ACYPCh. 5.4 - Prob. 3BCYPCh. 5.4 - Prob. 1CYUCh. 5.4 - Prob. 2CYUCh. 5.4 - Prob. 3CYUCh. 5.4 - Prob. 4CYUCh. 5.4 - Prob. 5CYUCh. 5.4 - Prob. 6PPSCh. 5.4 - Prob. 7PPSCh. 5.4 - Prob. 8PPSCh. 5.4 - Prob. 9PPSCh. 5.4 - Prob. 10PPSCh. 5.4 - Prob. 11PPSCh. 5.4 - Prob. 12PPSCh. 5.4 - Prob. 13PPSCh. 5.4 - Prob. 14PPSCh. 5.4 - Prob. 15PPSCh. 5.4 - Prob. 16PPSCh. 5.4 - Prob. 17PPSCh. 5.4 - Prob. 18PPSCh. 5.4 - Prob. 19PPSCh. 5.4 - Prob. 20PPSCh. 5.4 - Prob. 21PPSCh. 5.4 - Prob. 22PPSCh. 5.4 - Prob. 23PPSCh. 5.4 - Prob. 24PPSCh. 5.4 - Prob. 25PPSCh. 5.4 - Prob. 26PPSCh. 5.4 - Prob. 27PPSCh. 5.4 - Prob. 28PPSCh. 5.4 - Prob. 29PPSCh. 5.4 - Prob. 30PPSCh. 5.4 - Prob. 31PPSCh. 5.4 - Prob. 32PPSCh. 5.4 - Prob. 33PPSCh. 5.4 - Prob. 34PPSCh. 5.4 - Prob. 35PPSCh. 5.4 - Prob. 36PPSCh. 5.4 - Prob. 37PPSCh. 5.4 - Prob. 38PPSCh. 5.4 - Prob. 39HPCh. 5.4 - Prob. 40HPCh. 5.4 - Prob. 41HPCh. 5.4 - Prob. 42HPCh. 5.4 - Prob. 43HPCh. 5.4 - Prob. 44STPCh. 5.4 - Prob. 45STPCh. 5.4 - Prob. 46STPCh. 5.4 - Prob. 47STPCh. 5.4 - Prob. 48SRCh. 5.4 - Prob. 49SRCh. 5.4 - Prob. 50SRCh. 5.4 - Prob. 51SRCh. 5.4 - Prob. 52SRCh. 5.4 - Prob. 53SRCh. 5.4 - Prob. 54SRCh. 5.4 - Prob. 55SRCh. 5.4 - Prob. 56SRCh. 5.4 - Prob. 57SRCh. 5.4 - Prob. 58SRCh. 5.4 - Prob. 59SRCh. 5.4 - Prob. 60SRCh. 5.4 - Prob. 61SRCh. 5.4 - Prob. 62SRCh. 5.4 - Prob. 63SRCh. 5.4 - Prob. 64SCh. 5.4 - Prob. 65SCh. 5.4 - Prob. 66SCh. 5.4 - Prob. 67SCh. 5.4 - Prob. 68SCh. 5.4 - Prob. 69SCh. 5.4 - Prob. 70SCh. 5.4 - Prob. 71SCh. 5.4 - Prob. 72SCh. 5.5 - Prob. 1BCYPCh. 5.5 - Prob. 2CYPCh. 5.5 - Prob. 3ACYPCh. 5.5 - Prob. 3BCYPCh. 5.5 - Prob. 20PPSCh. 5.5 - Prob. 24PPSCh. 5.5 - Prob. 25PPSCh. 5.5 - Prob. 26PPSCh. 5.5 - Prob. 27PPSCh. 5.5 - Prob. 28PPSCh. 5.5 - Prob. 29PPSCh. 5.5 - Prob. 32PPSCh. 5.5 - Prob. 33PPSCh. 5.5 - Prob. 34PPSCh. 5.5 - Prob. 35PPSCh. 5.5 - Prob. 36PPSCh. 5.5 - Prob. 37PPSCh. 5.5 - Prob. 38PPSCh. 5.5 - Prob. 39PPSCh. 5.5 - Prob. 40PPSCh. 5.5 - Prob. 41PPSCh. 5.5 - Prob. 43HPCh. 5.5 - Prob. 44HPCh. 5.5 - Prob. 45HPCh. 5.5 - Prob. 1ACYPCh. 5.5 - Prob. 1CYUCh. 5.5 - Prob. 2CYUCh. 5.5 - Prob. 3CYUCh. 5.5 - Prob. 4CYUCh. 5.5 - Prob. 5CYUCh. 5.5 - Prob. 6CYUCh. 5.5 - Prob. 7CYUCh. 5.5 - Prob. 8PPSCh. 5.5 - Prob. 9PPSCh. 5.5 - Prob. 10PPSCh. 5.5 - Prob. 11PPSCh. 5.5 - Prob. 12PPSCh. 5.5 - Prob. 13PPSCh. 5.5 - Prob. 14PPSCh. 5.5 - Prob. 15PPSCh. 5.5 - Prob. 16PPSCh. 5.5 - Prob. 17PPSCh. 5.5 - Prob. 18PPSCh. 5.5 - Prob. 19PPSCh. 5.5 - Prob. 21PPSCh. 5.5 - Prob. 22PPSCh. 5.5 - Prob. 23PPSCh. 5.5 - Prob. 30PPSCh. 5.5 - Prob. 31PPSCh. 5.5 - Prob. 42PPSCh. 5.5 - Prob. 46HPCh. 5.5 - Prob. 47HPCh. 5.5 - Prob. 48STPCh. 5.5 - Prob. 49STPCh. 5.5 - Prob. 50STPCh. 5.5 - Prob. 51STPCh. 5.5 - Prob. 52SRCh. 5.5 - Prob. 53SRCh. 5.5 - Prob. 54SRCh. 5.5 - Prob. 55SRCh. 5.5 - Prob. 56SRCh. 5.5 - Prob. 57SRCh. 5.5 - Prob. 58SRCh. 5.5 - Prob. 59SRCh. 5.5 - Prob. 60SRCh. 5.5 - Prob. 61SCh. 5.5 - Prob. 62SCh. 5.5 - Prob. 63SCh. 5.5 - Prob. 64SCh. 5.5 - Prob. 65SCh. 5.5 - Prob. 66SCh. 5.5 - Prob. 67SCh. 5.5 - Prob. 68SCh. 5.6 - Prob. 13PPSCh. 5.6 - Prob. 14PPSCh. 5.6 - Prob. 15PPSCh. 5.6 - Prob. 16PPSCh. 5.6 - Prob. 17PPSCh. 5.6 - Prob. 20PPSCh. 5.6 - Prob. 21PPSCh. 5.6 - Prob. 22PPSCh. 5.6 - Prob. 25PPSCh. 5.6 - Prob. 1ACYPCh. 5.6 - Prob. 1BCYPCh. 5.6 - Prob. 2ACYPCh. 5.6 - Prob. 2BCYPCh. 5.6 - Prob. 3ACYPCh. 5.6 - Prob. 3BCYPCh. 5.6 - Prob. 4CYPCh. 5.6 - Prob. 1CYUCh. 5.6 - Prob. 2CYUCh. 5.6 - Prob. 3CYUCh. 5.6 - Prob. 4CYUCh. 5.6 - Prob. 5CYUCh. 5.6 - Prob. 6CYUCh. 5.6 - Prob. 7CYUCh. 5.6 - Prob. 8CYUCh. 5.6 - Prob. 9CYUCh. 5.6 - Prob. 10CYUCh. 5.6 - Prob. 11CYUCh. 5.6 - Prob. 12PPSCh. 5.6 - Prob. 18PPSCh. 5.6 - Prob. 19PPSCh. 5.6 - Prob. 23PPSCh. 5.6 - Prob. 24PPSCh. 5.6 - Prob. 26PPSCh. 5.6 - Prob. 27PPSCh. 5.6 - Prob. 28PPSCh. 5.6 - Prob. 29PPSCh. 5.6 - Prob. 30PPSCh. 5.6 - Prob. 31PPSCh. 5.6 - Prob. 32PPSCh. 5.6 - Prob. 33PPSCh. 5.6 - Prob. 34PPSCh. 5.6 - Prob. 35PPSCh. 5.6 - Prob. 36PPSCh. 5.6 - Prob. 37PPSCh. 5.6 - Prob. 38PPSCh. 5.6 - Prob. 39PPSCh. 5.6 - Prob. 40PPSCh. 5.6 - Prob. 41PPSCh. 5.6 - Prob. 42PPSCh. 5.6 - Prob. 43PPSCh. 5.6 - Prob. 44PPSCh. 5.6 - Prob. 45PPSCh. 5.6 - Prob. 46HPCh. 5.6 - Prob. 47HPCh. 5.6 - Prob. 48HPCh. 5.6 - Prob. 49HPCh. 5.6 - Prob. 50HPCh. 5.6 - Prob. 51STPCh. 5.6 - Prob. 52STPCh. 5.6 - Prob. 53STPCh. 5.6 - Prob. 54STPCh. 5.6 - Prob. 55SRCh. 5.6 - Prob. 56SRCh. 5.6 - Prob. 57SRCh. 5.6 - Prob. 58SRCh. 5.6 - Prob. 59SRCh. 5.6 - Prob. 60SRCh. 5.6 - Prob. 61SRCh. 5.6 - Prob. 62SRCh. 5.6 - Prob. 63SRCh. 5.6 - Prob. 64SRCh. 5.6 - Prob. 65SCh. 5 - Prob. 1SGRCh. 5 - Prob. 2SGRCh. 5 - Prob. 3SGRCh. 5 - Prob. 4SGRCh. 5 - Prob. 5SGRCh. 5 - Prob. 6SGRCh. 5 - Prob. 7SGRCh. 5 - Prob. 9SGRCh. 5 - Prob. 10SGRCh. 5 - Prob. 11SGRCh. 5 - Prob. 12SGRCh. 5 - Prob. 13SGRCh. 5 - Prob. 14SGRCh. 5 - Prob. 15SGRCh. 5 - Prob. 16SGRCh. 5 - Prob. 17SGRCh. 5 - Prob. 18SGRCh. 5 - Prob. 20SGRCh. 5 - Prob. 21SGRCh. 5 - Prob. 22SGRCh. 5 - Prob. 23SGRCh. 5 - Prob. 24SGRCh. 5 - Prob. 25SGRCh. 5 - Prob. 26SGRCh. 5 - Prob. 30SGRCh. 5 - Prob. 41SGRCh. 5 - Prob. 44SGRCh. 5 - Prob. 46SGRCh. 5 - Prob. 47SGRCh. 5 - Prob. 48SGRCh. 5 - Prob. 49SGRCh. 5 - Prob. 50SGRCh. 5 - Prob. 51SGRCh. 5 - Prob. 52SGRCh. 5 - Prob. 53SGRCh. 5 - Prob. 15PTCh. 5 - Prob. 16PTCh. 5 - Prob. 19PTCh. 5 - Prob. 20PTCh. 5 - Prob. 21PTCh. 5 - Prob. 22PTCh. 5 - Prob. 23PTCh. 5 - Prob. 24PTCh. 5 - Prob. 1QCCh. 5 - Prob. 2QCCh. 5 - Prob. 3QCCh. 5 - Prob. 4QCCh. 5 - Prob. 5QCCh. 5 - Prob. 6QCCh. 5 - Prob. 7QCCh. 5 - Prob. 8QCCh. 5 - Prob. 9QCCh. 5 - Prob. 10QCCh. 5 - Prob. 11QCCh. 5 - Prob. 12QCCh. 5 - Prob. 13QCCh. 5 - Prob. 14QCCh. 5 - Prob. 15QCCh. 5 - Prob. 1MCQCh. 5 - Prob. 2MCQCh. 5 - Prob. 3MCQCh. 5 - Prob. 4MCQCh. 5 - Prob. 5MCQCh. 5 - Prob. 6MCQCh. 5 - Prob. 7MCQCh. 5 - Prob. 8MCQCh. 5 - Prob. 9MCQCh. 5 - Prob. 10MCQCh. 5 - Prob. 11MCQCh. 5 - Prob. 12MCQCh. 5 - Prob. 13MCQCh. 5 - Prob. 14MCQCh. 5 - Prob. 15MCQCh. 5 - Prob. 16MCQCh. 5 - Prob. 17MCQCh. 5 - Prob. 18MCQCh. 5 - Prob. 19MCQCh. 5 - Prob. 20MCQCh. 5 - Prob. 21MCQCh. 5 - Prob. 22MCQCh. 5 - Prob. 23MCQCh. 5 - Prob. 24MCQCh. 5 - Prob. 25MCQCh. 5 - Prob. 26MCQCh. 5 - Prob. 27MCQCh. 5 - Prob. 8SGRCh. 5 - Prob. 19SGRCh. 5 - Prob. 27SGRCh. 5 - Prob. 28SGRCh. 5 - Prob. 29SGRCh. 5 - Prob. 31SGRCh. 5 - Prob. 32SGRCh. 5 - Prob. 33SGRCh. 5 - Prob. 34SGRCh. 5 - Prob. 35SGRCh. 5 - Prob. 36SGRCh. 5 - Prob. 37SGRCh. 5 - Prob. 38SGRCh. 5 - Prob. 39SGRCh. 5 - Prob. 40SGRCh. 5 - Prob. 42SGRCh. 5 - Prob. 43SGRCh. 5 - Prob. 45SGRCh. 5 - Prob. 1PTCh. 5 - Prob. 2PTCh. 5 - Prob. 3PTCh. 5 - Prob. 4PTCh. 5 - Prob. 5PTCh. 5 - Prob. 6PTCh. 5 - Prob. 7PTCh. 5 - Prob. 8PTCh. 5 - Prob. 9PTCh. 5 - Prob. 10PTCh. 5 - Prob. 11PTCh. 5 - Prob. 12PTCh. 5 - Prob. 13PTCh. 5 - Prob. 14PTCh. 5 - Prob. 17PTCh. 5 - Prob. 18PTCh. 5 - Prob. 25PTCh. 5 - Prob. 26PTCh. 5 - Prob. 1STPCh. 5 - Prob. 2STPCh. 5 - Prob. 3STPCh. 5 - Prob. 4STPCh. 5 - Prob. 5STPCh. 5 - Prob. 6STPCh. 5 - Prob. 7STPCh. 5 - Prob. 8STPCh. 5 - Prob. 9STPCh. 5 - Prob. 10STPCh. 5 - Prob. 11STPCh. 5 - Prob. 12STPCh. 5 - Prob. 13STPCh. 5 - Prob. 14STPCh. 5 - Prob. 15STPCh. 5 - Prob. 16STPCh. 5 - Prob. 17STP
Additional Math Textbook Solutions
Find more solutions based on key concepts
Read about basic ideas of statistics in Common Core Standards for grades 3-5, and discuss why students at these...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Moving shadow A 5-foot-tall woman walks at 8 ft/s toward a streetlight that is 20 ft above the ground. What is ...
Calculus: Early Transcendentals (2nd Edition)
Explain the meaning of the term “statistically significant difference” in statistics terminology.
Intro Stats, Books a la Carte Edition (5th Edition)
The current value of car
Pre-Algebra Student Edition
CHECK POINT I Let p and q represent the following statements: p : 3 + 5 = 8 q : 2 × 7 = 20. Determine the truth...
Thinking Mathematically (6th Edition)
Genetics. In Exercises 15–20, refer to the accompanying table, which describes results from groups of 8 births ...
Elementary Statistics (13th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- 8) Solve the given system using the Gaussian Elimination process. 2x8y = 3 (-6x+24y = −6arrow_forward7) Solve the given system using the Gaussian Elimination process. (5x-4y = 34 (2x - 2y = 14arrow_forward33 (a) (b) Let A(t) = = et 0 0 0 cos(t) sin(t) 0-sin(t) cos(t)) For any fixed tЄR, find det(A(t)). Show that the matrix A(t) is invertible for any tЄ R, and find the inverse (A(t))¹.arrow_forward
- Use the infinite geometric sum to convert .258 (the 58 is recurring, so there is a bar over it) to a ratio of two integers. Please go over the full problem, specifying how you found r. Thank you.arrow_forwardH.w: Find the Eigen vectors for the largest Eigen value of the system X1+ +2x3=0 3x1-2x2+x3=0 4x1+ +3x3=0arrow_forwardneed help with 5 and 6 pleasearrow_forward
- 1) Given matrix A below, answer the following questions: a) What is the order of the matrix? b) What is the element a13? c) What is the element a₁₁? 4 -1arrow_forward[25 points] Given the vector let v = ER² and the collection of vectors ε = E-{)·()}-{☹) (9)} = {(A)·(9)}· B: = and C = · {(6)·(})}· answer the following question. (a) (b) (c) (d) (e) verify Verify is a basis for R² and find the coordinate [] of under ε. Verify B is a basis for R2 and find the coordinate []B of ʊ Verify C is a basis for R2 and find the coordinate []c of under ε. under ε. Find the change-of-basis matrix [I]+B from basis B to basis ε, and EE+BUB Find the change-of-basis matrix [I]B+ε from basis Ɛ to basis B, and verify [U]B= [] B+EVEarrow_forwardExplain the following terms | (a) linear span (b) dimension of vector space (c) linearly independent (d) linearly dependent (e) rank of matrix Aarrow_forward
- 3. Let u = 3/5 √ = and = -4/5 -() Define V span{ū, }. (a) (b) (c) Show that {u, } is orthonormal and forms a basis for V. Explicitly compute Projy w. Explicitly give a non-zero vector in V+.arrow_forwardIs 1.1 0.65 -3.4 0.23 0.4 -0.44 a basis for R3? You must explain your answer 0arrow_forwardFind the values of x and y in the following scalar multiplication. 8 2 x 1 3 || y = 9 LY_ Show Calculatorarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
2.1 Introduction to inequalities; Author: Oli Notes;https://www.youtube.com/watch?v=D6erN5YTlXE;License: Standard YouTube License, CC-BY
GCSE Maths - What are Inequalities? (Inequalities Part 1) #56; Author: Cognito;https://www.youtube.com/watch?v=e_tY6X5PwWw;License: Standard YouTube License, CC-BY
Introduction to Inequalities | Inequality Symbols | Testing Solutions for Inequalities; Author: Scam Squad Math;https://www.youtube.com/watch?v=paZSN7sV1R8;License: Standard YouTube License, CC-BY