
Concept explainers
The period of the moon’s orbit about the Earth is 27.3 days, but the average time between full moons is approximately 29.3 days. The difference is due to the motion of the Earth about the sun.
- a. Through what fraction of its total orbital period does the Earth move in one period of the moon’s orbit?
- b. Draw a sketch of the sun, the Earth, and the moon with the moon in the full moon condition. Then, sketch the position the moon would be in 27.3 days later, when the Earth is in its new position. If the moon is in the same position relative to Earth as it was 27.3 days earlier, is this a full moon?
- c. How much farther would the moon have to go to reach the full moon condition? Show that this represents approximately an extra two days.
(a)
The fraction of total orbital period of earth through which it move in one period of the moon’s orbit.
Answer to Problem 6SP
The fraction of total orbital period of earth through which it move in one period of the moon’s orbit is
Explanation of Solution
Given Info: The period of moon’s orbit about the Earth is
The period of orbital motion of Earth is
Write the expression for the fraction of total orbital period of Earth through which it move in one period of the moon’s orbit.
Conclusion:
Thus the fraction of total orbital period of earth through which it move in one period of the moon’s orbit is
(b)
The sketch of the sun, the Earth and the moon with the moon in the Full moon condition and also the sketch of the position where the moon would be in
Answer to Problem 6SP
The sketch of the sun, the Earth and the moon with the moon in the Full moon condition is
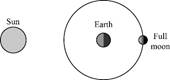
And the sketch of the position where the moon would be in
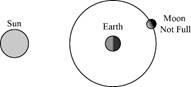
The moon will not be a full moon yet if the moon is in the same position relative to Earth as it was
Explanation of Solution
When the moon is full, it is on the opposite side of the Earth from the sun. The sketch of the sun, the Earth and the moon with the moon in the Full moon condition is plotted in figure 1.
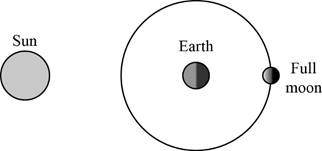
Figure 1
Write the expression for the angle through which the moon or the Earth advances in one day.
Substitute
Substitute
Find the net angle through the moon advances relative to the Earth.
Find the total angle advanced by the moon in
The moon is not full moon yet.
The sketch of the position where the moon would be in
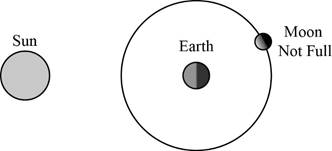
Figure 2
Conclusion:
Thus the sketch of the sun, the Earth and the moon with the moon in the Full moon condition is plotted in figure 1 and the sketch of the position where the moon would be in
(c)
The extra angle through which the moon have to go to reach the full moon condition and to show it represents approximately an extra two days.
Answer to Problem 6SP
The extra angle through which the moon have to go to reach the full moon condition is
Explanation of Solution
From part (b), the angle advanced by moon in
Find the time taken to cover
Conclusion:
Thus the extra angle through which the moon have to go to reach the full moon condition and to show it represents approximately an extra two days.
Want to see more full solutions like this?
Chapter 5 Solutions
Physics of Everyday Phenomena
- Solve and answer the question correctly please. Thank you!!arrow_forward་ The position of a particle is described by r = (300e 0.5t) mm and 0 = (0.3t²) rad, where t is in seconds. Part A Determine the magnitude of the particle's velocity at the instant t = 1.5 s. Express your answer to three significant figures and include the appropriate units. v = Value Submit Request Answer Part B ? Units Determine the magnitude of the particle's acceleration at the instant t = 1.5 s. Express your answer to three significant figures and include the appropriate units. a = Value A ? Unitsarrow_forwardSolve and answer the question correctly please. Thank you!!arrow_forward
- Solve and answer the question correctly please. Thank you!!arrow_forwardA spiral transition curve is used on railroads to connect a straight portion of the track with a curved portion. (Figure 1) Part A v = v₁ft/s 600 ft y = (106) x³ If the spiral is defined by the equation y = (106)³, where x and y are in feet, determine the magnitude of the acceleration of a train engine moving with a constant speed of v₁ = 30 ft/s when it is at point x = 600 ft. Express your answer to three significant figures and include the appropriate units. ? a = Value Unitsarrow_forwardsolve and answer the problem correctly please. Thank you!!arrow_forward
- Solve and answer the question correctly please. Thank you!!arrow_forwardSolve and answer the question correctly please. Thank you!!arrow_forwardWhen the motorcyclist is at A, he increases his speed along the vertical circular path at the rate of = (0.3t) ft/s², where t is in seconds. Take p = 360 ft. (Figure 1) Part A 60° Ρ B If he starts from rest at A, determine the magnitude of his velocity when he reaches B. Express your answer to three significant figures and include the appropriate units. v = Value Submit Request Answer ་ Part B ? Units If he starts from rest at A, determine the magnitude of his acceleration when he reaches B. Express your answer to three significant figures and include the appropriate units. 11 ? a = Value Unitsarrow_forward
- The car starts from rest at s = 0 and increases its speed at a₁ = 7 m/s². (Figure 1) Part A = 40 m Determine the time when the magnitude of acceleration becomes 20 m/s². Express your answer to three significant figures and include the appropriate units. ? t = Value Units Part B At what position s does this occur? Express your answer to three significant figures and include the appropriate units. s = Value Submit Request Answer ? Unitsarrow_forwardSolve and answer the question correctly please. Thank you!!arrow_forwardSolve and answer the question correctly please. Thank you!!arrow_forward
 An Introduction to Physical SciencePhysicsISBN:9781305079137Author:James Shipman, Jerry D. Wilson, Charles A. Higgins, Omar TorresPublisher:Cengage Learning
An Introduction to Physical SciencePhysicsISBN:9781305079137Author:James Shipman, Jerry D. Wilson, Charles A. Higgins, Omar TorresPublisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning AstronomyPhysicsISBN:9781938168284Author:Andrew Fraknoi; David Morrison; Sidney C. WolffPublisher:OpenStax
AstronomyPhysicsISBN:9781938168284Author:Andrew Fraknoi; David Morrison; Sidney C. WolffPublisher:OpenStax





