Chapter 5, Problem 2RE

(a)

To determine

The Riemann sum with four subintervals for the function at the right endpoints.

Answer to Problem 2RE

The Riemann sum with four subintervals at the right endpoint is 1.25.

Explanation of Solution

Given information:

The function as $f\left(x\right)=x^2-x$

The region lies between $x=0$ and $x=2$. Hence, the limits are $a=0$ and $b=2$.

Consider that the Number of rectangles as $n=4$.

The expression to find Riemann sum at the right endpoint $\left(R_n\right)$ is shown below:

$R_n={\displaystyle \sum _{i=1}^{n}f\left(x_i\right)\Delta x}$ (1)

Here, the height of sample at the right endpoint is $f\left(x_i\right)$ and the width is $\Delta x$.

Find the width $\left(\Delta x\right)$ using the relation:

$\Delta x=\frac{b-a}{n}$ (2)

Here, the upper limit is b, the lower limit is a, and the number of rectangles is n.

Substitute 2 for b, 0 for a, and 4 for n in Equation (2).

$\begin{array}{c}\Delta x=\frac{2-0}{4}\\ =0.5\end{array}$

Calculate $R_4$ using equation (1).

Substitute 4 for n in equation (1).

$R_4={\displaystyle \sum _{i=1}^{4}f\left(x_i\right)\Delta x}$ (3)

Substitute $i=1,2,3,\text{and 4}$ in equation (3).

$R_4=f\left(x_1\right)\Delta x+f\left(x_2\right)\Delta x+f\left(x_3\right)\Delta x+f\left(x_4\right)\Delta x$ (4)

At $x_1=0.5$ the value of $f\left(x_1\right)$ is,

$\begin{array}{c}f\left(x_1\right)={0.5}^2-0.5\\ =-0.25\end{array}$

At $x_2=1$ the value of $f\left(x_2\right)$ is,

$\begin{array}{c}f\left(x_2\right)=1^2-1\\ =0\end{array}$

At $x_3=1.5$ the value of $f\left(x_3\right)$ is,

$\begin{array}{c}f\left(x_3\right)={1.5}^2-1.5\\ =0.75\end{array}$

At $x_4=2$ the value of $f\left(x_4\right)$ is,

$\begin{array}{c}f\left(x_4\right)=2^2-2\\ =2\end{array}$

Draw four rectangles at the right endpoints based on the functions as shown in Figure (1).

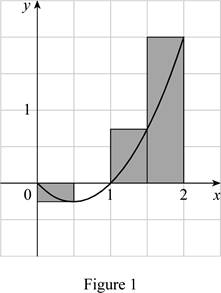
Substitute 0.5 for $\Delta x$, $-0.25$ for $f\left(x_1\right)$, 0 for $f\left(x_2\right)$, 0.75 for $f\left(x_3\right)$ and 2 for $f\left(x_4\right)$ in Equation (4).

$\begin{array}{c}{R}_6=\left(-0.25\right)\times 0.5+0\times 0.5+0.75\times 0.5+2\times 0.5\\ =1.25\end{array}$

Therefore, Riemann sum with four subintervals at the right endpoint is 1.25.

(b)

To determine

The value of the integral using the definition of a definite integral.

Answer to Problem 2RE

The value of the integral is $\left(\frac{2}{3}\right)$.

Explanation of Solution

Given information:

The function as ${\displaystyle \underset{0}{\overset{2}{\int }}\left(x^2-x\right)dx}$.

The region lies between $x=0$ and $x=2$.

The expression to find the value of the integral using the definition of a definite integral is shown below:

${\displaystyle \underset{0}{\overset{2}{\int }}\left(x^2-x\right)dx}=\underset{n\to \infty }{\lim }{\displaystyle \sum _{i=1}^{n}f\left(x_i\right)\Delta x}$ (5)

Substitute 2 for b and 0 for a in Equation (2).

$\begin{array}{c}\Delta x=\frac{b-a}{n}\\ =\frac{2-0}{n}\\ =\frac{2}{n}\end{array}$

To find the value of $x_i$ using the relation:

$x_i=a+i\Delta x$ (6)

Substitute 0 for a and $\frac{2}{n}$ for $\Delta x$ in equation (6).

$\begin{array}{c}{x}_i=0+i\frac{2}{n}\\ =\frac{2i}{n}\end{array}$

Substitute $\left(x_i^2-x_i\right)$ for $f\left(x_i\right)$ in equation (5).

${\displaystyle \underset{0}{\overset{2}{\int }}\left(x^2-x\right)dx}=\underset{n\to \infty }{\lim }{\displaystyle \sum _{i=1}^n\left(x_i^2-x_i\right)\Delta x}$ (7)

Substitute $\frac{2}{n}$ for $\Delta x$ and $\frac{2i}{n}$ for $x_i$ in equation (7).

$\begin{array}{c}{\displaystyle \underset{0}{\overset{2}{\int }}\left(x^2-x\right)dx}=\underset{n\to \infty }{\lim }{\displaystyle \sum _{i=1}^n\left(\frac{4i^2}{n^2}-\frac{2i}{n}\right)\left(\frac{2}{n}\right)}\\ =\underset{n\to \infty }{\lim }\left(\frac{2}{n}\right)\left(\frac{4}{n^2}{\displaystyle \sum _{i=1}^n{i}^2}-\frac{2}{n}{\displaystyle \sum _{i=1}^{n}i}\right)\\ =\underset{n\to \infty }{\lim }\left(\frac{8}{n^3}\times \frac{n\left(n+1\right)\left(2n+1\right)}{6}-\frac{4}{n^2}\times \frac{n\left(n+1\right)}{2}\right)\\ =\underset{n\to \infty }{\lim }\left(\frac{4}{3}\times \frac{\left(n+1\right)}{n}\times \frac{\left(2n+1\right)}{n}-2\times \frac{\left(n+1\right)}{n}\right)\end{array}$

$\begin{array}{c}{\displaystyle \underset{0}{\overset{2}{\int }}\left(x^2-x\right)dx}=\underset{n\to \infty }{\lim }\left(\frac{4}{3}\times \left(1+\frac{1}{n}\right)\times \left(2+\frac{1}{n}\right)-2\times \left(1+\frac{1}{n}\right)\right)\\ =\frac{4}{3}\times 1\times 2-2\times 1\\ =\frac{8-2\times 3}{3}\\ =\frac{2}{3}\end{array}$

Therefore, the value of the integral using the definition of a definite integral is $\left(\frac{2}{3}\right)$.

(c)

To determine

The value of the integral using the fundamental theorem.

Answer to Problem 2RE

The value of the integral is $\left(\frac{2}{3}\right)$.

Explanation of Solution

Given information:

The function as ${\displaystyle \underset{0}{\overset{2}{\int }}\left(x^2-x\right)dx}$.

The region lies between $x=0$ and $x=2$.

The expression to find the value of the integral using the fundamental theorem is shown below:

$\begin{array}{c}{\displaystyle \underset{0}{\overset{2}{\int }}\left(x^2-x\right)dx}={\left(\frac{1}{3}{x}^3-\frac{1}{2}{x}^2\right)}_0^2\\ =\left(\frac{1}{3}\times 2^3-\frac{1}{2}\times 2^2\right)-\left(\frac{1}{3}\times 0^3-\frac{1}{2}\times 0^2\right)\\ =\left(\frac{8}{3}-2\right)-0\\ =\frac{2}{3}\end{array}$

Therefore, the value of the integral using the fundamental theorem is $\left(\frac{2}{3}\right)$.

(d)

To determine

Draw a diagram to explain the geometric meaning of the integral.

Answer to Problem 2RE

The geometric meaning of the integral is ${\displaystyle \underset{0}{\overset{2}{\int }}\left(x^2-x\right)dx}=A_1-A_2$.

Explanation of Solution

Given information:

The function as ${\displaystyle \underset{0}{\overset{2}{\int }}\left(x^2-x\right)dx}$.

The region lies between $x=0$ and $x=2$.

Draw the diagram based on the functions as in part (a) as shown in Figure (2).

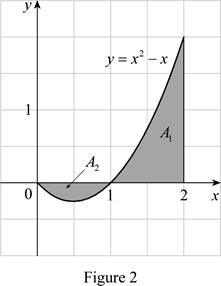
Refer Figure (1).

Upper portion of the diagram is represented as $A_1$ and lower portion of the diagram is represented as $A_2$.

The expression to find the geometric value of the function from the diagram as shown below:

${\displaystyle \underset{0}{\overset{2}{\int }}\left(x^2-x\right)dx}=A_1-A_2$

Therefore, the geometric mean of the integral $\left(A_1-A_2\right)$.