Precalculus

9th Edition

ISBN: 9780321716835

Author: Michael Sullivan

Publisher: Addison Wesley

See similar textbooks

Concept explainers

Polynomials

Try substituting 1. You will get 6 and not 0. Try other real values also. What About -2? Let us check.

Videos

Question

Chapter 5, Problem 10CR

(a)

To determine

The end behavior of polynomial $f(x)=3x4−15x3−12x2+60x$ .

(a)

Expert Solution

Answer to Problem 10CR

Solution:

The graph of the function $f(x)=3x4−15x3−12x2+60x$ behaves like $y=3x4$ for large value of $|x|$ .

Explanation of Solution

Given information:

The polynomial function $f(x)=3x4−15x3−12x2+60x$ .

Explanation:

The leading term of $f(x)$ is $3x4$ .

The graph of the function $f(x)=3x4−15x3−12x2+60x$ behaves like $y=3x4$ for large value of $|x|$ .

A polynomial function $f(x)$ with even degree and positive leading coefficient has end behavior $x→−∞, f(x)→∞ and x→∞, f(x)→∞$ .

The degree of polynomial $f(x)=3x4−15x3−12x2+60x$ is $4$ , which is even, and the leading coefficient is $3$ , which is positive.

Therefore, the end behavior of polynomial $f(x)=3x4−15x3−12x2+60x$ would be $x→−∞, f(x)→∞ and x→∞, f(x)→∞$ .

(b)

To determine

The $x$ and $y$ intercepts of the graph of $f(x)=3x4−15x3−12x2+60x$ .

(b)

Expert Solution

Answer to Problem 10CR

Solution:

The $y−$ intercept of the graph of $f(x)=3x4−15x3−12x2+60x$ is $(0, 0)_$ , and $x−$ intercepts are $(−2, 0), (0, 0),(2, 0),  (5, 0)_$ .

Explanation of Solution

Given information:

The polynomial function $f(x)=3x4−15x3−12x2+60x$ .

Explanation:

To find $y−$ intercept of the graph of $f(x)$ , substitute $x=0$ in $y=3x4−15x3−12x2+60x$ ,

$⇒ y=3(0)4−15(0)3−12(0)2+60(0)$

$⇒ y=0$

Therefore, the $y−$ intercept of the graph of $f(x)=3x4−15x3−12x2+60x$ is $(0, 0)$ .

To find $x-$ intercept of the graph of $f(x)$ , substitute $y=0$ in $y=3x4−15x3−12x2+60x$ ,

$⇒ 3x4−15x3−12x2+60x=0$

As $x$ is common factor, it can be factored out.

Using distributive property,

$⇒ 3x(x3−5x2−4x+20)=0$

$⇒ 3x=0, x3−5x2−4x+20=0$

$⇒ x=0, x3−5x2−4x+20=0$

The equation $x3−5x2−4x+20=0$ can be solved by factoring method.

Factor out $x2$ from first two terms and $−4$ from last two terms of equation $x3−5x2−4x+20=0$ .

Using distributive property,

$⇒x2(x−5)−4(x−5)=0$

$⇒(x2−4)(x−5)=0$

$⇒ x2−4=0, x−5=0$

Add $5$ to both sides of $x−5=0$ , and add $4$ to both sides of $x2−4=0$ ,

$⇒ x=5, x2=4$

Take square root on both sides of $x2=4$ ,

$x2=±4$

$⇒ x=−2, 2$

The $x−$ intercepts of function $f(x)$ are at $x=−2, 0, 2, 5$ .

Therefore, the $x−$ intercepts of function $f(x)$ are $(−2, 0), (0, 0),(2, 0),  (5, 0)$ .

(c)

To determine

The real zeros of polynomial $f(x)=3x4−15x3−12x2+60x$ with their multiplicities, and to determine if the graph crosses or touches the $x−$ axis at each intercept.

(c)

Expert Solution

Answer to Problem 10CR

Solution:

The real zeros of polynomial $f(x)=3x4−15x3−12x2+60x$ are $x=−2, 0, 2, 5_$ , with each zero havingmultiplicity $1$ , and the graph crosses $x−$ axis at each intercept.

Explanation of Solution

Given information:

The polynomial function $f(x)=3x4−15x3−12x2+60x$ .

Explanation:

The real zeros of polynomial $f(x)=3x4−15x3−12x2+60x$ are nothing but the $x−$ intercepts of $f(x)=3x4−15x3−12x2+60x$ .

From part (b), $x−$ intercepts of $f(x)=3x4−15x3−12x2+60x$ are $x=−2, 0, 2, 5$ .

Therefore, the real zeros of $f(x)=3x4−15x3−12x2+60x$ are $x=−2, 0, 2, 5$ .

The degree of $f(x)$ is $4$ , and there are $4$ distinct real zeros of $f(x)$ . Since each zero appears once, the multiplicity of each real zero is $1$ .

The multiplicity of each interceptis $1$ , which is odd. So, graph of $f(x)$ will cross $x−$ axis at each intercept.

(d)

To determine

The maximum number of turning points on the graph of polynomial $f(x)=3x4−15x3−12x2+60x$ .

(d)

Expert Solution

Answer to Problem 10CR

Solution:

The graph of polynomial $f(x)=3x4−15x3−12x2+60x$ has at most $3$ turning points.

Explanation of Solution

Given information:

The polynomial function $f(x)=3x4−15x3−12x2+60x$ .

Explanation:

If a polynomial function $f$ is of degree $n$ , then the graph of $f$ has at most $n−1$ turning points.

The degree of polynomial $f(x)=3x4−15x3−12x2+60x$ is $4$ . So, graph of $f(x)=3x4−15x3−12x2+60x$ will have at most $4−1=3$ number of turning points.

Therefore, the graph of polynomial $f(x)=3x4−15x3−12x2+60x$ has at most $3$ turning points.

(e)

To determine

To graph: The polynomial function $f(x)=3x4−15x3−12x2+60x$ .

(e)

Expert Solution

Explanation of Solution

Given information:

The polynomial function $f(x)=3x4−15x3−12x2+60x$ .

Graph:

The graph of the function $f(x)=3x4−15x3−12x2+60x$ behaves like $y=3x4$ for large value of $|x|$ .

The $x−$ intercepts of polynomial $f(x)=3x4−15x3−12x2+60x$ are $(−2, 0), (0, 0),(2, 0),  (5, 0)$ , and the $y−$ intercept is $(0, 0)$ .

Create a table of values as shown below:

$xy(x, y)−1−54(−1, −54)136(1, 36)3−90(3, −90)4−144(4, −144)$

Plot the $x$ and $y$ intercepts and points shown in the table on $xy$ plane, and draw the curve passing through all these points.

Therefore, the graph of $f(x)=3x4−15x3−12x2+60x$ is:

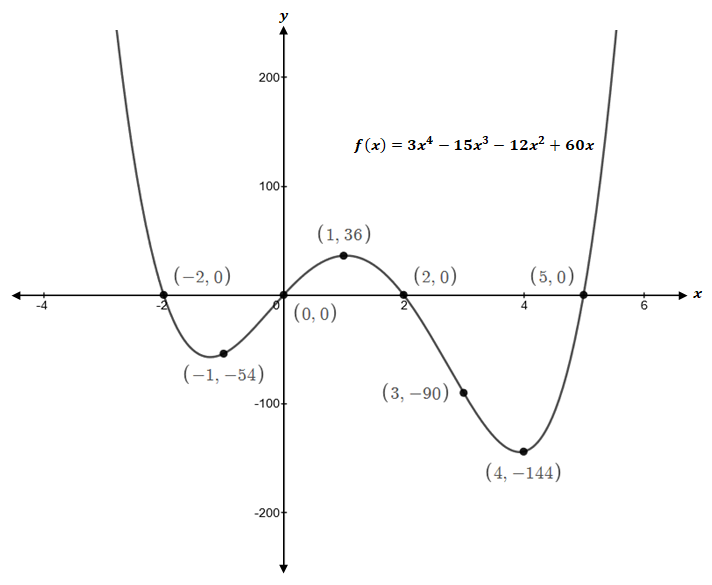
Precalculus, Chapter 5, Problem 10CR

Interpretation:

The graph represents the polynomial function $f(x)=3x4−15x3−12x2+60x$ , which has real zeros $x=−2, 0, 2, 5$ , and behaves the same as $y=3x4$ for large value of $|x|$ .

Chapter 5, Problem 9CR

Chapter 5, Problem 11CR

Chapter 5 Solutions

Precalculus

Ch. 5.1 - Find f( 3 ) if f( x )=4 x 2 +5x . (pp. 60-62)Ch. 5.1 - Find f(3x) if f(x)=42 x 2 . (pp. 60-62)Ch. 5.1 - Find the domain of the function f(x)= x 2 1 x 2 25...Ch. 5.1 - Prob. 4AYU Ch. 5.1 - Prob. 5AYU Ch. 5.1 - Prob. 6AYU Ch. 5.1 - In Problems 9 and 10, evaluate each expression...Ch. 5.1 - In Problems 9 and 10, evaluate each expression...Ch. 5.1 - In Problems 11 and 12, evaluate each expression...Ch. 5.1 - In Problems 11 and 12, evaluate each expression...

Additional Math Textbook Solutions

Find more solutions based on key concepts

Determine the number of vectors , such that each is either 0 or 1 and

A First Course in Probability (10th Edition)

Earnings A sociologist says, “Typically, men in the United States still earn more than women.” What does this s...

Introductory Statistics

Position, velocity, and acceleration Suppose the position of an object moving horizontally after t seconds is g...

Calculus: Early Transcendentals (2nd Edition)

Answer the following regarding the English alphabet. a. Determine the ratio of vowels to consonants. b. What is...

A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)

Express the limits in Exercises 1–8 as definite integrals. 1. , where P is a partition of [0, 2]

University Calculus: Early Transcendentals (4th Edition)

To find in which decades the population change was least.

Pre-Algebra Student Edition

Knowledge Booster

$Calculus$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.