
A particle moves according to a law of motion s = f(t), t ≥ 0, where t is measured in seconds and s in feet.
(a) Find the velocity at time t.
(b) What is the velocity after 1 second?
(c) When is the particle at rest?
(d) When is the particle moving in the positive direction?
(e) Find the total distance traveled during the first 6 seconds.
(f) Draw a diagram like Figure 2 to illustrate the motion of the particle.
(g) Find the acceleration at time t and after 1 second.
(h) Graph the position, velocity, and acceleration functions for 0 ≤ t ≤ 6.
(i) When is the particle speeding up? When is it slowing down?
FIGURE 2
f(t) = sin(πt/2)
(a)
To find: The velocity at time t.
Answer to Problem 3E
The velocity at time is
Explanation of Solution
Given:
The given equation is as below.
Calculation:
Calculate the velocity at time
Differentiate the equation (1) with respect to time.
Therefore, the velocity at time
(b)
To find: The velocity after 1 second.
Answer to Problem 3E
The velocity after 1 second is
Explanation of Solution
Calculate the velocity after 1 second.
Substitute 1 for
Therefore, the velocity after 1 second is
(c)
To find: The time when particle at rest.
Answer to Problem 3E
The particle never is at rest when
Explanation of Solution
Calculate the time when particle will be at rest.
The velocity will be zero, when the particle is at rest.
Substitute 0 for
Here,
(d)
To find: The particle moving in the positive direction.
Answer to Problem 3E
The velocity of particle always moves in positive direction when
Explanation of Solution
Calculate the time at which the particle will be moving in the positive direction.
The particle will move in positive direction when
(e)
To find: The total distance traveled during the first 6 seconds.
Answer to Problem 3E
The total distance travelled during first 6 second is
Explanation of Solution
Calculate the total distance traveled during first 6 seconds.
Here, the velocity changes from 1, 3 and 5 which appears in the interval of [0,6].
Substitute 1 and 0 for
The total distance travelled is as below.
Therefore, the total distance travelled during first 6 seconds is
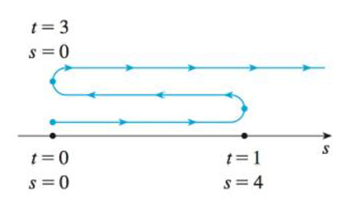
(f)
To find: The diagram to illustrate the motion of the particle.
Answer to Problem 3E
The diagram to illustrate the motion of particle is shown in the figure (1).
Explanation of Solution
Calculate the distance s using the expression.
Substitute 0 for
Calculate the value of t and s as shown in the table (1).
| t | s |
| 0 | 0 |
| 1 | 1 |
| 3 | -1 |
| 5 | 1 |
| 6 | 0 |
Show the diagram to illustrate the motion of the particle as shown below in figure (1).
(g)
To find: The acceleration at time t and after 1 second.
Answer to Problem 3E
The acceleration at time is
Explanation of Solution
Calculate the acceleration at any time t.
Differentiate the equation (2) with respect to t.
Therefore, the acceleration at any time is
Calculate the acceleration after 1 second.
Substitute 1 for
Therefore, the acceleration after 1 second is
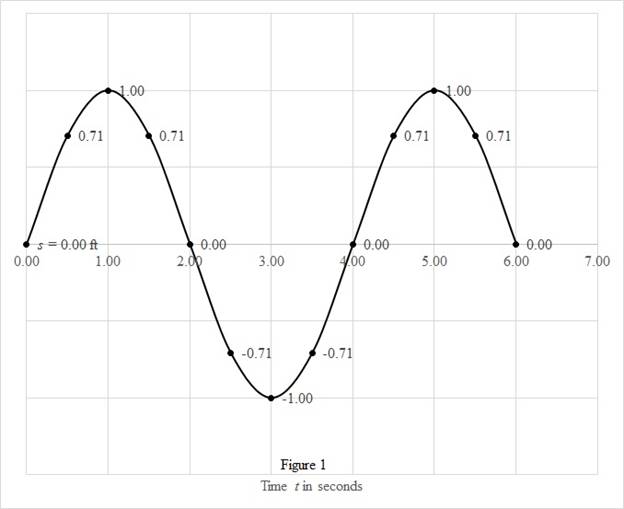
(h)
To sketch: The graph the position, velocity, and acceleration function for
Answer to Problem 3E
The position, velocity, and acceleration functions are plotted for time limits
Explanation of Solution
Calculate the position with respect to time using the formula.
Substitute 0 for
Similarly, calculate the remaining values.
Calculate the value of
| 0.00 | 0.00 |
| 0.50 | 0.71 |
| 1.00 | 1.00 |
| 1.50 | 0.71 |
| 2.00 | 0.00 |
| 2.50 | -0.71 |
| 3.00 | -1.00 |
| 3.50 | -0.71 |
| 4.00 | 0.00 |
| 4.50 | 0.71 |
| 5.00 | 1.00 |
| 5.50 | 0.71 |
| 6.00 | 0.00 |
Calculate the velocity using the expression.
Substitute 0 for
Similarly, calculate the remaining values.
Calculate the value of
| 0.00 | 1.57 |
| 0.50 | 1.11 |
| 1.00 | 0.00 |
| 1.50 | -1.11 |
| 2.00 | -1.57 |
| 2.50 | -1.11 |
| 3.00 | 0.00 |
| 3.50 | 1.11 |
| 4.00 | 1.57 |
| 4.50 | 1.11 |
| 5.00 | 0.00 |
| 5.50 | -1.11 |
| 6.00 | -1.57 |
Calculate the acceleration using the formula.
Substitute 0 for
Similarly, calculate the remaining values.
Calculate the value of
| 0.00 | 0.00 |
| 0.50 | -1.74 |
| 1.00 | -2.47 |
| 1.50 | -1.74 |
| 2.00 | 0.00 |
| 2.50 | 1.74 |
| 3.00 | 2.47 |
| 3.50 | 1.74 |
| 4.00 | 0.00 |
| 4.50 | -1.74 |
| 5.00 | -2.47 |
| 5.50 | -1.74 |
| 6.00 | 0.00 |
Draw the position as a function of time curve as shown in the Figure (2).

Draw the speed as a function of time curve as shown in the figure (3).

Draw the acceleration as a function of time curve as shown in the figure (4).

(i)
To find: The time when the particle is speeding up and slowing down.
Answer to Problem 3E
The acceleration is negative when the value of time is
Explanation of Solution
Calculate the time when particle speeding up and slowing down.
Substitute 0 for
Here, variable
Therefore, the acceleration is negative when the value of time is
Chapter 3 Solutions
Single Variable Calculus: Concepts and Contexts, Enhanced Edition
Additional Math Textbook Solutions
Graphical Approach To College Algebra
Precalculus: A Unit Circle Approach (3rd Edition)
Elementary Statistics: Picturing the World (7th Edition)
Elementary & Intermediate Algebra
College Algebra (Collegiate Math)
- Find the ONe sided limit lim 2x X-2 1-xarrow_forwardFor each function, identify all points of discontinuity and label them as removable, jump, or infinite. A) f(x) = x-4 (X+15)(x-4) B) f(x) = (x²-1 x ≤2 14-2x 2arrow_forwardFind the one sided limit 2 lim Flx) where f(x) = (x²-4_xarrow_forwardRequired information A telephone cable is clamped at A to the pole AB. The tension in the left-hand portion of the cable is given to be T₁ = 815 lb. T₁ 15° A 25° T₂ I B Using trigonometry, determine the corresponding magnitude of R. The corresponding magnitude of R is lb.arrow_forwardTwo forces are applied as shown to a hook support. The magnitude of P is 38 N. 50 N 25° DC a Determine the corresponding magnitude of R. The magnitude of R is N.arrow_forwardLet y(t) represent your retirement account balance, in dollars, after t years. Each year the account earns 7% interest, and you deposit 8% of your annual income. Your current annual income is $34000, but it is growing at a continuous rate of 2% per year. Write the differential equation modeling this situation. dy dtarrow_forwardDetermine Whether series converge or diverge if it converge what is the limit. $\{ \frac {(-1)^{n-2}n^{2}}{4+n^{3}}\} _{n=0}^{\infty }$arrow_forwardLet y(t) represent your retirement account balance, in dollars, after t years. Each year the account earns 7% interest, and you deposit 8% of your annual income. Your current annual income is $34000, but it is growing at a continuous rate of 2% per year. Write the differential equation modeling this situation. dy dtarrow_forward8:37 ▬▬▬▬▬▬▬▬▬ Ο Graph of f The figure shows the graph of a periodic function f in the xy-plane. What is the frequency of f? 0.5 B 2 C 3 D 8 3 of 6 ^ Oli Back Next apclassroom.collegeboard.orgarrow_forward2. The growth of bacteria in food products makes it necessary to time-date some products (such as milk) so that they will be sold and consumed before the bacteria count is too high. Suppose for a certain product that the number of bacteria present is given by f(t)=5000.1 Under certain storage conditions, where t is time in days after packing of the product and the value of f(t) is in millions. The solution to word problems should always be given in a complete sentence, with appropriate units, in the context of the problem. (a) If the product cannot be safely eaten after the bacteria count reaches 3000 million, how long will this take? (b) If t=0 corresponds to January 1, what date should be placed on the product?arrow_forward2.6 Applications: Growth and Decay; Mathematics of Finances 1. A couple wants to have $50,000 in 5 years for a down payment on a new house. (a) How much should they deposit today, at 6.2% compounded quarterly, to have the required amount in 5 years? (b) How much interest will be earned? (c) If they can deposit only $30,000 now, how much more will they need to complete the $50,000 after 5 years? Note, this is not 50,000-P3.arrow_forwardThe graph of f(x) is given below. Select each true statement about the continuity of f(x) at x = 1. Select all that apply: ☐ f(x) is not continuous at x = 1 because it is not defined at x = 1. ☐ f(x) is not continuous at x = 1 because lim f(x) does not exist. x+1 ☐ f(x) is not continuous at x = 1 because lim f(x) ‡ f(1). x+→1 ☐ f(x) is continuous at x = 1.arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning