Chapter 3.15, Problem 56AAP

Determine the Miller-Bravais direction indices of the basal plane of the vectors originating at the center of the lower basal plane and exiting at the midpoints between the principal planar axes.

To determine

The Miller-Bravais direction indices of the basal plane of the vectors originating at the center of the lower basal plane and existing at the midpoints between the principal planer axes.

Answer to Problem 56AAP

The Miller-Bravais Direction indices of the vector OA is $\left(\overline{3}034\right)$.

The Miller-Bravais Direction indices of the vector OB is $\left(\overline{3}304\right)$.

The Miller-Bravais Direction indices of the vector OC is $\left(03\overline{3}4\right)$.

The Miller-Bravais Direction indices of the vector OD is $\left(30\overline{3}4\right)$.

The Miller-Bravais Direction indices of the vector OE is $\left(3\overline{3}04\right)$

The Miller-Bravais Direction indices of the vector OF is $\left(0\overline{3}34\right)$

Explanation of Solution

The coordinates of intercept $\left(a_1,a_2,a_3\text{and}c\right)$ of the basal plane of the vectors originating at the center of the lower basal plane and existing at the midpoints between the principal planer axes of,

Directions OA is $\left(-\frac{1}{3},\infty ,\frac{1}{3},\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right).$

Directions OB is $\left(-\frac{1}{3},\frac{1}{3},\infty ,\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$.

Directions OC is $\left(\infty ,\frac{1}{3},-\frac{1}{3},\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$.

Directions OD is $\left(\frac{1}{3},\infty ,-\frac{1}{3},\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$.

Directions OE is $\left(\frac{1}{3},-\frac{1}{3},\infty ,\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$.

and directions OF is $\left(\infty ,-\frac{1}{3},\frac{1}{3},\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$.

Conclusion:

Direction vector originating at the centre of the lower basal plane and ending at the end point of the upper basal plane for a Hexagonal closed packed unit cell.

Figure below represent the Hexagonal closed packed unit cell.

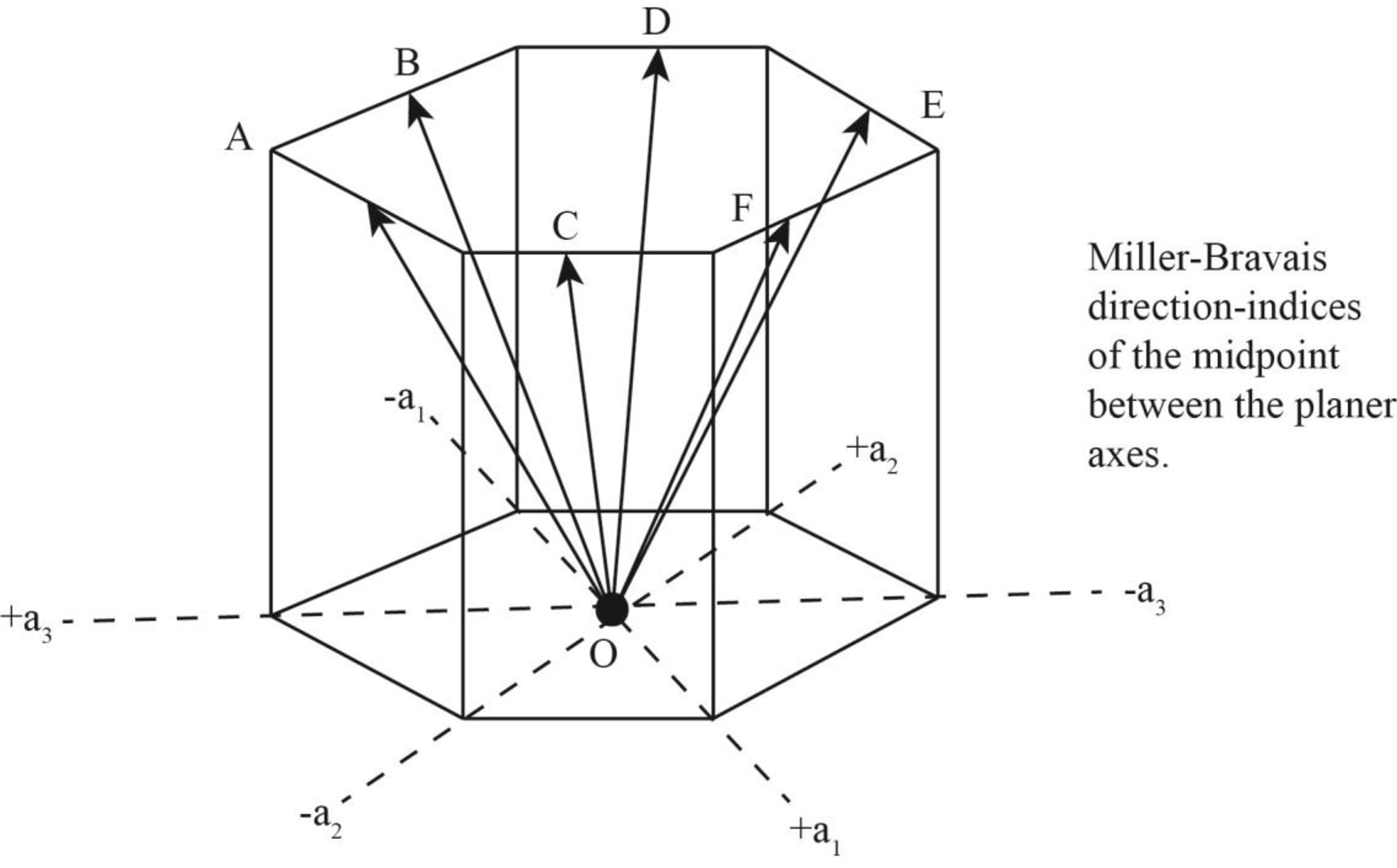
Figure-(1)

In the figure-(1) closed packing is Hexagonal closed packing and the direction vector of the planes are shown in figure-1. Here, the originating vector at the centre of the lower basal plane and ending at the end point of the upper basal plane for a Hexagonal closed packed unit cell is defined in the figure-(1).

Miller-Bravais direction Indices for the directions is tabulated below.

Direction vectors	Co-ordinates of intercepts	Reciprocal of intercept	Miller-Bravais Direction indices
OA	$\left(-\frac{1}{3},\infty ,\frac{1}{3},\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$	$\left(-3,0,3,4\right)$	$\left(\overline{3}034\right)$
OB	$\left(-\frac{1}{3},\frac{1}{3},\infty ,\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$	$\left(-3,3,\infty ,4\right)$	$\left(\overline{3}304\right)$
OC	$\left(\infty ,\frac{1}{3},-\frac{1}{3},\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$	$\left(0,3,-3,4\right)$	$\left(03\overline{3}4\right)$
OD	$\left(\frac{1}{3},\infty ,-\frac{1}{3},\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$	$\left(3,0,-3,4\right)$	$\left(30\overline{3}4\right)$
OE	$\left(\frac{1}{3},-\frac{1}{3},\infty ,\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$	$\left(3,-3,0,4\right)$	$\left(3\overline{3}04\right)$
OF	$\left(\infty ,-\frac{1}{3},\frac{1}{3},\left(\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\right)\right)$	$\left(0,-3,3,4\right)$	$\left(0\overline{3}34\right)$

Thus, the Miller-Bravais Direction indices of the vector OA is $\left(\overline{3}034\right)$.

Thus, the Miller-Bravais Direction indices of the vector OB is $\left(\overline{3}304\right)$.

Thus, the Miller-Bravais Direction indices of the vector OC is $\left(03\overline{3}4\right)$.

Thus, the Miller-Bravais Direction indices of the vector OD is $\left(30\overline{3}4\right)$.

Thus, the Miller-Bravais Direction indices of the vector OE is $\left(3\overline{3}04\right)$

The Miller-Bravais Direction indices of the vector OF is $\left(0\overline{3}34\right)$