
(a)
Interpretation:
The orbital which is higher in energy should be identified in the given pairs of hydrogen orbitals.
Concept Introduction:
The energies of orbitals in the hydrogen atom depend on the value of the principal quantum number (n). When n increases, energy also increases. For this reason, orbitals in the same shell have the same energy in spite of their subshell. The increasing order of energy of hydrogen orbitals is
1s < 2s = 2p < 3s = 3p = 3d < 4s = 4p = 4d = 4f
In the case of one 2s and three 2p orbitals in the second shell, they have the same energy. In the third shell, all nine orbitals (one 3s, three 3p and five 3d) have the same energy. All sixteen orbitals (one 4s, three 4p, five 4d and seven 4f) in the fourth shell have the same energy.
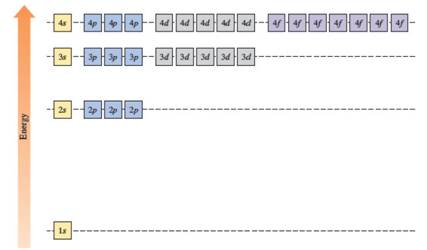
The energy levels of the different orbitals in hydrogen atom are easily explained by considering the given diagram. Here, each box represents one orbital. Orbitals with the same principal quantum number (n) have the same energy.
Principal Quantum Number (n)
The principal quantum number (n) assigns the size of the orbital and specifies the energy of an electron. If the value of n is larger, then the average distance of an electron in the orbital from the nucleus will be greater. Therefore the size of the orbital is large. The principal quantum numbers have the integral values of 1, 2, 3 and so forth and it corresponds to the quantum number in
To find: Identify the orbital which is higher in energy in the given pair 1s, 2s orbitals of hydrogen.
Find the value of ‘n’
(b)
Interpretation:
The orbital which is higher in energy should be identified in the given pairs of hydrogen orbitals.
Concept Introduction:
The energies of orbitals in the hydrogen atom depend on the value of the principal quantum number (n). When n increases, energy also increases. For this reason, orbitals in the same shell have the same energy in spite of their subshell. The increasing order of energy of hydrogen orbitals is
1s < 2s = 2p < 3s = 3p = 3d < 4s = 4p = 4d = 4f
In the case of one 2s and three 2p orbitals in the second shell, they have the same energy. In the third shell, all nine orbitals (one 3s, three 3p and five 3d) have the same energy. All sixteen orbitals (one 4s, three 4p, five 4d and seven 4f) in the fourth shell have the same energy.

The energy levels of the different orbitals in hydrogen atom are easily explained by considering the given diagram. Here, each box represents one orbital. Orbitals with the same principal quantum number (n) have the same energy.
Principal Quantum Number (n)
The principal quantum number (n) assigns the size of the orbital and specifies the energy of an electron. If the value of n is larger, then the average distance of an electron in the orbital from the nucleus will be greater. Therefore the size of the orbital is large. The principal quantum numbers have the integral values of 1, 2, 3 and so forth and it corresponds to the quantum number in Bohr’s model of the hydrogen atom. If all orbitals have the same value of ‘n’, they are said to be in the same shell (level). The total number of orbitals for a given n value is n2. As the value of ‘n’ increases, the energy of the electron also increases.
To find: Identify the orbital which is higher in energy in the given pair 2p, 3p orbitals of hydrogen
Find the value of ‘n’
(c)
Interpretation:
The orbital which is higher in energy should be identified in the given pairs of hydrogen orbitals.
Concept Introduction:
The energies of orbitals in the hydrogen atom depend on the value of the principal quantum number (n). When n increases, energy also increases. For this reason, orbitals in the same shell have the same energy in spite of their subshell. The increasing order of energy of hydrogen orbitals is
1s < 2s = 2p < 3s = 3p = 3d < 4s = 4p = 4d = 4f
In the case of one 2s and three 2p orbitals in the second shell, they have the same energy. In the third shell, all nine orbitals (one 3s, three 3p and five 3d) have the same energy. All sixteen orbitals (one 4s, three 4p, five 4d and seven 4f) in the fourth shell have the same energy.
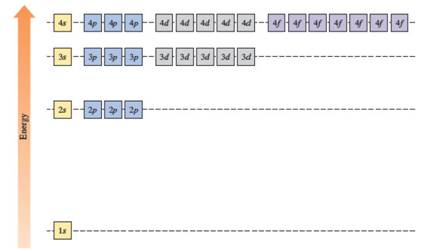
The energy levels of the different orbitals in hydrogen atom are easily explained by considering the given diagram. Here, each box represents one orbital. Orbitals with the same principal quantum number (n) have the same energy.
Principal Quantum Number (n)
The principal quantum number (n) assigns the size of the orbital and specifies the energy of an electron. If the value of n is larger, then the average distance of an electron in the orbital from the nucleus will be greater. Therefore the size of the orbital is large. The principal quantum numbers have the integral values of 1, 2, 3 and so forth and it corresponds to the quantum number in Bohr’s model of the hydrogen atom. If all orbitals have the same value of ‘n’, they are said to be in the same shell (level). The total number of orbitals for a given n value is n2. As the value of ‘n’ increases, the energy of the electron also increases.
To find: Identify the orbital which is higher in energy in the given pair 3dxy, 3dyz orbitals of hydrogen
Find the value of ‘n’
(d)
Interpretation:
The orbital which is higher in energy should be identified in the given pairs of hydrogen orbitals.
Concept Introduction:
The energies of orbitals in the hydrogen atom depend on the value of the principal quantum number (n). When n increases, energy also increases. For this reason, orbitals in the same shell have the same energy in spite of their subshell. The increasing order of energy of hydrogen orbitals is
1s < 2s = 2p < 3s = 3p = 3d < 4s = 4p = 4d = 4f
In the case of one 2s and three 2p orbitals in the second shell, they have the same energy. In the third shell, all nine orbitals (one 3s, three 3p and five 3d) have the same energy. All sixteen orbitals (one 4s, three 4p, five 4d and seven 4f) in the fourth shell have the same energy.

The energy levels of the different orbitals in hydrogen atom are easily explained by considering the given diagram. Here, each box represents one orbital. Orbitals with the same principal quantum number (n) have the same energy.
Principal Quantum Number (n)
The principal quantum number (n) assigns the size of the orbital and specifies the energy of an electron. If the value of n is larger, then the average distance of an electron in the orbital from the nucleus will be greater. Therefore the size of the orbital is large. The principal quantum numbers have the integral values of 1, 2, 3 and so forth and it corresponds to the quantum number in Bohr’s model of the hydrogen atom. If all orbitals have the same value of ‘n’, they are said to be in the same shell (level). The total number of orbitals for a given n value is n2. As the value of ‘n’ increases, the energy of the electron also increases.
To find: Identify the orbital which is higher in energy in the given pair 3s, 3d orbitals of hydrogen
Find the value of ‘n’
(e)
Interpretation:
The orbital which is higher in energy should be identified in the given pairs of hydrogen orbitals.
Concept Introduction:
The energies of orbitals in the hydrogen atom depend on the value of the principal quantum number (n). When n increases, energy also increases. For this reason, orbitals in the same shell have the same energy in spite of their subshell. The increasing order of energy of hydrogen orbitals is
1s < 2s = 2p < 3s = 3p = 3d < 4s = 4p = 4d = 4f
In the case of one 2s and three 2p orbitals in the second shell, they have the same energy. In the third shell, all nine orbitals (one 3s, three 3p and five 3d) have the same energy. All sixteen orbitals (one 4s, three 4p, five 4d and seven 4f) in the fourth shell have the same energy.

The energy levels of the different orbitals in hydrogen atom are easily explained by considering the given diagram. Here, each box represents one orbital. Orbitals with the same principal quantum number (n) have the same energy.
Principal Quantum Number (n)
The principal quantum number (n) assigns the size of the orbital and specifies the energy of an electron. If the value of n is larger, then the average distance of an electron in the orbital from the nucleus will be greater. Therefore the size of the orbital is large. The principal quantum numbers have the integral values of 1, 2, 3 and so forth and it corresponds to the quantum number in Bohr’s model of the hydrogen atom. If all orbitals have the same value of ‘n’, they are said to be in the same shell (level). The total number of orbitals for a given n value is n2. As the value of ‘n’ increases, the energy of the electron also increases.
To find: Identify the orbital which is higher in energy in the given pair 4f, 5s orbitals of hydrogen
Find the value of ‘n’
Want to see the full answer?
Check out a sample textbook solution
Chapter 3 Solutions
Chemistry: Atoms First V1
- The rate coefficient of the gas-phase reaction 2 NO2 + O3 → N2O5 + O2 is 2.0x104 mol–1 dm3 s–1 at 300 K. Indicate whether the order of the reaction is 0, 1, or 2.arrow_forward8. Draw all the resonance forms for each of the following molecules or ions, and indicate the major contributor in each case, or if they are equivalent. (4.5 pts) (a) PH2 سمةarrow_forward3. Assign absolute configuration (Rors) to each chirality center. a. H Nitz C. он b. 0 H-C. C H 7 C. ་-4 917-417 refs H 1つ ८ ડુ d. Но f. -2- 01 Ho -OH 2HNarrow_forward
- How many signals do you expect in the H NMR spectrum for this molecule? Br Br Write the answer below. Also, in each of the drawing areas below is a copy of the molecule, with Hs shown. In each copy, one of the H atoms is colored red. Highlight in red all other H atoms that would contribute to the same signal as the H already highlighted red. Note for advanced students: In this question, any multiplet is counted as one signal. Number of signals in the 'H NMR spectrum. For the molecule in the top drawing area, highlight in red any other H atoms that will contribute to the same signal as the H atom already highlighted red. If no other H atoms will contribute, check the box at right. No additional Hs to color in top molecule For the molecule in the bottom drawing area, highlight in red any other H atoms that will contribute to the same signal as the H atom already highlighted red. If no other H atoms will contribute, check the box at right. No additional Hs to color in bottom moleculearrow_forwardIn the drawing area below, draw the major products of this organic reaction: 1. NaOH ? 2. CH3Br If there are no major products, because nothing much will happen to the reactant under these reaction conditions, check the box under the drawing area instead. No reaction. Click and drag to start drawing a structure. ☐ : A คarrow_forwardPredict the major products of the following organic reaction: NC Δ ? Some important Notes: • Draw the major product, or products, of the reaction in the drawing area below. • If there aren't any products, because no reaction will take place, check the box below the drawing area instead. • Be sure to draw bonds carefully to show important geometric relationships between substituents. Note: if your answer contains a complicated ring structure, you must use one of the molecular fragment stamps (available in the menu at right) to enter the ring structure. You can add any substituents using the pencil tool in the usual way. Click and drag to start drawing a structure. Х аarrow_forward
- Predict the major products of this organic reaction. Be sure you use dash and wedge bonds to show stereochemistry where it's important. + ☑ OH 1. TsCl, py .... 文 P 2. t-BuO K Click and drag to start drawing a structure.arrow_forwardConsider this organic reaction: ( Draw the major products of the reaction in the drawing area below. If there won't be any major products, because this reaction won't happen at a significant rate, check the box under the drawing area instead. Click and drag to start drawing a structure. Х : а ค 1arrow_forwardIn the drawing area below, draw the major products of this organic reaction: If there are no major products, because nothing much will happen to the reactant under these reaction conditions, check the box under the drawing area instead. 1. NaH 2. CH3Br ? Click and drag to start drawing a structure. No reaction. : ☐ Narrow_forward
- + Predict the major product of the following reaction. : ☐ + ☑ ค OH H₂SO4 Click and drag to start drawing a structure.arrow_forwardConsider this organic reaction: ... OH CI Draw the major products of the reaction in the drawing area below. If there won't be any major products, because this reaction won't happen at a significant rate, check the box under the drawing area instead. ☐ No Reaction. Click and drag to start drawing a structure. : аarrow_forwardConsider the following reactants: Br Would elimination take place at a significant rate between these reactants? Note for advanced students: by significant, we mean that the rate of elimination would be greater than the rate of competing substitution reactions. yes O no If you said elimination would take place, draw the major products in the upper drawing area. If you said elimination would take place, also draw the complete mechanism for one of the major products in the lower drawing area. If there is more than one major product, you may draw the mechanism that leads to any of them. Major Products:arrow_forward
 Chemistry for Engineering StudentsChemistryISBN:9781337398909Author:Lawrence S. Brown, Tom HolmePublisher:Cengage Learning
Chemistry for Engineering StudentsChemistryISBN:9781337398909Author:Lawrence S. Brown, Tom HolmePublisher:Cengage Learning Chemistry: The Molecular ScienceChemistryISBN:9781285199047Author:John W. Moore, Conrad L. StanitskiPublisher:Cengage Learning
Chemistry: The Molecular ScienceChemistryISBN:9781285199047Author:John W. Moore, Conrad L. StanitskiPublisher:Cengage Learning General Chemistry - Standalone book (MindTap Cour...ChemistryISBN:9781305580343Author:Steven D. Gammon, Ebbing, Darrell Ebbing, Steven D., Darrell; Gammon, Darrell Ebbing; Steven D. Gammon, Darrell D.; Gammon, Ebbing; Steven D. Gammon; DarrellPublisher:Cengage Learning
General Chemistry - Standalone book (MindTap Cour...ChemistryISBN:9781305580343Author:Steven D. Gammon, Ebbing, Darrell Ebbing, Steven D., Darrell; Gammon, Darrell Ebbing; Steven D. Gammon, Darrell D.; Gammon, Ebbing; Steven D. Gammon; DarrellPublisher:Cengage Learning
 ChemistryChemistryISBN:9781305957404Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCostePublisher:Cengage Learning
ChemistryChemistryISBN:9781305957404Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCostePublisher:Cengage Learning Chemistry: An Atoms First ApproachChemistryISBN:9781305079243Author:Steven S. Zumdahl, Susan A. ZumdahlPublisher:Cengage Learning
Chemistry: An Atoms First ApproachChemistryISBN:9781305079243Author:Steven S. Zumdahl, Susan A. ZumdahlPublisher:Cengage Learning





