
Concept explainers
A piece of insulated wire is shaped into a figure eight as shown in Figure P23.12. For simplicity, model the two halves of the figure eight as circles. The radius of the upper circle is 5.00 cm and that of the lower circle is 9.00 cm. The wire has a uniform resistance per unit length of 3.00 Ω/m. A uniform magnetic field is applied perpendicular to the plane of the two circles, in the direction shown. The magnetic field is increasing at a constant rate of 2.00 T/s. Find (a) the magnitude and (b) the direction of the induced current in the wire.
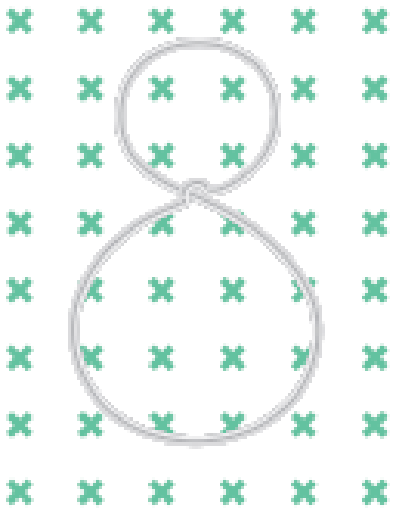
Figure P23.12
(a)
The magnitude of the induced current
Answer to Problem 12P
The induced current has a magnitude of
Explanation of Solution
The magnetic field in which the wire is placed is increasing and points into the page. This increasing flux is opposed by a magnetic field out of the page due to the counterclockwise current as a result of induced emf in the upper loop. Similarly, the lower loop also develops an induced emf.
The emf generated in the upper and the lower loops are in opposite directions as the two loops cross over. Write the equation for the net emf generated which is the difference between the emf generated in the two loops.
Here,
Here,
Here,
Here,
Multiply and divide the denominator of equation (V) with the length of the wire
The length of the wire is the sum of the circumferences of the lower loop and the upper loop. The circumference of the lower loop is
Conclusion:
Substitute
Therefore, the induced current has a magnitude of
(b)
The direction of the induced current
Answer to Problem 12P
The induced current is counterclockwise in the lower loop and clockwise in the upper loop.
Explanation of Solution
The lower loop has a larger area and hence the emf. As a result, the change in magnetic flux is also larger in the lower loop.
This increasing flux is opposed by a magnetic field out of the page due to the counterclockwise current as a result of induced emf in the upper loop.
Conclusion:
Therefore, the induced current is counterclockwise in the lower loop and clockwise in the upper loop.
Want to see more full solutions like this?
Chapter 23 Solutions
Principles of Physics: A Calculus-Based Text
- 7 Find the volume inside the cone z² = x²+y², above the (x, y) plane, and between the spheres x²+y²+z² = 1 and x² + y²+z² = 4. Hint: use spherical polar coordinates.arrow_forwardганм Two long, straight wires are oriented perpendicular to the page, as shown in the figure(Figure 1). The current in one wire is I₁ = 3.0 A, pointing into the page, and the current in the other wire is 12 4.0 A, pointing out of the page. = Find the magnitude and direction of the net magnetic field at point P. Express your answer using two significant figures. VO ΜΕ ΑΣΦ ? Figure P 5.0 cm 5.0 cm ₁ = 3.0 A 12 = 4.0 A B: μΤ You have already submitted this answer. Enter a new answer. No credit lost. Try again. Submit Previous Answers Request Answer 1 of 1 Part B X Express your answer using two significant figures. ΜΕ ΑΣΦ 0 = 0 ? below the dashed line to the right P You have already submitted this answer. Enter a new answer. No credit lost. Try again.arrow_forwardAn infinitely long conducting cylindrical rod with a positive charge λ per unit length is surrounded by a conducting cylindrical shell (which is also infinitely long) with a charge per unit length of −2λ and radius r1, as shown in the figure. What is σinner, the surface charge density (charge per unit area) on the inner surface of the conducting shell? What is σouter, the surface charge density on the outside of the conducting shell? (Recall from the problem statement that the conducting shell has a total charge per unit length given by −2λ.)arrow_forward
- A small conducting spherical shell with inner radius aa and outer radius b is concentric with a larger conducting spherical shell with inner radius c and outer radius d (Figure 1). The inner shell has total charge +2q, and the outer shell has charge −2q. What's the total charge on the inner surface of the small shell? What's the total charge on the outer surface of the small shell? What's the total charge on the inner surface of the large shell? What's the total charge on the outer surface of the large shell?arrow_forwardA small conducting spherical shell with inner radius a and outer radius b is concentric with a larger conducting spherical shell with inner radius cc and outer radius d (Figure 1). The inner shell has total charge +2q, and the outer shell has charge −2q. What is the direction of the electric field for b<r<c? Calculate the magnitude of the electric field for c<r<d. Calculate the magnitude of the electric field for r>d.arrow_forwardTICE D Conservation of Momentum 1. A 63.0 kg astronaut is on a spacewalk when the tether line to the shuttle breaks. The astronaut is able to throw a spare 10.0 kg oxygen tank in a direction away from the shuttle with a speed of 12.0 m/s, propelling the astronaut back to the shuttle. Assuming that the astronaut starts from rest with respect to the shuttle, find the astronaut's final speed with respect to the shuttle after the tank is thrown. 2. An 85.0 kg fisherman jumps from a dock into a 135.0 kg rowboat at rest on the west side of the dock. If the velocity of the fisherman is 4.30 m/s to the west as he leaves the dock, what is the final velocity of the fisher- man and the boat? 3. Each croquet ball in a set has a mass of 0.50 kg. The green ball, traveling at 12.0 m/s, strikes the blue ball, which is at rest. Assuming that the balls slide on a frictionless surface and all collisions are head-on, find the final speed of the blue ball in each of the following situations: a. The green…arrow_forward
- The 5.15 A current through a 1.50 H inductor is dissipated by a 2.15 Q resistor in a circuit like that in the figure below with the switch in position 2. 0.632/ C A L (a) 0.368/ 0+ 0 = L/R 2T 3r 4 (b) (a) What is the initial energy (in J) in the inductor? 0 t = L/R 2t (c) Эт 4t 19.89 ] (b) How long will it take (in s) the current to decline to 5.00% of its initial value? 2.09 S (c) Calculate the average power (in W) dissipated, and compare it with the initial power dissipated by the resistor. 28.5 1.96 x W X (ratio of initial power to average power)arrow_forwardImagine a planet where gravity mysteriously acts tangent to the equator and in the eastward directioninstead of radially inward. Would this force do work on an object moving on the earth? What is the sign ofthe work, and does it depend on the path taken? Explain by using the work integral and provide a sketch ofthe force and displacement vectors. Provide quantitative examples.arrow_forwardIf a force does zero net work on an object over a closed loop, does that guarantee the force is conservative? Explain with an example or counterexamplearrow_forward
- A futuristic amusement ride spins riders in a horizontal circle of radius 5 m at a constant speed. Thefloor drops away, leaving riders pinned to the wall by friction (coefficient µ = 0.4). What minimum speedensures they don’t slip, given g = 10 m/s²? Draw diagram (or a few) showing all forces, thevelocity of the rider, and their accelerationarrow_forwardYour RL circuit has a characteristic time constant of 19.5 ns, and a resistance of 4.60 MQ. (a) What is the inductance (in H) of the circuit? 0.00897 × H (b) What resistance (in MQ) should you use (instead of the 4.60 MQ resistor) to obtain a 1.00 ns time constant, perhaps needed for quick response in an oscilloscope? 8.97 * ΜΩarrow_forwardYour RL circuit has a characteristic time constant of 19.5 ns, and a resistance of 4.60 MQ. (a) What is the inductance (in H) of the circuit? H (b) What resistance (in MQ) should you use (instead of the 4.60 MQ resistor) to obtain a 1.00 ns time constant, perhaps needed for quick response in an oscilloscope? ΜΩarrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





