
An interesting pair of consecutive reactions involve the absorption of ethyl alcohol by the body, which is a first-order process, and the consequent oxidation of alcohol to acetaldehyde by liver alcohol dehydrogenase (LADH), which is a zeroth order process. The differential changes in the three states of ethanol can therefore be described as
which are slightly modified from equations
(a)
Interpretation:
The species
Concept introduction:
In the consecutive reactions the product of first reaction acts as the reactant for the second reaction and so on. The radioactive decays are one of the examples of the consecutive reactions. The simple two step consecutive reaction is shown below.
Answer to Problem 20.57E
The species
Explanation of Solution
The given consecutive reaction involves the absorption of ethyl alcohol by the body which is a first order reaction followed by the oxidation of ethyl alcohol to acetaldehyde in the presence of liver alcohol dehydrogenase (LADH).
The rate of change of concentration of the three species is given below.
From the above consecutive reaction it is concluded that
The species
(b)
Interpretation:
The integrated form for
Concept introduction:
In the consecutive reactions, the product of first reaction acts as the reactant for the second reaction and so on. The radioactive decays are one of the examples of the consecutive reactions. The simple two step consecutive reaction is shown below.
Answer to Problem 20.57E
The integrated form for
Explanation of Solution
The differential change in the concentration of
The integrated rate law for the first order reaction of
Substitute equation (2) in equation (1).
Integrate the above equation.
The integrated form for
(c)
Interpretation:
The integrated form for
Concept introduction:
In the consecutive reactions the product of first reaction acts as the reactant for the second reaction and so on. The radioactive decays are one of the examples of the consecutive reactions. The simple two step consecutive reaction is shown below.
Answer to Problem 20.57E
The integrated form for
Explanation of Solution
The differential change in the concentration of
The integrated form for
Substitute equation (4) in equation (3).
Integrate the above equation.
The above exponential function is integrated using the identity shown below.
On applying this identity on the exponential function the expression for the integrated form for
The integrated form for
(d)
Interpretation:
The graph for
Concept introduction:
In the consecutive reactions the product of first reaction acts as the reactant for the second reaction and so on. The radioactive decays are one of the examples of the consecutive reactions. The simple two step consecutive reaction is shown below.
Answer to Problem 20.57E
The graph for
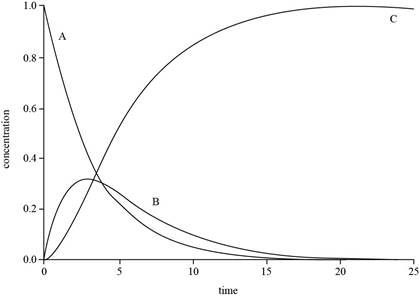
Explanation of Solution
The expressions for
The value of
The plot for
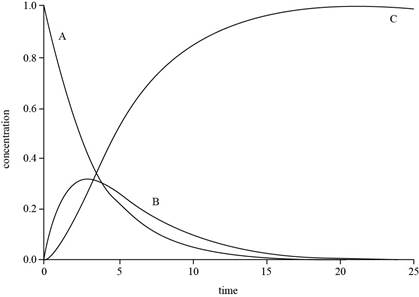
Figure 1
The concentration of
The graph for
Want to see more full solutions like this?
Chapter 20 Solutions
Bundle: Physical Chemistry, 2nd + Student Solutions Manual
- The data for the potential difference of a battery and its temperature are given in the table. Calculate the entropy change in J mol-1 K-1 (indicate the formulas used).Data: F = 96485 C mol-1arrow_forwardIn a cell, the change in entropy (AS) can be calculated from the slope of the E° vs 1/T graph. The slope is equal to -AS/R, where R is the gas constant. Is this correct?arrow_forwardUsing the Arrhenius equation, it is possible to establish the relationship between the rate constant (k) of a chemical reaction and the temperature (T), in Kelvin (K), the universal gas constant (R), the pre-exponential factor (A) and the activation energy (Ea). This equation is widely applied in studies of chemical kinetics, and is also widely used to determine the activation energy of reactions. In this context, the following graph shows the variation of the rate constant with the inverse of the absolute temperature, for a given chemical reaction that obeys the Arrhenius equation. Based on the analysis of this graph and the concepts acquired about the kinetics of chemical reactions, analyze the following statements: I. The activation energy (Ea) varies with the temperature of the system. II. The activation energy (Ea) varies with the concentration of the reactants. III. The rate constant (K) varies proportionally with temperature. IV. The value of the…arrow_forward
- In an electrolytic cell, indicate the formula that relates E0 to the temperature T.arrow_forward-- 14:33 A Candidate Identification docs.google.com 11. Compound A can transform into compound B through an organic reaction. From the structures below, mark the correct one: HO A تھے۔ די HO B ○ A) Compounds A and B are isomers. B) Both have the same number of chiral carbons. C) Compound A underwent an addition reaction of Cl2 and H2O to form compound B. D) Compound A underwent a substitution reaction forming the intermediate chlorohydrin to obtain compound B. E) Compound A underwent an addition reaction of Cl2 forming the chloronium ion and then added methanol to obtain compound B. 60arrow_forward-- 14:40 A Candidate Identification docs.google.com 13. The compound 1-bromo-hex-2-ene reacts with methanol to form two products. About this reaction, mark the correct statement: OCH3 CH3OH Br OCH3 + + HBr A B A) The two products formed will have the same percentage of formation. B) Product B will be formed by SN1 substitution reaction with the formation of an allylic carbocation. C) Product A will be formed by SN1 substitution reaction with the formation of a more stable carbocation than product B. D) Product A will be formed by an SN2 substitution reaction occurring in two stages, the first with slow kinetics and the second with fast kinetics. E) The two compounds were obtained by addition reaction, with compound B having the highest percentage of formation. 57arrow_forward
- -- ☑ 14:30 A Candidate Identification docs.google.com 10. Amoxicillin (figure X) is one of the most widely used antibiotics in the penicillin family. The discovery and synthesis of these antibiotics in the 20th century made the treatment of infections that were previously fatal routine. About amoxicillin, mark the correct one: HO NH2 H S -N. HO Figura X. Amoxicilina A) It has the organic functions amide, ester, phenol and amine. B) It has four chiral carbons and 8 stereoisomers. C) The substitution of the aromatic ring is of the ortho-meta type. D) If amoxicillin reacts with an alcohol it can form an ester. E) The structure has two tertiary amides. 62arrow_forwardThe environmental police of a Brazilian state received a report of contamination of a river by inorganic arsenic, due to the excessive use of pesticides on a plantation on the riverbanks. Arsenic (As) is extremely toxic in its many forms and oxidation states. In nature, especially in groundwater, it is found in the form of arsenate (AsO ₄ ³ ⁻ ), which can be electrochemically reduced to As ⁰ and collected at the cathode of a coulometric cell. In this case, Potentiostatic Coulometry (at 25°C) was performed in an alkaline medium (pH = 7.5 throughout the analysis) to quantify the species. What potential (E) should have been selected/applied to perform the analysis, considering that this is an exhaustive electrolysis technique (until 99.99% of all AsO ₄ ³ ⁻ has been reduced to As ⁰ at the electrode, or n( final) = 0.01% n( initial )) and that the concentration of AsO ₄ ³ ⁻ found in the initial sample was 0.15 mmol/L ? Data: AsO ₄ 3 ⁻ (aq) + 2 H ₂ O ( l ) + 2 e ⁻ → A s O ₂ ⁻ ( a…arrow_forward-- 14:17 15. Water-soluble proteins are denatured when there is a change in the pH of the environment in which they are found. This occurs due to the protonation and deprotonation of functional groups present in their structure. Choose the option that indicates the chemical bonds modified by pH in the protein represented in the following figure. E CH2 C-OH CH2 H₂C H₁C CH CH3 CH3 CH CH₂-S-S-CH₂- 910 H B -CH2-CH2-CH2-CH₂-NH3* −0—C—CH₂- ○ A) A, C e D. • В) Вес ○ C) DeE ○ D) B, De E ○ E) A, B e C 68arrow_forward
- Suppose sodium sulfate has been gradually added to 100 mL of a solution containing calcium ions and strontium ions, both at 0.15 mol/L. Indicate the alternative that presents the percentage of strontium ions that will have precipitated when the calcium sulfate begins to precipitate. Data: Kps of calcium sulfate: 2.4x10 ⁻ ⁵; Kps of strontium sulfate: 3.2x10 ⁻ ⁷ A) 20,2 % B) 36,6 % C) 62,9 % D) 87,5 % E) 98.7%arrow_forward14:43 A Candidate Identification docs.google.com 14. The following diagrams represent hypothetical membrane structures with their components numbered from 1 to 6. Based on the figures and your knowledge of biological membranes, select the correct alternative. | 3 5 || 人 2 500000 6 A) Structures 1, 3, 5, 2 and 4 are present in a constantly fluid arrangement that allows the selectivity of the movement ○ of molecules. Structure 4, present integrally or peripherally, is responsible for this selection, while the quantity of 6 regulates the fluidity. B) The membranes isolate the cell from the environment, but allow the passage of water-soluble molecules thanks to the presence of 2 and 3. The membrane in scheme is more fluid than that in 55arrow_forward12. Mark the correct statement about reactions a and b : a. Br + -OH Br b. + Br H₂O + Br -OH + H₂O A) The reactions are elimination reactions, with reaction "a" being of type E2 and reaction "b" being of type E1. B) Reaction "a" is an E2 type elimination occurring in one step and reaction "b" is an SN1 type substitution. C) Both reactions can result in the formation of carbocation, but in reaction "b" the most stable carbocation will be formed. D) Both reactions occur at the same rate ○ and have the same number of reaction steps. E) Reaction "b" is an E2 type elimination occurring in two steps and reaction "a" is an SN2 type substitution.arrow_forward
 Chemistry: The Molecular ScienceChemistryISBN:9781285199047Author:John W. Moore, Conrad L. StanitskiPublisher:Cengage Learning
Chemistry: The Molecular ScienceChemistryISBN:9781285199047Author:John W. Moore, Conrad L. StanitskiPublisher:Cengage Learning Chemistry: An Atoms First ApproachChemistryISBN:9781305079243Author:Steven S. Zumdahl, Susan A. ZumdahlPublisher:Cengage Learning
Chemistry: An Atoms First ApproachChemistryISBN:9781305079243Author:Steven S. Zumdahl, Susan A. ZumdahlPublisher:Cengage Learning Chemistry: Principles and PracticeChemistryISBN:9780534420123Author:Daniel L. Reger, Scott R. Goode, David W. Ball, Edward MercerPublisher:Cengage Learning
Chemistry: Principles and PracticeChemistryISBN:9780534420123Author:Daniel L. Reger, Scott R. Goode, David W. Ball, Edward MercerPublisher:Cengage Learning Chemistry & Chemical ReactivityChemistryISBN:9781337399074Author:John C. Kotz, Paul M. Treichel, John Townsend, David TreichelPublisher:Cengage Learning
Chemistry & Chemical ReactivityChemistryISBN:9781337399074Author:John C. Kotz, Paul M. Treichel, John Townsend, David TreichelPublisher:Cengage Learning Chemistry by OpenStax (2015-05-04)ChemistryISBN:9781938168390Author:Klaus Theopold, Richard H Langley, Paul Flowers, William R. Robinson, Mark BlaserPublisher:OpenStax
Chemistry by OpenStax (2015-05-04)ChemistryISBN:9781938168390Author:Klaus Theopold, Richard H Langley, Paul Flowers, William R. Robinson, Mark BlaserPublisher:OpenStax ChemistryChemistryISBN:9781305957404Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCostePublisher:Cengage Learning
ChemistryChemistryISBN:9781305957404Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCostePublisher:Cengage Learning





