
Introductory Mathematics for Engineering Applications
1st Edition
ISBN: 9781118141809
Author: Nathan Klingbeil
Publisher: WILEY
expand_more
expand_more
format_list_bulleted
Concept explainers
Textbook Question
Chapter 2, Problem 3P
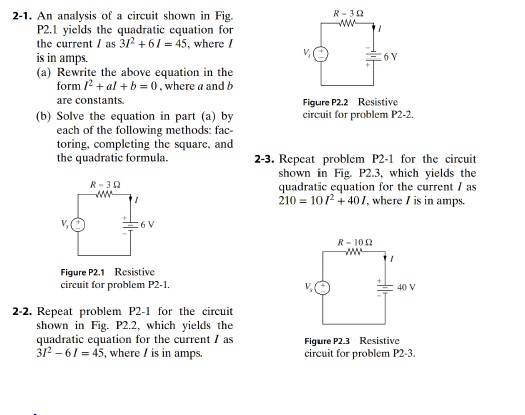
Repeat problem P2-I for the circuit shown in Fig. P2.3, which yields the
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Solve the linear system of equations attached using Gaussian elimination (not Gauss-Jordan) and back subsitution.
Remember that:
A matrix is in row echelon form if
Any row that consists only of zeros is at the bottom of the matrix.
The first non-zero entry in each other row is 1. This entry is called aleading 1.
The leading 1 of each row, after the first row, lies to the right of the leading 1 of the previous row.
7. Show that for R sufficiently large, the polynomial P(z) in Example 3, Sec. 5, satisfies
the inequality
|P(z)| R.
Suggestion: Observe that there is a positive number R such that the modulus of
each quotient in inequality (9), Sec. 5, is less than |an|/n when |z| > R.
9. Establish the identity
1-
1+z+z² +
2n+1
...
+z" =
1- z
(z1)
and then use it to derive Lagrange's trigonometric identity:
1
1+ cos cos 20 +... + cos no =
+
2
sin[(2n+1)0/2]
2 sin(0/2)
(0 < 0 < 2л).
Suggestion: As for the first identity, write S = 1+z+z² +...+z" and consider
the difference S - zS. To derive the second identity, write z =
eie in the first one.
Chapter 2 Solutions
Introductory Mathematics for Engineering Applications
Ch. 2 - An analysis of a circuit shown in Fig. P2.1 yields...Ch. 2 - Repeat problem P2-I for the circuit shown in Fig....Ch. 2 - Repeat problem P2-I for the circuit shown in Fig....Ch. 2 - The current flowing through the inductor shown in...Ch. 2 - The voltage across the capacitor shown in Fig....Ch. 2 - In the purely resistive circuit shown in Fig....Ch. 2 - Repeat problem P2-6 if R=1600 and R2=R1+500.Ch. 2 - The energy dissipated by a resistor shown in...Ch. 2 - The equivalent capacitance C of two capacitors...Ch. 2 - Repeat problem P2-9 if C=75F and C2=C1+200F
Ch. 2 - The equivalent capacitance C of three capacitors...Ch. 2 - The equivalent inductance L of three inductors...Ch. 2 - Repeat problem R2-12 if L=150 mH and L1=L2+400 mH.Ch. 2 - The equivalent inductance L of three inductors...Ch. 2 - A model rocket is launched in the vertical plane...Ch. 2 - The ball shown in Fig. P2.16 is dropped from a...Ch. 2 - At time t=0, a hail is thrown vertically from the...Ch. 2 - To springs connected in series shown in Fig. P2.18...Ch. 2 - The equivalent stiffness of a series-parallel...Ch. 2 - An assembly of three springs connected in series...Ch. 2 - Consider a capacitor C and an inductor L connected...Ch. 2 - Assume that the total reactance in problem P2-21...Ch. 2 - If L=0.5 H. C=0.005 F, and the total reactance in...Ch. 2 - When converting resistances connected in a ∆...Ch. 2 - When converting resistances connected in a...Ch. 2 - When converting resistances connected in a ∆...Ch. 2 - When converting resistances connected in a...Ch. 2 - The characteristic equation of a series RLC...Ch. 2 - The characteristic equation of a parallel RLC...Ch. 2 - The characteristic equation of a mass, spring, and...Ch. 2 - The perimeter of an rectangle shown in Fig. P2.31...Ch. 2 - A diver jumps off a diving board 2.0 m above the...Ch. 2 - A level pipeline is required to pass through a...Ch. 2 - A research group is using a drop test to measure...Ch. 2 - The modulus of elasticity (E ) is a measure of a...Ch. 2 - Consider the following reaction having an...Ch. 2 - Consider the following reaction having an...Ch. 2 - An engineering co-op wants to hire an asphalt...Ch. 2 - Repeat problem P2-38 if the total area of the new...Ch. 2 - A city wants to hire a contractor to build a...
Additional Math Textbook Solutions
Find more solutions based on key concepts
In the loan payment formula, assuming all other variables are constant, the monthly payment
Increases as P incr...
Using and Understanding Mathematics: A Quantitative Reasoning Approach (6th Edition)
Mathematical Connections Explain why a number and a numeral are considered different.
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
The solution of the given equation
Pre-Algebra Student Edition
NOTE: Write your answers using interval notation when appropriate.
CHECKING ANALYTIC SKILLS Fill in each blank ...
Graphical Approach To College Algebra
The following set of data is from sample of n=5: a. Compute the mean, median, and mode. b. Compute the range, v...
Basic Business Statistics, Student Value Edition
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Similar questions
- 8. Prove that two nonzero complex numbers z₁ and Z2 have the same moduli if and only if there are complex numbers c₁ and c₂ such that Z₁ = c₁C2 and Z2 = c1c2. Suggestion: Note that (i≤ exp (101+0) exp (01-02) and [see Exercise 2(b)] 2 02 Ꮎ - = = exp(i01) exp(101+0) exp (i 01 - 02 ) = exp(102). i 2 2arrow_forwardnumerical anaarrow_forwardNo chatgpt pls will upvote Already got wrong chatgpt answer .arrow_forward
- If you use any chatgpt will downvote.arrow_forward1) Compute the inverse of the following matrix. 0 1 1 A = 5 1 -1 2-3 -3arrow_forward2) Consider the matrix M = [1 2 3 4 5 0 2 3 4 5 00345 0 0 0 4 5 0 0 0 0 5 Determine whether the following statements are True or False. A) M is invertible. B) If R5 and Mx = x, then x = 0. C) The last row of M² is [0 0 0 0 25]. D) M can be transformed into the 5 × 5 identity matrix by a sequence of elementary row operations. E) det (M) 120 =arrow_forward
- 3) Find an equation of the plane containing (0,0,0) and perpendicular to the line of intersection of the planes x + y + z = 3 and x y + z = 5. -arrow_forward1) In the xy-plane, what type of conic section is given by the equation - √√√(x − 1)² + (y − 1)² + √√√(x + 1)² + (y + 1)² : - = 3?arrow_forward3) Let V be the vector space of all functions f: RR. Prove that each W below is a subspace of V. A) W={f|f(1) = 0} B) W = {f|f(1) = ƒ(3)} C) W={ff(x) = − f(x)}arrow_forward
- Translate the angument into symbole from Then determine whether the argument is valid or Invalid. You may use a truth table of, it applicable compare the argument’s symbolic form to a standard valid or invalid form. pot out of bed. The morning I did not get out of bed This moring Mat woke up. (1) Cidt the icon to view tables of standard vald and braild forms of arguments. Let prepresent."The morning Must woke up "and let a represent “This morning I got out of bed.” Seled the cared choice below and II in the answer ber with the symbolic form of the argument (Type the terms of your expression in the same order as they appear in the original expression) A. The argument is valid In symbolic form the argument is $\square $ B. The angunent is braid In symbolic form the argument is $\square $arrow_forwardWrite the prime factorization of 8. Use exponents when appropriate and order the factors from least to greatest (for example, 22.3.5). Submitarrow_forwardNo chatgpt pls will upvote Already got wrong chatgpt answer Plzarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
10 - Roots of polynomials; Author: Technion;https://www.youtube.com/watch?v=88YUeigknNg;License: Standard YouTube License, CC-BY