
An ideal gas is trapped inside a tube of uniform cross-sectional area sealed at one end as shown in Figure P19.49. A column of mercury separates the gas from the outside. The tube can be turned in a vertical plane. In Figure P19.49A, the column of air in the tube has length L1, whereas in Figure P19.49B, the column of air has length L2. Find an expression (in terms of the parameters given) for the length L3 of the column of air in Figure P19.49C, when the tube is inclined at an angle θ with respect to the vertical.
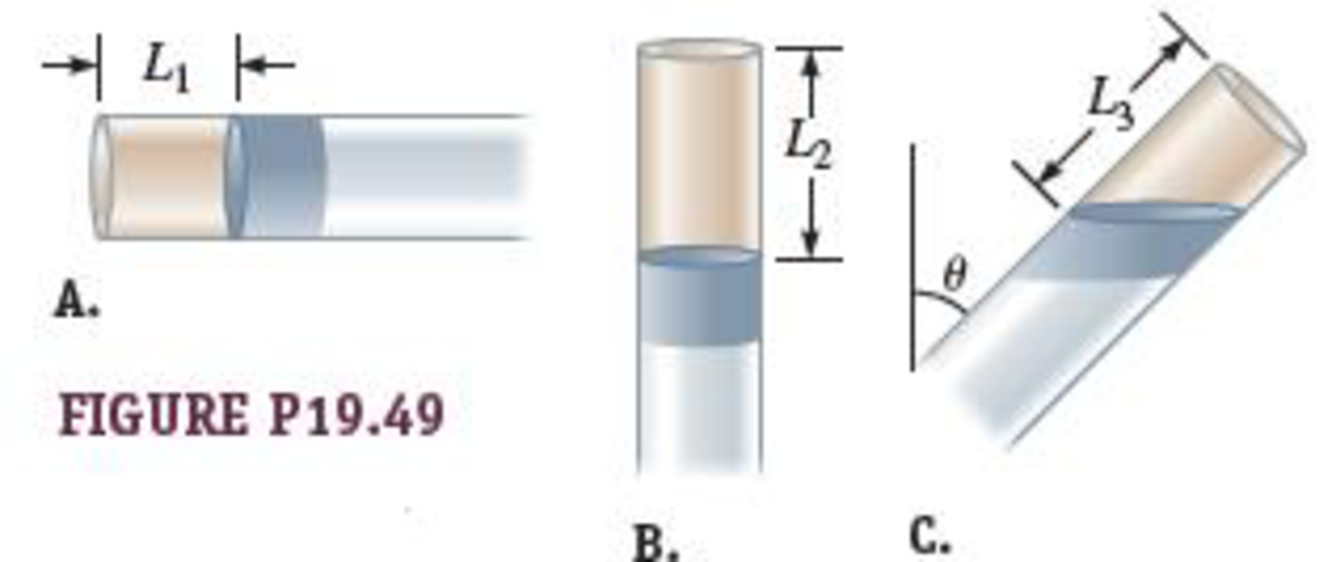
FIGURE P19.49
The expression for the length
Answer to Problem 49PQ
The expression for the length
Explanation of Solution
Three cases are depicted here. The first case in which the length of the air column is
In all the three cases mercury separates the air from outside. In all the three cases mercury should be in static equilibrium. The forces experienced by the mercury are the force due to the pressure inside the tube, the force from the atmospheric pressure, and the force due to the weight of the mercury. Here the tube is maintained at constant pressure. So apply Boyle’s law.
Consider Figure 1.
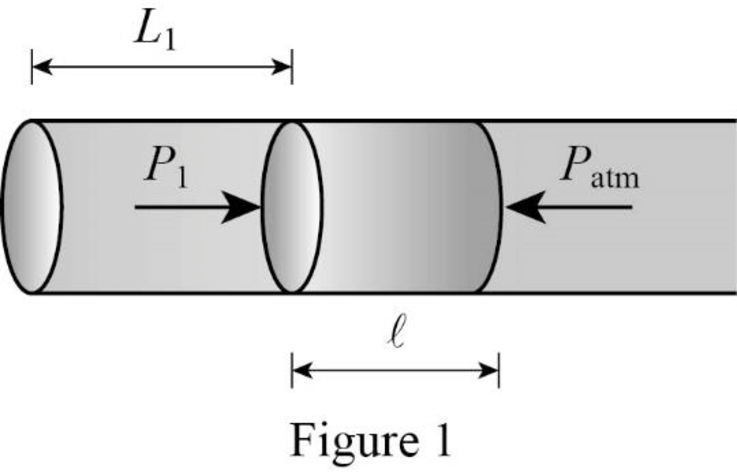
Weight of mercury acts perpendicular to the orientation of the tube. Thus the mercury is in equilibrium whenever the atmospheric pressure is equal to the pressure inside the tube.
Here,
Consider Figure 2.
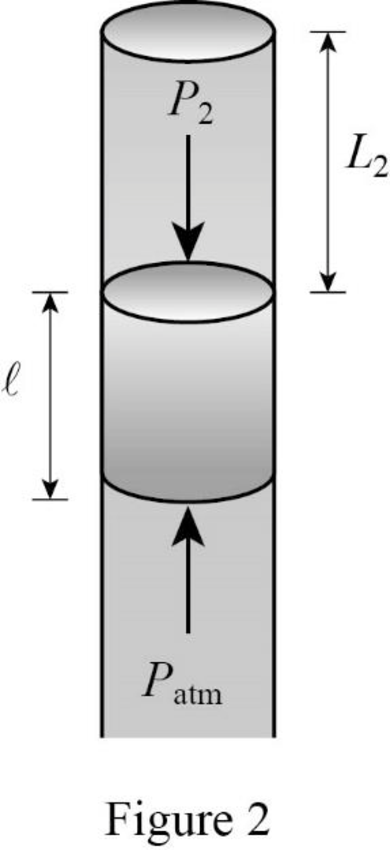
Here the outside atmospheric pressure is balanced by the sum of pressure in the tube due to the air column and the pressure due the weight of mercury.
Write the expression for the pressure due to the weight of mercury in position B.
Here,
Write the expression for the density of mercury.
Here,
Solve equation (III) for
Use expression (IV) in (II).
Here,
The atmospheric pressure at position B is balanced by the sum of pressures due to the weight of mercury, and pressure due to the column of air in the tube.
Here,
Consider the position 3.
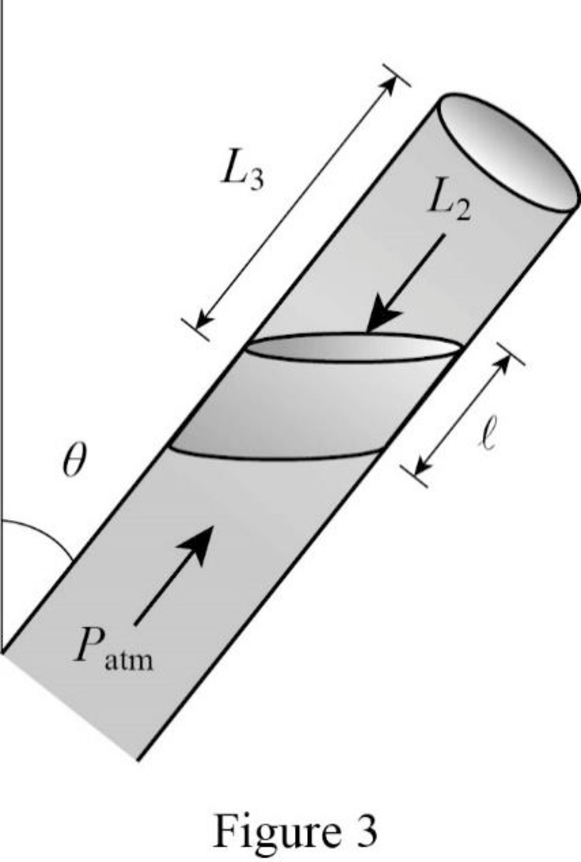
Here the pressure due to atmosphere is balanced by pressure inside the tube due to gas column denoted by
Write the expression for the vertical component of pressure due to the weight of mercury column.
Here,
Write the expression for the balance of pressure in the tube kept in position 3.
Since the temperature is constant, apply Boyle’s law. Boyle’s law states that the volume of a gas is directly proportional to the pressure of the gas at constant temperature.
Write the expression for Boyle’s law for case A and case B.
Write the expression for volume of air in tube 1.
Here,
Write the expression for volume of air in tube 2.
Here,
Use expression (XI), (VII), (XII) and (I) in expression (X).
Solve expression for
Write the expression for Boyle’s law for case A and case C.
Write the expression for volume of air in tube 3.
Here,
Use expression (XI), (XVI), (IX) and (I) in expression (XV).
Solve expression (XVII) for
Equate the right hand sides of equations (XIV) and (XVIII) and solve for
Solve expression (XIX) for
Conclusion:
Therefore, the expression for the length
Want to see more full solutions like this?
Chapter 19 Solutions
Physics for Scientists and Engineers: Foundations and Connections
- how would i express force in vector form I keep getting a single numberarrow_forwardplease help me solve this questions. show all calculations and a good graph too :)arrow_forwardWhat is the force (in N) on the 2.0 μC charge placed at the center of the square shown below? (Express your answer in vector form.) 5.0 με 4.0 με 2.0 με + 1.0 m 1.0 m -40 με 2.0 μCarrow_forward
- What is the force (in N) on the 5.4 µC charge shown below? (Express your answer in vector form.) −3.1 µC5.4 µC9.2 µC6.4 µCarrow_forwardAn ideal gas in a sealed container starts out at a pressure of 8900 N/m2 and a volume of 5.7 m3. If the gas expands to a volume of 6.3 m3 while the pressure is held constant (still at 8900 N/m2), how much work is done by the gas? Give your answer as the number of Joules.arrow_forwardThe outside temperature is 25 °C. A heat engine operates in the environment (Tc = 25 °C) at 50% efficiency. How hot does it need to get the high temperature up to in Celsius?arrow_forward
- Gas is compressed in a cylinder creating 31 Joules of work on the gas during the isothermal process. How much heat flows from the gas into the cylinder in Joules?arrow_forwardThe heat engine gives 1100 Joules of energy of high temperature from the burning gasoline by exhausting 750 Joules to low-temperature . What is the efficiency of this heat engine in a percentage?arrow_forwardL₁ D₁ L₂ D2 Aluminum has a resistivity of p = 2.65 × 10 8 2. m. An aluminum wire is L = 2.00 m long and has a circular cross section that is not constant. The diameter of the wire is D₁ = 0.17 mm for a length of L₁ = 0.500 m and a diameter of D2 = 0.24 mm for the rest of the length. a) What is the resistance of this wire? R = Hint A potential difference of AV = 1.40 V is applied across the wire. b) What is the magnitude of the current density in the thin part of the wire? Hint J1 = c) What is the magnitude of the current density in the thick part of the wire? J₂ = d) What is the magnitude of the electric field in the thin part of the wire? E1 = Hint e) What is the magnitude of the electric field in the thick part of the wire? E2 =arrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning





