
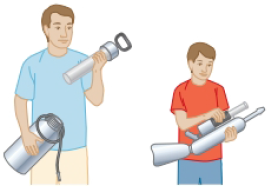
Your father and your younger brother are confronted with the same puzzle. Your father’s garden sprayer and your brother’s water cannon both have tanks with a capacity of 5.00 L (Fig. P18.18). Your father puts a negligible amount of concentrated fertilizer into his tank. They both pour in 4.00 L of water and seal up their tanks, so the tanks also contain air at atmospheric pressure. Next, each uses a hand-operated pump to inject more air until the absolute pressure in the tank reaches 2.40 atm. Now each uses his device to spray out water—not air—until the stream becomes feeble, which it does when the pressure in the tank reaches 1.20 atm. To accomplish spraying out all the water, each finds he must pump up the tank three times. Here is the puzzle: most of the water sprays out after the second pumping. The first and the third pumping-up processes seem just as difficult as the second but result in a much smaller amount of water coming out. Account for this phenomenon.
Figure P18.18
Want to see the full answer?
Check out a sample textbook solution
Chapter 19 Solutions
Physics for Scientists and Engineers, Technology Update (No access codes included)
- A ball of mass m = 1.95 kg is released from rest at a height h = 57.0 cm above a light vertical spring of force constant k as in Figure [a] shown below. The ball strikes the top of the spring and compresses it a distance d = 7.80 cm as in Figure [b] shown below. Neglecting any energy losses during the collision, find the following. т m a d T m b i (a) Find the speed of the ball just as it touches the spring. 3.34 m/s (b) Find the force constant of the spring. Your response differs from the correct answer by more than 10%. Double check your calculations. kN/marrow_forwardI need help with questions 1-10 on my solubility curve practice sheet. I tried to my best ability on the answers, however, i believe they are wrong and I would like to know which ones a wrong and just need help figuring it out.arrow_forwardQuestion: For a liquid with typical values a = 10-3K-¹ K = 10-4 bar-1 V=50 cm³ mol-1, Cp 200 J mol-1K-1, calculate the following quantities at 300 K and 1 bar for one mole of gas: 1. () P ән 2. (9) T 3. (V) T 4. (1) P 5. (9) T 6. Cv 7. (OF)Tarrow_forward
- A,B,C AND Darrow_forwardA bungee jumper plans to bungee jump from a bridge 64.0 m above the ground. He plans to use a uniform elastic cord, tied to a harness around his body, to stop his fall at a point 6.00 m above the water. Model his body as a particle and the cord as having negligible mass and obeying Hooke's law. In a preliminary test he finds that when hanging at rest from a 5.00 m length of the cord, his body weight stretches it by 1.55 m. He will drop from rest at the point where the top end of a longer section of the cord is attached to the bridge. (a) What length of cord should he use? Use subscripts 1 and 2 respectively to represent the 5.00 m test length and the actual jump length. Use Hooke's law F = KAL and the fact that the change in length AL for a given force is proportional the length L (AL = CL), to determine the force constant for the test case and for the jump case. Use conservation of mechanical energy to determine the length of the rope. m (b) What maximum acceleration will he…arrow_forward210. Sometimes the Helmholtz free energy F(T, V, N) divided by temperature, T, is an interesting quantity. For example, the quantity is proportional to the logarithm of the equilibrium constant or solubilities. A. Derive a relationship showing that Find the constant of proportionality. a F αυ ƏT T B. Suppose F(T) depends on temperature in the following way: F(T)=2aT²+bT. Find S(T) and U(T).arrow_forward
- choosing East (e) is not correct!arrow_forwarddisks have planes that are parallel and centered Three polarizing On a common axis. The direction of the transmission axis Colish dashed line) in each case is shown relative to the common vertical direction. A polarized beam of light (with its axis of polarization parallel to the horizontal reference direction) is incident from the left on the first disk with int intensity So = 790 W/m². Calculate the transmitted intensity if 81=28.0° O2-35.0°, and O3 = 40.0° w/m² horizontal Өз 02arrow_forwardA polarized light is incident on several polarizing disks whose planes are parallel and centered on common axis. Suppose that the transmission axis of the first polarizer is rotated 20° relative to the axis of polarization of the incident and that the transmission axis of each exis of light, additional analyzer is rotated 20° relative to the transmission axis the previous one. What is the minimum number of polarizer needed (whole number), so the transmitted light through all polarizing sheets has an Striking intensity that is less then 10% that the first polarizer?arrow_forward
- A high energy pulsed laser emits 1.5 nano second-long pulse of average power 1.80x10" W. The beam is cylindrical with 2.00 mm in radius. Determine the rms value of the B-field? -Tarrow_forwardA 23.0-mw (mill:-Watts) laser puts out a narrow cyclindrical beam 50 mm in diameter. What is the average N/C. rms E-field?arrow_forwardThe average intensity of light emerging from a polarizing sheet is. 0.550 W/m², and the average intensity of the horizontally polarized light incident on the sheet is 0.940 W/m². Determine the angle that the transmission axis of the polarizing sheet makes with the horizontalarrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning





