Chapter 18.3, Problem 18.142P

(a)

To determine

The rate of precession ${\dot{\varphi }}_0$ of the sphere in its initial position.

Answer to Problem 18.142P

The rate of precession ${\dot{\varphi }}_0$ of the sphere in its initial position is $\underset{\_}{\sqrt{\frac{15g}{11a}}}$.

Explanation of Solution

Given information:

The position of the sphere $\beta$ is zero.

The largest value of $\beta$ in the ensuing motion is $30°$.

Calculation:

Conservation of angular momentum about the Z and z axes:

The only external forces are acting in homogenous sphere is weight of the sphere and reaction at A. Hence, the angular momentum is conserved about the Z and z axes.

Choose the principal axes $A_{xyz}$ with taking y horizontal and pointing into the paper.

Write the expression for the angular velocity $\text{ω}$.

$\text{ω}=-\dot{\varphi }\cos \beta \text{i}+\dot{\beta }\text{j}+\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\text{k}$

The principal moment of inertia are  $I_x=I_y=m\left(\frac{2}{5}{a}^2+{\left(2a\right)}^2\right)$ and $I_z=\frac{2}{5}m{a}^2$.

Draw the Free body diagram of homogeneous sphere and the forces acting on it as in Figure (1).

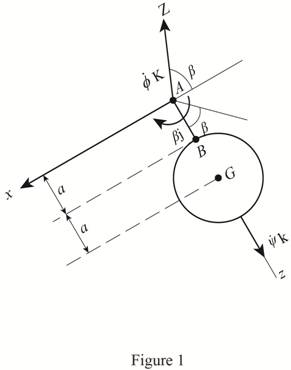
Write the expression for the angular momentum about point A.

${\text{H}}_A=I_x{\omega }_x\text{i}+I_y{\omega }_y\text{j}+I_z{\omega }_z\text{k}$

Substitute $m\left(\frac{2}{5}{a}^2+{\left(2a\right)}^2\right)$ for $I_x$, $-\dot{\varphi }\cos \beta$ for ${\omega }_x$, $m\left(\frac{2}{5}{a}^2+{\left(2a\right)}^2\right)$ for $I_y$, $\dot{\beta }$ for ${\omega }_y$, , $\frac{2}{5}m{a}^2$ for $I_z$, and $\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)$ for ${\omega }_z$.

$\begin{array}{c}{\text{H}}_A=m\left(\frac{2}{5}{a}^2+{\left(2a\right)}^2\right)\left(-\dot{\varphi }\cos \beta \right)\text{i}+m\left(\frac{2}{5}{a}^2+{\left(2a\right)}^2\right)\dot{\beta }\text{j}+\frac{2}{5}m{a}^2\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\text{k}\\ =-\frac{22}{5}m{a}^2\dot{\varphi }\cos \beta \text{i}+\frac{22}{5}m{a}^2\dot{\beta }\text{j}+\frac{2}{5}m{a}^2\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\text{k}\end{array}$

Consider ${\text{H}}_z=\text{constant}$ or ${\text{H}}_A\cdot \text{K}=\text{constant}$.

The scalar value of $\text{i}\cdot \text{K}=-\cos \beta$, $\text{j}\cdot \text{K}=0$, and $\text{k}\cdot \text{K}=-\sin \beta$.

Determine the conservation of angular momentum about fixed Z axis ${\text{H}}_A\cdot \text{K}$.

${\text{H}}_A\cdot \text{K}=\text{constant}$

Substitute $-\frac{22}{5}m{a}^2\dot{\varphi }\cos \beta \text{i}+\frac{22}{5}m{a}^2\beta \text{j}+\frac{2}{5}m{a}^2\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\text{k}$ for ${\text{H}}_A$, $-\cos \beta$ for $\left(\text{i}\cdot \text{K}\right)$, 0 for $\left(\text{j}\cdot \text{K}\right)$, and $-\cos \beta$ for $\left(\text{k}\cdot \text{K}\right)$.

$\begin{array}{l}\left\{\begin{array}{l}-\frac{22}{5}m{a}^2\dot{\varphi }\cos \beta \left(\text{i}\cdot \text{K}\right)+\frac{22}{5}m{a}^2\dot{\beta }\left(\text{j}\cdot \text{K}\right)+\\ \frac{2}{5}m{a}^2\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\left(\text{k}\cdot \text{K}\right)\end{array}\right\}=\text{constant}\\ \left\{\begin{array}{l}-\frac{22}{5}m{a}^2\dot{\varphi }\cos \beta \left(-\cos \beta \right)+\frac{22}{5}m{a}^2\dot{\beta }\left(0\right)+\\ \frac{2}{5}m{a}^2\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\left(-\sin \beta \right)\end{array}\right\}=\text{constant}\\ \left\{-\frac{22}{5}m{a}^2\dot{\varphi }\cos \beta \left(-\cos \beta \right)+\frac{2}{5}m{a}^2\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\left(-\sin \beta \right)\right\}=\text{constant}\end{array}$ (1)

Substitute ${\dot{\varphi }}_0$ for $\dot{\varphi }$, 0 for $\dot{\psi }$, and 0 for $\beta$ in Equation (1).

$\begin{array}{l}\left\{\begin{array}{l}-\frac{22}{5}m{a}^2{\dot{\varphi }}_0\cos 0\left(-\cos 0\right)+\\ \frac{2}{5}m{a}^2\left(0-{\dot{\varphi }}_0\sin 0\right)\left(-\sin 0\right)\end{array}\right\}=\text{constant}\\ \text{constant}=\frac{22}{5}m{a}^2{\dot{\varphi }}_0\end{array}$

Substitute $\frac{22}{5}m{a}^2{\dot{\varphi }}_0$ for constant in Equation (1).

$\begin{array}{l}-\frac{22}{5}m{a}^2\dot{\varphi }\cos \beta \left(-\cos \beta \right)+\frac{2}{5}m{a}^2\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\left(-\sin \beta \right)=\frac{22}{5}m{a}^2{\dot{\varphi }}_0\\ \frac{2}{5}m{a}^2\left[11\dot{\varphi }{\cos }^2\beta -\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\sin \beta \right]=\frac{2}{5}\times 11{\dot{\varphi }}_0\\ 11\dot{\varphi }{\cos }^2\beta -\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\sin \beta =11{\dot{\varphi }}_0\end{array}$ (2)

Determine the constant value using the angular momentum along z–axis.

$\begin{array}{l}{\text{H}}_z=\text{constant}\\ I_z{\omega }_z=\text{constant}\end{array}$

Substitute $\frac{2}{5}m{a}^2$ for $I_z$ and $\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)$ for ${\omega }_z$.

$\frac{2}{5}m{a}^2\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)=\text{constant}$ (3).

Substitute ${\dot{\varphi }}_0$ for $\dot{\varphi }$, 0 for $\dot{\psi }$, and 0 for $\beta$ in Equation (3).

$\begin{array}{l}\frac{2}{5}m{a}^2\left(0-{\dot{\varphi }}_0\sin \left(0\right)\right)=\text{constant}\\ \text{constant}=0\end{array}$

Substitute 0 for constant in Equation (3).

$\begin{array}{l}\frac{2}{5}m{a}^2\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)=\text{0}\\ \dot{\psi }-\dot{\varphi }\sin \beta =0\end{array}$

Substitute 0 for $\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)$ in Equation (2).

$\begin{array}{l}11\dot{\varphi }{\cos }^2\beta -\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)\sin \beta =11{\dot{\varphi }}_0\\ 11\dot{\varphi }{\cos }^2\beta -0\left(\sin \beta \right)=11{\dot{\varphi }}_0\\ \dot{\varphi }=\frac{11{\dot{\varphi }}_0}{11{\cos }^2\beta }\\ \dot{\varphi }={\dot{\varphi }}_0{\sec }^2\beta \end{array}$

Conservation of energy:

Determine the value of kinetic energy T.

$T=\frac{1}{2}\left(I_x{\omega }_x^2+I_y{\omega }_y^2+I_z{\omega }_z^2\right)$

Substitute $m\left(\frac{2}{5}{a}^2+{\left(2a\right)}^2\right)$ for $I_x$, $-\dot{\varphi }\cos \beta$ for ${\omega }_x$, $m\left(\frac{2}{5}{a}^2+{\left(2a\right)}^2\right)$ for $I_y$, $\dot{\beta }$ for ${\omega }_y$, , $\frac{2}{5}m{a}^2$ for $I_z$, and $\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)$ for ${\omega }_z$.

$\begin{array}{c}T=\left\{\frac{1}{2}\left(\begin{array}{l}m\left(\frac{2}{5}{a}^2+{\left(2a\right)}^2\right){\left(-\dot{\varphi }\cos \beta \right)}^2+m\left(\frac{2}{5}{a}^2+{\left(2a\right)}^2\right){\left(\dot{\beta }\right)}^2+\\ \frac{2}{5}m{a}^2{\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)}^2\end{array}\right)\right\}\\ =\frac{1}{2}\left(\frac{22}{5}m{a}^2{\dot{\varphi }}^2{\cos }^2\beta +\frac{22}{5}m{a}^2{\left(\dot{\beta }\right)}^2+\frac{2}{5}m{a}^2{\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)}^2\right)\end{array}$

Select the datum at $\beta =0$.

Determine the value of conservation of energy using the relation.

$T+V=\text{constant}$

Here, E is the constant and V is the potential energy.

Substitute $\frac{1}{2}\left(\frac{22}{5}m{a}^2{\dot{\varphi }}^2{\cos }^2\beta +\frac{22}{5}m{a}^2{\left(\beta \right)}^2+\frac{2}{5}m{a}^2{\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)}^2\right)$ for T and $-2mga\sin \beta$ for V.

$\left\{\frac{1}{2}\left(\begin{array}{l}\frac{22}{5}m{a}^2{\dot{\varphi }}^2{\cos }^2\beta +\frac{22}{5}m{a}^2{\left(\dot{\beta }\right)}^2+\\ \frac{2}{5}m{a}^2{\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)}^2\end{array}\right)-2mga\sin \beta \right\}=\text{constant}$ (4)

Substitute ${\dot{\varphi }}_0$ for $\dot{\varphi }$, 0 for $\beta$, 0 for $\dot{\beta }$, and 0 for $\dot{\psi }$ in Equation (4).

$\begin{array}{l}\left\{\frac{1}{2}\left(\begin{array}{l}\frac{22}{5}m{a}^2{\dot{\varphi }}_0^2{\cos }^{2}0+\frac{22}{5}m{a}^2{\left(0\right)}^2+\\ \frac{2}{5}m{a}^2{\left(0-{\dot{\varphi }}_0\sin 0\right)}^2\end{array}\right)-2mga\sin 0\right\}=\text{constant}\\ \text{constant}=\frac{11}{5}m{a}^2{\dot{\varphi }}_0^2\end{array}$

Substitute $\frac{11}{5}m{a}^2{\dot{\varphi }}_0^2$ for constant in Equation (4).

$\begin{array}{l}\left\{\frac{1}{2}\left(\begin{array}{l}\frac{22}{5}m{a}^2{\dot{\varphi }}^2{\cos }^2\beta +\frac{22}{5}m{a}^2{\left(\dot{\beta }\right)}^2+\\ \frac{2}{5}m{a}^2{\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)}^2\end{array}\right)-2mga\sin \beta \right\}=\frac{11}{5}m{a}^2{\dot{\varphi }}_0^2\\ \frac{1}{2}\times \frac{2}{5}m{a}^2\left(\begin{array}{l}\left(11{\dot{\varphi }}^2{\cos }^2\beta +11{\left(\dot{\beta }\right)}^2+{\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)}^2\right)\\ -10\frac{g}{a}\sin \beta \end{array}\right)=\frac{11}{5}m{a}^2{\dot{\varphi }}_0^2\\ \left(\begin{array}{l}\left(11{\dot{\varphi }}^2{\cos }^2\beta +11{\left(\dot{\beta }\right)}^2+{\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)}^2\right)\\ -10\frac{g}{a}\sin \beta \end{array}\right)=11{\dot{\varphi }}_0^2\end{array}$ (5)

Consider $\dot{\beta }=0$ for the maximum value of $\beta$.

Substitute ${\dot{\varphi }}_0{\sec }^2\beta$ for $\dot{\varphi }$, 0 for $\left(\dot{\psi }-\dot{\varphi }\sin \beta \right)$, and 0 for $\dot{\beta }$ in Equation (5).

$\begin{array}{l}11{\left({\dot{\varphi }}_0{\sec }^2\beta \right)}^2{\cos }^2\beta +11{\left(0\right)}^2-10\frac{g}{a}\sin \beta =11{\dot{\varphi }}_0^2\\ 11{\dot{\varphi }}_0^2{\sec }^4\beta \times \frac{1}{{\sec }^2\beta }-10\frac{g}{a}\sin \beta =11{\dot{\varphi }}_0^2\\ 11{\dot{\varphi }}_0^2{\sec }^2\beta -11{\dot{\varphi }}_0^2=10\frac{g}{a}\sin \beta \\ 11{\dot{\varphi }}_0^2\left(\frac{1-{\cos }^2\beta }{{\cos }^2\beta }\right)=10\frac{g}{a}\sin \beta \end{array}$

$\begin{array}{l}{\dot{\varphi }}_0^2\left(\frac{{\sin }^2\beta }{{\cos }^2\beta }\right)=\frac{10}{11}\frac{g}{a}\sin \beta \\ {\dot{\varphi }}_0^2=\frac{10}{11}\frac{g}{a}\sin \beta \times \frac{{\cos }^2\beta }{{\sin }^2\beta }\\ {\dot{\varphi }}_0^2=\frac{10}{11}\frac{g}{a}\frac{{\cos }^2\beta }{\sin \beta }\end{array}$ (6)

Substitute $30°$ for $\beta$ in Equation (6).

$\begin{array}{l}{\dot{\varphi }}_0^2=\frac{10}{11}\frac{g}{a}\frac{{\cos }^{2}30°}{\sin 30°}\\ {\dot{\varphi }}_0=\sqrt{\frac{15g}{11a}}\left(\frac{4}{3}\right)\end{array}$

Therefore, the rate of precession ${\dot{\varphi }}_0$ of the sphere in its initial position is $\underset{\_}{\sqrt{\frac{15g}{11a}}}$.

(b)

To determine

The rates of precession $\dot{\varphi }$ and the spin $\dot{\psi }$.

Answer to Problem 18.142P

The rate of precession $\dot{\varphi }$ is $2\underset{\_}{\sqrt{\frac{20g}{33a}}}$.

The rate of spin $\dot{\psi }$ is $\underset{\_}{\sqrt{\frac{20g}{33a}}}$.

Explanation of Solution

Given information:

The position of the sphere $\beta$ is zero.

The largest value of $\beta$ in the ensuing motion is $30°$.

Calculation:

Determine the rate of precession $\dot{\varphi }$ using the relation.

$\dot{\varphi }={\dot{\varphi }}_0{\sec }^2\beta$

Substitute $\sqrt{\frac{15g}{11a}}$ for ${\dot{\varphi }}_0$ and $30°$ for $\beta$.

$\begin{array}{c}\dot{\varphi }=\sqrt{\frac{15g}{11a}}{\sec }^{2}30°\\ =\sqrt{\frac{15g}{11a}}\times \frac{1}{{\cos }^{2}30°}\\ =\sqrt{\frac{15g}{11a}}\frac{4}{3}\\ =\sqrt{\frac{15\times 16g}{11\times 9a}}\\ =2\sqrt{\frac{20g}{33a}}\end{array}$

Therefore, the rate of precession $\dot{\varphi }$ is $2\underset{\_}{\sqrt{\frac{20g}{33a}}}$.

Determine the rate of spin $\dot{\psi }$ using the relation.

$\dot{\psi }-{\dot{\varphi }}_0\sin \beta =0$

Substitute $2\sqrt{\frac{20g}{33a}}$ for $\dot{\varphi }$ and $30°$ for $\beta$.

$\begin{array}{l}\dot{\psi }-2\sqrt{\frac{20g}{33a}}\sin 30°=0\\ \dot{\psi }=\sqrt{\frac{20g}{33a}}\end{array}$

The rate of spin $\dot{\psi }$ is $\underset{\_}{\sqrt{\frac{20g}{33a}}}$.