
The precession axis, rates of precession, and the spin rate of the satellite after the impact.
Answer to Problem 18.129P
The precession along x, y, and z axis
The precession rate
The spin rate
Explanation of Solution
Given information:
The weight of geostationary satellite (W) is 800 lb.
The angular velocity of the satellite
The weight of a meteorite
The travelling velocity of the meteorite
The distance value b is 20 in..
The radii of gyration of the satellite along x, y, and z direction (
Calculation:
Determine the mass of the satellite (m).
Here, g is the acceleration due to gravity.
Substitute 800 lb for W and
Determine the principal moment of inertia along x axis.
Substitute
Determine the principal moment of inertia along y axis.
Substitute
Determine the principal moment of inertia along z axis.
Substitute
Determine the mass of the meteorite
Substitute 6 oz for
Determine the initial moment of meteorite
Substitute
Consider that the position of the satellite mass center plus the meteorite is essentially that of the satellite alone.
Determine the position of the point B relative to the mass center.
Here, x is the horizontal distance and y is the vertical distance.
Substitute 42 in. for x and 20 in. for y.
The angular velocity of satellite before impact
The angular velocity of satellite before impact along x, y, and z axis is
Determine the angular momentum of satellite–meteorite system before impact
Substitute
Principle of impulse and momentum for satellite–meteorite system:
The value of moments about G is
The expression for
Determine the angular velocity about x axis.
Substitute
Determine the angular velocity about y axis.
Substitute
Determine the angular velocity about z axis.
Substitute
Determine the vector format of angular velocity using the relation;
Substitute
Determine the magnitude of the angular velocity
Substitute
Determine the magnitude of angular momentum
Substitute
Motion after impact:
The moment of inertia about x and y axis is equal, the body moves as an axisymmetrical body with the y axis as the symmetry axis.
The moment of inertia about the symmetry axis is
The moment of inertia about a transverse axis through G is
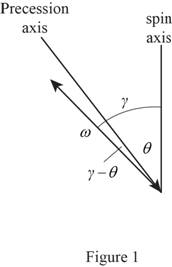
The precession is retrograde when the value of
Determine the angle
Substitute
Determine the angle
Substitute
Determine the angle
Substitute
Thus, the precession along x, y, and z axis
The angle
Determine the angle
Substitute
Determine the value of
Substitute
Draw the free body diagram of precession and spin axis as in Figure (1).
Draw the free body diagram of triangle of vector addition as in Figure (2).
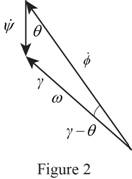
Write the relation between the angles using the sine law.
Determine the precession rate
Substitute
Therefore, the precession rate
Determine the rate of spin
Substitute
The precession is retrograde due to value of
Therefore, the spin rate
Want to see more full solutions like this?
Chapter 18 Solutions
Vector Mechanics for Engineers: Statics and Dynamics
- what is an air preheater, what are formulas, and their importance, define the diagram, and give me a script on how to explain the design of an air preheater, and how did values end up in that number. based on standardsarrow_forwardQf, Qa,Qm, Qcon,Qfg, Qbd, Qref,Qloss ( meaning, formula, percentage, and importance of higher value na qf, qa etc)arrow_forwardThe beam is supported by a fixed support at point C and a roller at point A. It also has an internal hinge at point B. The beam supports a point load at point D, a moment at point A and a distributed load on segment BC. a. calculate the support reactions at points A and C b. calculate the internal resultant loadings (N, V, M) at points E and F, which lies in the middle between points A and D P = 4 kip Ma = 5 kip-ft w1 = 3 kip/ft and w2 = 4 kip/ft a = 3 ftarrow_forward
- From the image of the pyramid, I want to find what s1 hat, s2 hat, and s3 hat are. I think s3 hat is just equal to e3 hat right? What about the others?arrow_forward(a) What kind of equation is it?(b) Is it linear or non-linear?(c) Is it a coupled system or uncoupled?arrow_forwardWhat kind of system is presented in Figure 2? Open loop or closed loop?arrow_forward
- What are the control hardware shown in the Figure?arrow_forwardQuestion 1. A tube rotates in the horizontal ry plane with a constant angular velocity w about the z-axis. A particle of mass m is released from a radial distance R when the tube is in the position shown. This problem is based on problem 3.2 in the text. R m 2R Figure 1 x a) Draw a free body diagram of the particle if the tube is frictionless. b) Draw a free body diagram of the particle if the coefficient of friction between the sides of the tube and the particle is = k = p. c) For the case where the tube is frictionless, what is the radial speed at which the particle leaves the tube? d) For the case where there is friction, derive a differential equation that would allow you to solve for the radius of the particle as a function of time. I'm only looking for the differential equation. DO NOT solve it. 1 e) If there is no friction, what is the angle of the tube when the particle exits? • Hint: You may need to solve a differential equation for the last part. The "potentially useful…arrow_forwardQuestion 2. A smooth uniform sphere of mass m and radius r is squeezed between two massless levers, each of length 1, which are inclined at an angle with the vertical. A mechanism at pivot point O ensures that the angles & remain the same at all times so that the sphere moves straight upward. This problem is based on Problem 3-1 in the text. P P r Figure 2 a) Draw appropriate freebody diagrams of the system assuming that there is no friction. b) Draw appropriate freebody diagrams of the system assuming that there is a coefficient of friction between the sphere and the right lever of μ. c) If a force P is applied between the ends of the levers (shown in the diagram), and there is no friction, what is the acceleration of the sphere when = 30°arrow_forward
- If you had a matrix A = [1 2 3; 4 5 6; 7 8 9] and a matrix B = [1 2 3], how would you cross multiply them i.e. what is the cross product of AxB. what would be the cross product of a dyadic with a vector?arrow_forwardProblem 3: The inertia matrix can be written in dyadic form which is particularly useful when inertia information is required in various vector bases. On the next page is a right rectangular pyramid of total mass m. Note the location of point Q. (a) Determine the inertia dyadic for the pyramid P, relative to point Q, i.e., 7%, for unit vectors ₁₁, 2, 3.arrow_forwardCan you solve for v? Also, what is A x uarrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





